Which expression is equivalent to (8×10−2) (2.4×10−3)?
Answers
The expression that is equivalent to the given expression, (8×10−2) (2.4×10−3), is 1.92 × 10⁻⁴
Determining an equivalent expressionFrom the question, we are to determine the expression that is equivalent to the given expression
From the given information,
The given expression is
(8×10−2) (2.4×10−3)
First, we will write this expression properly
The given expression written properly is
(8 × 10⁻²)(2.4 × 10⁻³)
Now, we will evaluate the expression
Evaluating the expression
(8 × 10⁻²)(2.4 × 10⁻³)
8 × 2.4 × 10⁻² × 10⁻³
19.2 × 10⁻⁵
= 1.92 × 10⁻⁴
Hence, the expression is 1.92 × 10⁻⁴
Learn more on Equivalent expression here: https://brainly.com/question/15775046
#SPJ1
Related Questions
Which of the following is not a real number?
A. √2
B. -2
C. √₁,
D. 0
Answers
Please help me thank you I appreciate it

Answers
Answer:
SRQ = 66 degrees
Step-by-step explanation:
QRT = 33 degrees
A bisector bisects angles evenly, which means that SRTis also 33 degrees. ADD these two together you get 66 degrees for this angle.
Answer:
<SRQ = 66
Step-by-step explanation:
bisect means to "cut" in half so the line pretty much separates half and half
the angles of a triangle is 2x,3x and 5x find the value of x
Answers
Here,
2x+3x+5x = 180 (sum of all angles of triangle are equal to 180)
or, 10x = 180
or, x = 180/10
x = 18
Therefore, the value of x is 18.
Hope this solution may help you☺
Answer:
2x + 3x + 5x = 180(sum of all the interior angles of a triangle is 180° )
10x = 180°
x=180/10
x = 18°
One red circle of an weighs 12 grams.write an inquality to represent the weight of one blue square
Answers
Inequality to represent the weight of one blue square:
s < 12
What is inequality?Inequalities are the mathematical expressions in which both sides are not equal. In inequality, unlike in equations, we compare two values. The equal sign in between is replaced by less than (or less than or equal to), greater than (or greater than or equal to), or not equal to sign.
Given,
One red circle of an weighs 12 grams
By the figure,
Weight of blue square is less than red circle
Let weight of blue square be s.
Then,
s < 12
Hence, s < 12 is the inequality to represent weight of blue square.
Learn more about inequality here:
https://brainly.com/question/30231190
#SPJ1

Peter has money in two savings accounts. one rate is 12% and the other 13%. if he $150 dollars more in the 13% account and the total interest is $54, how much is invested in each savings account?
Answers
Peter invested $138 in the 12% account and $288 in the 13% account.
Let x be the amount invested in the 12% account and y be the amount invested in the 13% account.
We know that the total amount invested is the sum of the two accounts, so: x + y = total amount invested
We also know that Peter has $150 more in the 13% account, so:
y = x + 150
The total interest earned is $54, which can be expressed as:
0.12x + 0.13y = 54
Substituting y with x + 150, we get:
0.12x + 0.13(x + 150) = 54
Simplifying and solving for x, we get:
0.12x + 0.13x + 19.5 = 54
0.25x = 34.5
x = 138
Therefore, Peter invested $138 in the 12% account and $288 in the 13% account.
To learn more about interests click here:
brainly.com/question/25857212
#SPJ1
Spring tides are extremely high and extremely low. This is created when the sun and moon pull together.
Answers
Answer:
yep true
Step-by-step explanation:
facts u know what u talking bout
On 1 April mazibane has R540, 00 in his credit card account. He buys a lounge suit for R8300, 00 on credit. There is no interest on the debit amount for the first month. Thereafter the interest is 16% per year calculated daily but compounded monthly. On 1 June Mazibane pays R5000 into the account.
How much must Mazibane pay into the account on 30 June to have no debt in the account
Answers
According to the information, we can infer that Mazibane must pay R3640 into the account on 30 June to have no debt.
How to calculate the amount Mazubane must pay on 30 June?To calculate the amount Mazibane must pay on 30 June to have no debt in the account, we need to consider the initial debt, the interest, and the previous payment.
Initial Debt:
On 1 April, Mazibane had a credit card debt of R8300.Interest Calculation:
The interest on the debt is 16% per year, calculated daily but compounded monthly. From 1 April to 1 June, a period of two months, there is no interest charged on the debt.Previous Payment:
On 1 June, Mazibane paid R5000 into the account.To determine the remaining debt on 1 June, we subtract the payment from the initial debt:
Remaining debt on 1 June = R8300 - R5000 = R3300.From 1 June to 30 June, a period of one month, interest is charged on the remaining debt.
To calculate the interest for one month, we use the formula:
Interest = Principal x (1 + (rate/100))^(time/12) - Principal,where the principal is the remaining debt, the rate is the monthly interest rate (16%/12), and the time is the number of months (1).
Interest for one month = R3300 x (1 + (16/100)/12)^(1/12) - R3300.To find the total debt on 30 June, we add the remaining debt on 1 June and the interest for one month:
Total debt on 30 June = R3300 + Interest for one month.To have no debt on 30 June, Mazibane must pay the total debt amount:
Mazibane must pay R3300 + Interest for one month on 30 June.Calculating the interest and summing up the values, we find that Mazibane must pay approximately R3640 into the account on 30 June to have no debt.
Learn more about debt in: https://brainly.com/question/31792485
#SPJ1
Ben flips a coin 25 times to simulate
students choosing tacos (H) or pizza (T) for
lunch. The coin lands on heads 14 times.
What is the experimental probability that
the next student will choose pizza?
Answers
Answer:
There's is a 99.99% the coin will land on tails
Step-by-step explanation:
the probability of landing on heads is 50%
to discover the probability of landing on heads 15 times in (50%)^15
which is 0.00003% chance so you subtract that total from 100% to receive 99.99% chance of receiving tails on the 15th flip
algebra 2: giving 20 points

Answers
The transformed function can be written as:
g(x) = 6*log₆(x) - 5
How to find the rule for g(x)?We start with the function f(x), if first we apply a vertical dilation of scale factor 6 we will get:
g(x) = 6*f(x)
If now we apply a translation of 5 units down, we will get:
g(x) = 6*f(x) - 5
We know that f(x)= log₆(x), then the transformed function is:
g(x) = 6*log₆(x) - 5
Learn more about transformations at:
https://brainly.com/question/4289712
#SPJ1
Triangle ABC is dilated about the origin to create triangle A′B′C′. Triangle ABC with vertices at A negative 6 comma negative 4, B negative 2 comma negative 4, and C negative 2 comma 2 and triangle A prime B prime C prime with vertices at A prime negative 9 comma negative 6, B prime negative 3 comma negative 6, and C prime negative 3 comma 3. Determine the scale factor used to create the image. two fifths 1.5 one half 2.5
Answers
Answer:
1.5
Step-by-step explanation:
To determine the scale factor used to create the image, we can compare the distances between corresponding vertices of the two triangles.
Let's start by finding the distance between A and B in triangle ABC:
distance AB = sqrt[(x_B - x_A)^2 + (y_B - y_A)^2]
= sqrt[(-2 - (-6))^2 + (-4 - (-4))^2]
= sqrt[16 + 0]
= 4
Now let's find the distance between A' and B' in triangle A'B'C':
distance A'B' = sqrt[(x_B' - x_A')^2 + (y_B' - y_A')^2]
= sqrt[(-3 - (-9))^2 + (-6 - (-6))^2]
= sqrt[36 + 0]
= 6
To find the scale factor, we can divide the distance between corresponding vertices in the image triangle by the distance between the corresponding vertices in the original triangle:
scale factor = distance A'B' / distance AB
= 6 / 4
= 1.5
Therefore, the scale factor used to create the image is 1.5.
To find the scale factor of a dilation, one can divide a side length of the enlarged shape by the corresponding side length of the original shape. In this case, the scale factor from triangle ABC to A'B'C' is 6/4 = 1.5.
Explanation:In Mathematics, we can find the scale factor of a dilation by comparing corresponding distances in the original shape and its image. The scale factor can be found by dividing a side length of the enlarged shape (triangle A′B′C′) by the corresponding side length of the original shape (triangle ABC).
For example, the distance between points A and B in the original triangle ABC is 4 units (-2 - (-6) = 4), and the distance between points A′ and B′ in the enlarged triangle A′B′C′ is 6 units (-3 - (-9) = 6). So, the scale factor from ABC to A'B'C' is 6/4 = 1.5.
Therefore, the scale factor used to create the image of triangle A'B'C' from triangle ABC is 1.5.
Learn more about scale factor here:https://brainly.com/question/35553153
#SPJ2
Two angles lie along a straight line. If m∠A is five times the sum of m∠B plus 7.2°, what is m∠B?
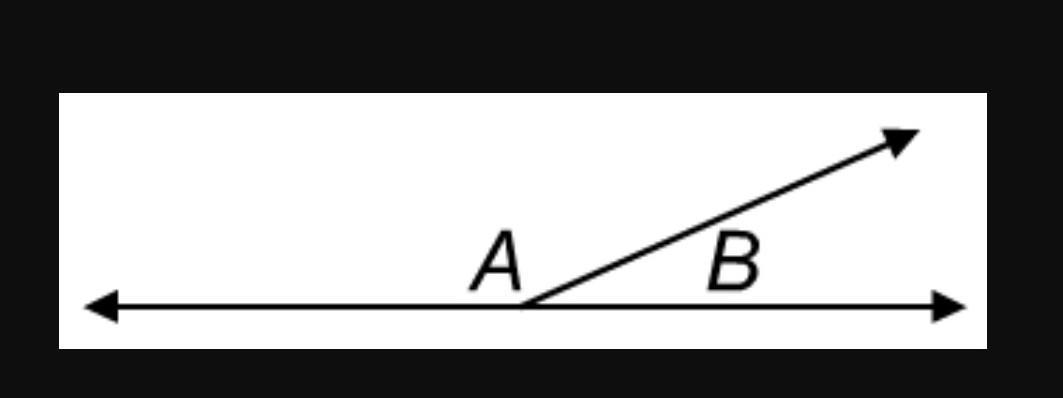
Answers
As a result, angle B has a 24 degree measure as the total of the two angles, which are along a straight line, is 180 degrees.
what is angle ?Thus according their size or measurement, angles can be categorised. An oblique angle is larger than 90 degrees but far less than 180 degrees, a straight angle is exactly 90 degree, a right angle is turned 90 degrees, and an acute angle is less than 90 degrees. Reflex angles are angles that are higher than 180o but a little less than 360 degrees, and complete angles are angles that measure exactly 360 degrees. Geometry, trigonometry, physics, engineering, and many other branches of mathematics and science all make use of angles.
given
The total of the two angles, which are along a straight line, is 180 degrees. Let's refer to the angle B's measurement as x.
The information provided in the problem can then be used to create an equation as follows:
m∠A = 5(m∠B + 7.2°)
Due to the fact that the two angles are perpendicular to one another, we may replace mA with 180 - mB:
180 - m∠B = 5(m∠B + 7.2°)
The right side is being widened:
180 - m∠B = 5m∠B + 36
Simplifying and putting all the mB words to one side:
6m∠B = 144
m∠B = 24
As a result, angle B has a 24 degree measure as the total of the two angles, which are along a straight line, is 180 degrees.
To know more about angles visit:
https://brainly.com/question/14569348
#SPJ1
How many cubic feet of warehouse space are needed for 350 boxes 11in. by 8in. by 12in?
Answers
Answer:
214 cubic feet (rounded to the nearest cubic foot)
Step-by-step explanation:
Step 1 Determine the volume of 1 box
Volume of a box or rectangular prism = dimensions multiplied by each other
Here, the given dimensions are 11 in by 8 in by 12 in
So the volume of one box would be 11 x 8 x 12 = 1056 cubic inches
Step 2 Determine volume of all 350 boxes
Volume of 1 box = 1056
So volume of all 350 boxes = 1056 x 350 = 369600 cubic in
Step 3 Convert cubic in to cubic ft
To convert to cubic feet we divide the amount of cubic inches by 1728 (this is because there are 1728 cubic inches in 1 cubic foot)
369600 / 1728 = 214 cubic feet (to the nearest cubic foot)
214 cubic feet of warehouse space is required for 350 boxes with the dimensions of 11in by 8in by 12in
The base angle of an isosceles triangle is 45. What is the measure of the vertex angle?

Answers
First, let's picture the problem:
Let's label the unknown vertex angle as x
Remember that the sum of angles in a triangle is 180
Hence,
\(\begin{gathered} 45^0+45^0+x=180^0 \\ x=180^0\text{ - }90^0 \\ =90^0 \end{gathered}\)For an isosceles triangle, the base angles are equal

what is the area of the figure

Answers
Answer:
A = 2184 cm²
Step-by-step explanation:
the area (A) of the figure is calculated as
A = \(\frac{1}{2}\) h(b₁ + b₂)
where h is the perpendicular height between the bases b₁ and b₂
here h = 42 , b₁ = 52 , b₂ = 52 , then
A = \(\frac{1}{2}\) × 42 × (52 + 52) = 21 × 104 = 2184 cm²
Answer:
A = 2184 cm2
Step-by-step explanation:
Area of a parallelogram:
\(A = b*h\)
\(b=52,h=42\)
\(A=(42)(52)=2184cm^{2}\)
Hope this helps.
How many solutions does this system of equations have y = -1/3x + 7. y = -2x^3 + 5x^2 + x - 2
Answers
The system of equations has one solution.
How many solutions does this system has?To check this, we can graph the two equations of the system:
y = (-1/3)x + 7
y = -2x³ + 5x² + x - 2
On the same coordinate axis, and check how many times do the graphs intercept.
The graph can be seen in the image at the end, there you can see that there is only one intecept point. Thus, the system of equations has only one solution.
Learn more about systems of equations at:
https://brainly.com/question/13729904
#SPJ1

Suppose you start at the origin, move along the x-axis a distance of 4 units in the positive direction, and then move downward along the z-axis a distance of 2 units. What are the coordinates of your position
Answers
Answer:
\(B = (x+4,y-2)\)
Step-by-step explanation:
Let the current position be A:
\(A = (x,y)\)
Required
Determine the new position
First, you move 4 units to the right.
This is represented as \(x + a\)
Where a is the number of units to the right
In this case:
\(a = 4\)
So, we have: \(x + 4\)
Then you move 2 units downwards
This is represented as \(y - b\)
Where b is the number of units downwards.
In this case:
\(b = 2\)
So, we have: \(y - 2\)
So, the new position (B) is:
\(B = (x+4,y-2)\)
What is the slope of the line x
=-1?
Answers
Answer:
Undefined
Step-by-step explanation:
Solve the simultaneous equations
3x - 4y = 8
9x + 5y = -1.5
Answers
Answer:
y = -1.5
x = 2/3
Step-by-step explanation:
Step 1. Multiply to eliminate x and solve for y
3(3x - 4y = 8)
9x + 5y = -1.5
Step 2. Eliminate
9x - 12y = 24
- 9x + 5y = -1.5
--------------------------
-17y = 25.5
y = -1.5
Step 3. Re-plug in and solve for x.
9x - 12(-1.5) = 24
9x + 18 = 24
9x = 6
x = 2/3
Answer:
x = \(\frac{2}{3} \) , y = - \(\frac{3}{2} \)
Step-by-step explanation:
3x - 4y = 8 → (1)
9x + 5y = - 1.5 → (2)
Multiplying (1) by - 3 and adding to (2) will eliminate the x- term
- 9x + 12y = - 24 → (3)
add (2) and (3) term by term to eliminate x
0 + 17y = - 25.5
17y = - 25.5 ( divide both sides by 17 )
y = \(\frac{-25.5}{17} \) = - \(\frac{3}{2} \)
substitute this value into either of the 2 equations and solve for x
Substituting into (2)
9x + 5(- \(\frac{3}{2} \) ) = - 1.5
9x - \(\frac{15}{2} \) = - \(\frac{3}{2} \) ( add \(\frac{15}{2} \) to both sides )
9x = \(\frac{12}{2} \) = 6 ( divide both sides by 9 )
x = \(\frac{6}{9} \) = \(\frac{2}{3} \)
please help me now this is due today

Answers
Answer:
-24+13x
Step-by-step explanation:


Review the simple interest rate based on FICO scores to answer the question:
FICO Score Simple Interest Rate
800–850 5.295%
740–799 6.597%
670–739 9.132%
580–669 10.358%
300–579 14.313%
Kamryn plans to borrow $13,250.00 with a simple interest rate loan. Determine the amount of interest Kamryn will save if she is able to raise her credit score from 665 to 680.
Answers
Kamryn would save $159.99 in interest by raising her credit score from 665 to 680 when borrowing $13,250.00 with a simple interest rate loan.
To determine the amount of interest Kamryn will save by raising her credit score from 665 to 680, we need to compare the interest rates associated with each credit score range.
According to the given information, a credit score of 665 falls within the range of 580-669, where the corresponding simple interest rate is 10.358%.
Let's calculate the interest Kamryn would pay on a loan of $13,250.00 at an interest rate of 10.358%.
Interest = Principal * Rate
= $13,250.00 * 0.10358
≈ $1,370.56
Therefore, if Kamryn were to borrow $13,250.00 with a credit score of 665, she would pay approximately $1,370.56 in interest.
Now, let's consider the scenario where Kamryn raises her credit score to 680. A credit score of 680 falls within the range of 670-739, where the corresponding simple interest rate is 9.132%.
Calculating the interest with a credit score of 680:
Interest = Principal * Rate
= $13,250.00 * 0.09132
≈ $1,210.57
Thus, if Kamryn were able to raise her credit score from 665 to 680, she would save approximately $1,370.56 - $1,210.57 = $159.99 in interest.
Therefore, Kamryn would save approximately $159.99 in interest by raising her credit score from 665 to 680 when borrowing $13,250.00 with a simple interest rate loan.
For more question on interest visit:
https://brainly.com/question/25720319
#SPJ8
What is the value of 8 if 8 is a constant?
Answers
Answer:
8.
Step-by-step explanation:
Constant = Constant
Question 6 (5 points)
Which of the following pairs of triangles can be proven similar through SSS
similarity?

Answers
The pairs of triangles can be proven similar through SSS similarity is given by Third pair.
We know that the SSS similarity criteria will have the ratio of three corresponding sides of both triangles congruent.
1. KL / EG = HL / DG = HK / DE
3/6 = 6.5/13 ≠ 4/10
Thus, SSS similarity does not follow.
2. KL / EG = HL / DG = HK / DE
3/10 ≠ 6.5/13 ≠ 4/10
Thus, SSS similarity does not follow.
3. KL / EG = HL / DG = HK / DE
3/6 = 6.5/13 = 5/10
Thus, SSS similarity follow.
4. All three angles are congruent.
Thus, SSS similarity does not follow.
Learn more about similarity here:
https://brainly.com/question/26451866
#SPJ1
A certain group of women has a .65% rate of red/green color blindness. If a woman is randomly selected, what is the probability that she does not have red/green color blindness?.
Answers
The probability that a randomly selected woman does not have red/green color blindness is approximately 0.994.
To calculate the probability of the complement event, we can subtract the probability of the event itself from 100%. So, the probability of not having red/green color blindness is:
100% - 0.65% = 99.35%
This means that if we randomly select a woman from this group, there is a 99.35% chance that she does not have red/green color blindness.
Finally, we can express this probability as a decimal or a percentage. In this case, the probability is:
0.9935 or approximately 0.994 (rounded to three decimal places)
So, The likelihood that a lady chosen at random does not have red/green colour blindness is about 0.994.
To learn more about probability please click on below link.
https://brainly.com/question/30034780
#SPJ1
A cheetah's speed was timed over a 50-yard distance. The cheetah was clocked running 60 miles per hour. Write an equation to represent this situation. (If your answering please show how you solved this)
Answers
An equation to represent this situation is y = 60x
What is the slope-intercept form?In Mathematics and Geometry, the slope-intercept form of the equation of a straight line is given by this mathematical equation;
y = mx + b
Where:
m represent the slope or rate of change.x and y are the points.b represent the y-intercept or initial value.Based on the information provided above, we can logically deduce that the cheetah was clocked running 60 miles per hour over a 50-yard distance. This ultimately implies that, an equation that models this situation is given by:
y = 60x
Where:
y represents the distance.x represents the time in hours.Read more on equation here: https://brainly.com/question/1382184
#SPJ1
Find the angle in degrees

Answers
Answer:
90 degrees
Step-by-step explanation:
its a 45-45-90 triangle
I just need answers. No need longer to explain.Solve a

Answers
We need to find the period of the sinusoidal function in this case we have the next form
\(y=A\sin \frac{2\pi}{T}(x+a)+b\)First, we need to find the amplitude in this case
\(A=\frac{5+1}{2}=\frac{6}{2}=3\)The amplitude is 3
Then we need to find the period
\(T=\frac{2\pi}{3}\)and the the displacement b is 2
Then for a we have
\(a=\frac{5}{12}\pi\)Therefore we have
\(y=3\sin 3(x-\frac{5\pi}{12})+2\)ANSWER
\(y=3\sin 3(x-\frac{5\pi}{12})+2\)The parabola X= √y-9 opens: right left down up?
Answers
The parabola x = √(y - 9) opens upwards.The given parabolic equation is x = √(y - 9). Let's identify the direction of opening of this parabola.The general form of the equation of a parabola is y = a(x - h)² + k.
Comparing this to the given equation, we can see that h = 0 and k = 9. The vertex is therefore (h, k) = (0, 9). Now, let's determine whether the parabola opens upwards or downwards.
If the coefficient of (x - h)² is positive, the parabola opens upwards, and if it's negative, the parabola opens downwards. In this case, since the coefficient of (x - h)² is 1, which is positive, the parabola opens upwards.
Therefore, the parabola x = √(y - 9) opens upwards.
For more question on equation
https://brainly.com/question/29174899
#SPJ8
3/2+b=7/4
what does b=
Answers
Answer:
b = 1/4.
Step-by-step explanation:
To solve for b, we need to isolate it on one side of the equation.
3/2 + b = 7/4
Subtract 3/2 from both sides:
b = 7/4 - 3/2
To add the fractions on the right-hand side, we need a common denominator:
b = (7/4) - (3/2) = (7/4) - (6/4) = 1/4
Therefore, b = 1/4.
Answer: \(\frac{1}{4}\)
Step-by-step explanation:
\(\frac{3}{2} +b=\frac{7}{4}\)
Move the constant to the right-hand side and change its sign
\(b=\frac{7}{4} -\frac{3}{2}\)
Subtract the fractions
\(b=\frac{1}{4}\)
Select the correct answer.
Which graph represents the solutions to this equation?
x2 + 8x = -20
(as a graph pls!)
Answers
Answer:
Step-by-step explanation:

Idk anything about math please I need help

Answers
Answer:
21 boys
Step-by-step explanation:
2:3=14:x you cross multiply this to find the amount of boys
2x=42
x=21
There are 21 boys in the class.
Basically, solve what you have there with cross-multiplication.
2x = 14 x 3
2x = 42
Then divide both sides by 2. (edited it bc didn't say what you actually had to do)
2x ÷ 2 = 42 ÷ 2
x = 21.
There are 21 boys in the class.