The coordinate plane shows the location of teammates during a game of ultimate Frisbee. Each interval on the coordinate plane represents 1 foot
Jonathan
4
Malik
3
Marianne
Ellena
If Marianne throws the Frisbee directly to Malik, how far, in feet (ft), will the Frisbee travel? Round your answer to the nearest tenth.

Answers
Related Questions
(3j+6)=
)G
I
Stuck? Watch a video or use a hint.
Answers
Answer:
= 3(j+2)
Step-by-step explanation:
Answer:
definitely watch a video!
Step-by-step explanation:
SHOW
YOUR
WORK!!
X -
-67 V6 ²-4ac
2a
The
equation d = n²-12n + 43
models the number of defective
items a produced in a manufacturing process where there are
workers in a restricted
area
a) solve for
n
When
d=30
INTL
DLL
Answers
Answer:
1.20, 10.8
Step-by-step explanation:
d = n^2 - 12n + 43
d = 30
30 = n^2 - 12n + 43
0 = n^2 - 12n + 13
now solve for n using quadratic formula:
a=1, b=-12, c=13
n = (-b +- sqrt( b^2 -4ac))/2a
two answers ( one for + the square root, one for - the square root)
n = 1.20, n = 10.8
The probability of getting heads on a single coin flip is ;
2
The probability of getting nothing but heads
on a series of coin flips decreases by
2
for each additional coin flip. Enter an exponential function for the
probability p(n) of getting all heads in a series of n coin flips. Give your answer in the form a (b)'. In the
event that a = 1, give your answer in the form (b)'.
Answers
What percentage of growth is needed annually to reach 400,000 in 3 years if today I have 200,000
Answers
Answer:
approx 26%
Step-by-step explanation:
you try find the multiplier
200000 * 1. ???? ^3= 400000
rearrange equation
\(\sqrt[3]{\frac{400000}{200000} }\) = multiplier
1.25992105
subract one
0.25992105
multiply by 100 to get percentage
25.9%
rounded to whole number = 26%
Solve the absolute value inequality |-2z+30| ≤ 30
Answers
The answer of the above question is [0,30]
An absolute value inequality is one with an absolute value sign and a variable inside.
An important point to remember: | x |= {x if x ≥ 0 }
| x |= {x if x< 0}
taking the expression |-2z+30| ≤ 30
Write the equivalent compound inequality.
-30≤ -2z+30 ≤ 30
= -30-30 ≤ -2z ≤ 30-30
= -60≤ -2z ≤ 0
= -60/2 ≤ -z ≤ 0
= -30 ≤ -z ≤ 0
Solve the compound inequality.
= 0 ≥ z ≥ 30 (reversing of signs)
Hence, the solution using interval notation [0,30].The check is left to you.
LEARN MORE ABOUT ABSOLUTE VALUE INEQUALITY HERE: https://brainly.com/question/13344207
#SPJ9
Pamela is 15 years younger than Jiri. The sum of their ages is 55. What is Jiri's age?
Answers
Answer:
Step-by-step explanation:
P = J - 15
P + J = 55
(J - 15) + J = 55
2J = 40
J = 20 years
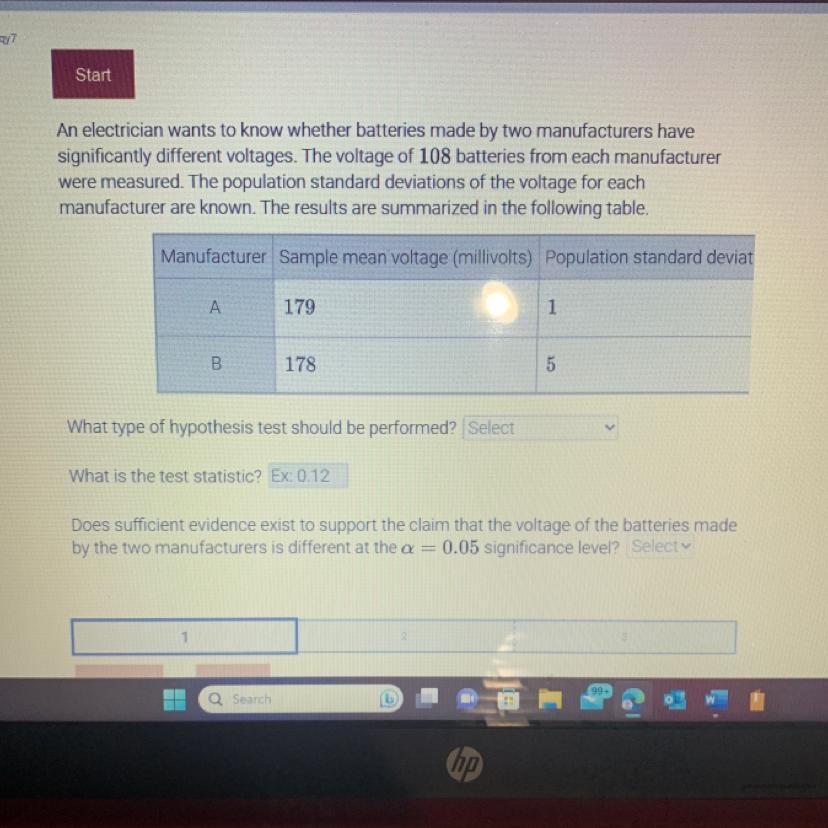
An electrician wants to know whether batteries made by two manufacturers have
significantly different voltages. The voltage of 108 batteries from each manufacturer
were measured. The population standard deviations of the voltage for each
manufacturer are known. The results are summarized in the following table.
Manufacturer Sample mean voltage (millivolts) Population standard deviat
A
B
179
178
1
What type of hypothesis test should be performed? Select
What is the test statistic? Ex: 0.12
Does sufficient evidence exist to support the claim that the voltage of the batteries made
by the two manufacturers is different at the a= 0.05 significance level?
Answers
The appropriate hypothesis test is a two-sample t-test for independent samples.
The appropriate hypothesis test in this scenario is a two-sample t-test for independent samples. Since the population standard deviations are known, we can use the Z-test instead.
The test statistic can be calculated using the formula:
t = (x1 - x2) / sqrt((s1^2 / n1) + (s2^2 / n2))
Where:
x1 and x2 are the sample means for Manufacturer A and Manufacturer B, respectively.
s1 and s2 are the population standard deviations for Manufacturer A and Manufacturer B, respectively.
n1 and n2 are the sample sizes for Manufacturer A and Manufacturer B, respectively.
Plugging in the given values:
x1 = 179, x2 = 178, s1 = 1, s2 = 5, n1 = n2 = 108
t = (179 - 178) / sqrt((1^2 / 108) + (5^2 / 108))
t = 1 / sqrt(0.00926 + 0.0463)
t ≈ 1 / sqrt(0.05556)
t ≈ 1 / 0.2357
t ≈ 4.241
To determine whether there is sufficient evidence to support the claim that the voltage of the batteries made by the two manufacturers is different, we compare the calculated t-value to the critical value from the t-distribution at the desired significance level (0.05).
The decision depends on the degrees of freedom for the test, which is calculated as (n1 + n2 - 2) = (108 + 108 - 2) = 214.
Using a t-table or statistical software, we find that the critical value for a two-tailed test with a significance level of 0.05 and 214 degrees of freedom is approximately ±1.972.
Since the calculated t-value of 4.241 exceeds the critical value of 1.972, we reject the null hypothesis and conclude that there is sufficient evidence to support the claim that the voltage of the batteries made by the two manufacturers is different at the 0.05 significance level.
For more such question on hypothesis
https://brainly.com/question/25263462
#SPJ8
When rolling a die what is the chance of getting a 3
Answers
The chance of rolling a die and landing on a three is 1/6. The reason for this is because there are 6 sides on a die, and only one 3 on the die.
2-11 Unit Rates
Part 1 of 3
Due 11/1
Challenge An arts academy repares there to be 4 teachers for every 60 students and 6 tutors for every 48 students. How many students does the academy heve per teacher? Por tudor? How many
tutors does the academy need if it has 00 students?
Answers
There are total 15 students per teacher and 8 students per tutor. For 100 students, total 13 tutors are required.
What is an arithmetic operation?The four fundamental operations of arithmetic are addition, subtract, multiply, and division of two or more numbers. Included in them is the study of integers, especially the order of operations, which is important for all other aspects of mathematics, notably algebra, information management, and geometry.
As per the given data provided in the question,
4 teachers = 60 students
For 1 teacher = 60/4 = 15 students
Similarly,
6 tutors = 48 students
For 1 tutor = 48/6 = 8 students.
For total 100 students, total number of tutors = 100/8
= 12.5 or 13 tutors are required.
To know more about arithmetic operation:
https://brainly.com/question/13585407
#SPJ1
SOMEBODY HELP MATH 100 POINTS BRAINLIEST⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️ HELP MATH 100 POINTS BRAINLIEST ⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️HELP MATH 100 POINTS BRAINLIESTHELP MATH 100 POINTS BRAINLIEST⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️ HELP MATH 100 POINTS BRAINLIEST ⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️HELP MATH 100 POINTS BRAINLIESTHELP MATH 100 POINTS BRAINLIEST⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️ HELP MATH 100 POINTS BRAINLIEST ⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️⚠️HELP MATH 100 POINTS BRAINLIEST

Answers
Answer:
\(\textsf{1.(a)} \quad x^2-14x+\boxed{49}=\left(x-\boxed{7}\right)^2\)
\(\textsf{1.(b)} \quad 9x^2 + 30x +\boxed{25}= \left(3x +\boxed{5}\right)^2\)
\(\begin{aligned}\textsf{2.(a)}\quad3x^2-24x+48&=3\left(x^2-\boxed{8}\:x+\boxed{16}\right)\\&=3\left(x-\boxed{4}\right)^2\end{aligned}\)
\(\begin{aligned}\textsf{2.(b)}\quad \dfrac{1}{2}x^2+8x+32&=\dfrac{1}{2}\left(x^2+\boxed{16}\:x+\boxed{64}\right)\\&=\dfrac{1}{2}\left(x+\boxed{8}\right)^2\end{aligned}\)
Step-by-step explanation:
Question 1(a) When completing the square for a quadratic equation in the form ax² + bx + c where the leading coefficient is one, we need to add the square of half the coefficient of the x-term:
\(x^2-14x+\left(\dfrac{-14}{2}\right)^2\)
\(x^2-14x+\left(-7\right)^2\)
\(x^2-14x+49\)
We have now created a perfect square trinomial in the form a² - 2ab + b². To factor a perfect square trinomial, use the following formula:
\(\boxed{a^2 -2ab + b^2 = (a -b)^2}\)
Therefore:
\(a^2=x^2 \implies a=1\)
\(b^2=49=7^2\implies b = 7\)
Therefore, the perfect square trinomial rewritten as a binomial squared is:
\(x^2-14x+\boxed{49}=\left(x-\boxed{7}\right)^2\)
(b) When completing the square for a quadratic equation where the leading coefficient is not one, we need to add the square of the coefficient of the x-term once it is halved and divided by the leading coefficient, and then multiply it by the leading coefficient:
\(9x^2 + 30x +9\left(\dfrac{30}{2 \cdot 9}\right)^2\)
\(9x^2 + 30x +9\left(\dfrac{5}{3}\right)^2\)
\(9x^2 + 30x +9 \cdot \dfrac{25}{9}\)
\(9x^2 + 30x +25\)
We have now created a perfect square trinomial in the form a² + 2ab + b². To factor a perfect square trinomial, use the following formula:
\(\boxed{a^2 +2ab + b^2 = (a +b)^2}\)
Therefore:
\(a^2=9x^2 = (3x)^2 \implies a = 3x\)
\(b^2=25 = 5^2 \implies b = 5\)
Therefore, the perfect square trinomial rewritten as a binomial squared is:
\(9x^2 + 30x +\boxed{25}= \left(3x +\boxed{5}\right)^2\)
\(\hrulefill\)
Question 2(a) Factor out the leading coefficient 3 from the given expression:
\(3x^2-24x+48=3\left(x^2-\boxed{8}\:x+\boxed{16}\right)\)
We have now created a perfect square trinomial in the form a² - 2ab + b² inside the parentheses. To factor a perfect square trinomial, use the following formula:
\(\boxed{a^2 -2ab + b^2 = (a -b)^2}\)
Factor the perfect square trinomial inside the parentheses:
\(=3\left(x-\boxed{4}\right)^2\)
(a) Factor out the leading coefficient 1/2 from the given expression:
\(\dfrac{1}{2}x^2+8x+32=\dfrac{1}{2}\left(x^2+\boxed{16}\:x+\boxed{64}\right)\)
We have now created a perfect square trinomial in the form a² + 2ab + b² inside the parentheses. To factor a perfect square trinomial, use the following formula:
\(\boxed{a^2+2ab + b^2 = (a +b)^2}\)
Factor the perfect square trinomial inside the parentheses:
\(=\dfrac{1}{2}\left(x+\boxed{8}\right)^2\)
Step-by-step explanation:
Since ABCD is a rectangle
⇒ AB = CD and BC = AD
x + y = 30 …………….. (i)
x – y = 14 ……………. (ii)
(i) + (ii) ⇒ 2x = 44
⇒ x = 22
Plug in x = 22 in (i)
⇒ 22 + y = 30
⇒ y = 8
Need help with a congruent answer! NEED IT FAST PLEASEE

Answers
Hope that helps
17. Rob purchased a boat three years ago. The boat decreased by 7% each year. Three years
later, the value of the boat was $32,174.28. Which equation initially models the value of Rob's
boat?
A. y = 40,000(0.93)*
B. y = 40,000(1.07)*
C. y = 32,174.28 (0.93)*
D. y = 32,174.28 (1.07)*
Answers
Answer:
The correct equation that initially models the value of Rob's boat is option A, y = 40,000(0.93)*.
Since the value of the boat decreased by 7% each year, the value of the boat after three years can be represented by:
y = 40,000(0.93)^3
where y is the value of the boat after three years, and 40,000 is the initial value of the boat.
Simplifying this equation, we get:
y = 40,000(0.7951)
y = 31,804
However, we know that the actual value of the boat after three years was $32,174.28. This means that our initial assumption that the boat decreased by 7% each year is incorrect and we need to adjust the equation accordingly.
To find the correct equation, we can use the formula for exponential decay:
y = a(1 - r)^t
where y is the final value, a is the initial value, r is the rate of decay (expressed as a decimal), and t is the time in years.
In this case, we know that the final value of the boat is $32,174.28, and that the boat was owned for three years. We also know that the value of the boat decreased by 7% each year.
So we can set up an equation:
32,174.28 = 40,000(0.93)^3
Simplifying this equation, we get:
32,174.28 = 31,804.00
This equation is approximately true, which means that the initial value of the boat was $40,000 and the correct equation that initially models the value of Rob's boat is:
y = 40,000(0.93)^t
where t is the time in years.
Students at a local elementary school were shown a painting and asked which emotion—joy, happiness, love, or anger—they felt by looking at the painting. The students were classified by their age. The following table summarizes the responses of the students by age-group. One student from the school will be selected at random. What is the probability that the student is in the age-
group of 6 to 8 years given that the selected student responded joy?
Answers
Answer:
28/89
Step-by-step explanation:
The probability that the student is in the age group of 6 to 8 years is 28/89 and this can be determined by using the given data.
Given :
Emotions - joy, happiness, love, or anger.The students were classified by their age.The following steps can be used in order to determine the probability that the student is in the age group of 6 to 8 years:
Step 1 - According to the table, the total number of students who responded joy is 89.
Step 2 - It is also given that the total number of students who responded joy of age group of 6 to 8 years is 28.
Step 3 - So, the probability that the student is in the age group of 6 to 8 years is:
\(P = \dfrac{28}{89}\)
Therefore, the correct option is E).
For more information, refer to the link given below:
https://brainly.com/question/795909
NO LINKS!! Given the exponential relationships below, find the following

Answers
Answer:
Exponential Function
General form of an exponential function with base \(e\):
\(f(x)=Ae^{kx}\)
where:
A is the y-intercepte (Euler's number) is the basek is some constantQuestion 30The curve crosses the y-axis at y = 40. Therefore, A = 40.
Substitute the found value of A into the formula along with (1, 56) and solve for \(k\):
\(\begin{aligned}f(x) & =Ae^{kx}\\\implies 56 & =(40)e^{k}\\e^k & =\dfrac{56}{40}\\k & =\ln (1.4)\end{aligned}\)
\(\textsf{Equation}: \quad f(x)=40e^{x\ln 1.4}\)
To find the population in 10 years, substitute \(x = 10\) into the found equation:
\(\begin{aligned}\implies f(10)&=40e^{10\ln 1.4}\\ & =1157.01862\\ & =1157\end{aligned}\)
Question 31The curve crosses the y-axis at y = 10. Therefore, A = 10.
Substitute the found value of A into the formula along with (1, 18) and solve for \(k\):
\(\begin{aligned}f(x) & =Ae^{kx}\\\implies 18 & =(10)e^{k}\\e^k & =\dfrac{18}{10}\\k & =\ln (1.8)\end{aligned}\)
\(\textsf{Equation}: \quad f(x)=10e^{x\ln 1.8}\)
To find the population in 8 years, substitute \(x = 8\) into the found equation:
\(\begin{aligned}\implies f(8)&=10e^{8\ln 1.8}\\ & =1101.996058\\ & =1102\end{aligned}\)
Conduct a survey based on the topic below and write a research report. You are required to collect, represent, analyse, interpret and report the data. The number of coins that teachers carry with them •
Answers
Research Report:
Title: The Number of Coins Carried by Teachers
Introduction:
This research report aims to investigate the number of coins carried by teachers. The study seeks to understand the reasons behind carrying coins and whether there are any patterns or correlations between the number of coins and certain factors such as age, gender, and occupation.
The data was collected through a survey distributed among teachers from various educational institutions. The findings of this study provide insights into teachers' habits and preferences when it comes to carrying coins.
Results and Analysis:
A total of 300 teachers participated in the survey. The data revealed that the majority of teachers (60%) carry less than 5 coins, while 25% carry between 5 and 10 coins. Only a small percentage (15%) reported carrying more than 10 coins.
Further analysis based on demographic factors indicated that age and occupation had a significant influence on the number of coins carried. Older teachers were more likely to carry fewer coins, with 70% of teachers above the age of 50 carrying less than 5 coins.
Additionally, primary school teachers tended to carry more coins compared to secondary school teachers.
Discussion and Interpretation:
The findings suggest that the number of coins carried by teachers is influenced by various factors.
Teachers may carry coins for a range of reasons, such as purchasing small items, providing change for students, or utilizing vending machines.
The lower number of coins carried by older teachers could be attributed to a shift towards digital payment methods or a preference for carrying minimal cash.
The discrepancy between primary and secondary school teachers could be due to differences in daily activities and responsibilities.
This research provides valuable insights into the habits and preferences of teachers regarding the number of coins they carry.
Understanding these patterns can assist in designing more efficient payment systems within educational institutions and potentially guide the development of tailored financial solutions for teachers.
Further research could explore the reasons behind carrying coins in more depth and investigate how the digitalization of payments affects teachers' behavior in different educational contexts.
for such more questions on investigate
https://brainly.com/question/32310280
#SPJ8
Simplify the following expression. 3 11 5 ÷ 3 − 9 5 A. 12 B. 1 81 C. 81 D.
Answers
Answer:
A
Step-by-step explanation:
To simplify the expression 3 11 5 ÷ 3 − 9 5, let's break it down step by step:
First, let's simplify the division 3 11 5 ÷ 3:
3 11 5 ÷ 3 = (3 × 115) ÷ 3 = 345 ÷ 3 = 115.
Next, let's subtract 9 5 from the result we obtained:
115 - 9 5 = 115 - (9 × 5) = 115 - 45 = 70.
Therefore, the simplified expression is 70.
The correct answer is A. 70.
The probability that Roger wins a tennis tournament (event A) is 0.45, and the probability that Stephan wins the tournament (event 8) is
0.40. The probability of Roger winning the tournament, given that Stephan wins, is O. The probability of Stephan winning the tournament.
given that Roger wins, is 0. Given this information, which statement is true?
O A
Events A and Bare not independent because P(AB) # PA)
Answers
The true statement is that C. Events A and B are not independent because P(A|B) ≠ P(A).
How to illustrate the information?The probability that Roger wins a tennis tournament (event A) is 0.45 i.e. P(A)=0.45
The probability that Stephan wins the tournament (event B) is 0.40 i.e. P(B)=0.40
It should be noted that if A and are independent events, then P(B|A) = P(B). This isn't possible in this situation.
Therefore, the true statement is that events A and B are not independent because P(A|B) ≠ P(A).
Learn more about probability on:
https://brainly.com/question/314763
#SPJ1
The probability that Roger wins a tennis tournament (event A) is 0.45, and the probability that Stephan wins the tournament (event B) is 0.40. The probability of Roger winning the tournament, given that Stephan wins, is 0. The probability of Stephan winning the tournament, given that Roger wins, is 0. Given this information, which statement is true?
1.Events A and B are independent because P(A|B) = P(A).
2.Events A and B are independent because P(A|B) ≠ P(A).
3. Events A and B are not independent because P(A|B) ≠ P(A).
4. Events A and B are not independent because P(A|B) = P(A).
What is the slope of this line?
4
−3
−3/4
3/4

Answers
Answer:
The answer is -3/4.
Answer: -3/4
Step-by-step explanation:
The line is pointing downwards, making it negative and having a negative slope. The line goes down 3, over 4, so its slope is -3/4.
The radius of a circle is 3 feet. What is the length of a 75° arc?
Answers
Therefore , the solution of the given problem of circle comes out to be arc length 3.93 feet.
How do circles work?Every area of the plane that is separated by a specific amount from this additional point makes a circle (center). As a result, it is a curve made up of spots that are separated from one another on the surface. Additionally, it rotates similarly about the centre at every angle. Every collection of endpoints in the confined, two-dimensional sphere of a circle is uniformly spaced apart from the "centre."
Here,
A circle with a radius of 3 feet is equal to its diameter as follows:
=> C = 2πr = 2π(3) = 6π feet
Since there are 360° of angles in a circle's centre, the angle for a 75° curve is:
5/24 of a complete circle is 75/360.
Therefore, the 75° arc's extent is
5/24 × 6π = 5π/4 ≈ 3.93 feet
To know more about circle visit:
https://brainly.com/question/29142813
#SPJ1
Cody donated $15 to the local Humane Society his brother donate at four dollars less than twice the amount that Cody donated how much money did Cody’s brother donate
Answers
Answer:
$26
Step-by-step explanation:
15 * 2= 30
30-4= 26
After a 10% decrease the cost of a new cooker is £ 198
Work out the original price of the cooker.
Answers
Answer:
£217.80
Step-by-step explanation:
198 increase 10% =
198 × (1 + 10%) = 198 × (1 + 0.1) = 217.8
There are 120 students and 10 teachers at a small private school. To keep the same
student to teacher ratio, how many teachers are needed for 240 students?
Answers
Answer:
20
Step-by-step explanation:
cover the costs:
Mrs. Smith is taking 120 students to a museum. She must collect the amounts below from each student
• $3.50 for the bus
• $2.70 for lunch
• $2.50 for admission to the museum
What is the total amount of money Mrs. Smith must collect from the students?
A. $720
OB. $934
C. $1,020
D. $1,044
Answers
Answer:
D. 1,044 is the total amount of money Mrs. Smith collected
Find the equation of the line that passes through the given point and has the given slope. (Use x as your variable.)(4, −3), m = −2
Answers
General equation of line:
\(y=mx+c\)Where,
\(\begin{gathered} m=\text{slope} \\ c=y-\text{intercept} \\ (x,y)=(4,-3) \end{gathered}\)Slope of line is -2 then:
\(\begin{gathered} y=mx+c \\ y=-2x+c \end{gathered}\)\((x,y)=(4,-3)\)\(\begin{gathered} y=-2x+c \\ -3=-2(4)+c \\ -3=-8+c \\ 8-3=c \\ 5=c \end{gathered}\)\(\begin{gathered} y=mx+c \\ y=-2x+5 \end{gathered}\)Equation of line is y=-2x+5
Consider the quadratic equation x2 + 2x - 35 = 0. Solve by factoring and using the zero-product property.
What are solutions to quadratic equations called? Show your work.
Answers
Answer:
x = -7
x = 5
Step-by-step explanation:
The standard form of quadratic equations is
Ax^2 + Bx + C = 0
The factors need to multiply together to be C and add together to be B.
The two numbers that will multiply together to be -35 and add together to be 2 are -5 & 7.
The factor pairs are (x+7)(x-5). Zero product property means we set each of those factor pairs = 0 and solve.
x + 7 = 0
-7 -7 Subtract 7 from each side to solve
x = -7
x - 5 = 0
+5 +5 Add 5 to each side to solve
x = 5
To solve the equation, factor x²+2x−35 use the formula x² +(a + b) x + ab = (x + a)(x + b). To find a and b, set up a system to be solved.
a + b = 2
ab = −35
Since ab is negative, a and b have opposite signs. Since a+b is positive, the positive number has a greater absolute value than the negative. Show all pairs of integers whose product is −35.
−1.35−5.7Calculate the sum of each pair.
−1 + 35 = 34−5 + 7 = 2The solution is the pair that gives sum 2.
a = −5b = 7Rewrite the factored expression (x + a)(x + b) with the values obtained.
(x − 5)(x + 7)To find solutions to equations, solve x−5=0 and x+7=0.
x = 5x = −7What are the solutions of quadratic equations called?The "solutions" of a Quadratic Equation are the values where the equation equals zero. They are also called "roots", or even "zeros".
ヘ( ^o^)ノ\(^_^ )If you want to learn more about mathematics, I share this link to complement your learning:
https://brainly.com/question/16413695To the nearest tenth of pound Does has need to buy?

Answers
Answer: She needs approximately 142.5 pounds
Explanation:
From the information given,
A pound of fertilizer covers 39 square feet of lawn.
If the lawn measures 5557.4 square feet, then
the number of pounds of fertilizer that she needs to buy = 5557.4/39 = 142.4975
Rounding to the nearest tenth,
She needs approximately 142.5 pounds
Classify each system of equations

Answers
since x = 2 and y = - 3 worked for the two equations I know that (2, - 3) is the solution to this system of equations.
To check a system of equations by substitution, you plug your values for x and y into the first equations. On the off chance that both simplified expressions are valid, your answer is right.
For instance: To check if (2, - 3) was the right solution for the system of equations:
y = - 2x + 1 and y= x - 5
substitute 2 for x and - 3 for y into every equation
y = - 2x + 1
-3 = - 2(2) + 1
-3 = - 4 + 1
-3 = - 3
also,
y = x - 5
-3 = 2 - 5
-3 = - 3
since x = 2 and y = - 3 worked for the two equations I know that (2, - 3) is the solution to this system of equations.
to know more about equations click here:
https://brainly.com/question/14107099
#SPJ1
NO LINKS!! Please assist me with this problem Part 1m

Answers
Answer:
(x + 8)² + (y - 8)² = 64=======================
Given ConditionsTangent to x-axis,Tangent to y-axis,In the second quadrant,Radius is 8 units.SolutionEquation of circle:
(x - h)² + (y - k)² = r², where (h, k) is center and r - radiusThe center is the radius long distance from the x- axis to left and y-axis up same distance, this makes it in the second quadrant.
So the coordinates of the center are:
x = - 8, y = 8The equation is:
(x - (-8))² + (y - 8)² = 8²(x + 8)² + (y - 8)² = 64
Answer:
\((x+8)^2+(y-8)^2=64\)
Step-by-step explanation:
Required conditions:
Tangent to both axes.Center in the second quadrant.Radius = 8 units.If the circle is tangent to both axes, its center will be the same distance from both axes. That distance is its radius.
If the center of the circle is in quadrant II, the center will have a negative x-value and a positive y-value → (-x, y).
Therefore, the coordinates of the center will be (0-r, 0+r) where r is the radius.
If the radius is 8 units, then the center is (-8, 8).
\(\boxed{\begin{minipage}{4 cm}\underline{Equation of a circle}\\\\$(x-a)^2+(y-b)^2=r^2$\\\\where:\\ \phantom{ww}$\bullet$ $(a, b)$ is the center. \\ \phantom{ww}$\bullet$ $r$ is the radius.\\\end{minipage}}\)
Substitute the found center and given radius into the formula to create an equation for the circle that satisfies the given conditions:
\(\implies (x-(-8))^2+(y-8)^2=8^2\)
\(\implies (x+8)^2+(y-8)^2=64\)

PLS HELP!!!
Solve.
2x-y+2z=-6
-3y+z=-2
2x-3z+4

Answers
The solution to the system of equations is x = -8/7, y = 4/7 and z = -2/7
How to solve the system of equations?The system of equations is given as
2x - y + 2z = -6
-3y + z = -2
2x - 3z = -4
Subtract 2x - 3z = -4 from 2x - y + 2z = -6
This is represented as
(2x - y + 2z = -6) - (2x - 3z = -4)
This gives
- y + 5z = -2
Multiply - y + 5z = -2 by 3
-3y + 15z = -6
Subtract -3y + 15z = -6 from -3y + z = -2
This is represented as
(-3y + z = -2) - (-3y + 15z = -6)
This gives
-14z = 4
Divide by -14
z = -2/7
Substitute z = -2/7 in - y + 5z = -2
- y - 5 * 2/7 = -2
This gives
- y - 10/7 = -2
Rewrite as:
y = 2 - 10/7
Evaluate
y = 4/7
Substitute z = -2/7 in 2x - 3z = -4
2x - 3 * 4/7 = -4
This gives
2x - 12/7 = -4
So, we have:
2x = 12/7 - 4
Evaluate
2x = -16/7
Divide by 2
x = -8/7
Hence, the solution to the system of equations is x = -8/7, y = 4/7 and z = -2/7
Read more about system of equations at
https://brainly.com/question/13729904
#SPJ1
Gordon types 3,724 words in 49 minutes. Find the unit rate. Gordon types words per minute.
Answers
Answer:
76 words per minute.
Step-by-step explanation:
49 min = 3724 words
1 min = ?
3724/49 = 76
1 min = 76 words
Hope this helps!!