solve with clear steps pleaseQuestion 5 Evaluate the integral. 2x² 3+x dx Solution:
Answers
The integral is ∫(2x²)/(3+x) dx = C.
To evaluate the integral ∫(2x²)/(3+x) dx, we can use the method of partial fractions.
First, we need to factor the denominator. We can factor 3+x as (x+3).
∫(2x²)/(3+x) dx = ∫(2x²)/(x+3) dx
Next, we need to express the integrand as a sum of simpler fractions with denominators (x+3) and (some constant).
2x²/(x+3) = A + B/(x+3)
Multiplying both sides by (x+3), we get:
2x² = A(x+3) + B
Substituting x=-3, we get:
-54 = -3A + B
Substituting x=0, we get:
0 = 3A + B
Solving for A and B, we get:
A = 0
B = 0
Therefore,
2x²/(x+3) = 0 + 0/(x+3)
So,
∫(2x²)/(3+x) dx = ∫0 dx = C
where C is the constant of integration.
Therefore,
∫(2x²)/(3+x) dx = C
To learn more about integration visit:https://brainly.com/question/18125359
#SPJ11
Related Questions
I need a step by step explanation on how to get y = mx + b

Answers
4y = 3x + 4
Y = mx + b
a manufacturer of potato chips would like to know whether its bag filling machine works correctly at the 411.0 gram setting. it is believed that the machine is underfilling the bags. a 35 bag sample had a mean of 406.0 grams. a level of significance of 0.05 will be used. state the hypotheses. assume the standard deviation is known to be 25.0.
Answers
Using a 35-bag sample with a mean of 406.0 grams, a known standard deviation of 25.0 grams, and a level of significance of 0.05, you can perform a one-tailed Z-test to determine whether to reject or fail to reject the null hypothesis.
To test if the potato chip manufacturer's bag filling machine is working correctly at the 411.0-gram setting, we will state the hypotheses using the given terms.
Null Hypothesis (H0): The machine fills bags correctly, with a mean weight of 411.0 grams (µ = 411.0 grams)
Alternative Hypothesis (H1): The machine is underfilling bags, with a mean weight less than 411.0 grams (µ < 411.0 grams)
To learn more about standard deviation, refer here:
https://brainly.com/question/23907081#
#SPJ11
A line passes through the points (6, 2) and (5, 0). What is its equation in slope-intercept form?
Answers
Answer:
y = 2x - 10
Step-by-step explanation:
y2 - y1/ x2 - x1
0 - 2 / 5 - 6
= 2
y = 2x + b
2 = 2(6) +b
2 = 12 + b
-10 = b
The equation is y = 2x - 10
x²-__x +25=0
Fill in the Blank to make a perfect trinomial
Answers
Answer:
10
Step-by-step explanation:
A perfect square of \((a-b)^2\) should be in the form \(a^2-2ab+b^2\). In this case, \(a^2=1\), and \(b^2 =25\), so a=1 and b=5. Now, we need to find 2ab, so we do
2(1)(5)=10, so 10 goes in the blank.
When can a correlation coefficient based on an observational study be used to support a claim of cause and effect? Never When the correlation coefficient is close to -1 or +1. When the correlation coefficient is equal to -1 or +1. When the scatterplot of the data has little vertical variation.
Answers
Never. Correlation coefficients are used to measure the strength of a linear relationship between two variables, not to prove cause and effect. To determine causation, it is necessary to conduct an experiment or study in which the independent variable is manipulated and the dependent variable is measured.
Correlation coefficients are used to measure the strength of a linear relationship between two variables. They can measure the degree to which variables move together, but they cannot be used to prove cause and effect. To determine causation, it is necessary to perform an experiment or study in which one variable is manipulated and the other is measured. This allows researchers to control for confounding variables and to determine if the manipulation of the independent variable had a direct effect on the dependent variable. Therefore, correlation coefficients cannot be used to support a claim of cause and effect.
Learn more about correlation here
https://brainly.com/question/28898177
#SPJ4
This is the last questions i need help with this plz

Answers
Answer:
add it + 2
Step-by-step explanation:
an orange has a volume of about 113.1 cm3. what is the area, in sq cm, of the cross-section when the orange is cut in half? round to the nearest tenth.
Answers
If there is orange with a volume of 113.1 cm3, the area of the cross-section when the orange is cut in half is approximately 34.9 sq cm.
If we assume that the orange is a sphere and has a volume of 113.1 cm3, we can use the formula for the volume of a sphere to find the radius of the orange. The formula for the volume of a sphere is V = (4/3) * pi * r^3, where r is the radius of the sphere.
Substituting the given volume of 113.1 cm3 and solving for the radius r, we find that r = (3V / (4 * pi))^(1/3) = (3 * 113.1 / (4 * pi))^(1/3) approximately 3.3 cm.
Next, we can use the formula for the surface area of a sphere to find the area of the cross-section when the orange is cut in half. The formula for the surface area of a sphere is A = 4 * pi * r^2, where r is the radius of the sphere. Substituting the value of the radius that we calculated above, we find that the area of the cross-section is A = 4 * pi * (3.3 cm)^2 approximately 34.9 sq cm.
To know more about Area
https://brainly.com/question/11952845
#SPJ4
Please help me guys I do not know what to do at this point, but to come to yall and I really need help with this math this is due today and please I neeed you guys help I do not want to put any burden on you I am just stress thanks for understanding
Instructions: Tell weather it is a function or not and tell why
Answers
Answer:
YesYesNoNoYesYesNoYesNoNoYesNoYesNoYesYesNoYesYesNoStep-by-step explanation:
A function does not have any repeating domains (x-coordinates).
Hope it helps!
What is the equation of a line passing through the points (-3,
-2) and (2, 0)?
Answers
Answer:
\(y=0.400x+-0.800\)
Step-by-step explanation:
Given the following question:
point A = (-3, -2)
point B = (2, 0)
To write the equation of the line that passes through two points we have to write the points in slope intercept form.
Formula for slope intercept:
\(y=mx+b\)
M is equal to the slope of the two points, so first we need to fine the slope of the two points.
\((-3,-2)=(x1,y1)\)
\((2,0)=(x2,y2)\)
\(m=\frac{y2-y1}{x2-x1}\)
\(m=\frac{0--2}{2--3} =\frac{2}{5}\)
\(m=\frac{2}{5} =0.400\)
\(y=mx+b\)
\(y=-2\)
\(m=0.400\)
\(x=-3\)
\(-2=0.400(-3)+b\)
\(0.400\times-3=-1.20\)
\(-2+1.2=-0.800\)
\(b=-0.800\)
\(y=0.400x+-0.800\)
Hope this helps.
what is the total amount of sap produced that day?
Answers
Answer:
8 gallons
Step-by-step explanation:
Already a question from another person.
Answer:
5 gallons
Step-by-step explanation:
What is the measure of the indicated angle?

Answers
please answer quickly easy 10 points but please answer quick

Answers
Answer:
He traveled 520 miles
Step-by-step explanation:
8 x 65 = 520
Answer: 520 miles
Step-by-step explanation:
Data: Juan drove 8 hours
Drove at an average Speed of 65 mph
Miles driven=x
Only step: Multiply 65 by 8 which is 520
Explanation: Since Juan drove at an average speed of 65 miles per hour, that means he drove 65 mile for every hour he drove. So since he drove for 8 hours, you multiply 8 hours by 65 miles so that you get get the total amount of hours driven by him.
Don't forget to add "Miles" to the end of your answer because most math teachers tend to want that in an answer like this.
I hope this helps(Mark brainiest if you want to, thanks)
Help fast pls I need to pass
Answers
Answer:
help with what? you can tell me and I would be glad to answer.
Step-by-step explanation:
Equilateral triangle T is inscribed in circle A , which has radius 10 . Circle B with radius 3 is internally tangent to circle A at one vertex of T . Circles C and D , both with radius 2, are internally tangent to circle A at the other two vertices of T. Circles B, C , and D are all externally tangent to circle E, which has radius m/n , where m and nare relatively prime positive integers. Find m+n
Answers
For the given figure, where m and nare relatively prime positive integers. The value of m+n is 32.
Explain Law of Cosines.The law of cosines, also known as the law of cosines, relates all three sides of a triangle to one angle of the triangle. Most useful for searching for missing information in triangles. For example, if you know all three sides of a triangle, you can use the law of cosines to find the measure of the angle. Similarly, if you know two sides and the angle between them, you can find the length of the third side by the law of cosines.
Let X be the intersection of the circles with centers B and E, and Y be the intersection of the circles with centers C and E.
Since the radius of B is 3, AX = 4.
Assume AE = p.
Then EX and EY are radii of circle E and have length 4 + p.
AC = 8, and ∠CAE = 60° because we are given that triangle T is equilateral.
Using the Law of Cosines on Δ CAE, we obtain
(6+p)² =p² + 64 - 2(8)(p) cos 60
The 2 and the cos 60 terms cancel out:
p² + 12p + 36 = p² + 64 - 8p
12p+ 36 = 64 - 8p
p = 28/20
= 7/5
The radius of circle E is 4 + (7/5)
= 27/5,
So, the answer is 27 + 5 = 32
To know more about law of cosines, visit:
https://brainly.com/question/17289163
#SPJ4
The time in seconds, t, it takes for a specific object being dropped from a particular height in feet above sea level, h, to reach the ground can be found by the radical function t equals one fourth times radical h period At what height should you drop an object in order for it to reach the ground in 16 seconds?

Answers
Answer:
B.) 4,096 feet
Step-by-step explanation:
You were given a time, and time in the equation is represented by "t". To find the height, represented by "h", you just need to plug the given value in for "t" and simplify to find "h".
t = (1/4)√x <--- Original equation
16 = (1/4)√x <--- Plug 16 in "t"
64 = √x <--- Divide both sides by (1/4)
4,096 ft = x <--- Square both sides
The object reach the ground in 16 seconds, you should drop it from a height of 4096 feet.
The equation is t=1/4 √x , where t is time in seconds and x is height in feet. To determine the height x at which an object should be dropped to reach the ground in 16 seconds, you can plug in the value of t=16 into the equation:
16 = 1/4 √x
Simplify the equation by multiplying both sides by 4:
4⋅16= √x
64= √x
Now, square both sides to isolate x:
64²=x
4096 = x
Therefore, to have the object reach the ground in 16 seconds, you should drop it from a height of 4096 feet.
To know more about height:
https://brainly.com/question/34133884
#SPJ11
mr. dyer pours 2 cups of blue paint into a jar for each art station. how many jars can he fill with 1 gallon of blue paint?
Answers
If Mr. Dyer pours 2 cups of blue paint into a jar for each art station, then the he can fill 8 jars with 1 gallon of blue paint.
Number of cups of blue paint for each art station = 2 cups
Given that,
One cup = 8 ounce
1 gallon = 128 ounce
2 cups of blue paint = 2 × 8
= 16 ounce of blue paint
1 gallon of blue paint = 128 ounce of blue paint
Number of jars that he can fill with 1 gallon of blue paint = 1 gallon of blue paint / 2 cups of blue paint
Here we have to use division
= 128/16
= 8 jars
Hence, if Mr. Dyer pours 2 cups of blue paint into a jar for each art station, then the he can fill 8 jars with 1 gallon of blue paint.
The complete question is:
Mr. dyer pours 2 cups of blue paint into a jar for each art station. how many jars can he fill with 1 gallon of blue paint? One cup = 8 ounce and 1 gallon = 128 ounce
Learn more about division here
brainly.com/question/21416852
#SPJ4
pls help ill rate 5 starts and thank. what is the value of x and is it a acute right obtuse scalene isotopes or equal tital

Answers
The sum of all angles in a triangle = 180 degree
So :
Angle x + Angle 5x + 72 = 180
x + 5x + 72 = 180
6x = 180 - 72
6x = 108
Divide both side by 6 :
6x/6 = 108/6
x = 18
x = 18
since all the angles are less than 90 degree so the triangle is an acute triangle,
as 5x = 90 thus one angle is of 90 degree
Thus triangle is Acute Right angle triangle
Answer : x = 18, acute right angle triangle
Pls help me
What is the volume of this figure?
Enter your answer in the box.

Answers
Answer:
Step-by-step explanation:
Volume of the figure = Base area * height
Base area:
Base area = area of trpezium + area of triangle
Trapezium:
a, b area the length of parallel sides and h is the height of trapezium.
a = 8 m ; b = 5 m & h = 3 m
\(\boxed{ \text{ Area of trapezium = $\dfrac{(a +b)*h}{2} $}}\)
\(=\dfrac{(8+5)*3}{2}\\\\\\=\dfrac{13*3}{2}\\\\\\= \dfrac{39}{2}\\\\\\= 19.5 \ m^{2}\)
Triangle:
height = 4 m & base = 8 m
\(\boxed{\text{Area of triangle =$\dfrac{1}{2}*base*height$}}\)
\(=\dfrac{1}{2}*8*4\\\\\\= 4*4\\\\= 16 \ m^{2}\)
Base area = 19.5 + 16
= 35.5 m²
Volume of the figure = Base area * height
= 35.5 * 14
= 497 m³
In the graph below find AB
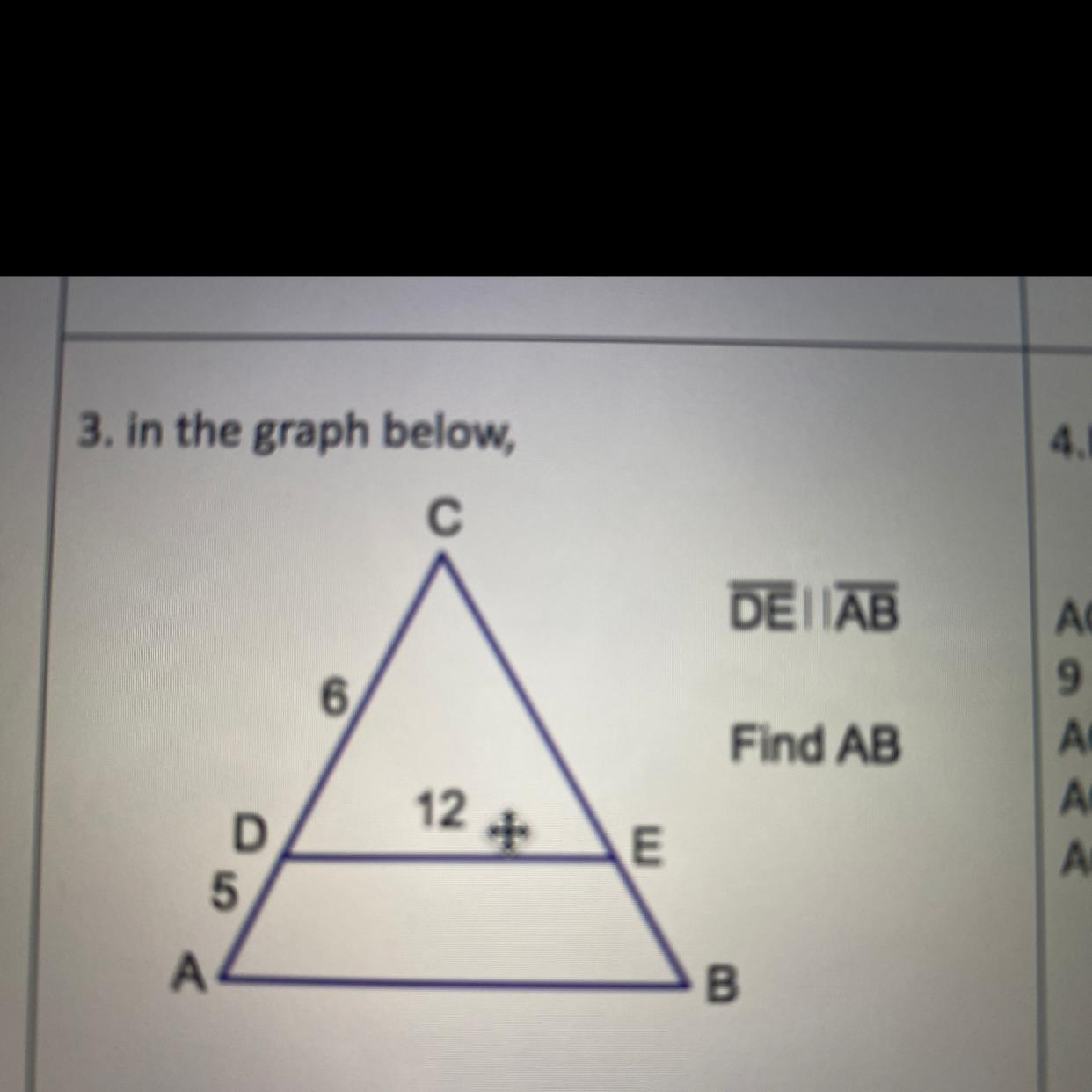
Answers
Answer:
DE/AB= DC/AC , 12/AB= 6/11 , AB= 12×11/6=2×11=22 , Sory i can't explain , i bad know english language. or so ABC~CDE because DE||AB=> DE/AB=DC/AC
A large company has two major departments, Development and Marketing. Twelve employees are randomly selected from each department, and the age of each employee, in years, is recorded in the accompanying samples. Both departments have an employee who is 56 years old. In which department is it more unusual to have a 56-year-old employee
Answers
The given company has two major departments: Development and Marketing. A total of twelve employees are selected randomly from each of the department and their ages are recorded. It is also mentioned that both of the departments have an employee who is of age 56 years. It is equally unusual to have a 56-year-old employee in both the departments.
To solve this problem, we need to determine the spread of the ages in each department. The spread of the ages in each department can be determined by calculating the range. The range is the difference between the maximum age and the minimum age in each department. We will then compare the range in both departments. The department with the greater range will be considered to be more unusual to have a 56-year-old employee.
The following are the ages of the employees in the Development and Marketing departments respectively: Development Department: 43, 24, 38, 56, 29, 22, 45, 47, 40, 33, 28, 30Marketing Department: 41, 56, 35, 23, 26, 49, 42, 50, 38, 29, 55, 46The range of the Development department can be calculated as: Range = Maximum Age - Minimum Age= 56 - 22= 34The range of the Marketing department can be calculated as: Range = Maximum Age - Minimum Age= 56 - 23= 33As we can see, the range in both departments is close. The range of the Development department is 34 and the range of the Marketing department is 33.
Therefore, it is difficult to determine which department is more unusual to have a 56-year-old employee. Hence, the answer is that it is equally unusual to have a 56-year-old employee in both the departments.
Let's learn more about Development :
https://brainly.com/question/24462624
#SPJ11
A diner served 5 pizzas during lunch and 3 during dinner today.
It served 8 ple slices yesterday. How many pizzas in all were served ?
Answers
This question's answer is 2 pizzas in total
In all= +
5+3=8
8+8=16
Which of the following equations shows a directly proportionate relationship?
y = -4x
y = 3x - 1
y = 2.75x - 3
y = 7x
Answers
Answer:
first and third
Step-by-step explanation:
the equation representing direct proportion is
y = kx ← k is the constant of proportion
y = - 4x is in this form with k = - 4
y = 7x is in this form with k = 7
Find the missing values in the ratio table. Then write the equivalent ratios in the order they appear in the table. Tea (cups) $3.75$ Milk (cups) $1.5$ $1$ $3.5$ $2.5$
Answers
The required missing values are $2.5 when the cup of milk is 1, $8.75 when the cup of milk is 3.5, and $6.25 when the cup of milk is 2.5.
The table is given in the question as :
Tea (cups) $3.75
Milk (cups) $1.5 $1 $3.5 $2.5
Let the missing value cup of tea would be x when the cup of milk is 1,
The missing value cup of tea would be y when the cup of milk is 3.5,
And the missing value cup of tea would be z when the cup of milk is 2.5,
According to the given question, we can write the ratio as:
$3.75 Tea (cups) : $1.5 Milk (cups) = x Tea (cups) : $1 Milk (cups)
⇒ 3.75 / 1.5 = x / 1
⇒ x = 3.75 / 1.5
⇒ x = $2.5
$3.75 Tea (cups) : $1.5 Milk (cups) = y Tea (cups) : $3.5 Milk (cups)
⇒ 3.75 / 1.5 = y / 3.5
⇒ y = (3.75 / 1.5) × 3.5
⇒ y = 2.5 × 3.5
Apply the multiplication operation,
⇒ y = $8.75
$3.75 Tea (cups) : $1.5 Milk (cups) = z Tea (cups) : $2.5 Milk (cups)
⇒ 3.75 / 1.5 = y / 2.5
⇒ z = (3.75 / 1.5) × 2.5
⇒ z = 2.5 × 2.5
Apply the multiplication operation,
⇒ z = $6.25
Thus, the required missing values are $2.5 when the cup of milk is 1, $8.75 when the cup of milk is 3.5, and $6.25 when the cup of milk is 2.5.
Learn more about the Ratio here:
brainly.com/question/1504221
#SPJ1
Three trolley tours leave from the same stop at 9:00 am. Tour A returns to the stop every 75 minutes, Tour B returns every 60 minutes, and Tour C returns every 40 minutes. In how many hours will all three tours return to the stop at the same time?
Answers
Given :
Three trolley tours leave from the same stop at 9:00 am.
Tour A returns to the stop every 75 minutes, Tour B returns every 60 minutes, and Tour C returns every 40 minutes.
To Find :
In how many hours will all three tours return to the stop at the same time.
Solution :
We know, they will return to the stop at the same time is the L.C.M of three i.e 75, 60 and 40 minutes.
So, their L.C.M is :
L.C.M = 600 minutes.
Now, 600 minutes = 600÷60 hours = 10 hours.
Therefore, they will all meet at stop in 10 hours.
Hence, this is the required solution.
The price Renu pays to visit the vet's clinic is calculated according to the formula 30 + 60n. Here n is the number of times she visits him in a year. This time it was 2. What was the cost of his visit?
Answers
Answer:
150
Step-by-step explanation:
30+60n=30+120=150
Please help me answer these 2 questions with a FULL explanation so I know how to do the rest myself. Giving brainliest.

Answers
Answer to 1:
\(\frac{x^3}{2y^25}\)
First thing you want to do is combine like terms on the bottom. Since it's multiplication, all you have to do is add the exponents. So for x you'd get x raised to the -9 power and for y you'd get 2y raised to the power of 7
Second thing is the numerator of the fraction. Since this is raising what is inside the paranthesis to a power, you must multiply this power by the powers of x and y, respectively. You should get x raised to the power of -6 and y raised to the power of -18
Here's where it gets complicated. to rationalize the problem, you must add parts from the numerator and denominator or vice versa. You cannot have any negative exponents anywhere in the fraction. So that means for x you have to add 9 to the x on the top to cancel out the -6 and rationalize the remaining x's. You should end up with x^3.
For y, you work the opposite way. Since the negatives are only on the top, you simply add 18 to the bottom y's and it remains rationalized.
If done correctly this should come out to x^3/2y^25
Answer to 2:
(x^20y^12)/256
First thing you can do on this one is to cancel out the x^0s. Anything to the power of 0 equals one so only the coefficients will remain. Multiply those together and you'll get 4.
Next you will rationalize the ys. Add the bottom 3 to the top to cancel out the denominator and you're left with y^-3. Leave it like this for now.
Now is the difficult part. You must take everything that is inside the parenthesis to the power of -4. That includes the coefficients and the exponents. 4^-4 = 1/256. This means that you now must move the coefficients to the bottom. You should currently have y^-3/(256x^5).
Now take the exponents to the power using the same rules as question 1. -3*-4 = 12 and 5*-4 = -20. You should now have y^12/(256x^-20)
Lastly, we must rationalize the denominator. To do so, move the x^-20 to the numerator and make the exponent positive. After doing this, you have the answer: (x^20y^12)/256
What is the height H of the roof?.
Answers
Answer:
Step-by-step explanation:
Per ASCE 7, the Mean Roof Height (h) is defined as the average of the roof eave height and the height to the highest point on the roof surface, except that, for roof angles of less than or equal to 10°, the mean roof height is permitted to be taken as the roof eave height
i hope it helps
SUCHE To test the hypothesis that the population mean mu-17.4, a sample size n-11 yields a sample mean 18.641 and sample standard deviation 1.905. Calculate the P value and choose the correct conclusion Yanıtınız: The P-value 0.009 is not significant and so does not strongly suggest that mu-17.4. The P-value 0.009 is significant and so strongly suggests that mu>17.4 The P-value 0.022 is not significant and so does not strongly suggest that mu-17.4. The P-value 0.022 is significant and so strongly suggests that mu-17.4 The P-value 0.004 is not significant and so does not strongly suggest that mu>17.4. The P-value 0.004 is significant and so strongly suggests that mu-17.4. The P-value 0.028 is not significant and so does not strongly suggest that mu-17 A. The P-value 0.028 is significant and so strongly suggests that mu-17.4. The P-value 0,003 is not significant and so does not strongly suggest that mu>17.4. The P-value 0.003 is significant and so strongly suggests that mu-17.4.
Answers
The correct conclusion is the P-value 0.028 is not significant and so does not strongly suggest that μ > 17.4
How to determine the P-valueFrom the information given, we have that;
Population mean, μ = 17.4,
sample mean = 18.641
Standard deviation (s = 1.905)
Sample size , n = 11
Using the the formula is given as;
t = (x - μ) / (s / √n)
Substitute the values, we have;
t = (18.641 - 17.4) / (1.905 / √11
t = 1.241/0.5743
Divide the values
t ≈ 2.161
Now, we have the degree of freedom as;
degree of freedom = 11 - 1 = 10
Using the t-distribution table or a statistical calculator, we have P-value as
P(0. 2151) = 0.028.
Then, we have to reject the hypothesis.
Learn more about P- value at: https://brainly.com/question/13786078
#SPJ4
What is the area of a rectangle with a length of 2 1/4 inches and a width of 2 3/4 inches
7 1/4in
6 3/16in
4 3/16in
3 3/2in
Answers
The area of a shape is the amount of space it occupies
The area of the rectangle is \(6\frac 3{16}\) inches square
How to determine the areaThe dimensions are given as:
\(Length= 2\frac 14\)
\(Width= 2\frac 34\)
The area is then calculated as:
\(Area = Length * Width\)
So, we have:
\(Area = 2\frac14 * 2\frac 34\)
This gives
\(Area = 6\frac 3{16}\)
Hence, the area of the rectangle is \(6\frac 3{16}\) inches square
Read more about areas at:
https://brainly.com/question/24487155
What is the scale factor in the dilation?

Answers
Answer:
3
Step-by-step explanation: