PLEASE HELP ME
A major shoe company is determines that to minimize costs, C for producing shoes is modeled by C = .1s2 - 2s +25. C is the total cost in billions of dollars and s is the number of units made in millions.
a) Find how many shoes (in millions)the company is able to produce while minimizing the cost.
b) Then determine the lowest cost (in billions) the company is able to achieve.
Answers
(a) The number of shoes (in millions)the company is able to produce while minimizing the cost is 1 million.
(b) The lowest cost (in billions) the company is able to achieve is 24 million.
Define quadratic function.f(x) = ax² + bx + c, where a, b, and c are integers and an is not equal to zero, is a quadratic function. A parabola is the shape of a quadratic function's graph.
The given function is
C = 1s² - 2s +25
(a) The number of shoes (in millions)the company is able to produce while minimizing the cost is given by
C(min+ = -b/2a
= -(-2)/2(1)
= 2/2
=1 million.
(b) The lowest cost (in billions) the company is able to achieve is given by
Put s =1 in the equation
C = 1(1)² - 2s +25
= 1 - 2 + 25
= 24 million
To know more about quadratic functions, visit:
https://brainly.com/question/27157081
#SPJ1
Related Questions
BC is tangent to OA at point B. Is each statement true for OA? Drag “true” or “False” to the end of each row

Answers
The answers are: BC || DE: False
∠ADE = ∠ACB: True
DE is tangent to A at point B: True
What is tangent in circle?In a circle, the tangent is a line that intersects the circle at exactly one point, called the point of tangency.
This point is where the tangent line is perpendicular to the radius of the circle that passes through it.
BC || DE: False (BC and DE are not parallel to each other)
∠ADE = ∠ACB: True (angles ADE and ACB are equal because they are opposite angles formed by a transversal intersecting two parallel lines)
DE is tangent to A at point : True (DE is tangent to A at point B, as given in the problem statement)
To know more about circle visit:
https://brainly.com/question/23265136
#SPJ1
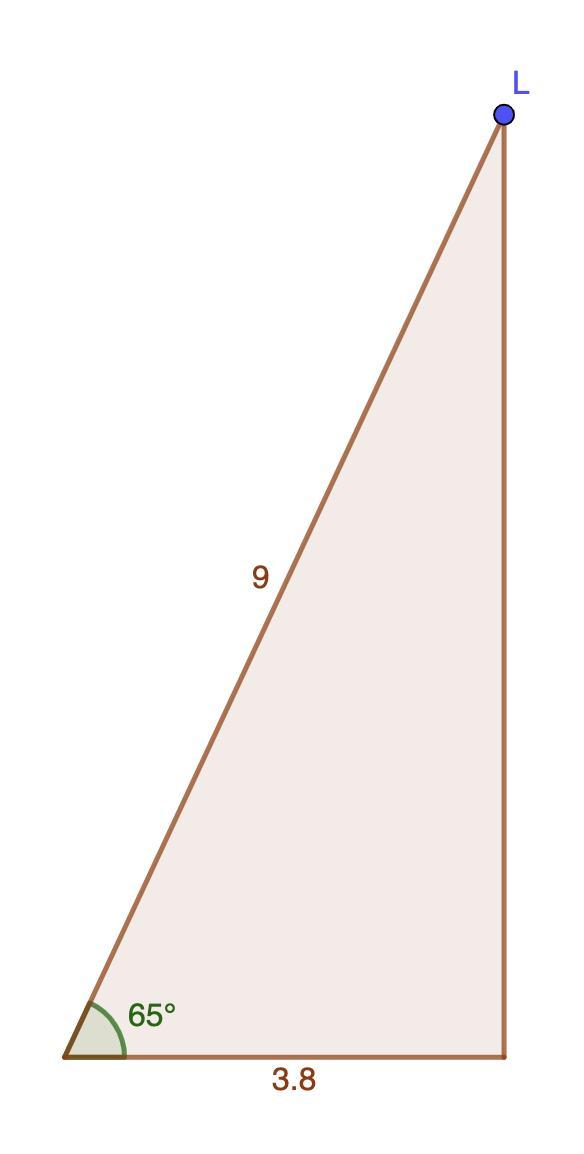
A ladder is leaning against a building forming an 65° angle with the ground. The length of the ladder is 9 ft. How far is the base of the ladder from the base of the building? Round your answer to the nearest tenth.
Answers
Answer:
3.8 feet
Step-by-step explanation:
You want to know the distance from a building to the base of a 9 ft ladder that makes a 65° angle with the ground.
CosineThe cosine relation is ...
Cos = Adjacent/Hypotenuse
In the right triangle that models this geometry, the hypotenuse is the length of the ladder (9 ft), and the distance from the base of the ladder to the base of the building is the side adjacent to the 65° angle.
Solving the above relation for the adjacent side, we have ...
Adjacent = Hypotenuse · Cos
distance to building = (9 ft)·cos(65°) ≈ 3.8 ft
The base of the ladder is about 3.8 ft from the base of the building.
What is a Type II Error?
Answers
A Type II Error, also known as a "false negative," is a statistical term used in hypothesis testing. In hypothesis testing, we start with two competing hypotheses: the null hypothesis (H0) and the alternative hypothesis (Ha).
The goal of hypothesis testing is to determine which hypothesis is more likely to be true given the data. A Type II Error occurs when we fail to reject the null hypothesis when it is actually false. In other words, we incorrectly conclude that the null hypothesis is true when it is actually false.
For example, consider a medical test for a certain disease. The null hypothesis is that the patient does not have the disease, and the alternative hypothesis is that the patient does have the disease.
If the test results are negative (i.e., the patient does not have the disease), and the patient actually does have the disease, then we have made a Type II Error.
The probability of making a Type II Error is represented by the Greek letter β (beta). A common goal in hypothesis testing is to keep the probability of making a Type II Error as low as possible, while also keeping the probability of making a Type I Error (false positive) low as well.
to know more about type 2 refer here
https://brainly.com/question/14778785#
#SPJ11
14129 Marks
***
Hamid has 20 T-shirts.
The information shows the colours of his T-shirts.
7 black
4 dark blue
4 red
1 light blue
2 white
2 grey
Hamid is going to take one of his T-shirts at random.
a) What is the probability that the T-shirt will be grey?
b) What is the probability that the T-shirt will not be red?
c) He takes one of his blue T-shirts at random.
What is the probability that the T-shirt is light blue?
Answers
Hamid is going to take one of his T-shirts at random.
The probability of selecting a light blue T-shirt is \(\frac{1}{4}\) or \(0.25\).
a) To find the probability that the T-shirt will be grey, we need to determine the ratio of grey T-shirts to the total number of T-shirts.
From the given information, we know that Hamid has 2 grey T-shirts out of a total of 20 T-shirts.
Therefore, the probability of selecting a grey T-shirt at random is \(\frac{2}{20}\), which simplifies to \(\frac{1}{10}\) or \(0.1\).
b) To find the probability that the T-shirt will not be red, we need to determine the ratio of non-red T-shirts to the total number of T-shirts.
Hamid has 4 red T-shirts, so the number of non-red T-shirts is
\(20 - 4 = 16\).
Therefore, the probability of selecting a T-shirt that is not red is \(\frac{16}{20}\), which simplifies to \(\frac{4}{5}\) or \(0.8\).
c) If Hamid takes one of his blue T-shirts at random, we need to consider the ratio of blue T-shirts to the total number of T-shirts.
From the information provided, we know that Hamid has a total of 4 blue T-shirts.
Therefore, the probability of selecting a blue T-shirt at random is \(\frac{4}{20}\), which simplifies to \(\frac{1}{5}\) or \(0.2\).
d) If Hamid takes one of his blue T-shirts at random, the probability that the T-shirt is light blue depends on the ratio of light blue T-shirts to the total number of blue T-shirts.
From the information provided, there is only 1 light blue T-shirt and a total of 4 blue T-shirts.
Hence, the probability of selecting a light blue T-shirt is \(\frac{1}{4}\) or \(0.25\).
For such more questions on probability
https://brainly.com/question/30390037
#SPJ8
You roll a standard, six-sided number cube. What is the probability of rolling a prime number
or a number greater than 2?
Answers
The probability of rolling a prime number is 50% since there are 6 sides on the cube and 3 of them contain a prime number.
The probability of rolling a number greater than 2 is 75% since there are 6 sides on the cube and 4 of them contain a number greater than 2.
suppose a triangle has sides 1,1,1 which of the following must be true
Answers
Answer:
1
Step-by-step explanation:
Find the volume of the composite figure round to the nearest tenth

Answers
Answer: \(1460.96ft^3\)
Step-by-step explanation:
You have a cylinder and a cuboid. Find each of these figures' volume individually and add them together.
Cylinder:
height = 4ft
radius = 4ft
\(Formula: V=\pi r^2h\)
\(V=(3.14)(4ft)^2(4ft)\\V=(3.14)(16ft^2)(4ft)\\V=(3.14)(64ft^3)\\V=200.96ft^3\)
---------------------------------------------------------------------------------------------------
Cuboid:
\(Formula: V = l*w*h\)
\(V=(15ft)(7ft)(12ft)\\V=1260ft^3\)
------------------------------------------------------------------------------------------------------
Add these two volumes together.
\(200.96ft^3+1260ft^3=1460.96ft^3\)
an arithmetic sequence $2,5,8, 11, 14,\ldots$ is written in order in a book, one hundred numbers per page, beginning on page one. on which page will the number $11{,}111$ appear? if you are having trouble with this question, read problem 10.4 in the textbook.
Answers
The page where the number 110 appears will be 37.
What is an arithmetic sequence?A series of integers called an arithmetic succession or arithmetic chain of events has a fixed difference between the terms.
Let a₁ be the first term and d be a common difference.
Then the nth term of the arithmetic sequence is given as,
aₙ = a₁ + (n - 1)d
An arithmetic sequence 2, 5, 8, 11, 14,... is written in the order in a book. Then the first term is 2 and the common difference is 3. Then the number of terms if the nth term is 110. Then we have
110 = 2 + (n - 1) x 3
108 = (n - 1) x 3
n - 1 = 36
n = 37
The page where the number 110 appears will be 37.
More about the arithmetic sequence link is given below.
https://brainly.com/question/12373434
#SPJ1
HURRY
what is the value of x

Answers
Answer:
x = 20
Step-by-step explanation:
7x - 3 and 6x + 17 are alternate exterior angles and are congruent , then
7x - 3 = 6x + 17 ( subtract 6x from both sides )
x - 3 = 17 ( add 3 to both sides )
x = 20
Answer Please ⠀⠀⠀⠀⠀⠀⠀⠀

Answers
Answer:
if;3=1.98 what about 2
3-1.98
2-?
2×1.98÷3=$1.32
Ans: 2 cans for $1.32I don't understand how to do this!

Answers
Answer:
\(\huge\boxed{m \ ||\ n}\)
Step-by-step explanation:
We can see that m∠8 and m∠12 are the exact same angles. This is because they are corresponding angles. In order for two angles to be the exact same that are near lines, the lines have to be parallel.
m∠8 is on line m. m∠12 is on line n. This means that lines m and n should be parallel.
Hope this helped!
Answer:
C is the correct answer.
Hope that answered your question. Please mark as Brainliest. Thank You! Have a great day!
HELPP PLS
similar triangles

Answers
The length BC for the similar triangle ∆ABC is derived to be equal to 20.
How to evaluate the for the length BC for the triangle ∆ABCThe triangles ABC and EBD are similar, this implies that the length AC of the smaller triangle is similar to the length ED of the larger triangle
similarly, BC is similar to BD so;
BC/(8 + BC) = 10/14
14BC = 10(8 + BC) {cross multiplication}
14BC = 80 + 10BC
14BC - 10BC = 80 {collect like terms}
4BC = 80
BC = 80/4 {divide through by 4}
BC = 20.
Therefore, the length BC for the similar triangle ∆ABC is derived to be equal to 20
Read more about similar triangles here:https://brainly.com/question/14285697
#SPJ1
List all sides and lengths
rhombus wxyz, wx = 4 cm
Answers
In the rhombus WXYZ, the length of one side, WX, is given as 4 cm. The other sides of the rhombus have the same length due to its properties, so all sides of the rhombus have a length of 4 cm.
A rhombus is a quadrilateral with all sides of equal length. In this case, the given length of one side, WX, is 4 cm. Since a rhombus has all sides congruent, we can conclude that all the sides of the rhombus WXYZ also have a length of 4 cm.
Hence, the lengths of all sides of the rhombus WXYZ are as follows:
WX = 4 cm
XY = 4 cm
YZ = 4 cm
ZW = 4 cm
All four sides of the rhombus WXYZ are equal in length and measure 4 cm.
Learn more about quadrilateral here:
https://brainly.com/question/29934440
#SPJ11
Х
Algebra Formative 10. 1-10. 3
Question 5 of 5
At a family reunion, family members are given the choice of swimming at the lake or going on a hike. The family constructed the following
frequency table to analyze the data. Complete the table.
Lake
Hike
Total
Children
6
Adults
9
Total
14
38
15
What does the relative frequency of
24
represent in the situation?
Answers
In the given frequency table, the relative frequency of 24 represents the proportion of family members who chose to go on a hike out of the total number of family members.
To calculate the relative frequency, we divide the frequency of the specific category (in this case, hike) by the total frequency. In this case, the frequency of the hike is 24, and the total frequency is 38.
Relative Frequency = Frequency of Hike / Total Frequency
Relative Frequency = 24 / 38
Simplifying the fraction, we get:
Relative Frequency ≈ 0.632
So, the relative frequency of 24 represents approximately 0.632 or 63.2%. This means that around 63.2% of the family members chose to go on a hike at the family reunion.
Learn more about relative frequency here:
https://brainly.com/question/28342015
#SPJ11
what is the value expression
2( X + 4) - (y * 8)
when x= 1/8
and y= 3/16
Answers
Answer:17/4
Step-by-step explanation:
Solution:
Your problem → 2((1/8)+ 4) - ((3/6)*8)
2((18)+4)-((36)⋅8)
=2⋅(1/8)+4-((36)⋅8)
=2⋅(1+4×8)/8-((36)⋅8)
=2⋅(1+32)/8-((36)⋅8)
=2⋅(33/8)-((36)⋅8)
=2⋅(33/8)-((36)⋅8)
=(33/4)-((36)⋅8)
=(33/4)-(36⋅8)
=(33/4)-(12⋅8)
=(33/4)-12⋅8
=(33/4)-4
=(33/4)-4
=(33-4×4)/4
=(33-16)/4
=17/4
=4.25 (in decimal)
Step-by-step explanation:
Your mother is very fond of collecting rare philodendron (an ornamental and
indoor plant). If she has a garden which measures 100 m2 (10 m by 10 m)
and each philodendron will occupy an estimated area of 0. 6 by 0. 6 m of the
garden, what is the maximum number of philodendron plants that will occupy
the whole area?
help me please
Answers
The maximum number of philodendron plants that will occupy the whole area is 256.
The term area in math is defined as the region bounded by the shape of an object
Here we have given that your mother is very fond of collecting rare philodendron and here we have know that If she has a garden which measures 100 m² and each philodendron will occupy an estimated area of 0. 6 x 0. 6 m of the garden.
Here we have to 10 meters divided by 0.6 of a meter gives 16 philodendrons in one direction and the same number in the other direction.
Then the maximum number of plants is calculated as,
=> 16 x 16 = 256
To know more about area here.
https://brainly.com/question/14994710
#SPJ4
Which values of x are solutions to the equation below 15x^2 - 56 = 88 - 6x^2?
a. x = -4, x = 4
b. x = -4, x = -8
c. x = 4, x = 8
d. x = -8, x = 8
Answers
A quadratic equation is a polynomial equation of degree 2, which means the highest power of the variable is 2. It is generally written in the form: ax^2 + bx + c = 0. Option (d) x = -8, x = 8 is the correct answer.
The given equation is 15x^2 - 56 = 88 - 6x^2.
We need to find the values of x that are solutions to the given equation.
Solution: We are given an equation 15x² - 56 = 88 - 6x².
Rearrange the equation to form a quadratic equation in standard form as follows: 15x² + 6x² = 88 + 56 21x² = 144
x² = 144/21 = 48/7
Therefore x = ±sqrt(48/7) = ±(4/7)*sqrt(21).
The values of x that are solutions to the given equation are x = -4/7 sqrt(21) and x = 4/7 sqrt(21).
To Know more about quadratic equation visit:
https://brainly.com/question/29269455
#SPJ11
The given equation is 15x² - 56 = 88 - 6x². Values of x are solutions to the equation below 15x² - 56 = 88 - 6x² are x = -2.62, 2.62 or x ≈ -2.62, 2.62.
Firstly, let's add 6x² to both sides of the equation as shown below.
15x² - 56 + 6x² = 88
15x² + 6x² - 56 = 88
Simplify as shown below.
21x² = 88 + 56
21x² = 144
Now let's divide both sides by 21 as shown below.
x² = 144/21
x² = 6.86
Now we need to solve for x.
To solve for x we need to take the square root of both sides.
Therefore, x = ±√(6.86).
Therefore, the values of x are solutions to the equation below are x = -2.62, 2.62 or x ≈ -2.62, 2.62.
To know more about solution, visit:
https://brainly.com/question/14603452
#SPJ11
help please will mark brainliest

Answers
Answer:
1.) 20z^5
2.) 8m^5 · 6p^7 · 3mp^0
Step-by-step explanation:
4z^3 · z^2 · 5z
first, multiply 4z · 1z = 4z and add the exponents 3 + 2 = 5
4z^5 · 5z
multiply 4z · 5z and keep the exponents
20z^5
2m^3p^4 · 4m^2p^3 · 3mp^0
find just the "m" variables: 2m^3 · 4m^2 and same as before multiply then add the exponents = 8m^5
now do the same for the "p" variables: 3p^4 · 2p^3 = 6p^7
and for the "mp" variable: 3mp^0
put it all together and this is the simplest form because we don;t know the values of the variables:
8m^5 · 6p^7 · 3mp^0
Hope this helps! If you have any additional questions please don't hesitate to ask me or your teacher to be sure you master the subject. :) Stay safe and please mark brainliest!
2 (4 minus 3 x) + 5 (2 x minus 3) = 20 minus 5 x?
Answers
Answer:
x=3
Step-by-step explanation:
2(4-3x)+5(2x-3)=20-5x
8-6x + 10x - 15= 20-5x
subtract 8 from 15
subtract 6x from 10x getting you
4x-7= 20-5x
add 5x to both sides
add 7 to both sides
getting you
9x=27
divide by 9 to both side getting you x=3
Consumers in a certain state can choose between three long-distance telephone services: GTT, NCJ, and Dash. Aggressive marketing by all three companies results in a continual shift of customers among the three services. Each year, GTT loses 20% of its customers to NCJ and 15% to Dash, NCJ loses 5% of its customers to GTT and 5% to Dash, and Dash loses 25% of its customers to GTT and 15% to NCJ. Assuming that these percentages remain valid over a long period of time, what is each company's expected market share in the long run?
GTT's expected market share:
NCJ's expected market share:
Dash's expected market share:
Answers
GTT's expected market share is 45.45%, NCJ's expected market share is 31.82%, and Dash's expected market share is 22.73%. these percentages add up to 100%, as expected.
To find the long-run expected market share for each company, we need to use the concept of steady-state or equilibrium. In the long run, the market share of each company will remain constant if the number of customers gained is equal to the number of customers lost. This means that the rate of change of each company's market share will be zero.
Let's define the market share of each company at any point in time as follows:
GTT's market share = SGTT
NCJ's market share = SNCJ
Dash's market share = SDash
We can write the equations for the rate of change of each company's market share as follows:
dSGTT/dt = -0.2 SGTT + 0.05 SNCJ + 0.25 SDash
dSNCJ/dt = -0.05 SNCJ + 0.05 SGTT + 0.15 SDash
dSDash/dt = -0.15 SDash + 0.25 SGTT + 0.15 SNCJ
Note that the negative coefficients represent the percentage of customers lost by the company, and the positive coefficients represent the percentage of customers gained by the company.
To find the steady-state values of SGTT, SNCJ, and SDash, we need to set the rate of change of each company's market share to zero:
-0.2 SGTT + 0.05 SNCJ + 0.25 SDash = 0
-0.05 SNCJ + 0.05 SGTT + 0.15 SDash = 0
-0.15 SDash + 0.25 SGTT + 0.15 SNCJ = 0
We can solve these equations to get the steady-state values of SGTT, SNCJ, and SDash:
SGTT = 0.4545
SNCJ = 0.3182
SDash = 0.2273
Therefore, the expected long-run market share for each company is as follows:
GTT's expected market share: 45.45%
NCJ's expected market share: 31.82%
Dash's expected market share: 22.73%
Therefore, these percentages add up to 100%, as expected.
for such more question on equilibrium
https://brainly.com/question/29398344
#SPJ11
in a distribution, a random variable can take any value in a specified range. group of answer choices discrete probability cumulative relative frequency continuous probability
Answers
In a continuous probability distribution, a random variable can take any value in a specified range. (Option D)
A probability distribution refers to the mathematical function that provides the probabilities of occurrence of different possible outcomes for an experiment. Continuous probability distribution refers to a probability distribution with continuous types of data or random variables where the random variable can take on any value and is continuous. As there are infinite values that the variable can assume, the probability of the variable assuming any one specific value is zero. A continuous distribution is characterized by a range of values that are infinite, and therefore uncountable. The normal distribution is one example of a continuous distribution.
Learn more about Continuous probability distribution:
https://brainly.com/question/25023966
#SPJ4
Complete the following table with the properties used to solve 4(x+3) = 20
Answers
Answer:
4(x+3) = 20
Distrbute
4x+12 = 20
-12 from both sides
4x = 8
divide both sides by 4
x = 2
Step-by-step explanation:
James wants to draw a line diagonally across a rectangular piece of paper. If the paper measures 9 inches by 12 inches, what
is the minimum length of the straight edge he needs to make the line?
15 inches
21 inches
3 inches
108 inches
Answers
pls help me with this maths question, I forgot how to do it :))

Answers
Step-by-step explanation:
thats the answer i think


A function g(x) has x-intercepts at (startfraction 1 over 2 endfraction, 0) and (6, 0)
Answers
The x-intercepts of the function g(x) are at (1/2, 0) and (6, 0).
1. The x-intercepts occur when the value of g(x) is equal to zero.
2. So, we set g(x) equal to zero and solve for x.
3. By setting g(x) equal to zero, we get the equations:
g(1/2) = 0 and g(6) = 0.
To find the x-intercepts of the function g(x), we need to solve the equation g(x) = 0.
By substituting the given values, we get g(1/2) = 0 and g(6) = 0.
Setting g(x) equal to zero, we can solve for x. At x = 1/2 and x = 6, the value of g(x) is equal to zero, which means the function intersects the x-axis at these points.
Therefore, the x-intercepts of the function g(x) are at (1/2, 0) and (6, 0).
To learn more about function
https://brainly.com/question/30721594
#SPJ11
Number 4, please help

Answers
Hi there!
From the given conditions:
\(1→1 ⟹1 \times 1 = 1\)
\(5→7⟹ 5 \times 7 = 35\)
\(8→11 ⟹8 \times 11 = 88\)
\(9→4 ⟹9 \times 4 = 36\)
\(10 →2 ⟹10 \times 2 = 20\)
Sum = 180, no. of customers = 25
\(average = \frac{sum}{no.ofcustomers} \)
\( ⟹ \frac{180}{25} = 7.2\)
Therefore, on average, the satisfaction rating of the landscape company was 7.2
1 2/5 a = 19 1/4 please help!
Answers
Answer:
a=13 3/4 (13.75)
Step-by-step explanation:
1 2/5a=19 1/4
a=19 1/4÷1 2/5
a=19 1/4÷7/5
a=19 1/4x5/7
a=77/4x5/7
a=385/28
a=13 21/28
a=13 3/4
a number greater than 2
Answers
Step-by-step explanation:
3
???
is that really the answer you are looking for ? there are infinite possibilities.
Answer:
\(\bold{Infinitely~many!}\)
Step-by-step explanation:
Hi there!
Remember. there are infinitely many numbers.
Here are some numbers greater than 2:
3, 4, ...400, ...5000, ...
It goes on for ever...
Hope it helps! Enjoy your day!
~Just a teenager girl willing to help
\(\bold{Besties4Ever}\)
Let A be a 4 X 5 matrix: If a1, a2, and a4 are linearly independent and a3 = a1 + 4a2, a5 -2a1 a2 + 3a4 determine the reduced row echelon form of A
Answers
The reduced row echelon form of the \(4\)×\(5\) matrix is
\(\left[\begin{array}{ccccc}1&0&1&0&-3\\0&1&5&0&-1\\0&0&0&1&4\\ 0&0&0&0&0\end{array}\right]\)
What is reduced row echelon form ?
When all of a matrix's pivots equal 1, and the pivots are the only non-zero elements in the fundamental columns, we say that the matrix is in reduced row echelon form.
Given that \(a_{1} ,a_{2}\) and \(a_{3}\) will be the independent vector, lets select them as the basis vector.
\(a_{1} =\left[\begin{array}{c}1&0&0\\0\end{array}\right]\) , \(a_{0} =\left[\begin{array}{c}0&1&0\\0\end{array}\right]\)
\(a_{3}= a_{1} +5a_{2}\)
= \(\left[\begin{array}{c}1&0&0\\0\end{array}\right]+5\left[\begin{array}{c}0&1&0\\0\end{array}\right]\)
=\(\left[\begin{array}{c}1&5&0\\0\end{array}\right]\)
\(a_{4}\) = \(\left[\begin{array}{c}0&0&0\\1\end{array}\right]\) and
\(a_{5} = -3a_{1} -a_{2} +4a_{4}\)
= \(-3\left[\begin{array}{c}1&0&0\\0\end{array}\right]-\left[\begin{array}{c}0&1&0\\0\end{array}\right]+4\left[\begin{array}{c}0&0&0\\1\end{array}\right]\)
= \(\left[\begin{array}{c}-3&-1&0\\4\end{array}\right]\)
Hence the \(4\)×\(5\) matrix will be in the form
\(\left[\begin{array}{ccccc}1&0&1&0&-3\\0&1&5&0&-1\\0&0&0&0&0\\ 0&0&0&1&4\end{array}\right]\)
Since the one complete row is already zero, hence for calculating the row reduced echelon form we just need to replace the 3rd and 4th row.
\(\left[\begin{array}{ccccc}1&0&1&0&-3\\0&1&5&0&-1\\0&0&0&1&4\\ 0&0&0&0&0\end{array}\right]\)
To know more about reduced row echelon form from the given link
https://brainly.in/question/11967829
#SPJ4
The reduced row echelon form of the4 × 5matrix is
\(\left[\begin{array}{ccccc}1&0&1&0&-3\\0&1&5&0&-1\\0&0&0&1&4\\0&0&0&0&0\end{array}\right]\)
What is reduced row echelon form ?When all of a matrix's pivots equal 1, and the pivots are the only non-zero elements in the fundamental columns, we say that the matrix is in reduced row echelon form.
Given that a1,a0,and a3 will be the independent vector, lets select them as the basis vector.
\(a_{1}=\left[\begin{array}{c}1&0&0&0\end{array}\right]\), \(a_{0}=\left[\begin{array}{c}0&1&0&0\end{array}\right]\)
a3 = a1 + 5a0
\(\left[\begin{array}{c}1&0&0&0\end{array}\right]+5\left[\begin{array}{c}0&1&0&0\end{array}\right]\)
\(a_{4}= \left[\begin{array}{c}0&0&0&1\end{array}\right]\)
a5 = -3a1 - a2 + 4a4
\(-3\left[\begin{array}{c}1&0&0&0\end{array}\right]-\left[\begin{array}{c}0&1&0&0\end{array}\right]+4 \left[\begin{array}{c}0&0&0&1\end{array}\right]\)
\(=\left[\begin{array}{c}-3&-1&0&4\end{array}\right]\)
Hence the × matrix will be in the form
\(=\left[\begin{array}{ccccc}1&0&1&0&-3\\0&1&5&0&-1\\0&0&0&0&0\\0&0&0&1&4\end{array}\right]\)
Since the one complete row is already zero, hence for calculating the row reduced echelon form we just need to replace the 3rd and 4th row.
To know more about reduced row echelon form from the given link
brainly.com/question/14693506
#SPJ4
Find a vector function r(t), that represents the curve of intersection of the two surfaces. the cylinder x2 y2=36 and the surface z=4xy
Answers
Given:
\(\begin{aligned}&x^2+y^2=16 \\&z=x y\end{aligned}\)
Express 16 as \(4^{2}\): \(x^2+y^2=16\)
\(x^2+y^2=4^2\\x^2+y^2=4^2 \times 1\)
Trignometry,
\(\cos ^2(t)+\sin ^2(t)=1\)
Now, substitute \(\cos ^2(t)+\sin ^2(t)\) for 1:
\(\begin{aligned}&x^2+y^2=4^2 \times 1 \\&x^2+y^2=4^2 \times\left[\cos ^2(t)+\sin ^2(t)\right]\end{aligned}\\x^2+y^2=4^2 \times \cos ^2(t)+4^2 \times \sin ^2(t)\)
Law of indicates:
\(\begin{aligned}&x^2+y^2=[4 \times \cos (t)]^2+[4 \times \sin (t)]^2 \\&x^2+y^2=[4 \cos (t)]^2+[4 \sin (t)]^2\end{aligned}\\x^2=[4 \cos (t)]^2 \text { and } y^2=[4 \sin (t)]^2\)
Taking positive square roots as follows:
\(x=4 \cos (t), y=4 \sin (t)\)
Recall that, z = xy.
Now, we have:
\(\begin{aligned}&z=4 \cos (t) \times 4 \sin (t) \\&z=16 \cos (t) \cdot \sin (t)\end{aligned}\)
Now, substitute the values:
\(r(t)=x_t i+y_t j+z_t k\)
So, the vector r(t) is: \(r(t)=(4 \cos (t)) i+(4 \sin (t)) i+(16 \cos (t) \cdot \sin (t)) i\)
Therefore, the vector function r(t) is written as: \(r(t)=x_t i+y_t j+z_t k\)
Know more about vector functions here:
https://brainly.com/question/14895420
#SPJ4