is y=-x+11 a function
Answers
Answer:
Yes
Step-by-step explanation:
It is a straight line so no x values repeat
Answer:
Yes it is
Step-by-step explanation:
You can prove this is a function using the vertical line test. You have to graph y= -x+11 and do the vertical line test. As long as it doesn't interfere with the line more than once, than it is a function.
Related Questions
Write Expressions Word Problems 1.
You run a small business and would like to print some business cards.
Staples offers color business cards for a small fee.
The cost is $.75 per card.
A) Write an expression for the total cost (in dollars) if you print b business cards. B) How much does it cost you to print 50 business cards?
Answers
Step-by-step explanation:
cost of business card - $.75 ( per card )
I want to print 50
So we will multiply 75 × 50
75
×50
00
355×
=375
$.375 will be the cost of the same
A rectangular garden has a perimeter of 578 yds.
The length is one more than three times the width.
Find the dimensions of the garden.
Answers
Answer:
Width (w) = 72 yds
Length (L) = 217 yds
Step-by-step explanation:
Let L represent the length.
Let w represent the width.
From the question given above:
Perimeter (P) = 578 yds.
Length (L) is one more than three times the width (w). This can be written as. Follow:
three times the width (w) = 3w
Therefore, the length (L) is given by:
L = 1 + 3w
Next, we shall determine the value of length (L) and width (w). This can be obtained as follow:
Recall:
Perimeter of rectangle is given by:
P = 2(L + w)
P = 2L + 2w
Perimeter (P) = 578 yds.
Length (L) = 1 + 3w
Width (w) = w
P = 2L + 2w
578 = 2(1 + 3w) + 2w
Clear bracket
578 = 2 + 6w + 2w
578 = 2 + 8w
Collect like terms
578 - 2 = 8w
576 = 8w
Divide both side by 8
w = 576/8
w = 72
Therefore, the width is 72 yds
L = 1 + 3w
w = 72
L = 1 + 3(72)
L = 1 + 216
L = 217
Therefore, the length is 217 yds.
The dimensions of the garden is given below:
Width (w) = 72 yds.
Length (L) = 217 yds.
determine a formula for ∫ x^ne^xdx in terms of x^ne^x -n ∫ x^n−1e^xdx, where n is a positive integer
Answers
The formula for integral ∫ xⁿeˣdx in terms of xⁿeˣ and n ∫ xⁿ⁻¹eˣdx is ∫ xⁿeˣdx = xⁿeˣ - n ∫ xⁿ⁻¹eˣdx
This formula uses integration by parts, where u = xⁿ and dv/dx = eˣdx. By applying the product rule, we get du/dx = nxⁿ⁻¹ and v = eˣ.
Using this formula, we can simplify the integration process for functions with the form xⁿeˣ. Instead of having to integrate the entire function, we can break it down into smaller parts that are easier to integrate. This can save time and make the integration process more manageable.
Overall, this formula is a useful tool for solving integrals involving xⁿeˣ functions. By breaking down the function into simpler parts, we can solve the integral more easily and efficiently.
To know more about integration by parts click on below link:
https://brainly.com/question/14402892#
#SPJ11
7 is 1/10 of
pls help and thank you :)
Answers
7 is 1/10 of 70. You can figure this out by multiplying 7 by 10
Samir, a carpenter, spent $300 on specialty tools to make toy horses for children. For each horse he makes, he spends $75 on supplies. He charges $125 for each horse. How many horses must Samir sell in order to break even?
Answers
Answer:
Samir must sell 6 horses in order to break even.
The number of horses that Samir must sell in order to break even is 6 horses.
Calculations and Parameters:Given:
Fixed cost = $300Variable cost = $75 on each supplyRevenue = $125 on each horse.To find breakeven, we must remember that the revenue and cost are equal.
Therefore, we create a function and use this to solve:
125x= 300 + 75x
125x-75x = 300
50x = 300
x= 6
Read more about breakeven here:
https://brainly.com/question/24150813
#SPJ9
in a blueprint, each square has a side length of 1/4 inch. a reduced drawing of a blueprint. the bedroom has 5 square along the length and 4 squares along the width. the bathroom has 4 squares along the length and 2 squares along the width. the living room has 7 squares along the length and 4 squares along the width. a. ceramic tile costs $5 per square foot. how much does it cost to tile the bathroom? it would cost $ to tile the bathroom. b. carpet costs $18 per square yard. how much does it cost to carpet the bedroom and living room? it would cost $ to carpet the bedroom and living room. skip to navigation
Answers
The total cost to put tile in bathroom and carpet in bedroom and living room as the calculated area is equal to $640 and $1535.94.
As given in the question ,
Scale measure of blue print = 1/4 inch
1 inch = 4 ft
Area wise = 4 × 4
= 16 square feet
Dimensions of bathroom are:
length = 4 squares
= 4 × 4
= 16ft
and width = 2squares
= 8ft
Area of bathroom = (16 × 8)
= 128 square feet
Cost of ceramic tile is $5 per square foot
Cost to put tiles in a bathroom = 128 × 5
= $640
Area of bedroom = ( 5×4) ×(4×4)
= 320 square foot
Area of living room = ( 7×4) ×(4×4)
= 448 square foot
Total area of (bedroom and living room) = 768 square feet
= 85.33 square yards
Cost of a carpet is $18 per square yard
Total cost to use carpet for bedroom and living room
= ( 85.33) × 18
= $1535.94
Therefore, the cost to tile a bathroom as per calculated area is $640 and similarly cost to put carpet in bedroom and living room is $1535.94.
Learn more about area here
brainly.com/question/27683633
#SPJ4
This recipe makes 4 pancakes.
Sanjay follows the recipe but wants to make 24 pancakes.
How much of each ingredient does he need?
Recipe: Makes 4
135 g flour
1 teaspoon (tsp) baking powder
2 tablespoons (tbsps) sugar
130 ml milk
1 egg
2 tablespoons (tbsps) oil
Answers
Answer:
810 g flour
6 teaspoon (tsp) baking powder
12 tablespoons (tbsps) sugar
780 ml milk
6 egg
12 tablespoons (tbsps) oil
Step-by-step explanation:
24/4=6, so you need to multiply everything by six
what is the gram matrix of this bilinear form (with respect to the standard basis)
Answers
To find the Gram matrix of a bilinear form with respect to the standard basis, we need to compute the matrix that represents the bilinear form. A bilinear form is a function that takes two vectors as input and returns a scalar value, and it is linear in each of its arguments.
In this case, you haven't provided the specific bilinear form you'd like to compute the Gram matrix for. However, I can explain the general steps to find it:
1. Define the bilinear form, say B(u, v), where u and v are vectors from a vector space.
2. Choose the standard basis for the vector space, e.g., {e1, e2, ..., en}.
3. Compute the matrix elements using the bilinear form and the standard basis vectors: Gij = B(ei, ej), where Gij is the element in the ith row and jth column of the Gram matrix.
4. Form the matrix G using the computed elements Gij.
Learn more about matrix:
https://brainly.com/question/28180105
#SPJ11
ronnie is playing poker and is dealt his hand of 5 cards from a standard 52-card deck. what is the probability that ronnie is dealt 1 diamond, 1 club, 1 heart, and 2 spades?
Answers
The probability of being dealt 1 diamonds, 1 clubs, 1 hearts, and 2 spade is approximately 0.065936 .
There are several steps involved in calculating the probability of being dealt a specific hand of 5 cards from a standard 52 card deck. We can use the formula for the probability of a combination:
P(combination) = (number of ways to get the combination) / (total number of possible hands)
In this case, we want to find the probability of being dealt 1 diamonds, 1 clubs, 1 hearts, and 2 spade.
Number of ways to get this combination:
Choose 1 diamonds out of 13: C(13,1) = 13
Choose 1 clubs out of 13: C(13,1) = 13
Choose 1 hearts out of 13: C(13,1) = 13
Choose 2 spade out of 13: C(13,2) = 78
Total number of possible hands:
Choose 5 cards out of 52: C(52,5) = 2598960
Putting it all together:
P(1 diamonds, 1 clubs, 1 hearts, 2 spade) = (13 * 13 * 13 * 78) / 2598960
= 0.065936
Learn more about probability here :-
https://brainly.com/question/11234923
#SPJ4
11. Engineering The maximum load for a certain elevator is 2000 pounds. The total
weight of the passengers on the elevator is 1400 pounds. A delivery man who weighs
243 pounds enters the elevator with a crate of weight w. Write, solve, and graph an
inequality to show the values of w that will not exceed the weight limit of the elevator.
Answers
The inequality to show the values of [w] that will not exceed the weight limit of the elevator is w + 1643 ≤ 2000. On solving the inequality, we get w ≤ 357. The graph of the inequality is attached.
What is inequality?In mathematics, an inequality is a relation which makes a non-equal comparison between two numbers or other mathematical expressions. It is used most often to compare two numbers on the number line by their size.An inequality is a mathematical relationship between two expressions and is represented using one of the following -≤ : less than or equal to
≥ : greater than or equal to
< : less than
> : greater than
≠ : not equal to
Given is the maximum load for a certain elevator is 2000 pounds. The total weight of the passengers on the elevator is 1400 pounds. A delivery man who weighs 243 pounds enters the elevator with a crate of weight [w].
We can write the inequality as follows -1400 + 243 + w ≤ 2000
w + 1643 ≤ 2000
Solving the inequality, we get -w + 1643 ≤ 2000
w ≤ 2000 - 1643
w ≤ 357
Refer to the graph attached.Therefore, the inequality to show the values of [w] that will not exceed the weight limit of the elevator is w + 1643 ≤ 2000. On solving the inequality, we get w ≤ 357. The graph of the inequality is attached.
To solve more questions on inequalities, we get -
https://brainly.com/question/11897796
#SPJ1

A fair coin is flipped three times. Find the
probability of getting heads all three times.
Answers
Answer:
13/100, or 0.13%.
Step-by-step explanation:
You have a fair coin: this means it has a 50% chance of landing heads up and a 50% chance of landing tails up. You flip it three times and these flips are independent. What is the probability that it lands heads up all three times, 0.13.
ABCD is a trapezium with AB parallel to DC.
DC= 15 cm and AB= x cm
The perpendicular distance between AB and DC is 3 cm less than the length of AB. The area of ABCD is 75 cm square.
i) show that x2 + 12x - 195= 0
Answers
Answer:
Step-by-step explanation:a or b ?
Helppppppp
Find rational zeros of each polynomial function, then write each function in factored form.
f(x)= x3 + 2x2 – 13x+10
Answers
Answer:
The rational zeros of the polynomial function f(x) = x^3 + 2x^2 - 13x + 10 are x = -1, x = 1, and x = 10/3.
Explanation:
To find the rational zeros of the polynomial function f(x) = x^3 + 2x^2 - 13x + 10, we can use the rational zero theorem, which states that if p/q is a rational zero of a polynomial function f(x) with integer coefficients, then p is a factor of the constant term of f(x) (in this case, 10) and q is a factor of the leading coefficient (in this case, 1).
We can then use this information to create a list of potential rational zeros by listing out the factors of 10 (which are -1, 1, -2, 2, -5, 5, and -10) and dividing them by the factors of 1 (which are -1 and 1). This gives us the following list of potential rational zeros: -1, 1, -2, 2, -5, 5, and -10.
To determine which of these values are actually zeros of the polynomial function, we can substitute each value into the function and see if it makes the function equal to 0. We find that x = -1, x = 1, and x = 10/3 are zeros of the function, but the other values are not.
We can then use these zeros to factor the polynomial function:
f(x) = (x + 1)(x - 1)(x - 10/3)
This is the factored form of the polynomial function.
4. Show that the matrix [XX-X'Z(ZZ)-¹Z'X). where both the x & matrix X and the x matrix Z. have full column rank and m2, is positive definite. Discuss the implications of this result in econometrics.
Answers
To show that the matrix A = [XX - X'Z(ZZ)^(-1)Z'X] is positive definite, we need to demonstrate two properties: (1) A is symmetric, and (2) all eigenvalues of A are positive.
Symmetry: To show that A is symmetric, we need to prove that A' = A, where A' represents the transpose of A. Taking the transpose of A: A' = [XX - X'Z(ZZ)^(-1)Z'X]'. Using the properties of matrix transpose, we have:
A' = (XX)' - [X'Z(ZZ)^(-1)Z'X]'. The transpose of a sum of matrices is equal to the sum of their transposes, and the transpose of a product of matrices is equal to the product of their transposes in reverse order. Applying these properties, we get: A' = X'X - (X'Z(ZZ)^(-1)Z'X)'. The transpose of a transpose is equal to the original matrix, so: A' = X'X - X'Z(ZZ)^(-1)Z'X. Comparing this with the original matrix A, we can see that A' = A, which confirms that A is symmetric. Positive eigenvalues: To show that all eigenvalues of A are positive, we need to demonstrate that for any non-zero vector v, v'Av > 0, where v' represents the transpose of v. Considering the expression v'Av: v'Av = v'[XX - X'Z(ZZ)^(-1)Z'X]v
Expanding the expression using matrix multiplication : v'Av = v'X'Xv - v'X'Z(ZZ)^(-1)Z'Xv. Since X and Z have full column rank, X'X and ZZ' are positive definite matrices. Additionally, (ZZ)^(-1) is also positive definite. Thus, we can conclude that the second term in the expression, v'X'Z(ZZ)^(-1)Z'Xv, is positive definite.Therefore, v'Av = v'X'Xv - v'X'Z(ZZ)^(-1)Z'Xv > 0 for any non-zero vector v. Implications in econometrics: In econometrics, positive definiteness of a matrix has important implications. In particular, the positive definiteness of the matrix [XX - X'Z(ZZ)^(-1)Z'X] guarantees that it is invertible and plays a crucial role in statistical inference.
When conducting econometric analysis, this positive definiteness implies that the estimator associated with X and Z is consistent, efficient, and unbiased. It ensures that the estimated coefficients and their standard errors are well-defined and meaningful in econometric models. Furthermore, positive definiteness of the matrix helps in verifying the assumptions of econometric models, such as the assumption of non-multicollinearity among the regressors. It also ensures that the estimators are stable and robust to perturbations in the data. Overall, the positive definiteness of the matrix [XX - X'Z(ZZ)^(-1)Z'X] provides theoretical and practical foundations for reliable and valid statistical inference in econometrics.
To learn more about eigenvalues click here: brainly.com/question/29861415
#SPJ11
Help! please and thank you!!

Answers
Answer:
y = (x - 4)(x + 2)
Step-by-step explanation:
Quadratic Formula:
ax² + bx + c = 0Substitute 1 for a, -2 for b, and -8 - y for c in the quadratic formula.
Answer:
\(y= (x-4)(x+2)\)
Step-by-step explanation:
To factor polynomials you have to use FOIL. Which stands for First, Outer, Inner, and Last. This means that you have to multiply the first numbers of each part by each other, the outer two between both parts, the inner two between both parts, and the last two of each part, respectively. Then, you would simplify. If you go and do this for each answer choice, the last one would be the only correct one.
can some one please help me im confused on this im really stuck :(

Answers
Because moving 5 units up from -9 , you will end up at -4 ( 4 units below sea level)
Is the line y = 0.6x - 1

Answers
Answer:yes!
Step-by-step explanation:
Answer:
Yes
Step-by-step explanation:
To prove check out this screenshot Mark my answer the brainliest to tell you the 4 math calculators :)

HELP PLEASE 5MIN LEFT
a 84
b 630
c 426
d 28
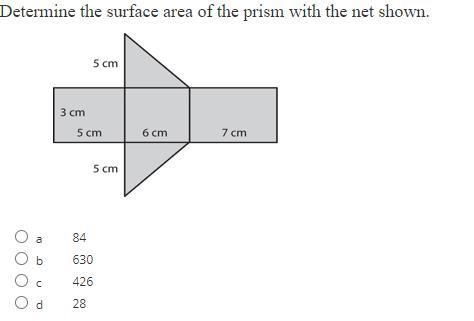
Answers
Answer:
a 84, I may be wrong, sorry if I am.
For Items 12–14, use the figure shown.
Two vertical parallel lines are intersected by two diagonal transversals, one slanting up and one slanting down. They intersect the left line at the same point, creating 6 angles, starting at the bottom left of the right line and moving clockwise, they are: 3, 2, 1, left parenthesis 4 y times 3 right parenthesis, left parenthesis 3 x times 9 right parenthesis, and left parenthesis 5 x right parenthesis. The line slanting up intersects the right vertical line creating 4 angles, starting at the bottom left and moving clockwise, they are: 6, 4, 5, and left parenthesis 9 x times 3 right parenthesis. The line slanting down intersects the right vertical line creating 4 angles, starting at the bottom left and moving clockwise, they are: 8, 7, left parenthesis 7 y times 6 right parenthesis, and 9.
What is m∠2?
A. 12
B. 18
C. 45
D. 120
Answers
Coplanar lines are referred to as parallel lines because they may be extended indefinitely without ever intersecting. || is the sign meaning "parallel to." If two lines are drawn, they don't have to be parallel, and a third line runs across them as seen in the diagram below. We may obtain eight distinct angles by drawing two parallel lines, and then drawing a transverse line through them.
Four pairs of matching angles will be created from all eight angles. One of the pairings is represented by angles F and B in the preceding diagram. If the two lines are parallel, corresponding angles are congruent.
To know more about lines refer to https://brainly.com/question/18831322
#SPJ1
I NEED HELP ASAP PLS HURRY


Answers
Answer: C
Step-by-step explanation: The slope of Function A is 6 and the slope of Function B is 5.
How do I solve this I get so confused on fractions if you can solve can I have an explanation

Answers
(10.05 LC)
If sin(x) =½
what is cos(x) and tan(x)? Explain your steps in complete sentences. (10 points)
Answers
Answer:
Cos x = \(\frac{base}{hypotenuse}=\frac{\sqrt{3} }{2}\)
Tan x = \(\frac{perpendicular}{base} = \frac{1}{\sqrt{3} }\)
Step-by-step explanation:
Sin x = \(\frac{perpendicular}{hypotenuse} = \frac{1}{2}\)
So, Perp = 1 and hyp = 2
Putting this in Pythagorean theorem to get the base
\(c^2 = a^2+b^2\)
\(a^2 = c^2-b^2\)
base ² = 4-1
base² = 3
Taking square root on both sides
Base = \(\sqrt{3}\)
Now
Cos x = \(\frac{base}{hypotenuse}=\frac{\sqrt{3} }{2}\)
Tan x = \(\frac{perpendicular}{base} = \frac{1}{\sqrt{3} }\)
A square based pyramid has a surface area of 111.6 in2. The sides of the base
of the pyramid measure 4 inches. What is the slant height of the figure?
Answers
Answer:
11.95 in
Step-by-step explanation:
We can find the slant height of a square-based pyramid by solving the area formula for the unknown value.
__
The formula for the area of a square-based pyramid can be written ...
A = s(s +2h)
where s is the side of the square base, and h is the slant height.
Filling in the given values, we can solve for h:
111.6 = 4(4 +2h)
27.9 = 4 +2h . . . . . divide by 4
13.95 = 2 +h . . . . . divide by 2
11.95 = h . . . . . . . . subtract 2
The slant height of the figure is 11.95 inches.
Zero Property: (-10 + 10) divided by 17 =
Answers
Zero divided by any real number is equal to zero.
\((-10+10)/17=\)\(0/17=0\)Find the solution to the linear system of differential equations {x′= 22x + 60y, y′= -6x - 16y
satisfying the initial conditions satisfying the initial conditions x(0)=5 and y(0)=3:
Answers
The solution to the system of differential equations that satisfies the initial conditions x(0)=5 and y(0)=3 is:
x(t) = (29/3) \(e^{\frac{22t}{3} }\) - (4/3) \(e^{\frac{-16t}{3} }\)
y(t) = (-13/3) \(e^{\frac{22t}{3} }\) + (2/3) \(e^{\frac{-16t}{3} }\)
To solve the system of differential equations, we can use matrix exponential. The system can be written in matrix form as follows:
X' = AX, where X = [x y], A = [22 60; -6 -16]
The matrix exponential of A can be calculated as follows:
\(e^{(At)}\) = I + At + \(\frac{(At)^{2}}{2!}\) + \(\frac{(At)^{3}}{3!}\) + ...
where I is the identity matrix and t is the variable of integration.
We can substitute A and t = 1 into the formula to get:
\(e^{A}\)= I + A + \(\frac{(A)^{2}}{2!}\) + \(\frac{(A)^{3}}{3!}\)+ ...
= [1 0; 0 1] + [22 60; -6 -16] + [44 192; -36 -104]/2! + [ -256 -768; 96 272]/3! + ...
= [1 + 22 + \(\frac{44}{2!}\) - \(\frac{256}{3!}\)*60 + \(\frac{192}{2!}\) - \(\frac{768}{3!}\);
-6 + \(\frac{(-6)}{2!}\) + \(\frac{96}{3!}\) - 16 + \(\frac{(-104)}{2!}\)+ \(\frac{272}{3!}\)]
= [\(\frac{29}{3}\) \(\frac{102}{3}\);
\(\frac{-13}{3}\) \(\frac{-4}{3}\) ]
Now we can use the initial conditions to find the constants of integration. We have:
X(0) = [x(0) y(0)] = [5 3]
So,
[\(e^{A}\)] [\(c_{1}\)] = [5]
[\(c_{2}\)] [3]
Multiplying both sides by the inverse of \(e^{A}\), we get:
[\(c_{1}\)] = [29/3 102/3]^(-1) [5]
[\(c_{2}\)] [-13/3 -4/3] [3]
Solving this system of linear equations, we get:
\(c_{1}\) = -4/3
\(c_{2}\) = 2/3
Therefore, the solution to the system of differential equations that satisfies the initial conditions x(0)=5 and y(0)=3 is:
x(t) = (29/3) \(e^{\frac{22t}{3} }\) - (4/3) \(e^{\frac{-16t}{3} }\)
y(t) = (-13/3) \(e^{\frac{22t}{3} }\) + (2/3) \(e^{\frac{-16t}{3} }\)
To learn more about differential equations:
https://brainly.com/question/14620493
#SPJ4
Write 28 1/4 as a decimal.
Answers
Answer:
28.25
Step-by-step explanation:
10. Mrs. Hammonds made a deposit of $100 into her checking
account. Then she wrote checks for $97.54 and $48.27. If her
previous balance was $320.39, what is her new balance?
Answers
Previously available balance = $320.39
Deposit made by Mrs. Hammonds = $100
Cheques used for withdrawal,
$97.54
$48.27
The new balance = $320.39 + $100 - $97.54 - $48.27
= 420.39 - 145.81
= 274.58
So, the new balance in her account is $274.58.
What is Withdrawal?According to accounting terminology, a company withdrawal is the removal of funds, but not to compensate the owner as in an owner's draw. A withdrawal can be made for many different purposes, such as paying an employee back for a company expense or covering a cash-intensive business expense like entertaining a significant client at a sporting event. A withdrawal can also refer to the departure of money from an employer-sponsored retirement plan, like a 401k. Additionally, some businesses have a petty cash fund set up for various modest charges; certain incidental expenses may also be paid out of this account. An owner's draw or accounting drawing is not taxed in the traditional sense.To learn more about Withdrawal, refer to:
https://brainly.com/question/14289883
#SPJ13
PLEASE HELP NOW --- >N is a 2-digit even number. If the last two digits of N^2 is the same as N, what is the sum of digits of N?
Answers
Answer:
76.
Step-by-step explanation:
It is given that N is a 2-digit number.
Last two digits of N^2 is the same as N.
We know that, a number is even if it ends with 0,2,4,6,8.
\(2^2=4,4^2=16,6^2=36,8^2=64\)
If 0 is in end then we get two zeros in the square of that number.
It is clear that, number should ends with 6 to get the same number at the end.
\(16^2=256\)
\(26^2=676\)
\(36^2=1296\)
\(46^2=2116\)
\(56^2=3136\)
\(66^2=4356\)
\(76^2=5776\)
\(86^2=7396\)
\(96^2=9216\)
It is clear that last two digits of (76)^2 is the same as 76.
Therefore, the required number is 76.
7w − 12 = 23
what does w =
Answers
Answer:
w = 5
Step-by-step explanation:
Someone please explain to me how to do this ‼️

Answers
Answer:
37
Step-by-step explanation:
A triangle always have to finish with 180 degrees so
47+96=143
180-143=37
HOPE THIS HELPS :)