Tony is building a new silo to store corn as animal feed. It will be a cylinder
topped with a half-sphere, and must store 21 000 t of corn. The entire silo can be
filled with corn. Tony wants to minimize the surface area of the silo to reduce
materials and paint costs. He has the following information:
• 1 cubic m of corn has a mass of 700 kg.
• Building costs are $8/m2, taxes included.
• Paint comes in 3.8 L cans.
Each can covers 40 sq m and costs $35,
taxes included.
• Corn costs $140 per tonne ($140/t), taxes
included.
02
Recall that 1 ton = 1000kg
What is the total cost to build, paint, and fill a silo with the least surface area?
Answers
Answer:
its $2,400
Step-by-step explanation:
if this helps please mark brainliest
Related Questions
Please Help me With this 30 points and brainliest to first person

Answers
Answer:
(4 * (1 + 2 * 3))^5
Step-by-step explanation:
Try many possibilites and see which one is greater.
The '5' should be an exponent, as it will make the result very large.
Multiplication is usually an operation that makes numbers very big.
Hopefully!!!
The point (3,-1)( 3 , − 1 ) is a solution to this system of equations:
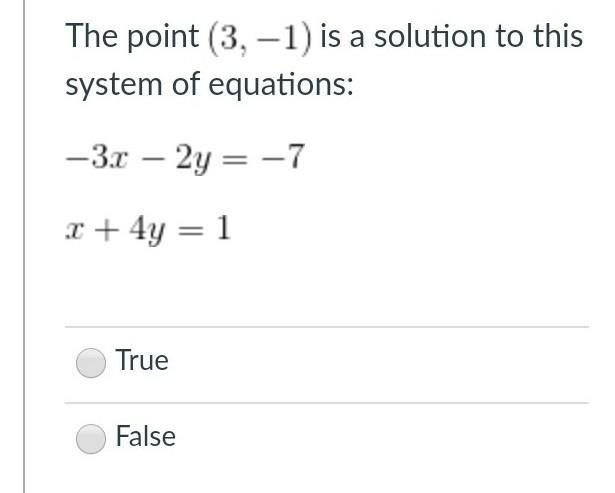
Answers
The system of equations we have is:
\(\begin{gathered} -3x-2y=-7 \\ x+4y=1 \end{gathered}\)And we need to check if the point (3,-1) is a solution.
Step 1. Identify the values of x and y from the point.
In a point, the first number represents the x-value, and the second number represents the y-value. So in point (3,-1) 3 is the x-value, and -1 is the y-value:
\(\begin{gathered} x=3 \\ y=-1 \end{gathered}\)Step 2. Substitute the x and y-value into the first equation and check that the result is equal to -7.
The first equation is:
\(-3x-2y=-7\)Substituting x=3 and y=-1:
\(-3(3)-2(-1)=\)Solve the operations:
\(-9+2=-7\)Since we get -7 as the result, (3,-1) is a solution for the first equation. But we still need to check if the point is a solution for the second equation.
Step 3. Substitute the x and y values into the second equation and check that the result is 1.
The second equation of the system is:
\(x+4y=1\)Substituting x=3 and y=-1:
\(3+4(-1)=\)Solving the operations:
\(3-4=-1\)The result we get is -1, not 1. Thus, since the point (3,-1) is not a solution for the second equation, it is also not a solution to the system of equation.
Answer: False
dwfegrhtytg pls helpp

Answers
Answer:
The answer to Y/X is 4/1
If a line has a slope of -4 and a y-intercept of 1, which of the following is its graph?

Answers
Answer: Yes its b
Step-by-step explanation:
Answer:
The answer is B I just finish doing that. Can I get a Brainlist please
Step-by-step explanation:
a 2.3-m-long string is under 26 n of tension. a pulse travels the length of the string in 54 ms .
Answers
In this scenario, we are given a string that is 2.3 meters long and under a tension of 26 N. Additionally, a pulse travels the length of the string in 54 ms.
When a pulse travels through a string, it causes the string to vibrate and move. The tension of the string determines how quickly the pulse can travel and how far it can go. In this case, the tension of 26 N is relatively high, which means that the pulse can travel quickly and over a significant distance.
The fact that the pulse travels the length of the string in 54 ms tells us something about the speed of the pulse. We can use the formula speed = distance / time to calculate the speed of the pulse. In this case, the distance is the length of the string, which is 2.3 m. The time is 54 ms, or 0.054 s.
So, speed = distance / time = 2.3 m / 0.054 s = 42.59 m/s.
We now know the speed of the pulse, but what about the tension and length of the string? We can use the formula v = sqrt(T/μ) to calculate the speed of a pulse in a string, where v is the speed of the pulse, T is the tension of the string, and μ is the mass per unit length of the string.
Rearranging this formula, we get T = μv^2. We can use this formula to find the tension of the string. Plugging in the values we know, we get:
T = μv^2 = (mass per unit length of string) * (speed of pulse)^2
We don't know the mass per unit length of the string, but we can find it using the formula μ = m / L, where m is the mass of the string and L is its length.
Assuming the string has a uniform density, we can calculate its mass using the formula m = ρAL, where ρ is the density of the string, A is its cross-sectional area, and L is its length.
We don't know the cross-sectional area, but we can make a rough estimate based on the thickness of the string. Assuming the string has a circular cross-section, we can use the formula A = πr^2, where r is the radius of the string.
Again, we don't know the radius of the string, but we can make a rough estimate based on its diameter. Assuming the string has a diameter of 2 mm, its radius is 1 mm, or 0.001 m.
Plugging in these values, we get:
A = π(0.001 m)^2 = 7.85 x 10^-7 m^2
m = ρAL = (density of string) * (cross-sectional area) * (length of string)
= (density of string) * (7.85 x 10^-7 m^2) * (2.3 m)
We don't know the density of the string, but assuming it is made of nylon or a similar material, its density is around 1100 kg/m^3. Plugging in this value, we get:
m = 2.039 x 10^-3 kg
μ = m / L = 2.039 x 10^-3 kg / 2.3 m = 8.86 x 10^-4 kg/m
Now we can use the formula T = μv^2 to find the tension of the string. Plugging in the values we know, we get:
T = μv^2 = (8.86 x 10^-4 kg/m) * (42.59 m/s)^2 = 159.3 N
So the tension of the string is 159.3 N, which is much higher than the original tension of 26 N. This makes sense, since the pulse travels quickly and over a significant distance, indicating that the tension must be high.
To know more about tension visit:
https://brainly.com/question/30794023
#SPJ11
PLEASE I NEED THIS
SO MANY POINTS

Answers
The speed of Sali is 18.75 km/hr.
Given,
Total distance travelled by Jane and Sali =63 km.
The total time is taken by Jane=3.5 hours.
Sali started to cycle 4 minutes after Jane started to cycle \(=\frac{4}{60}=\frac{1}{15}\) hours.
What is the formula to find speed?The formula to find speed is \(Speed=\frac{Distance}{Time} \ km/hr\).
The speed of Jane\(=\frac{63}{3.5}=18 \ km/hr\).
Let the speed of Sali be \(x \ km/hr\).
Sali caught up with Jane when they had both cycled 30 km.
Thus, \(\frac{30}{18}-\frac{30}{x}=\frac{1}{15}\)
\(\implies \ \frac{30x-540}{18x} =\frac{1}{15}\)
\(\implies \ 15(30x-540)=18x\)
\(\implies \ 5(30x-540)=6x\)
\(\implies \ 150x-2700=6x\)
\(\implies \ 144x=2700\implies \ x=18.75\)
Therefore, the speed of Sali is 18.75 km/hr.
To learn more about the speed visit:
https://brainly.com/question/7359669.
#SPJ1
Your company wants to purchase some equipment for recycling metals. Machine A costs $323,000 and has a useful life of 10 years. Its operating costs are $2.40 per ton of metal processed. Machine B costs $178,000 and has a useful life of 6 years. Its operating costs are $8.00 per ton of metal processed. How many tons of metal per year must your company process to favor Machine A over Machine B? Assume an MARR of 18% per year.
Previous question
Next question
Answers
To determine the number of tons of metal per year needed for Machine A to be favored over Machine B, we compare their costs. Machine A costs $323,000 with an annual operating cost of $2.40/ton, while Machine B costs $178,000 with an annual operating cost of $8.00/ton. The machines have useful lives of 10 years and 6 years, respectively, and the minimum attractive rate of return (MARR) is 18% per year.
By calculating the equivalent annual costs (EAC) for each machine using the given formula and comparing them, we can determine the point at which Machine A becomes more favorable. However, specific values for the discounting factors and the tons per year needed are missing, making it impossible to provide an exact answer.
Learn more about investment analysis here: brainly.com/question/32546870
#SPJ11
a person tosses a coin 15 times. in how many ways can he get 3 heads?
Answers
A person tosses a coin 15 times. In how many ways can he get 3 heads? The answer is 455 ways.
To find the answer, we can use the formula for combinations, which is:
C(n, k) = n! / (k! * (n-k)!)
Where n is the total number of tosses, and k is the number of heads we want to get.
In this case, n = 15 and k = 3, so we can plug these values into the formula:
C(15, 3) = 15! / (3! * (15-3)!)
C(15, 3) = 15! / (3! * 12!)
C(15, 3) = (15 * 14 * 13 * 12!)/ (3! * 12!)
C(15, 3) = 455
So there are 455 ways to get 3 heads when tossing a coin 15 times.
Learn more about probability theory and combinatorics here:https://brainly.com/question/29601156
#SPJ11
Write an expression that represents the temperature (in degrees Fahrenheit) of the water after Raul added the ice cubes. Write the expression as the sum of two numbers.
Answers
As a result of answering the given question, we may state that This equation is the product of two numbers: T0 and -x * y * C.
What is equation?In mathematics, an equation is a statement stating the equality or two expressions. An equation consists of two sides split by an algebraic equation (=). For illustration, the argument "2x + 3 = 9" argues that the statement "2x + 3" is equal to the value "9". The goal of equation solving is to find the value or values of the variable(s) to make the equation true. Simple or complex equations, regular or nonlinear, and including one or more factors are all possible. For example, the equation "x2 + 2x - 3 = 0" has the variable x raised to the second power. Lines are used in many fields of mathematics, including algebra, calculus, or geometry.
Provided the temperature of the water before adding the ice cubes is known and given by T0 (in degrees Fahrenheit), and Raul added a certain number of ice cubes to the water, we can represent the temperature of the water after adding the ice cubes as:
T = T₀ + ΔT
ΔT = -x * y * C
where C is a constant representing water's heat capacity.
Putting it all together, the temperature of the water after adding the ice cubes can be expressed as:
T = T₀ - x * y * C
This expression is the product of two numbers: T0 and -x * y * C.
To know more about equation visit:
https://brainly.com/question/649785
#SPJ1
Write an equation of the tangent line to the circle x2+y2=37 at (6,1)
Answers
The equation of the tangent line to the circle x^2 + y^2 = 37 at the point (6,1) is y = -6x + 37.
The equation of the tangent line to the circle x^2 + y^2 = 37 at the point (6,1) can be found by first finding the slope of the tangent line at that point and then using point-slope form to write the equation of the tangent line.
To find the slope of the tangent line at (6,1), we need to take the derivative of the circle equation with respect to x:
2x + 2y dy/dx = 0
Solving for dy/dx, we get:
dy/dx = -x/y
At the point (6,1), the slope of the tangent line is:
dy/dx = -(6)/(1) = -6
Now we can use point-slope form to write the equation of the tangent line:
y - y1 = m(x - x1)
where (x1,y1) is the point (6,1) and m is the slope we just found.
Substituting the values, we get:
y - 1 = -6(x - 6)
Simplifying the equation, we get:
y = -6x + 37
To learn more about equation click on,
https://brainly.com/question/1539917
#SPJ4
Given â³ABC with a 2+ b 2= c 2 , write a two-column proof of the Converse of the Pythagorean Theorem
Answers
The converse of the Pythagorean Theorem is that when the sum of the squares of the other two sides square of the length of the longest side of a triangle, then the triangle is a right triangle.
To understand the Pythagorean Theorem let's assume 2 right-angled triangles with the 2 legs = a and b in each case and the longest side = c in one triangle and e in the other.
Pythagoras' Theorem is = \(a^2 + b^2 = c^2\) (Given)
Also in the other triangle \(a^2 + b^2 = e^2\), if it is right-angled.
Therefore a^2 = l^2 and a = e.
So the 2 triangles are congruent by SSS.
So m < e in one triangle = m < e the angles opposite the hypotenuse
Therefore the second triangle is right-angled
The two triangles are said to be congruent by the SSS rule when all three sides of one triangle are equivalent to the corresponding three sides of the second triangle.
To learn more about the Pythagorean Theorem :
https://brainly.com/question/28981380
#SPj4

-xy - (-x + y) if x = -3 and y= -4
Answers
Answer:
-11
Step-by-step explanation:
--3*-4 - (--3-4)
-12 - (3-4)
-12 - (-1)
-12+1
-11
PLEASE HELP QUICK!!!!
Bernie borrowed b books from his friend Grant's collection of 29 books. What does the expression 29 - b represent?
Answers
the amount of books grant has left
explanation:
if you take away b books, he is left with 29-b books
What is the solution of the inequality shown
below?
y+7≤-1
Answers
The solution to the inequality is y ≤ -8. This means that any value of y that is less than or equal to -8 will satisfy the original inequality.
To solve the inequality y + 7 ≤ -1, we need to isolate the variable y on one side of the inequality sign.
Starting with the given inequality:
y + 7 ≤ -1
We can begin by subtracting 7 from both sides of the inequality:
y + 7 - 7 ≤ -1 - 7
y ≤ -8
The solution to the inequality is y ≤ -8. This means that any value of y that is less than or equal to -8 will satisfy the original inequality.
In the context of a number line, all values to the left of -8, including -8 itself, will make the inequality true. For example, -10, -9, -8, -8.5, and any other value less than -8 will satisfy the inequality. However, any value greater than -8 will not satisfy the inequality.
For such more question on solution:
https://brainly.com/question/24644930
#SPJ8
The following question may be like this:
What is a solution of the inequality shown below? y+7≤-1
many psychologists consider the distinguishing feature of adolescent thought to be ability to think in terms of ___.
Answers
Many psychologists consider the distinguishing feature of adolescent thought to be the ability to think in terms of abstract concepts or hypothetical situations.
During adolescence, individuals begin to develop more advanced cognitive abilities, including the capacity for abstract reasoning and hypothetical thinking.Abstract thinking involves understanding and manipulating ideas and concepts that are not directly tied to concrete objects or experiences. It allows adolescents to consider possibilities beyond immediate reality and to engage in complex reasoning, such as formulating and testing hypotheses or contemplating multiple perspectives.Hypothetical thinking, on the other hand, enables adolescents to mentally explore potential scenarios and outcomes. They can consider "what if" situations, contemplate alternative solutions to problems, and weigh the consequences of different choices.These cognitive abilities play a crucial role in adolescent development, facilitating problem-solving, decision-making, and the exploration of identity and future possibilities.
For more such questions on Abstract thinking
https://brainly.com/question/27410184
#SPJ4
The circumference of a circle is 177 ft. Find its diameter, in feet. Answer: d = I ft Submit Answer
Answers
Remember that the formula for the circumference of a circle is:
\(C=\pi D\)Solving for D (diameter), we get:
\(C=\pi D\rightarrow D=\frac{C}{\pi}\)Using the data given, we'll get that:
\(D=\frac{177ft}{\pi}\Rightarrow D=56.34ft\)Therefore, the diameter of the cirle is 56.34 feet
write the quadratic equation I(x)=50x-x^2 into a linear factor form
Answers
The quadratic equation I(x)=50x-x² into a linear factor form is x - 25.
What does a quadratic equation's linear factor mean?The formula for linear factors is ax + b, an x + b. A polynomial cannot be factorised further over the real numbers, and there are no actual zeros or x values that would make an irreducible quadratic factor equal to 0. A linear factor model establishes a linear equation-based link between the return on an asset (such as a stock, bond, mutual fund, or other type of investment) and the values of a small number of variables.
50x- x²
=x(50-x)
= product of 2 linear factors
zeros are 0 and 50 = x intercepts or roots
You could rewrite it in vertex form
-(x² - 50x + 25²) + 25²
= -(x-25)² + 625
with vertex = (25,625) = max point of a downward opening parabola
= -(x-25)(x-25) + 624
where (x-25)² is the product or square of 2 linear factors x - 25 and x-25
Learn more about the Quadratic Equation here: https://brainly.com/question/1214333
#SPJ1
Ava is trying to add together 3/5 and 2/9
Her first step is to rewrite the fractions using their lowest common denominator.
What denominator should she use?
Answers
Explanation:
The LCM of 5 and 9 is 5*9 = 45
This is the LCD of the two fractions.
3/5 = 27/45 after multiplying top and bottom by 92/9 = 10/45 after multiplying top and bottom by 5So,
3/5 + 2/9 = 27/45 + 10/45 = 37/45
ill mark brainliswt plss help

Answers
Answer:
z = 24
Step-by-step explanation:
35/30 = 7/6
28/7 = 4
6 x 4 = 24
28/24 = 7/6
Answer:
x = 24
Step-by-step explanation:
All you have to do is cross multiply like this..
30 x 28 = 35 x X
=
x = 30 x 28/35
30 x 28 = 840 / 35 =
24
So x is equal to 24
Hope this helps :)
give an example of a function that is differentiable on r with exactly two roots but its derivative has 5 roots. the example can be a graph of a function.
Answers
One possible example is the function \(f(x) = (x+1)(x-2)^{2}(x-3)^{2}\), which has roots at x = -1 and x = 2, but whose derivative \(f'(x) = 2(x-2)(x-3)(3x^2-10x+7)\) has roots at x = 1, \(x = 2 - \sqrt(2)\),\(x = 2 + \sqrt(2)\), x = 5/3, and x = 7/3.
To construct such an example, we need a function whose roots are simple and occur at two distinct points, and whose derivative has multiple roots. One way to do this is to take a function that has multiple roots of high multiplicity, and then perturb it so that some of those roots split into simple roots.
In the example given, we start with the function \(f(x) = (x+1)(x-2)^4(x-3)^4\), which has roots at x = -1, x = 2, and x = 3, each with multiplicity 4. We then perturb the function slightly by replacing one of the factors \((x-2)^4\) with \((x-2)^2\), so that the root at x = 2 splits into two simple roots. This gives us the function \(f(x) = (x+1)(x-2)^2(x-3)^4\), which has roots at x = -1 and x = 2, but whose derivative \(f'(x) = 2(x-2)(x-3)^3(3x-10)\) has five roots, as stated above.
Learn more about derivative here:
https://brainly.com/question/29144258
#SPJ11
An equilateral triangle has a perimeter of
216 inches. What is the length of one side of the
triangle?
Answers
All 3 sides are the same length
Answer:
72
Step-by-step explanation:
hard to explain
If you walk 9 miles due north, then turn around and walk 5 miles due south, how much total distance have you traveled and what is your net displacement from your starting point respectively?
Answers
The total distance traveled is 14 miles, and the net displacement from the starting point is 4 miles south.
To determine the total distance traveled, we add the distances traveled in each direction. In this case, we walk 9 miles due north and then 5 miles due south. Therefore, the total distance traveled is 9 miles + 5 miles = 14 miles.
Net displacement refers to the change in position or the straight-line distance from the starting point to the final position. Since we walked 9 miles north and then 5 miles south, the net displacement is determined by subtracting the distance traveled in the opposite direction. Thus, the net displacement is 9 miles - 5 miles = 4 miles south.
It's important to note that while the total distance traveled is 14 miles, the net displacement is only 4 miles south. This indicates that even though we covered a total distance of 14 miles, our final position is 4 miles south of the starting point.
Learn more about Displacement
brainly.com/question/11934397
brainly.com/question/29769926
#SPJ11
The circle below is made up of nine parts that are all the same size, Vincent marks the angle in one part of the circle as shown. Complete the statements to show the connection between the fraction of Vincent's circle marked by the angle and the angle measure. The measure of the angle turns through 2 of the circle. The angle measure can be found using the expression Х

Answers
Since the circle is divided into 9 equal parts, this will serve as our denominator. Each slice is 1/9 of the circle.
Since Vincent marked two parts of the circle, that measures 2/9 of the circle.
We know that one whole circle is one revolution and that is 360 degrees.
Hence, to measure the marked angle, we can use the expression:
\(\frac{2}{9}\times360\)Terry’s age is 3 less than three times Cody’s age. The sum of their ages is 57. How
old are Terry and Cody?
Answers
Answer:
let Cedric's age be c
tyshon= 3c-3
3c-3 +c =3c+c-3 = 4c-3
4c-3=57
4c=60
c=15
tyshon=15×3-3 = 42
Answer:
16
Step-by-step explanation:
57dvied by 3 -3=16
FOR BRAIBLIEST AND 50 POINTS! I NEED THIS ASAP!
Directions: Graph these in one cartesian plane(clue: it would form a triangle)

Answers
The solution is the area between the three lines, a triangle with vertices marked (see attached).

Simplify 12 − 32 + (5 − 1)2.
A) 12
B) 18
C) 19
D) 97
Answers
12-32+[5-1]x2
-20+4x2
-20+8
=-12
The simplified form of the given expression is -12. Therefore, option A is the correct answer.
What is simplify?Simplify simply means to make it simple. In mathematics, simply or simplification is reducing the expression/fraction/problem in a simpler form. It makes the problem easy with calculations and solving.
The given expression is 12-32+(5-1)2.
Here, 12-32+4×2
= -20+8
= -12
Therefore, option A is the correct answer.
Learn more about the simplification here:
brainly.com/question/2804192.
#SPJ6
1. ADEA has vertices D(8,4), E(2, 6), and F(3, 1). What are the vertices of the image after a dilation with a scale factor of 5 using the origin as the center of dilation? TO
Answers
Answer:
The vertices of the image are D' (40, 20), E' (10, 30), F' (15, 5)
Step-by-step explanation:
If the point (x, y) dilated by a scale factor k using the origin as a center of dilation, then its image is (kx, ky)
Let us use this rule to solve our question
∵ The vertices of ΔDEF are D (8, 4), E (2, 6), and F (3, 1)
∵ ΔDEF dilated by a scale factor 5
∴ k = 5
∵ The origin as the center of dilation
→ That means, multiply the coordinates of every point by 5
∴ D' = (8 × 5, 4 × 5)
∴ D' = (40, 20)
∴ E' = (2 × 5, 6 × 5)
∴ E' = (10, 30)
∴ F' = (3 × 5, 1 × 5)
∴ D' = (15, 5)
∴ The vertices of the image are D' (40, 20), E' (10, 30), F' (15, 5)
Use the unit circle to find the inverse function value in degrees.
sin–1Question 3
A. 60°
B. 240°
C. 30°
D. 300°
SOMEONE PLEASE HELP!!!
Answers
The inverse function value in degrees will be 45°. Option C is correct.
What is a function's inverse?Assuming function 'f' is a one-to-one and onto function which is a need for inverses to exist. The inverse of the considered function is. f⁻¹(x).
The given function is;
f(x)=sin⁻¹(1/2)
The given function is arranged is;
x=sin⁻¹(1/2)
The given function in terms of sin;
sinx=1/2
Putting the values in terms of sin we get;
sinx=sin30°
x=30°
The inverse function value in degrees will be 45°.
Hence,option C is correct.
To learn more about inverse function here:
https://brainly.com/question/19425567
#SPJ1
Can someone help me with this?

Answers
Using multiplication or division, we can have either of the numbers from the operation.
Multiplication and Division of Numbersmultiplication is the repeated addition of numbers. But the rules for multiplication of integers are different from that of addition. It includes three possibilities. They are:
Multiplication between two positive numbers,Multiplication between two negative numbers; andMultiplication between a positive number and a negative number.The product of two integers with similar sign numbers will always be positive. This means the product of two positive numbers or two negative numbers will always be positive. While the product of a positive number and a negative number (integers with different signs) will always be negative.
In this case, the three numbers are interconnected via arithmetic operation.
135 ÷ 15 = 9
9 × 15 = 135
135 ÷ 9 = 15
So, which ever way we go with, these numbers are connected to each other.
Learn more on multiplication and division here;
https://brainly.com/question/4158962
#SPJ1
Answer The questions Given Below

Answers
(i) The value of angle x, ∠x, is 70°
(ii) ΔABC and ΔADC are congruent by the side-angle-side (SAS) criterion
(iii) The value of angle m, ∠m, is 20°
Calculating the measure of an angles and Congruent trianglesFrom the question, we are to determine the value of angle x.
From the given diagram, we can write that
m ∠x + 110° = 180° (Linear pair theorem)
Thus,
m ∠x = 180° - 110°
m ∠x = 70°
(ii) ΔABC and ΔADC are congruent by the side-angle-side (SAS) criterion
(iii) From the given information, the park was converted into a rectangular form
Thus,
m ∠P = 90°
From the diagram, we can write that
m ∠m + m ∠x + m ∠P = 180° (Sum of angles in a triangle)
Then,
m ∠m + 70° + 90° = 180°
m ∠m = 180° - 90° - 70°
m ∠m = 20°
Hence, the value of angle m is 20°
Learn more on Calculating the measure of angles here: https://brainly.com/question/22535376
#SPJ1