Expresa en segundos (")
40 54' =
230 56' 35" =
460 27' 38" =
Answers
230º 56’ 35” = 831395 segundos
no se la ultima
Related Questions
Which of these represents the expression "7 less than twice x"? O 7-2x O 2X-7 O 7 < 7 < 2x O 2x
Answers
Answer:
the answer for this question is 7-2x
Evaluate the expression for the given value of the variable(s).
-2x² + 8x – 4; x = -3
Answers
please help me i will give brainliest

Answers
Answer:
i would help you bet i cant read that. Maybe put the paper in better lighting?
Please help me out! I can’t do this on my own.

Answers
Answer:
y = 6x
Step-by-step explanation:
y = mx + b
Slope (m) = 6/1 up 6, right 1
Y-intercept (b) = 0
What is the name of the green segment in the hyperbola below
Answers
The Length of the conjugate axis is equal to 2b. The transverse axis is an essential feature of a hyperbola, as it determines the overall shape of the hyperbola.
In a hyperbola, the name of the green segment is called the transverse axis. The transverse axis is the longest distance between any two points on the hyperbola, and it passes through the center of the hyperbola. It divides the hyperbola into two separate parts called branches.
The transverse axis of a hyperbola lies along the major axis, which is perpendicular to the minor axis. Therefore, it is also sometimes called the major axis.
The other axis of a hyperbola is called the conjugate axis or minor axis. It is perpendicular to the transverse axis and passes through the center of the hyperbola. The length of the conjugate axis is usually shorter than the transverse axis.In the hyperbola above, the green segment is the transverse axis, and it is represented by the letters "2a". Therefore, the length of the transverse axis is equal to 2a.
The blue segment is the conjugate axis, and it is represented by the letters "2b".
Therefore, the length of the conjugate axis is equal to 2b.The transverse axis is an essential feature of a hyperbola, as it determines the overall shape of the hyperbola. In particular, the distance between the two branches of the hyperbola is determined by the length of the transverse axis.
If the transverse axis is longer, then the branches of the hyperbola will be further apart, and the hyperbola will look more stretched out. Conversely, if the transverse axis is shorter, then the branches of the hyperbola will be closer together, and the hyperbola will look more compressed.
For more questions on Length .
https://brainly.com/question/28322552
#SPJ8
Using Laplace Transforms, find the solution of the initial value problem: d²y +9y =9. sin(t). U(t - 3), = y(0) = y'(0) = 0 dx²
Answers
The solution to the given initial value problem, obtained using Laplace transforms, is y(x) = 0. This means that the function y(x) is identically zero for all values of x.
To find the solution of the initial value problem using Laplace transforms for the equation d²y/dx² + 9y = 9sin(t)u(t - 3), where y(0) = y'(0) = 0, we can follow these steps:
Take the Laplace transform of the given differential equation.
Applying the Laplace transform to the equation d²y/dx² + 9y = 9sin(t)u(t - 3), we get:
s²Y(s) - sy(0) - y'(0) + 9Y(s) = 9 * (1/s² + 1/(s² + 1))
Since y(0) = 0 and y'(0) = 0, the Laplace transform simplifies to:
s²Y(s) + 9Y(s) = 9 * (1/s² + 1/(s² + 1))
Solve for Y(s).
Combining like terms, we have:
Y(s) * (s² + 9) = 9 * (1/s² + 1/(s² + 1))
Multiply through by (s² + 1)(s² + 9) to get rid of the denominators:
Y(s) * (s⁴ + 10s² + 9) = 9 * (s² + 1)
Simplifying further, we have:
Y(s) * (s⁴ + 10s² + 9) = 9s² + 9
Divide both sides by (s⁴ + 10s² + 9) to solve for Y(s):
Y(s) = (9s² + 9)/(s⁴ + 10s² + 9)
Partial fraction decomposition.
To proceed, we need to decompose the right side of the equation using partial fraction decomposition:
Y(s) = (9s² + 9)/(s⁴ + 10s² + 9) = A/(s² + 1) + B/(s² + 9)
Multiplying through by (s⁴ + 10s² + 9), we have:
9s² + 9 = A(s² + 9) + B(s² + 1)
Equating the coefficients of like powers of s, we get:
9 = 9A + B
0 = A + B
Solving these equations, we find:
A = 0
B = 0
Therefore, the decomposition becomes:
Y(s) = 0/(s² + 1) + 0/(s² + 9)
Inverse Laplace transform.
Taking the inverse Laplace transform of the decomposed terms, we find:
L^(-1){Y(s)} = L^(-1){0/(s² + 1)} + L^(-1){0/(s² + 9)}
The inverse Laplace transform of 0/(s² + 1) is 0.
The inverse Laplace transform of 0/(s² + 9) is 0.
Combining these terms, we have:
Y(x) = 0 + 0
Therefore, the solution to the initial value problem is:
y(x) = 0
To learn more about Laplace transforms visit : https://brainly.com/question/29583725
#SPJ11
Show me work!!!!!!!!!!!

Answers
Answer:
(2, 4)
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightAlgebra I
Midpoint Formula: \(\displaystyle (\frac{x_1+x_2}{2},\frac{y_1+y_2}{2})\)Step-by-step explanation:
Step 1: Define
Endpoint A(1, 2)
Endpoint B(3, 6)
Step 2: Find Midpoint
Simply plug in your coordinates into the midpoint formula to find midpoint
Substitute in points [MF]: \(\displaystyle (\frac{1+3}{2},\frac{2+6}{2})\)[Fraction] Add: \(\displaystyle (\frac{4}{2},\frac{8}{2})\)[Fraction] Divide: \(\displaystyle (2,4)\)Please help I need to do this

Answers
Answer:
4:
b) f(-1) = 0.50; f(-2) = 0.25; f(-3) = 0.125
c) Graph is attached -but you will need to do this by hand on the blank graph on the assignment.
5:
a) Increasing, y-intercept at (0, 125)
b) Decreasing, y-intercept at (0, 22)
c) Increasing, y-intercept at (0, 256)

Rapid Rental Car charges a $55.00 rental fee plus $0.25 per mile driven. Capital Cars charges a $45.00 rental fee and $0.35 per mile driven.
A. Write an equation that can be used to find the number of miles for which the rental cost at both companies would be the same.
Answers
Answer:
100 miles
Step-by-step explanation:
Given data
let the number of miles driven be x
and the total charge for x miles be y
Hence the expression for the total charge is
Rapid Rental Car
charges a $55.00 rental fee plus $0.25 per mile driven.
y= 55+0.25x--------------1
Capital Cars charges a $45.00 rental fee and $0.35 per mile driven.
y= 45+0.35x--------------2
Equate 1 and 2
55+0.25x= 45+0.35x
collect like terms
55-45=0.35x-0.25x
10=0.1x
x= 10/0.1
x= 100 miles
Hence the mile is 100 miles
Fill in the blank
The equation *blank* describes the relationship
(simplify your answer)
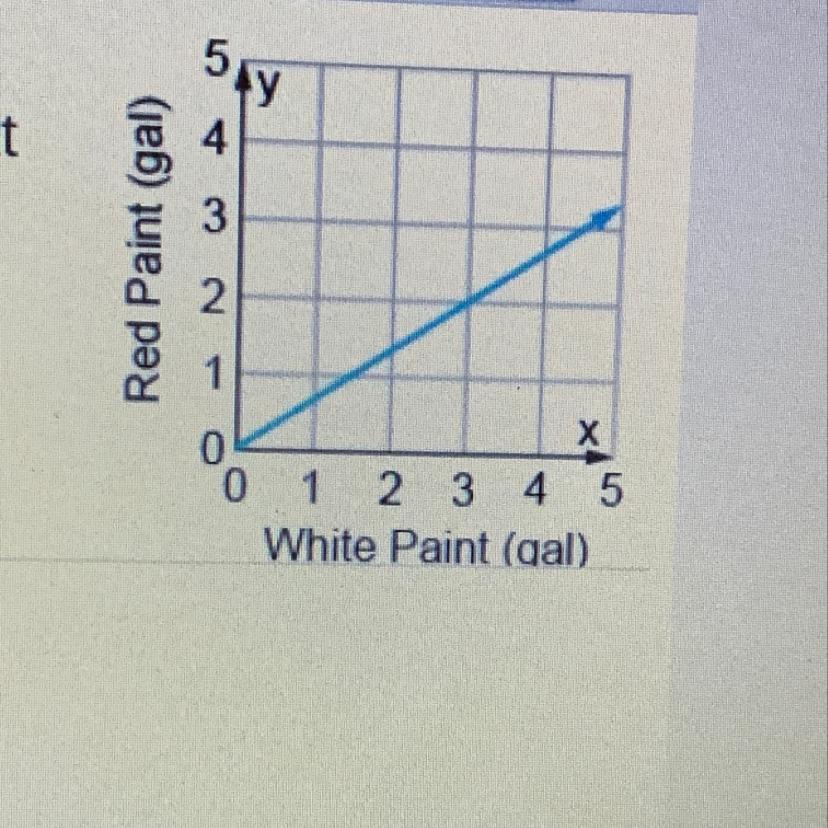
Answers
Answer:
y = 1.5x
Step-by-step explanation:
in the graph you can see that when y=2, x= 3 and this graph is linares (im sorry if its not the right word). so this means for every 3gal of white paint youll need 2 gal of red paint. so 2y=3x: y = 3/2x
Two samples, each with n = 6 subjects, produce a pooled variance of 20. Based on this information, the estimated standard error for the sample mean difference would be _____. Explain your response. a-20/6 b-20/12 c-the square root of (20/6 + 20/6) d-the square root of (20/5 + 20/5)
Answers
The estimated standard error for the sample mean difference is the square root of 6.6667. The closest option provided is: c- the square root of (20/6 + 20/6)
The estimated standard error for the sample mean difference can be calculated using the formula:
Standard Error = sqrt[(s1^2/n1) + (s2^2/n2)]
Where:
s1^2: Variance of the first sample
n1: Sample size of the first sample
s2^2: Variance of the second sample
n2: Sample size of the second sample
In this case, both samples have the same sample size (n = 6) and the same pooled variance of 20. Therefore, the formula simplifies to:
Standard Error = sqrt[(20/6) + (20/6)]
Simplifying further, we get:
Standard Error = sqrt[(40/6)]
To find the exact value, we can simplify the expression further:
Standard Error = sqrt[6.6667]
Therefore, the estimated standard error for the sample mean difference is the square root of 6.6667.
The closest option provided is:
c- the square root of (20/6 + 20/6)
So, the correct answer is (c).
Visit here to learn more about standard error brainly.com/question/13179711
#SPJ11
EASY SCIENCE ILL GIVE BRIANLIST PLEASE HELP
pleas give thre right answer
calculate the velocity of a doll with a mass of 50 kg and with a momentum of 2 kg m\s
Answers
Answer:
4.0 kg and a velocity of -25m/s.
jeana´s calculator display shows the number to the right.
a. express this number in scientific notation.
number to the right is 3.76EE13
Answers
Answer:
3.76EE13 is 3.76 * 10^13
Step-by-step explanation:
Here, we are to express what is written on the calculator in scientific notation.
Mathematically, that would be
3.76 * 10^13
The terms EE13 represent 10^13
Aslam's age is half of his father's age but 15 years ago his age was just
the father's age. Find his present age now.
Answers
Answer:
not possible or not determined is answer
Step-by-step explanation:
because let his father be 33
ad his son be 17
15 years ago
son will be 2
and father will be 18
not possible father's age ≠son's age in any respect unless and until father's age is decreased
Marcie jogs each morning. The relationship between the total number of miles she jogged this week, m, to the total number of miles she jogged last week, t, is represented by the equation m = 2t . Which description best represents this situation? Marcie jogged 2 less miles last week than she jogged this week. Marcie jogged 2 more miles last week than she jogged this week. Marcie jogged three times as many miles last week as she jogged this week. Marcie jogged twice as many miles this week as she jogged this week.
Answers
Answer: Marcie jogged twice as many miles this week as she jogged last week
Step-by-step explanation:
When examining group difference where the direction of the difference is specified, which of the following is used? Select one: a. two-tailed test b. one-tailed test C. directional hypothesis o d. critical value
Answers
When examining group differences with a specified direction, a one-tailed test is used i.e., option b is correct.
In statistical hypothesis testing, researchers often have a specific direction in mind when comparing two groups.
For example, they may hypothesize that Group A performs better than Group B or that Group A has a higher mean than Group B. In such cases, a one-tailed test is appropriate.
A one-tailed test is designed to detect differences in a specific direction. It focuses on evaluating whether the observed data significantly deviates from the null hypothesis in the specified direction.
The null hypothesis assumes no difference or no relationship between the groups being compared.
In a one-tailed test, the critical region is defined on only one side of the distribution, corresponding to the specified direction of the difference.
The critical value, which determines whether the observed difference is statistically significant, is chosen based on the desired level of significance (e.g., alpha = 0.05).
On the other hand, a two-tailed test is used when the direction of the difference is not specified, and the researchers are interested in determining whether there is a significant difference between the groups in either direction.
In this case, the critical region is divided equally between the two tails of the distribution.
A directional hypothesis (option C) is a statement that specifies the expected direction of the difference, but it is not the statistical test itself. The critical value (option D) is the value used to determine the cutoff for rejecting or accepting the null hypothesis.
Therefore, when examining group differences with a specified direction, a one-tailed test is used to assess the statistical significance of the observed difference in that particular direction.
Learn more about one-tailed test here:
https://brainly.com/question/31327677
#SPJ11
(b) if it is really the situation that p=0.13p=0.13, how likely is it that the test procedure in part (a) will not reject the null hypothesis? round your answer to two decimal places (e.g. 98.76).
Answers
The data do not present enough evidence against null-hypothesis, because there is insufficient evidence to conclude that proportion of "crankshaft-bearings" exhibits excess surface-roughness exceeds 0.10.
To find whether data denotes strong evidence that proportion of crankshaft-bearings represents excess surface roughness exceeds 0.10, we need to perform a hypothesis test. We define null and alternative hypotheses based on information.
Null Hypothesis (H₀): The proportion of crankshaft bearings exhibiting excess surface roughness is equal to or less than 0.10.
Alternative Hypothesis (H₁): The proportion of crankshaft bearings exhibiting excess "surface-roughness" exceeds 0.10.
We use significance level (α) of 0.05, for hypothesis testing.
Next, we perform hypothesis test using given sample data. The data states that out of random sample of 85 automobile engine crankshaft bearings, 10 have surface finish roughness that exceeds specifications.
We calculate test statistic and compare it to critical value to determine if we have strong evidence against the null hypothesis.
The test-statistic we will use is the sample proportion, which is calculated as : p' = x/n
Where : p' = sample proportion (proportion of bearings with excess surface roughness)
x = number of bearings with excess surface roughness (10 in this case)
n = sample size (85 in this case)
So, p' = 10/85 ≈ 0.1176
To assess whether this proportion is significantly greater than 0.10, we perform one-sample proportion z-test. The test statistic formula is:
z = (p' - p₀) / √((p₀ × (1 - p₀)) / n),
Where : p₀ = hypothesized proportion under null hypothesis (0.10 in this case),
The test statistic is : z = (0.1176 - 0.10) /√((0.10 × (1 - 0.10)) / 85) = 0.518,
The critical-value, with a significance level of 0.05 is 1.645.
Since the test statistic (0.5180) is less than the critical value (1.645), we fail to reject the null hypothesis.
Therefore, There is insufficient evidence to conclude that proportion of crankshaft bearings exhibiting excess surface roughness exceeds 0.10.
Learn more about Hypotheses here
https://brainly.com/question/14178486
#SPJ4
The given question is incomplete, the complete question is
In a random sample of 85 automobile engine crankshaft bearing, 10 have a surface finish roughness that exceeds the specifications. Do these data present strong evidence that the proportion of crankshaft bearing exhibiting excess surface roughness exceeds 0.10? also state the appropriate Hypotheses.
Answer asap! WiIl give brainliest!!!
Worth 11 points!

Answers
Step-by-step explanation:
So 300% is the same as the fraction 300/100. Cancel the zeros and you find 3/1, which is just 3.
The purchased cost of a 5-m3 stainless steel tank in 1995 was $10,900. The 2-m-diameter tank is cylindrical with a flat top and bottom. If the entire outer surface of the tank is to be covered with 0.05-m-thickness of magnesia block, estimate the current total cost for the installed and insulated tank. The 1995 cost for the 0.05-m-thick magnesia block was $40 per square meter while the labor for installing the insulation was $95 per square meter.
Answers
The estimated current total cost for the installed and insulated tank is $12,065.73.
The first step is to calculate the surface area of the tank. The surface area of a cylinder is calculated as follows:
surface_area = 2 * pi * r * h + 2 * pi * r^2
where:
r is the radius of the cylinder
h is the height of the cylinder
In this case, the radius of the cylinder is 1 meter (half of the diameter) and the height of the cylinder is 1 meter. So the surface area of the tank is:
surface_area = 2 * pi * 1 * 1 + 2 * pi * 1^2 = 6.283185307179586
The insulation will add a thickness of 0.05 meters to the surface area of the tank, so the total surface area of the insulated tank is:
surface_area = 6.283185307179586 + 2 * pi * 1 * 0.05 = 6.806032934459293
The cost of the insulation is $40 per square meter and the cost of labor is $95 per square meter, so the total cost of the insulation and labor is:
cost = 6.806032934459293 * (40 + 95) = $1,165.73
The original cost of the tank was $10,900, so the total cost of the insulated tank is:
cost = 10900 + 1165.73 = $12,065.73
Therefore, the estimated current total cost for the installed and insulated tank is $12,065.73.
Learn more about cylinder current total cost https://brainly.com/question/25109150
#SPJ11
Find the integral of √(x² +4) W.R.T x
Answers
Answer:
\(\frac{x}{2} *\sqrt{x^{2} +4}\) +\(\frac{1}{2}\)*LN(|\(\frac{x+\sqrt{x^{2} +4} }{2}\)|) +C
Step-by-step explanation:
we will have to do a trig sub for this
use x=a*tanθ for sqrt(x^2 +a^2) where a=2
x=2tanθ, dx= 2 sec^2 (θ) dθ
this turns \(\int\limits {\sqrt{x^{2}+4 } } \, dx\) into integral(sqrt( [2tanθ]^2 +4) * 2sec^2 (θ) )dθ
the sqrt( [2tanθ]^2 +4) will condense into 2sec^2 (θ) after converting tan^2(θ) into sec^2(θ) -1
then it simplifies into integral(4*sec^3 (θ)) dθ
you will need to do integration by parts to work out the integral of sec^3(θ) but it will turn into (1/2)sec(θ)tan(θ) + (1/2) LN(|sec(θ)+tan(θ)|) +C
then you will need to rework your functions of θ back into functions of x
tanθ will resolve back into \(\frac{x}{2}\) (see substitutions) while secθ will resolve into \(\frac{\sqrt{x^{2} +4} }{2}\)
sec(θ)=\(\frac{\sqrt{x^{2} +4} }{2}\) is from its ratio identity of hyp/adj where the hyp. is \(\sqrt{x^{2} +4}\) and adj is 2 (see tan(θ) ratio)
after resolving back into functions of x, substitute ratios for trig functions:
= \(\frac{x}{2} *\sqrt{x^{2} +4}\) + \(\frac{1}{2}\)*LN(|\(\frac{x+\sqrt{x^{2} +4} }{2}\)|) +C
Alan makes a fruit salad using strawberries and blueberries. He uses 5
cups of strawberries for every 3 cups of blueberries. Which measure
represents the amount of strawberries Alan uses for every 1 cup of fruit
salad?
3/8 cup
3/5 cup
5/8 cup
5/3 cup

Answers
Answer:
5/8
Total = 5 cup of strawberry + 3 cup of blueberries
=8
1 cup of fruits=8 cup of mix fruits(5 cup of strawberry + 3 cup of blueberries)
=8-3
=5/8
Let Pij = the production of product i in period j. To specify that production of product 2 in period 4 and in period 5 differs by no more than 80 units, we need to add which pair of constraints?
P52-P42 <= 80; P42-P52 <= 80
None of the other above.
P24 - P25 <= 80; P25-P24 >= 80
O P24 - P25 >= 80; P25-P24 >= 80
P24 - P25 <= 80; P25-P24 <= 80
Answers
The correct pair of constraints that needs to be added to specify that production of product 2 in period 4 and in period 5 differs by no more than 80 units is: P24 - P25 <= 80; P25-P24 <= 80. Therefore, the correct option is 5.
Here, the given information is Pij = the production of product i in period j. We need to find the pair of constraints that will specify that production of product 2 in period 4 and in period 5 differs by no more than 80 units. Thus, let the production of product 2 in period 4 and in period 5 be represented as P24 and P25 respectively.
Therefore, we can write the following inequalities:
P24 - P25 <= 80
This is because the production of product 2 in period 5 can be at most 80 units less than that of period 4. This inequality represents the difference being less than or equal to 80 units.
P25-P24 <= 80
This is because the production of product 2 in period 5 can be at most 80 units more than that of period 4. This inequality represents the difference being less than or equal to 80 units.
Therefore, we need to add the pair of constraints P24 - P25 <= 80 and P25-P24 <= 80 to specify that production of product 2 in period 4 and in period 5 differs by no more than 80 units. Hence, option 5 is the correct answer.
Learn more about Constraints:
https://brainly.com/question/3469433
#SPJ11
An amusement park has two types of season passes. Plan 1 charges a one-time fee of $170.00 for admission plus $8.00 every trip for parking. Plan 2 charges a one-time fee of $93.00 for parking plus $15.00 every trip for admission. For what number of trips is the cost of these plans the same?
Answers
Answer:
Plan1:
y = 170 + 8x
Plan2:
y = 93 + 15x
170 + 8x = 93 + 15x
7x = 77
x = 11 Trips
Step-by-step explanation:
The number of trips when the cost of plan 1 and plan 2 would cost the same amount of money is 11 trips.
When the plans cost the same amount of money?The equation that reprepsents the total cost of both plans is:
Total cost = one time fee + (cost of parking x number of trips)
Plan 1 : 170 + 8t
Plan 2: 93 + 15t
When both plans cost the same, the two above equations would be equal.
170 + 8t = 93 + 15t
In order to determine the value of t, take the following steps:
Combine similar terms
170 - 93 = 15t - 8t
Add similar terms
77 = 7t
Divide both sides by 7
t = 77/7
t = 11 trips
To learn when two plans would cost the same, please check: https://brainly.com/question/25711114
Determine if the sequence below is arithmetic or geometric and determine the common difference / ratio in simplest form.16,8,4,...
Answers
Given sequence is geometric sequence and common ratio between consecutive term is
\(16,8,4\)In Arithmetic sequence , common difference between consecutive terms should be equal.
In Geometric sequence, common ratio between consecutive terms should be equal.
Given sequence, 16, 8, 4, ...
Since, common difference = 8 - 16 ≠ 4 - 8 , this is not arithmetic sequence.
Common ratio = T2/T1 = T3/T2 = 8/16 = 4/8 = 1/2 Because common ratio is are equal. Thus, given sequence is geometric sequence.
Hence the sequence is a geometric sequence and the common ratio = 1/2
14.Find the value of <A in the following figure.

Answers
Given:
The figure of triangle ABC.
To find:
The measure of angle A.
Solution:
From the given figure, we get
\(\angle ABC+120^\circ=180^\circ\) (Linear pair)
\(\angle ABC=180^\circ-120^\circ\)
\(\angle ABC=60^\circ\)
According to the exterior angle theorem, the exterior angle of a triangle is equal to the sum of two opposite interior angles.
\(\angle A+\angle ABC=105^\circ\) (Exterior angle theorem)
\(\angle A+60^\circ=105^\circ\)
\(\angle A=105^\circ-60^\circ\)
\(\angle A=45^\circ\)
Therefore, the measure of angle A is 45 degrees.
which of the following is true about the expression 5 x 1/4
Answers
Answer:
It represents the product of two rational numbers and is equivalent to a rational number.
Step-by-step explanation:
a bottle of lemonade normally contains 300 ml. special edition bottles have 10 % extra free. how much lemonade is in the special edition bottles?
Answers
Hence, there is 330 ml of lemonade in the special edition bottles.
The special edition bottles have 10% extra free, which means the total amount of lemonade in the special edition bottle is 100% (normal amount) + 10% (extra amount).
To calculate the amount of lemonade in the special edition bottles, we first need to determine the 10% of the normal amount (300 ml).
10% of 300 ml = (10/100) * 300 ml
= 30 ml
Therefore, the special edition bottles contain an extra 30 ml of lemonade.
To find the total amount of lemonade in the special edition bottles, we add the normal amount (300 ml) to the extra amount (30 ml):
Total amount of lemonade in special edition bottles = 300 ml + 30 ml
= 330 ml
To know more about lemonade,
https://brainly.com/question/20566002
#SPJ11
The coordinates of point T are (0,4). The midpoint of ST is (1, -3). Find the coordinates of point S.
Answers
Answer:
S=(2,-2) ...............
Geometry
Solve for x. Round to the nearest tenth, if necessary.
Bottom letter is F

Answers
Answer:
1. \(x \approx 229.8\)
2. \(x \approx 2.6\)
Step-by-step explanation:
1. We can use the following trigonometric ratio since we are given the adjacent side and need to find the hypotenuse from the angle:
\(cos(x) = \frac{adjacent}{hypotenuse}\\cos(72) = \frac{71}{x}\\x = \frac{71}{cos(72)}\\x \approx 229.8\)
2. We can use the following trigonometric ratio since we are given the adjacent side and need to find the opposite from the angle:
\(tan(x) = \frac{opposite}{adjacent}\\tan(43) = \frac{x}{2.8}\\x = tan(43) \times 2.8\\x \approx 2.6\)
In terms of relative growth rate, what is the defining property of exponential growth?
Answers
In terms of relative growth rate Exponential growth is characterized by a constant relative growth rate.
Exponential growth is the process of increasing quantity over time. Occurs when the instantaneous rate of change of a quantity over time is proportional to the quantity itself. The exponential growth model has the form
y (t) = C eᵏᵗ, where k is the rate constant.
Relative Growth Rate:Relative growth rate (RGR) is the rate of growth relative to size. That is, the rate of growth per unit time relative to the size at that point in time and y'(t)/y(t) is the relative growth rate of a function y at time t.
1/y dy/dt = 1/y d(C eᵏᵗ)/dt
= 1/y(kC eᵏᵗ)
= 1/y ( ky) ( since, y (t) = C eᵏᵗ)
= k
Therefore a constant relative growth rate.
To learn more about Exponential growth model, refer:
https://brainly.com/question/13223520
#SPJ4
Complete question:
In terms of relative growth rate, what is the defining property of exponential growth? Choose the correct answer below.
A. The relative growth rate at time t is the slope of the exponential function at time t.
B. dy dt If y represents a population, then the relative growth rate can be represented by dy/dt
C. The relative growth rate is proportional to the size of the population
D. The relative growth rate is constant.