6 - 4 2/7 =
ANSWER ASAP AND BE RIGHT
EXPLAINNNN STEP BY STEP
TO BE BRAINLIEST
Answers
Answer:
6 - 4 2/7 = 1.71428571
Step-by-step explanation:
is this good enough?
Related Questions
Which is larger than 8.15: 55/9 or 57/7 or 41/5 or 65/8
Answers
57/7 = 8.14
41/5 = 8.2
65/8 = 8.125
The answer is 41/5 is larger than 8.15
Step-by-Step Explanation:
Simply divide each of the fractions, and leave your answer in 2 decimal places.
1. 55/9 = 6.11
2. 57/7 = 8.14
3. 41/5 = 8.20
4. 65/8 = 8.12
From the results, we find out that 41/5 is equal to 8.20 which is bigger than 8.15.
Hence, 41/5 is your answer.
PLS HELP ASAP THANKS ILL GIVE BRAINLKEST PLS THANKS

Answers
Answer:
I think it's y=x+2 if not then I'm sorry
Answer: y=x-7
Step-by-step explanation:
Here’s the photo of my explanation, if it helps

The scores of a random sample of 8 students on a physics test are as follows: (a) Test to see if the sample mean is significantly different from 85 at the 0.05 level. Report the t and p values. Are these scores significantly different from 85 at the 0.05 level? A. Yes B. No C. Maybe
Answers
The given problem is asking for a test to see if the sample mean is significantly different from 85 at the 0.05 level. To solve the problem, we can use the following formula:$$t = \frac{\bar{x} - \mu}{\frac{s}{\sqrt{n}}}$$where$\bar{x}$ = sample mean$\mu$ = population mean$s$ = sample standard deviation$n
$ = sample sizeTo calculate the t-value, we need to calculate the sample mean and the sample standard deviation. The sample mean is calculated as follows:$$\bar{x} = \frac{\sum_{i=1}^{n} x_i}{n}$$where $x_i$ is the score of the $i$th student and $n$ is the sample size.
Using the given data, we get:$$\bar
{x} = \frac{78+89+67+85+90+83+81+79}{8}
= 81.125$$The sample standard deviation is calculated as follows:$$
s = \sqrt{\frac{\sum_{i=1}^{n} (x_i - \bar{x})^2}{n-1}}$$Using the given data, we get:$$
s = \sqrt{\frac{(78-81.125)^2+(89-81.125)^2+(67-81.125)^2+(85-81.125)^2+(90-81.125)^2+(83-81.125)^2+(81-81.125)^2+(79-81.125)^2}{8-1}}
= 7.791$$Now we can calculate the t-value as follows:$$
t = \frac{\bar{x} - \mu}{\frac{s}
To know more about problem visit:
https://brainly.com/question/31816242
#SPJ11
PLEASE HELP ASAP
Use the graph below for this question
What is the average rate of change from x = 0 to x = 2?
-4
-8
1
5
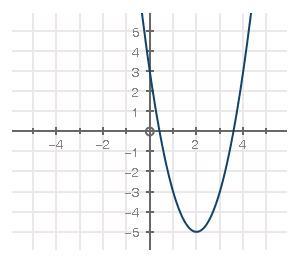
Answers
Answer:
The average rate will be: -4
Hence, option A is correct.
Step-by-step explanation:
Given the interval
0 ≤ x ≤ 2
From the graph, it is clear that:
at x₁ = 0, f(x₁) = 3
at x₂ = 2, f(x₂) = -5
Using the formula to determine the average rate of change:
Average rate = [f(x₂) - f(x₁)] / [ x₂ - x₁]
= [-5 - 3] / [2-0]
= -8 / 2
= - 4
Therefore, the average rate will be: -4
Hence, option A is correct.
Please Help
A triangle has a base of 13 inches and an area of 82.5 inches. What is the height of the triangle?
Answers
Answer:
Height - 12.692 in
Step-by-step explanation:
Answer:
A = 1/2 bh
Step-by-step explanation:
Replace are and base to get:
82.5 = 1/2 x 15 x height
Multiply both sides by 2:
165 = 15 x height
Divide both sides by 15:
Height = 165 / 15
Height = 11 inches.
Carly's family traveled 3/10 of the distance to her grandmother's house on Saturday. They traveled 3/7 of the remaining distance on Sunday. What fraction of the total distance to her grandmother's house was traveled on Sunday?
Answers
Answer:
3/10 of the total distance to her grandmother's house was traveled on Sunday
Step-by-step explanation:
Carly's family traveled distance of Saturday = \(\frac{3}{10}\)
Remaining distance =\(1-\frac{3}{10}=\frac{7}{10}\)
They traveled 3/7 of the remaining distance on Sunday.
So, Distance traveled on Sunday =\(\frac{3}{7}(\frac{7}{10})\)
Distance traveled on Sunday =\(\frac{3}{10}\)
Fraction of the total distance to her grandmother's house was traveled on Sunday =\(\frac{3}{10}\)
Hence 3/10 of the total distance to her grandmother's house was traveled on Sunday
Danielle eams an 8.25% commission on everything she sells at the electronics store where she works. She
also earns a base salary of $700 per week. How much did she earn last week if she sold $4,700 in
electronics merchandise? Round your intermediate calculations and answer to the nearest cent.
Danielle eamed a total of $
Answers
Based on the total amount that Danielle sold last week, the amount that she earned from her commission and base salary was $1,087.75
How to find the amount earned by Danielle?Danielle gets a total earning of both her commission which is 8.25% on goods sold, and a base salary of $700 per week.
In the given week, she sold $4,700 which means that the commission was:
= 4,700 x 8.25%
= $387.75
This means that Danielle's total earnings for the week was:
= 700 + 387.75
= $1,087.75
Find out more on commission earned at https://brainly.com/question/19607777
#SPJ1
A fair coin is flipped three times, and the number of times it comes up "heads” is recorded.
Have the conditions for a binomial setting been met for this scenario?
No, this is a fair coin.
Yes, all four conditions in BINS have been met.
No, the coin flips are not independent of one another.
No, the coin needs to be flipped until “heads” appears. The number of trials needed to get “heads” should be recorded.
Answers
Answer:
B)Yes, all four conditions in BINS have been met.
Step-by-step explanation:
Yes, all four conditions in BINS have been met.
Step-by-step explanation:Definition of conditional probabilityConditional probability is defined as the likelihood of an event or outcome occurring, based on the occurrence of a previous event or outcome. Conditional probability is calculated by multiplying the probability of the preceding event by the updated probability of the succeeding, or conditional, event.
To know more about conditional probability click on given link
https://brainly.com/question/23412390
#SPJ2
The perpendicular bisectors of AABC intersect at point
G. Find the length of BG.
B

Answers
Answer:
\(\overline{GB}\) = 9
Step-by-step explanation:
The given parameters are;
The point of intersection of the perpendicular bisectors of the sides \(\overline{BC}\), \(\overline{BA}\), and \(\overline{CA}\) intersect (meet) at point G
Let D represent the point of intersection of the perpendicular bisector from G to \(\overline{BC}\), we have for ΔBGD and ΔCGD;
\(\overline{GD}\) ≅ \(\overline{GD}\) by reflexive property
∠GDB = ∠GDC = 90° given that \(\overline{GD}\) is the perpendicular bisector of \(\overline{BC}\)
Similarly, \(\overline{DB}\) ≅ \(\overline{DC}\), also given that \(\overline{GD}\) is the perpendicular bisector of \(\overline{BC}\)
Therefore;
ΔBGD ≅ ΔCGD by Side-Angle-Side (SAS) rule of congruency
\(\overline{GB}\) ≅ \(\overline{GC}\) by Congruent Parts of Congruent Triangles are Congruent, (CPCTC)
\(\overline{GB}\) = \(\overline{GC}\) = 9 by definition of congruency
∴ \(\overline{GB}\) = 9.
would a 7 inch long pen fit in a box with dimensions of 3 in x 4 in x 5 in? explain please
Answers
The 7 inches pen would not fit in the box with dimensions 3 in × 4 in × 5 in
How to answer the question on dimensionsThe 7 inches long pen would not fit in a box with dimensions of 3 inches x 4 inches x 5 inches because the dimensions of the box are not long enough to accommodate the length of the pen.
Specifically, the length dimension of the box (3 inches) is less than the length of the pen (7 inches), so the pen would not be able to fit inside the box.
Additionally, even if the length of the box was greater than 7 inches, the width and height dimensions of the box (4 inches and 5 inches, respectively) would likely be too small to accommodate the diameter of the pen.
In conclusion, the pen is too long for the box, and the box is not wide or tall enough to fit the pen.
Know more about dimensions here:https://brainly.com/question/27934593
#SPJ1
Solve the equation x + 5 = 12. Show and justify each step you take to solve the equation. x + 5 = 12 Write the equation. = 12 x + 5 Х X = Coning is not nermitted.
Answers
Answer:
7
Step-by-step explanation:
x+5=12
x=12-5
x=7

The prom committee is decorating the venue for prom and wants to hang lights above the diagonals of the rectangular room. If DH=44.5 feet, EF=39 feet, and m∠GHF=128° , find m∠HEF .
Answers
The measure of the angle m∠HEF = 64°, for the diagonals of rectangular room.
Define the term linear pair?A linear pair is just a pair of neighboring angles created by the intersection of two lines. 1 and 2 create a linear pair in the illustration. The same holds true for pairs 1, 2, 3, and 4. A linear pair's two angles are always supplementary, that means that the sum of their measurements is 180 degrees.The given data:
DH=44.5 feet, EF=39 feet, and m∠GHF=128°As, GE is the straight line.
Thus,
m∠EHF = 180 - 128 (sum of all angles of line is 180, linear pair)
= 52°
Now,
m∠HEF = 180 - 52 / 2 (isosceles triangle)
m∠HEF = 64°
Thus, the measure of the angle m∠HEF = 64°, for the diagonals of rectangular room.
To know more about the linear pair, here
https://brainly.com/question/18944511
#SPJ1
The diagram for the question is attached.

X and Y have a constant joint density on the triangle where OSX SY51. What type of probability mass/density of Random Variables is this? Choose the one that best applies. A. X,Y Joint Density of a C. Uniform Random Variable and a function of that Random Variable B. X,Y Joint Mass of Two Bernoulli Random Variables C. X,Y Joint Mass of a D. Uniform Random Variable and a function of that Random Variable OD. X,Y Joint Density of Two Continuous Random Variables E. X,Y Joint Constant Density of 2 Random Variables over a Region OF. X,Y Joint Mass of Two Discrete Random Variables
Answers
The appropriate answer is option E: X,Y Joint Constant Density of 2 Random Variables over a Region.
The given scenario states that X and Y have a constant joint density on the triangle where OSX SY51. This means that the joint density of X and Y is constant over the given region. The correct option that applies to this situation is E. X,Y Joint Constant Density of 2 Random Variables over a Region. Because, options A, B, and D do not fit the scenario as they involve uniform, Bernoulli, and uniform functions of a random variable, respectively. Option C involves a discrete uniform random variable, which does not match the continuous nature of the given problem. Option F involves two discrete random variables with joint mass, which again does not fit the continuous nature of the problem.
Learn more about: joint density - https://brainly.com/question/30010853
#SPJ11
ABC is a triangle inscribed in a circle AB=AC=10cm , BC=16cm. The chord AE is at right angle to the chord BC at D.Calculate DE and the radius of the circle
Answers
help 6th grade math i will give brainliest

Answers
Step-by-step explanation:
plz give brainlyest
4* 53+1/(8-5)^3
4(54)/(8−5)3
4(54/3^3)
4(54/27)
(4)(2)=8 the answer is 8
Answer:
Add 53 + 1
Step-by-step explanation:

24-x-24=-16-24
Solve each step.
Answers
24-x-24=-16-24
1. Subtract the numbers
-x=-16-24
2. -x=-16-24
-x=-40
3.Divide both sides of the equation by the same term
-x=-40
-x/-1=-40/-1
4. simplify
x=40
What is the term for the probability that a value falling outside the control limits is still due to normal variation
Answers
The term for the probability that a value falling outside the control limits is still due to normal variation is called the Type I error or the alpha error.
The term for the probability that a value falling outside the control limits is still due to normal variation is called the Type I error or alpha error.
It refers to the probability of rejecting a true null hypothesis (in this case, the process is in control) when it should not be rejected. In other words, it is the probability of concluding that there is a problem with the process when there is actually no problem, and the observed value is simply a result of random variation.
This means that the value appears to be abnormal, but it is actually just a result of the natural variation in the process. It is important to minimize the risk of Type I errors to ensure that only truly abnormal values are addressed and corrected.
Know more about probability here:
https://brainly.com/question/13604758
#SPJ11
Please help
7r+18=-2(6-6r)
Answers
Answer:
r = 6
Step-by-step explanation:
Distribute -2 to (6 - 6r):
7r + 18 = -12 + 12r
Subtract 7r from both sides of the equation:
7r + 18 - 7r = -12 + 12r - 7r
18 = -12 + 5r
Add 12 to both sides of the equation to isolate 5r:
18 + 12 = -12 + 5r + 12
30 = 5r
Divide both sides of the equation by 5 to isolate r:
\(\frac{30}{5} = \frac{5r}{5}\)
6 = r
To double check whether the value of r = 6 is correct, plug it into the original equation:
7r + 18 = -2(6 - 6r)
7(6) + 18 = -2[6 - 6(6)]
42 + 18 = -12 + 72
60 = 60
Suppose there are two producers in a market with the following supply functions. Supply 1: P=6+0.7Q Supply 2:P=16+0.6Q When the price is [Answer], the total quantity supplied is 250. (In decimal numbers, with two decimal places, please.) Answer:
Answers
The price at which the total quantity supplied is 250 is $11.58.
In order to find the price at which the total quantity supplied is 250, we need to equate the total quantity supplied by both producers (Supply 1 and Supply 2) and solve for the price.
Supply 1: P = 6 + 0.7Q
Supply 2: P = 16 + 0.6Q
To find the equilibrium price, we set the total quantity supplied equal to 250:
0.7Q + 0.6Q = 250
1.3Q = 250
Q = 250 / 1.3 ≈ 192.31
Now that we have the quantity, we can substitute it back into either supply function to find the price. Let's use Supply 1:
P = 6 + 0.7Q
P = 6 + 0.7 * 192.31
P ≈ 6 + 134.62
P ≈ 140.62
Therefore, the price at which the total quantity supplied is 250 is approximately $11.58.
Learn more about Equilibrium price
brainly.com/question/29099220
#SPJ11
Rectangle with width labeled on the right, width equals 4 and 1 over 2 inches. Below the rectangle, it says, length equals question mark. Inside the rectangle, it says area equals 36 and 3 over 4 square inches.
Answers
Answer:
length = \(8\frac{1}{6}\) inches
Step-by-step explanation:
A rectangle is a quadrilateral which has opposite sides to be equal.
Area of a rectangle = length × width
Given that: Area = \(36\frac{3}{4}\) square inches, width = \(4\frac{1}{2}\) inches, length = l. Thus;
\(36\frac{3}{4}\) = l × \(4\frac{1}{2}\)
\(\frac{147}{4}\) = l × \(\frac{9}{2}\)
divide both sides by \(\frac{9}{2}\),
l = \(\frac{147}{4}\) ÷ \(\frac{9}{2}\)
= \(\frac{147}{4}\) x \(\frac{2}{9}\)
= \(\frac{49}{6}\)
l = \(8\frac{1}{6}\)
Therefore, the length of the rectangle is \(8\frac{1}{6}\) inches.
According to a 2009 Reader's Digest article, people throw away about 10% of what they buy at the grocery store. Assume this is the true proportion and you plan to randomly survey 119 grocery shoppers to investigate their behavior. What is the probability that the sample proportion does not exceed 0.16? Note: You should carefully round any z-values you calculate to 4 decimal places to match wamap's approach and calculations. Answer = ? (Enter your answer as a number accurate to 4 decimal places.)
Answers
To calculate the probability that the sample proportion does not exceed 0.16, we use the normal distribution and assume that the true proportion is 10%. The sample size is 119 grocery shoppers. The answer should be provided as a number accurate to four decimal places.
To calculate the probability, we need to standardize the sample proportion using the standard error formula for proportions:
Standard Error = sqrt[(p * (1-p)) / n]
Where p is the assumed true proportion (10%) and n is the sample size (119). Plugging in the values:
Standard Error = sqrt[(0.10 * (1-0.10)) / 119] ≈ 0.0301
Next, we calculate the z-score using the formula:
z = (x - p) / Standard Error
Plugging in x = 0.16 (sample proportion), p = 0.10, and the calculated Standard Error:
z = (0.16 - 0.10) / 0.0301 ≈ 1.9934
Finally, we find the probability using the standard normal distribution table or calculator. The probability that the sample proportion does not exceed 0.16 is approximately 0.9767.
To know more about standard normal distribution here: brainly.com/question/15103234
#SPJ11
Can you figure this out for me ? I’m having issues .

Answers
Answer:
71
you just had to add them
Step-by-step explanation:
1. suppose a point is to be selected at random from the unit square. write the sample space associated with this random experiment.
Answers
a) The x-range of a unit square is from o to 1 and y-range is also from 0 to 1
Sample space = {(x, y)} , 0≤x≤1, 0≤y≤1.
So, sample space associated with this random experiment is
{(x, y)} , 0≤x≤1, 0≤y≤1.
b) From the given data,
Sample space for A : {1, 3, 5, 7, 9, 11,...}
Sample space for B: {5, 10, 15, 20, 25,...}
Therefore, the sample space for A∩B will be the set of all odd natural numbers divisible by 5 which is
{5, 15, 25, 35,....}
c) First letter is the capital letter = 26 Possibilities
then four digits = 9*10*10*10
two lower case letters = 26*26
Total possible ways = 26*9*10*10*10*26*26
= 15,81,84,000 ways.
Learn more about Possibilities:
https://brainly.com/question/30034044
#SPJ4
Complete question:
Suppose a point is to be selected at random from the unit square. Write the sample space associated with this random experiment. 2. Let S = {0, 1, 2, 3, 4, ...), A = the set of odd natural numbers, and B = the set of natural numbers divisible by 5. What is the set An B? 3. A computer password consists of two lowercase letters followed by a capital letter and four digits. Find the total number of possible passwords.
assume that 30% of students at a university wear contact lenses. a random sample of 100 students is selected.
Answers
30% of students mean 30 students wear contact lenses from a random selection of 100 students studying in a university.
What is meant by percentage?Percentage is a fraction or a ratio of a random sample in which the value of whole is always 100. For example, if Sam received 30% on his arithmetic test, he received 30 points out of 100. It is expressed as 30/100 in fractional form and 30:100 in ratio form.
Percentage is defined as a certain fraction or number out of a hundred. It is a fraction with the denominator 100, and the sign for it is "%."
Finding the proportion of a whole in terms of 100 is the goal of percentage calculations. There are two methods to calculate a percentage:
Use the unitary approach or try adjusting the fraction's denominator to 100.
How to solve?
30 % = 30/100 of a sample = 0.3 of a sample
In order to learn more about percentage, visit:
https://brainly.com/question/29306119
#SPJ4
Vasco's utility function is: U=10x
2
z The price of X is p
X
=$10, the price of Z is p
Z
=$2, and his income is $150. What is his optimal bundle? (round your answer to two decimal places) x
0
= units z
0
= units
Answers
Vasco's optimal bundle, we need to maximize his utility function U = 10x²z subject to his budget constraint.
His budget constraint can be written as:10x + 2z = 150
To solve this problem, we can use the method of Lagrange multipliers. The Lagrangian function is:L = 10x²z + λ(150 - 10x - 2z)
Taking the partial derivatives with respect to x, z, and λ, and setting them equal to zero, we have:
∂L/∂x = 20xz - 10λ = 0 (1)
∂L/∂z = 10x² - 2λ = 0 (2)
∂L/∂λ = 150 - 10x - 2z = 0 (3)
From equation (1), we have: 20xz = 10λ (4)
From equation (2), we have: 10x² = 2λ (5)
Dividing equation (4) by equation (5), we get:
(20xz) / (10x²) = (10λ) / (2λ)
2z / x = 5
Rearranging the equation, we have:
z = 5x / 2
Substituting this into equation (3), we have: 150 - 10x - 2(5x / 2) = 0
Simplifying the equation, we get: 150 - 10x - 5x = 0
15x = 150
x = 10
Substituting the value of x into z = 5x / 2, we have:
z = 5(10) / 2
z = 25
Therefore, the optimal bundle for Vasco is x = 10 units of X and z = 25 units of Z.
Learn more about Function here:
https://brainly.com/question/30721594
#SPJ11
How many solutions does the equation (2 sin x + 1)(sin x – 1) = 0 have over the interval 0 ≤ x < 2π?
a. 1
b. 2
c. 3
d. 4
Answers
let ⊂ , ⊂ be any two disjoint events such that: P() = 0.4, P( ∪ ) = 0.7. Find: ) P( c). ii) P( c ), iii)probability that exactly one of the events A,B occurs
Answers
The proababilities are: i) P(Aᶜ) = 0.6, ii) P(Bᶜ) = 0.4
iii) Probability that exactly one of the events A, B occurs = 0.7
Let A and B be any two disjoint events such that P(A) = 0.4 and P(A ∪ B) = 0.7. We need to find the following probabilities:
i) P(Aᶜ): This is the probability of the complement of event A, which represents the probability of not A occurring. Since A and B are disjoint, Aᶜ and B are mutually exclusive and their union covers the entire sample space.
Therefore, P(Aᶜ) = P(B) = 1 - P(A) = 1 - 0.4 = 0.6.
ii) P(Bᶜ): This is the probability of the complement of event B, which represents the probability of not B occurring. Since A and B are disjoint, Bᶜ and A are mutually exclusive and their union covers the entire sample space.
Therefore, P(Bᶜ) = P(A) = 0.4.
iii) Probability that exactly one of the events A, B occurs: This can be calculated by subtracting the probability of both events occurring (P(A ∩ B)) from the probability of their union (P(A ∪ B)).
Since A and B are disjoint, P(A ∩ B) = 0.
Therefore, the probability that exactly one of the events A, B occurs is P(A ∪ B) - P(A ∩ B) = P(A ∪ B) = 0.7.
To summarize:
i) P(Aᶜ) = 0.6
ii) P(Bᶜ) = 0.4
iii) Probability that exactly one of the events A, B occurs = 0.7
Note: The provided values of P(A), P(A ∪ B), and the disjoint nature of A and B are used to derive the above probabilities.
To learn more about Probability visit:
brainly.com/question/30034780
#SPJ11
which parent function is f(x)=|x|?
Answers
Answer: We can see that it has a parabola for its graph, so we can say that f(x) is a quadratic function. This means that f(x) has a parent function of y = x2.
Step-by-step explanation:
(Hope this helped Btw not mine)
Answer:
Absolute value parent function
Step-by-step explanation:
In the circle below, segment CB is a diameter. If the length of CDB is 14, what is the length of the radius of the circle?

Answers
GIVEN:
We are given a circle with center A and diameter CB.
The length of arc CDB is 14;
\(arcCDB=14\pi\)Required;
We are required to calculate the lenght of the radius.
Step-by-step solution;
The length of an arc with the central angle measured in radians is;
\(s=r\theta\)The length of the arc is given, so also is the angle theta (180 degrees, that is half the circle).
We can convert the degree measure of the angle into radians as follows;
\(\begin{gathered} Degrees\Rightarrow Radians \\ \frac{r}{\pi}=\frac{d}{180} \end{gathered}\)Now we substitute the value of d (degree measure);
\(\begin{gathered} \frac{r}{\pi}=\frac{180}{180} \\ \\ \frac{r}{\pi}=1 \\ \\ Cross\text{ }multiply: \\ \\ r=\pi \end{gathered}\)Now we take the formula for the length of an arc as follows;
\(\begin{gathered} s=r\theta \\ Therefore: \\ \\ 14\pi=r\pi \end{gathered}\)Divide both sides by pi;
\(14=r\)Therefore, the radius is
ANSWER:
\(Radius=14\text{ }units\)Diego, age 28, married Dolores, age 27, in 2020. Their salaries for the year amounted to $49,300 and they had interest income of $875. Diego and Dolores' deductions for adjusted gross income amounted to $1,660; their itemized deductions were $10,425, and they have no dependents. Table for the standard deduction
Filing Status 2017 Standard Deduction
Single $ 6,350
Married, filing jointly 12,700
Married, filing separately 6,350
Head of household 9,350
Qualifying widow(er) 12,700
a. What is the amount of their adjusted gross income?
b. In order to minimize taxable income, Diego and Dolores will in the amount of $.
c. What is the amount of their taxable income?
d. What is their tax liability for 2017?
Answers
a. The amount of their adjusted gross income is $48,515.
b. Diego and Dolores should itemize deductions in the amount of $10,425 to minimize taxable income.
c. Their taxable income is $38,090.
d. Without the specific year's tax brackets and rates, the exact tax liability can not be provided.
We have,
a. To calculate the adjusted gross income (AGI), we need to subtract the deductions from their total income:
Total income = Salaries + Interest income
Total income = $49,300 + $875
Total income = $50,175
Adjusted gross income = Total income - Deductions for adjusted gross income
Adjusted gross income = $50,175 - $1,660
Adjusted gross income = $48,515
b. To minimize taxable income, Diego and Dolores should choose the higher value between the standard deduction for married filing jointly ($12,700) and their itemized deductions ($10,425).
Therefore, they should itemize deductions in the amount of $10,425.
c. Taxable income is calculated by subtracting deductions (either standard or itemized) from the adjusted gross income:
Taxable income = Adjusted gross income - Deductions
Taxable income = $48,515 - $10,425
Taxable income = $38,090
d. To determine the tax liability, we need to refer to the tax brackets and rates for the specific year.
Since the information provided does not specify the year, It is unable to provide the exact tax liability.
Tax brackets and rates can vary from year to year.
Thus,
a. The amount of their adjusted gross income is $48,515.
b. Diego and Dolores should itemize deductions in the amount of $10,425 to minimize taxable income.
c. Their taxable income is $38,090.
d. Without the specific year's tax brackets and rates, the exact tax liability can not be provided.
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ4