You are at a restaurant and the check comes to a total of $23. If you want to leave a 20% tip, how much total money should you pay, to the nearest cent?
Answers
Answer:
$27.60
Step-by-step explanation:
23×1.2=27.60 is the answer
Related Questions
Can you please help me
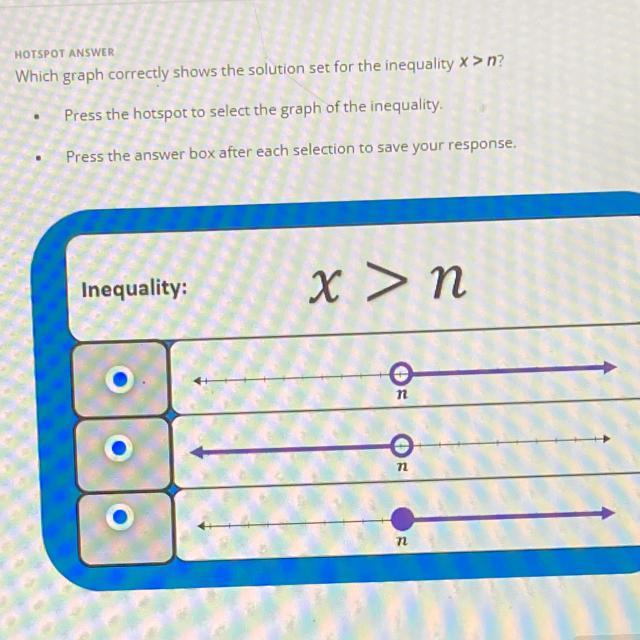
Answers
Answer:
first graph
Step-by-step explanation:
x > n
the graph will have an open circle at the value n , indicating that x cannot equal n ( note solid circle if x ≥ n )
the arrow from n will point in the right direction, indicating values of x greater than n.
the graph representing x > n is the first graph
Please help, I’ll mark your answer as brainliest.

Answers
Loudness, pitch, and timbre are the three psychological aspects of sound that are influenced by the physical properties of sound waves.
What is a sound wave?When energy moves through a medium (such as air, water, or any other liquid or solid matter), it creates a pattern of disturbance known as a sound wave that propagates away from the sound source.
When an object vibrates, sound waves are produced that are similar to pressure waves, like when a phone rings.
Pitch, intensity, and timbre are three key components of the field of sound psychology or psychoacoustics. People can distinguish the harmonics of a complex periodic sound wave under certain conditions thanks to the way that humans perceive sound and music.
Thus, loudness, pitch, and timbre are the three psychological aspects of sound that are influenced by the physical properties of sound waves.
Learn more about the sound wave here,
https://brainly.com/question/21995826
#SPJ1
Which inequalities have -0.5, 2.5, and 4 as part of the solution set? Select all that apply.
z > -3
z 0
Answers
Answer: o.5 , z >-3
Step-by-step explanation:
just divide
Answer:
z > -3 (this is correct)
z < 5 (this is also correct)
z < 4 (this is incorrect)
z Less-than-or-equal-to 4 (this is correct)
z > 0 (this is incorrect)
Find the area of the trapezoid.
( The answer 10.5 is not correct )

Answers
Answer:
42 units
Step-by-step explanation:
Area of a trapezoid is always 1/2(b1+b2)*h
so here base 1 is 4 units, and base 2 is 10 units. When we add these we get 14, divided by 2 which is 7, and multiplied by height of 6, which is 42
determine the maximum and minimum values of the function, at what values of x are they achieved? (without using a derivative)
\(y=\sin^3x-\sin^6x\)
Answers
The maximum and minimum values of the function is solved
Given data ,
We can find the maximum and minimum values of the function by taking the derivative of y with respect to x and setting it equal to zero.
y = (sin x)³ - (sin x)⁶
y' = 3(sin x)² cos x - 6(sin x)⁵ cos x
Setting y' equal to zero:
0 = 3(sin x)² cos x - 6(sin x)⁵ cos x
0 = 3(sin x)² cos x (1 - 2(sin x)³)
sin x = 0 or (sin x)³ = 1/2
If sin x = 0, then x = kπ for any integer k.
If (sin x)³ = 1/2, then sin x = (1/2)^(1/3) ≈ 0.866. This occurs when x = π/3 + 2kπ/3 or x = 5π/3 + 2kπ/3 for any integer k.
To determine whether these values correspond to a maximum or minimum, we can use the second derivative test.
y'' = 6(sin x)³ cos² x - 15(sin x)⁴ cos² x - 9(sin x)⁴ cos x + 6(sin x)⁵ cos x
y'' = 3(sin x)³ cos x (4(sin x)² - 5(sin x)² - 3cos x + 2)
For x = kπ, y'' = 3(0)(-3cos(kπ) + 2) = 6 or -6, depending on the parity of k. This means that these points correspond to a maximum or minimum, respectively.
For x = π/3 + 2kπ/3 or x = 5π/3 + 2kπ/3, y'' = 3(1/2)^(5/3) cos x (4(1/2)^(2/3) - 5(1/2)^(1/3) - 3cos x + 2). This expression is positive for x = π/3 + 2kπ/3 and negative for x = 5π/3 + 2kπ/3, which means that the former correspond to a minimum and the latter to a maximum.
Hence , the maximum value of the function is y = 27/64, which occurs at x = 5π/3 + 2kπ/3, and the minimum value is y = -1/64, which occurs at x = π/3 + 2kπ/3
To learn more about function rule click :
https://brainly.com/question/3760195
#SPJ1
Answer:
maximum: 0.25minimum: -2Step-by-step explanation:
You want the maximum and minimum values of the function ...
y = sin³(x) -sin⁶(x)
SolutionWhen we substitute sin³(x) = z, we have the quadratic expression ...
y = z -z² . . . . . a quadratic function
Adding and subtracting 1/4, we can put this in vertex form:
y = -(z -1/2)² +1/4
MaximumThis version of the function describes a parabola that opens downward and has a vertex at (z, y) = (1/2, 1/4). The y-value of the vertex represents the maximum value of the function.
The maximum value of y is 1/4.
MinimumThe sine function is a continuous function with a range of [-1, 1]. Then z will be a continuous function of x, with a similar range. We already know that y describes a function of z that is a parabola opening downward with a line of symmetry at z = 1/2. This means the most negative value of y will be found at z = -1 (the value of z farthest from the line of symmetry). That value of y is ...
y = (-1) -(-1)² = -1 -1 = -2
The minimum value of y is -2.
__
Additional comment
The range of y is confirmed by a graphing calculator.
<95141404393>

VERY EASY, WILL GIVE 50 POINTS FOR CORRECT ANSWER ASAP AND WILL GIVE BRAINLIEST.

Answers
Answer: (4,-11)
Step-by-step explanation: To perform a 180 degree rotation we switch the signs of the coordinates
Answer:
4,-11
Step-by-step explanation:
The answer is 4,-11 because if you think that you go over -4 and go up 11. Now switch the negative sign to the y coordinate because it is a 180 degree turn then you have your answer. 4,-11
pls mark as brainliest!!
help help help help help help

Answers
Answer:
1.parenthesis, exponents, multiplication, division, addition, subtraction (pemdas is the Oder of operations)
2. please enjoy my dish and seafood
3.14
4. 4
5. 5
6. 7
7.25
please help me asap!!

Answers
Answer:
21
Step-by-step explanation:
RS = 21
The compression ratio in a certain engine is 7.5 to 1. If the expanded volume of a cylinder is 15 cu in., what is the compressed volume?
Answers
On solving the provided question, we can say that inches if a cylinder's enlarged capacity is 45 in3, its compressed volume is 6 in cubic .
what is cylinder?One of the most fundamental curved geometric forms is the cylinder, which is often a three-dimensional solid. It is referred to as a prism with a circle as its base in elementary geometry. Several contemporary fields of geometry and topology also define a cylinder as an indefinitely curved surface. A three-dimensional object known as a "cylinder" consists of curving surfaces with circular tops and bottoms. A cylinder is a three-dimensional solid figure that has two bases that are both identical circles joined by a curving surface at the height of the cylinder, which is determined by the distance between the bases from the center. Examples of cylinders are cold beverage cans and toilet paper wicks.
In a certain engine, the compression ratio is 7.5: 1.
A cylinder's enlarged volume is 45 in3 in.
We must determine the cylinder's compressed volume.
Let v represent the cylinder's compressed volume.
The aforementioned circumstance can be described as
7.5 : 1 = expanded volume : compressed volume
7.5/1 = 45/v
7.5 = 45/v
v = 45/7.5
v = 6 cubic inches .
Therefore, if a cylinder's enlarged capacity is 45 in3, its compressed volume is 6 in cubic .
To know more about cylinder visit:
https://brainly.com/question/16134180
#SPJ1
find the ratio of 20 : 50
Answers
Answer:
20:50
20/50=40%
The circumference of a circle is 18pi meters. What is the radius?Give the exact answer in simplest form. ____ meters. (pi, fraction)
Answers
Given:
The circumference of a circle, C=18π m.
The expression for the circumference of a circle is given by,
\(C=2\pi r\)Put the value of C in the above equation to find the radius.
\(\begin{gathered} 18\pi=2\pi r \\ r=\frac{18\pi}{2\pi} \\ r=9\text{ m} \end{gathered}\)Therefore, the radius of the circle is 9 m.
NEED HELP ASAP WILL GIVE BRAINLIEST REAL ANSWERS ONLY PLZ WILL REPORT FAKE ANSWERS

Answers
Answer:
Option C. f(n) = 16(3/2)⁽ⁿ¯¹⁾
Step-by-step explanation:
To know which option is correct, do the following:
For Option A
f(n) = 3/2(n – 1) + 16
n = 1
f(n) = 3/2(1 – 1) + 16
f(n) = 3/2(0) + 16
f(n) = 16
n = 2
f(n) = 3/2(n – 1) + 16
f(n) = 3/2(2 – 1) + 16
f(n) = 3/2(1) + 16
f(n) = 3/2 + 16
f(n) = 1.5 + 16
f(n) = 17.5
For Option B
f(n) = 3/2(16)⁽ⁿ¯¹⁾
n = 1
f(n) = 3/2(16)⁽¹¯¹⁾
f(n) = 3/2(16)⁰
f(n) = 3/2 × 1
f(n) = 1
For Option C
f(n) = 16(3/2)⁽ⁿ¯¹⁾
n = 1
f(n) = 16(3/2)⁽¹¯¹⁾
f(n) = 16(3/2)⁰
f(n) = 16 × 1
f(n) = 16
n = 2
f(n) = 16(3/2)⁽ⁿ¯¹⁾
f(n) = 16(3/2)⁽²¯¹⁾
f(n) = 16(3/2)¹
f(n) = 16(3/2)
f(n) = 8 × 3
f(n) = 24
n = 3
f(n) = 16(3/2)⁽ⁿ¯¹⁾
f(n) = 16(3/2)⁽³¯¹⁾
f(n) = 16(3/2)²
f(n) = 16(9/4)
f(n) = 4 × 9
f(n) = 36
For Option D
f(n) = 8n + 8
n = 1
f(n) = 8(1) + 8
f(n) = 8 + 8
f(n) = 16
n = 2
f(n) = 8n + 8
f(n) = 8(2) + 8
f(n) = 16 + 8
f(n) = 24
n = 3
f(n) = 8n + 8
f(n) = 8(3) + 8
f(n) = 24 + 8
f(n) = 32
From the above illustration, only option C describes the sequence.
The Price of Pollo
In El Salvador, "Country Chicken" is the most popular fried chicken franchise
in the country. Like most fast-food establishments, they provide a carry-out
service on their menu. You can buy their chicken in several different
quantities: 2, 6, 9, 15, or 21 pieces per box.
Over the years, prices have steadily risen, as things have a way of doing in
many areas of modern life. For example, on July 1, 1993, a box of two
pieces cost 8.35 colones, and on December 31, 1995, that same purchase
would cost you 11.25 colones.
(Note: prices are given in their original Salvadorean currency, colones; $1 U.S
colones.)
Using these two data 'points', you can form a linear equation of the slope-inter
mx + b. The independent variable x is time; the dependent variable y represe
Your task for this problem is to:
1. Find this equation.
2. Use your equation to predict what the price should have been for a 2
July 1, 1999.
Answers
Answer:
Hope I helped!~
Step-by-step explanation:
To find the equation of the line, we can use the slope-intercept form of the equation:
y = mx + b
where m is the slope of the line and b is the y-intercept.
Using the two data points given, we can calculate the slope:
m = (11.25 - 8.35) / (1995 - 1993) = 1.95
To find the y-intercept, we can use one of the data points:
8.35 = 1.95(1993) + b
b = -3884.65
So the equation of the line is:
y = 1.95x - 3884.65
To predict the price of a box of two pieces on July 1, 1999, we can substitute x = 6 (since 1999 is 6 years after 1993) into the equation:
y = 1.95(6) - 3884.65
y = 11.7 - 3884.65
y = -3872.95
This gives us a negative price, which obviously does not make sense. It is likely that the price of a box of two pieces was not linearly increasing during this time period, or that there were other factors influencing the price. Therefore, we cannot use this equation to accurately predict the price of a box of two pieces on July 1, 1999.
Standard normal curve. Very confused

Answers
Each shaded area represents a probability. The first plot, for instance, represents the probability P (-z ≤ Z ≤ z) = 0.8064 for some number z that you have to find. To find these z, you'll need to be familiar with properties of the normal distribution as well as with lookup tables for z-scores or using inverse CDFs. (I'll provide a link in the comments to a calculator you can use.)
(a) The area under the curve between -z and z is 0.8064. This means the area outside this interval is 1 - 0.8064 = 0.1936. Because the distribution is symmetric about its mean (0 for the standard normal distribution), you know that the area under the curve to either side of the shaded region is half of this, or 0.0968. This area corresponds to the probability P (Z ≤ -z).
So you have
P (-z ≤ Z ≤ z) = P (Z ≤ z) - P (Z ≤ -z) … … … (a property of continuous distributions)
0.8064 = P (Z ≤ z) - 0.0968 ==> P (Z ≤ z) = 0.9032
and looking up the z-score, you would find z = 1.3.
(b) Because this distribution is symmetric about the mean, the mean is the same as the median, which is the number z such that P (Z ≤ z) = 0.5. In other words, half of the entire distribution falls to either side of the mean.
We use this fact to write
P (0 ≤ Z ≤ z) = P (Z ≤ z) - P (Z ≤ 0) = P (Z ≤ z) - 0.5 = 0.4452
==> P (Z ≤ z) = 0.9452
==> z ≈ 1.6000
(c) Keep in mind that the CDF is defined as P (Z ≤ z), so in order to find a probability like P (Z ≥ z), you have to take the complement:
P (Z ≥ z) = 1 - P (Z ≤ z) = 0.1711 ==> P (Z ≤ z) = 0.8289
==> z ≈ 0.9498
(d) Same reasoning as in (c):
P (Z ≥ z) = 1 - P (Z ≤ z) = 0.9918 ==> P (Z ≤ z) = 0.0082
==> z ≈ -2.3999
(e) Much more straightforward:
P (Z ≤ z) = 0.9115 ==> z ≈ 1.3501
Sandy is working as a waitress and wants to average
over $90 a night in tips. She works 4 days a week. So
far, she has made $95, $87, and $88 this week. How
much must she make on her last day of work to meet
her goal?
Answers
what is the distance of 53 yards square
Answers
Answer:
477 square foot
Step-by-step explanation:
cause yea
write a cube root function that has been horizontally dilated by 2 and shifted up 3 and left 8 with a reflection over the x-axis
Answers
The cube root function f(x) = ∛x will transform by given transformation rule is -∛(x - 8)/2 - 3.
What is the transformation of a graph?Transformation is rearranging a graph by a given rule it could be either increment of coordinate or decrement or reflection.
Reflection is a mirror image of a graph about any axis.
Let's consider a cube root function,
f(x) = ∛x
Horizontal dilated by 2 →
f(x) / 2 → ∛x/2
3 units up →
⇒∛x/2 + 3
8 units left →
⇒ ∛(x-8)/2 + 3
Reflection about the x-axis will change the sign of function but the value will remain the same.
⇒ - { ∛(x-8)/2 + 3 }
⇒ -∛(x - 8)/2 - 3
Hence "The cube root function f(x) = ∛x will transform by given transformation rule is -∛(x - 8)/2 - 3".
To learn more about the transformation of graphs,
brainly.com/question/3099136
#SPJ1
The perimeters of the triangles shown are equal. Find the side lengths of each triangle.
AB = PQ=
BC= QR=
AC= PR=

Answers
Answer:
Perimeter of both triangles is equal to 21
Step-by-step explanation:
By remembering our triangle facts, we know that the perimeter of a triangle is equal to its lengths added together.
By adding the lengths of triangle ABC we get...
2x + 5 + 2x + 4 + 2x
When we rearrange and add these together we get...
6x + 9
By adding the lengths of triangle PQR we get...
2x + 2x + 3x + 7
When we rearrange and add these together we get...
7x + 7
Because both triangles perimeters are equal we can say that...
6x + 9 = 7x + 7
Now lets solve.
-7 from both sides so we are left with
6x + 2 = 7x
From here we should be able to recognise that by adding our 2 we are just adding 1 lot of x.
Therefore, x = 2.
Use x = 2 solve the perimeter for each triangle to confirm.
What is identity matrix with example.
Answers
Answer:
An identity matrix is a square matrix having 1s on the main diagonal, and 0s everywhere else. For example, the 2×2 and 3×3 identity matrices are shown below. These are called identity matrices because, when you multiply them with a compatible matrix , you get back the same matrix
Step-by-step explanation:
mark as brainliest pls :)Answer:
An identity matrix is a square matrix having 1s on the main diagonal, and 0s everywhere else. For example, the 2×2 and 3×3 identity matrices are shown below. These are called identity matrices because, when you multiply them with a compatible matrix , you get back the same matrix.
Identity Matrix is the matrix which is n × n square matrix where the diagonal consist of ones and the other elements are all zeros. It is also called as a Unit Matrix or Elementary matrix. It is represented as In or just by I, where n represents the size of the square matrix. Identity Matrix is denoted with the letter “In×n”, where n×n represents the order of the matrix.
One of the important properties of identity matrix is: A×In×n = A, where A is any square matrix of order n×n.
Hope this helped
Use the relationship represented in this Venn diagram to identify the true statement.
A. All trapezoids are rhombuses
B. All rhombuses are trapezoids
C. No trapezoids are rhombuses
D. No rhombuses are trapezoids
Answers
The true statement based on the relationship depicted in the Venn diagram is that no rhombuses are trapezoids (option D).
To identify the true statement using the relationship represented in the Venn diagram, we need to analyze the overlapping regions and the properties of the shapes involved.
A trapezoid is a quadrilateral with at least one pair of parallel sides, while a rhombus is a quadrilateral with all sides of equal length. Let's evaluate the options:
A. All trapezoids are rhombuses: This statement is not true based on the diagram. The overlapping region between the trapezoids and rhombuses shows that there are trapezoids that are not rhombuses. Therefore, option A is incorrect.
B. All rhombuses are trapezoids: This statement is true based on the diagram. The entire region representing rhombuses is also included within the region representing trapezoids. Every rhombus can be considered a trapezoid because it has at least one pair of parallel sides. Thus, option B is correct.
C. No trapezoids are rhombuses: This statement is not true based on the diagram. The overlapping region indicates that there are trapezoids that are indeed rhombuses. Therefore, option C is incorrect.
D. No rhombuses are trapezoids: This statement is true based on the diagram. There is no overlap between the regions representing rhombuses and trapezoids, implying that no rhombus can be considered a trapezoid. Hence, option D is correct.
for such more question on trapezoids
https://brainly.com/question/22351006
#SPJ8
What is the greatest common factor (GCF) of 64 and 48?
Answers
Answer:
16
Step-by-step explanation:
it is the greatest common factor.
Answer:
The answer should be 16 because you can do 16x3 and get 48 and also do 16x4 to get 64
Hope that helps
The Baker's monthly grocery bill has been growing. In January the bill was $340, in February $346, in March $354, and in April the bill was $364. If this pattern continues, what will it be in May?
Answers
Answer:
$376
Step-by-step explanation:
first we identify the pattern so we can calculate the answer.
to do this we take away first bill from second bill. And take away second bill from third bill. And take away third bill from fourth bill.
$346-$340 = $6
The second bill grew by $6
$354-$346= $8
The third bill grew by $8
$364-$354=$10
The forth bill grew by $10
We can work out that the bill grows by $2 + previus increase.
So the bill in May will be $376 because $364+$10+$2 = $376
Two sides of a triangle have lengths 18m and 23m. Describe the possible length of the thired side
Answers
The possible length of the third side will be values that are less than 41 and greater than 5
Triangular theoremFor three sides to be the sides of a triangle, the sum of any two sides of the triangle must be greater than the third.
Given the two sides of a triangle have lengths 18m and 23m, the sum of the sides is given as:
sum = 18 + 23
Sum = 41
Let the third side be x, such that;
x + 18 >23
23 + x > 18
x > 23 - 18
x > 5
Similarly
x > -5
Hence the possible length of the third side will be values that are less than 41 and greater than 5
Learn more on side of triangle here: https://brainly.com/question/12240062
#SPJ1
Multiply. -8. -9/3 . 2/-5Write your answer in simplest form.
Answers
To multiply these numbers, the first step is to writhe "-8" as an improper fraction, to do so, divide it by 1
\((-\frac{8}{1})\cdot(-\frac{9}{3})\cdot(-\frac{2}{5})\)Next is to solve the multiplication, to do so, first multiply the first two terms of the multiplication:
\((-\frac{8}{1})\cdot(-\frac{9}{3})\)The multiplication is between two negative numbers, when you multiply two negative numbers, the minus signs cancel each other and turn into a positive value, this is called "double-negative"
\((-\frac{8}{1})\cdot(-\frac{9}{3})=\frac{8\cdot9}{1\cdot3}=\frac{72}{3}\)Next multiply the result by the third fraction -2/5
This time you are multiplying a positive and a negative number, so the result of the calculation will be negative
\(\frac{72}{3}\cdot(-\frac{2}{5})=-\frac{72\cdot2}{3\cdot5}=-\frac{144}{15}\)Final step is to simplify the result, both 144 and 15 are divisible by 3, so divide the numerator and denominator by 3 to simplify the result to the simplest form:
\(-\frac{144\div3}{15\div3}=-\frac{48}{5}\)Which of the following is the correct graph of the solution to the inequality -13> -5x+2> -28?

Answers
Answer:
first option
Step-by-step explanation:
solving the inequality
- 13 > - 5x + 2 > - 28 ( subtract 2 from each interval )
- 15 > - 5x > - 30
divide each interval by - 5 , reversing the symbols as a result of dividing by a negative quantity.
3 < x < 6
since the symbol is < then end values will be an open circle with the values between shaded blue, that is the first graph
What is the reference angle for the angle with measure −π4?
A 7π4
B π
C π4
D −7π4
Answers
Answer:
What is the reference angle for the angle with measure −π/4?
Answer: n/4
pie/4
Step-by-step explanation:
I just took the test lol the other answer is wrong.
Answer:
π/4
I took the quiz
Buddy and Michael get into a
snow fight with some boys on the
way home from school. It seems
as though Buddy can throw 4
times as many snowballs as the
boys can. If Buddy throws 1200
snowballs, how many did the boys
throw? Write an equation and
solve.
Answers
Answer:
1500
Step-by-step explanation:
1200/4=300
1200+300=1500
Answer:
This means the boys throw 300 snowballs while Buddy throws 1200 snowballs
Step-by-step explanation:
Equations
Let's call
x = number of snowballs the other boys throw.
4x = number of snowballs Buddy throws.
We know Buddy throws 1200 snowballs, thus
4x = 1200
Divide by 4:
x = 1200/4
x = 300
This means the boys throw 300 snowballs while Buddy throws 1200 snowballs
turning point of y=(x+3)(x-7)
Answers
Explanation:
Let's find the roots aka x intercepts.
Plug in y = 0 and solve for x.
y=(x+3)(x-7)
(x+3)(x-7) = 0
x+3 = 0 or x-7 = 0
x = -3 or x = 7 are the two roots.
Now find the midpoint of those roots.
Add them up and divide in half.
(-3+7)/2 = 4/2 = 2
The midpoint happens at x = 2, which is the x coordinate of the vertex. This is the axis of symmetry where the vertex is located.
The vertex in this case is the lowest point on the parabola. It's the turning point where it goes from decreasing to increasing.
Plug x = 2 back into the original equation to find its paired y value.
y=(x+3)(x-7)
y=(2+3)(2-7)
y=(5)(-5)
y=-25
Therefore, the vertex is located at (2, -25) as indicated in the graph below. I used GeoGebra to make the graph. Desmos is another good choice.

(Diversifying Portfolios MC)
Two investment portfolios are shown with the amount of money placed in each investment and the ROR.
Investment
Tech Company Stock $2,800
Government Bond $3,200
Junk Bond
$950
Common Stock
$1,500
Portfolio 1 Portfolio 2
$1,275
$2,200
$865
$1,700
O Portfolio 2 earns $38.17 more.
Which portfolio earns the most, and by how much?
O Portfolio 1 earns $38.17 more.
O Portfolio 1 earns $76.20 more.
ROR
-3.75%
2.95%
4.56%
O Portfolio 2 earns $76.20 more..
7.18%
Answers
Answer: Portfolio 1:
Tech Company Stock: $2,800 * 2.95% = $82.60
Government Bond: $3,200 * 4.56% = $145.92
Total return for Portfolio 1 = $82.60 + $145.92 = $228.52
Portfolio 2:
Junk Bond: $950 * (-3.75%) = -$35.63
Common Stock: $1,500 * 7.18% = $107.70
Total return for Portfolio 2 = -$35.63 + $107.70 = $72.07
From the calculations, we can see that Portfolio 1 earns $228.52 in returns, while Portfolio 2 earns $72.07. Therefore, Portfolio 1 earns $228.52 - $72.07 = $156.45 more than Portfolio 2.
Step-by-step explanation:
The figures below are rectangles. What polynomial in standard form represents the area of the shaded region?

Answers
Answer:
A
Step-by-step explanation:
(x+5)(x-1)-(x+1)(x-4)=7x-1