Write an appropriate and interesting word for 15000 and 3000.solve it
Answers
An appropriate and interesting word for 15,000 is "fifteen thousand," while for 3,000, it is "three thousand."
To solve this question, we need to write the numbers 15,000 and 3,000 as words. To do this, first, we look at the place values of each digit. In 15,000, the "15" is in the thousands place, so we write it as "fifteen thousand." Similarly, in 3,000, the "3" is in the thousands place, so we write it as "three thousand."
By doing this, we have expressed the numbers using their word forms, which helps in understanding and communicating numerical values more effectively, especially in written or spoken contexts. Remember, when writing large numbers as words, we use the place values to guide us in expressing them accurately and understandably.
To know more about numerical values click on below link:
https://brainly.com/question/13085451#
#SPJ11
Related Questions
There are 5 red markers, 2 orange markers, 3 yellow markers, and 5 green markers in a desk drawer.What is the probability of choosing a red marker?
Answers
Answer:
the probability is 5/12 or 0.416 or 41%
Step-by-step explanation:
Hope I helped!
what is the result of the following expression? 10 + 5 * 3 - 20
Answers
prove that cos theta by 1 minus tan theta + sin theta by 1 minus cot theta equal to sin theta + cos theta
Answers
Here is the answer for prove that cos theta by 1 minus tan theta + sin theta by 1 minus cot theta equal to sin theta + cos theta

the question is on the picture

Answers
Answer:
B
Step-by-step explanation:
Volume of a triangular pyramid = \(\frac{1}{3} bh\)
where b = area of base and h = height
We are already given that the height is 17in
All we need to do is find the area of the base.
The base is a triangle with a base length of 12 in and a height of 10 in
To find the area of a triangle we multiply the base length by the height then divide by 2
so area = (12 * 10 ) / 2
12 * 10 = 120
120 / 2 = 60
Hence the area of the base is 60in²
Now we plug in the values into the formula for volume of a pyramid
\(V=\frac{1}{3} 60*17\\\frac{1}{3} 60=20\\20*17=340\)
V = 340
Hence the answer is B
Factor the polynomial expression 8x^3-1
Answers
Answer:
Step-by-step explanation:

A cylindrical tin filled with oil has a diameter of 12cm and a height of 14cm. The oil is then poured in rectangular tin 16cm long and 11cm wide. What is the depth of the oil in the tin
Answers
The volume of cylindrical tin is 1584 \(cm^3\). The depth of the oil in the tin is 9cm.
\(V_1 =\) VOLUME OF CYLINDRICAL TIN
\(= \pi r^2 h\)
\(=\frac{22}{7}\) x 6 x 6 x 14
= 44 x 36
= 1584 \(cm^3\)
\(V_2 =\) VOLUME OF RECTANGULAR TIN
= lbh = 1584
= (16)(11)(h) = 1584
= 176h =1584
= h = 1584 / 176
= h = 9 cm
A cylinder is a three-dimensional shape that consists of a circular base and a curved surface that extends upward to meet at a point known as the apex. The volume of a cylinder is the amount of space occupied by the shape and is given by the formula V = πr²h, Once we have calculated the area of the circular base, we can multiply it by the height of the cylinder to get the volume.
To calculate the volume of a cylinder, we need to know its dimensions, which are the radius and height. The radius is the distance from the center of the circular base to the edge, while the height is the distance between the two circular bases.
To learn more about Volume of cylindrical visit here:
brainly.com/question/30981845
#SPJ4
A sample of 4 different calculators is randomly selected from a group containing 13 that are defective and 39 that have no defects. What is the probability that at least one of the calculators is defective? Show your answer in decimal format to three places (0.000).
Answers
The probability that at least one of the calculators is defective is approximately 0.522.
First, we need to find the probability that none of the calculators are defective.
The probability that the first calculator selected is not defective is 39/52. The probability that the second calculator selected is also not defective is 38/51. The probability that the third calculator selected is not defective is 37/50. And finally, the probability that the fourth calculator selected is also not defective is 36/49.
So, the probability that all four calculators are not defective is:
(39/52) * (38/51) * (37/50) * (36/49) ≈ 0.478
Therefore, the probability that at least one of the calculators is defective is:
1 - 0.478 ≈ 0.522
So, the probability that at least one of the calculators is defective is approximately 0.522.
Learn more about probability here
https://brainly.com/question/13604758
#SPJ11
I have 45 questions and i need help

Answers
the awaer is -5 see if that is right step by step
The slope of a line is 1/2 and passes the point (2, -5)find the equation
Answers
THE GENERAL EQUATION OF A STRAIGHT LINE IS y=mx+c
TO FIND c I WILL SUBSTITUTE THE GIVEN KNOWN POINTS.
\( - 5= \frac{1}{2} (2) + c \\ - 5= 1 + c \\ c = - 5 - 1 \\ c = - 6\)
THEREFORE WE NOW HAVE
\( y= \frac{1}{2} x - 6\)
ATTACHED IS THE SOLUTION
During one week, Sheila made
several changes to her bank account.
She made three withdrawals of $30
each from an ATM. She also used
her check card for a $156 purchase.
Then she deposited her paycheck of
$325.
By how much did the amount in her
bank account change during that
week?
Answers
$59 increase in her bank account during that week.
What is algebraic operation ?Any of the basic arithmetic operations—addition, subtraction, multiplication, division, raising to the power of a whole number, and taking roots (fractional power)—is considered an algebraic operation. These procedures could be applied to numerical data, in which case they are frequently referred to as arithmetic operations. Similar operations can be carried out on variables, algebraic expressions, and, more generally, on components of algebraic structures like groups and fields. Another way to describe an algebraic operation is as a function from the Cartesian power of a set to the same set.
Given that : Sheila made four withdrawals of each from an ATM. . She also made a $156 purchase with her check card. She then deposited her $375 salary.
It's difficult to determine the solution because we don't know how much money she had in her bank account before making 4 withdrawals, thus a like and rating would be really appreciated. We know she took out $40 four times, which is $160. She also used her card to make a $156 purchase, so when we sum the two amounts, we get $316. She then placed a check for $375 into the bank account, therefore we must deduct $316 from that sum to get at $59; but, since we deducted, Given that we end up with $59 rather than the money we wasted at the beginning, the fact that the price is higher than $316 indicates that there has been an increase.
To learn more about algebraic operation visits:
brainly.com/question/12501646
#SPJ9
Find the inverse of the function y = 3x - 4
Answers
Write the sum of 7√28x6+4√7x6 in simplest form, if x≠0
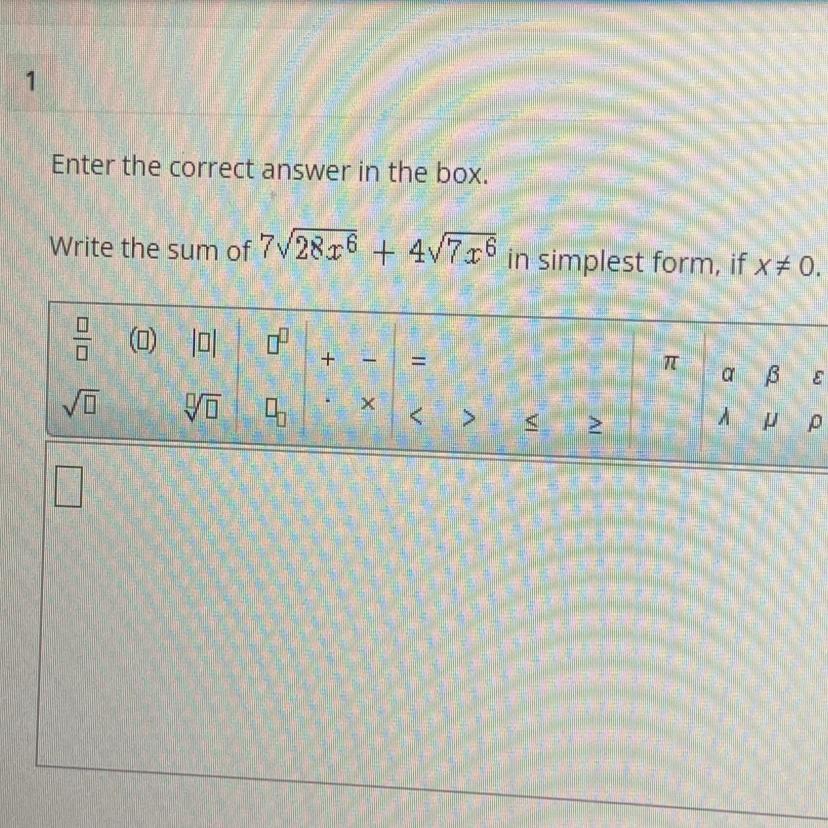
Answers
Answer:
0
Step-by-step explanation:
7√2(8)(06)+4√7(06)
7√2(8)(06)+4√7(06)
=0
A sample of 250 observations is selected at random from a n infinite population. Given that the population proportion is 0.25, the standard error of the sampling distribution of the sample proportion will be:
Answers
When a sample of 250 observations is selected at random from an infinite population with a population proportion of 0.25, we can calculate the standard error of the sampling distribution of the sample proportion using a specific formula. The standard error (SE) measures the variability or dispersion of the sample proportion from the true population proportion.
To compute the standard error of the sample proportion, use the following formula:
SE = sqrt[(P * (1 - P)) / n]
where P is the population proportion (0.25 in this case) and n is the sample size (250).
SE = sqrt[(0.25 * (1 - 0.25)) / 250]
SE = sqrt[(0.25 * 0.75) / 250]
SE = sqrt[0.1875 / 250]
SE ≈ 0.027
Thus, the standard error of the sampling distribution of the sample proportion, given a population proportion of 0.25 and a sample size of 250, is approximately 0.027. This value represents the expected variation in the sample proportions from the true population proportion, providing an estimate of the precision of the sample proportion as a representation of the population proportion.
To know more about standard error visit:
https://brainly.com/question/13179711
#SPJ11
Find an equation of the line that satisfies the given conditions.Through (−9, −11); perpendicular to the line passing through (−6, 1) and (−2, −1)
Answers
The equation of the line passing through (9,11) and perpendicular to the line passing through (6,1) and (2,1) is x = 6
Find the slope of the line passing through (6,1) and (2,1):
The slope of a line passing through two points (x1,y1) and (x2,y2) is given by:
slope = (y2 - y1) / (x2 - x1)
Substituting the given coordinates, we get:
slope = (1 - 1) / (2 - 6) = 0
Therefore, the slope of the line passing through (6,1) and (2,1) is 0.
Find the slope of the line perpendicular to the line passing through (6,1) and (2,1):
The slope of a line perpendicular to a line with slope m is given by:
perpendicular slope = -1/m
Substituting the slope of the line passing through (6,1) and (2,1), we get:
perpendicular slope = -1/0 (which is undefined)
This means that the line perpendicular to the line passing through (6,1) and (2,1) is a vertical line passing through the point (6,1) and (2,1).
Write the equation of the line passing through (9,11) and perpendicular to the line passing through (6,1) and (2,1):
Since the line passing through (6,1) and (2,1) is a horizontal line with equation y = 1, the line perpendicular to it is a vertical line passing through the point (6,1) and (2,1). The equation of a vertical line passing through a point (a,b) is given by:
x = a
Substituting the value of x = 6 (since the vertical line passes through (6,1) and (2,1)), we get:
x = 6
Learn more about slope of the line here
brainly.com/question/16180119
#SPJ4
Does anyone know what point a would equal???

Answers
The values of a, b, c, and d in the parallelogram are:
a = -2
b = 10
c = 4
d = 2
To determine the values of a, b, c, and d in the parallelogram, we can use the properties of parallelograms.
Since opposite sides of a parallelogram are parallel, the y-coordinate of the point opposite (4, 10) should also be 10.
Then, we have:
Point (a, 10) and Point (c, d)
The x-coordinate of the opposite point is the same for both pairs of opposite sides. So we can set:
a = -2
c = 4
Now, we need to find the value of d. Since the opposite sides of a parallelogram are also equal in length, we can use the y-coordinate of another given point, (-2, 2), to find d.
d = 2
So the values of a, b, c, and d in the parallelogram are:
a = -2
b = 10
c = 4
d = 2
Learn more about Coordinates here:
https://brainly.com/question/22261383
#SPJ1
How many favorable outcomes are expressed in the fraction 12/50?
Answers
Mast 1 broadcast radio signals that can be heard within a radius of 15km.
Mast 2 broadcast radio signals that can be hear within a radius of 20km.
Answers
The difference in the areas of the signals based on the information given is 549.5km².
How to calculate the area?The formula to calculate the area of a circle will be:
= πr²
where r = radius
Mast 1 broadcast radio signals that can be heard within a radius of 15km. The area will be:
= πr²
= 3.14 × 15²
= 706.5km²
Mast 2 broadcast radio signals that can be hear within a radius of 20km. The area will be:
= πr²
= 3.14 × 20²
= 1256km²
The difference in the areas will be:
= 1256km² - 706.5km²
= 549.5km²
Learn more about area on:
brainly.com/question/25292087
#SPJ1
Complete question
Mast 1 broadcast radio signals that can be heard within a radius of 15km.
Mast 2 broadcast radio signals that can be hear within a radius of 20km.
Calculate the difference in the areas of the signals.
Two sides of an obtuse triangle measure 12 inches and 14 inches. The longest side measures 14 inches.
What is the greatest possible whole-number length of the unknown side?
2 inches
3 inches
7 inches
9 inches
Answers
Answer:
c
Step-by-step explanation:
The greatest possible whole-number length of the unknown side among the option is 9 inches.
Obtuse triangle
Obtuse triangle is a triangle that has one of it internal angle greater than 90 degrees.
Triangle inequality theorem:The sum of the lengths of any two sides of a triangle is greater than the length of the third side.
If the sides of the triangles are x, y and z. Therefore,
x + y > z
x + z > y
y + z > x
Therefore, the greatest possible length among the option is 9.
Proof
12 + 14 > 9
12 + 9 > 14
14 + 9 > 12
learn more on triangle here: https://brainly.com/question/10373668
Compare the two groups of data. which statement is true?

Answers
The true statement is the range of Mrs Plum's class is 14 greater than the range of Mr Scarlet's class.
What is the range?A box plot is a graph that is used to describe a dataset. A box plot is made up of two lines and a box. The two lines are known as whiskers.
The end of the first whisker represents the minimum number and the end of the second whisker represents the maximum number. The difference between the whiskers is the range.
Range is used to measure the variation of a dataset. It is the difference between the maximum number and the minimum number.
Range = maximum number - minimum number
Range of Miss Scarlet's class = 98 - 65 = 33
Range of Miss Plum's class = 98 - 51 = 47
Difference in range = 47 - 33 = 14
To learn more about box plots, please check: https://brainly.com/question/27215146
#SPJ1
Indicate below whether the equation in the box is true or false 4/10=1/2

Answers
Answer:
False
Step-by-step explanation:
4/10's numerator (top number) and denominator (bottom number) can both be divided by 2. Therefore, when we do that, it will be equal to 2/5. 2/5 is not equal to 1/2. Therefore, the answer is false.
If this helps please mark as brainliest
Can someone help me with this question plssssssss brainly

Answers
Answer:
C) -x²+9x²-27+27
Hope it helps
:)
if there are 10 people per race, and 2222 people have come in first place, then how many races have happened?
Answers
Answer:
22,220
Step-by-step explanation:
Because you multiply by 10 because 2222 times 1 is 1 person per race, so if there is 10 then you multiply by 10.
EASY MATH!!! GIVING BRAINLIEST

Answers
Answer:
it might be 30. If you lose half every 90 days, You lose half of 120, which is 60. another 45 days would make it half again, equaling 30
Answer: 30
Step-by-step explanation:
Express the perimeter of the triangle as a polynomial.

Answers
Answer:
perimeter=11x-2Step-by-step explanation:
Given in the ∆ that,
the sides of triangle∆ is
5x-22x-34x+3As we know that,
perimeter of the ∆ is the addition of all sides
so,
perimeter= 5x-2+2x-3+4x+3
=11x-2
When entering large numbers in the answer box, do not use commas. For example, enter 1276400 for the number 1,276,400. Do not enter 1,276,400. If you accidentally enter commas, you will receive feedback as a reminder. Answer the following question by typing the numeric answer into the answer box. What is the sum of 9260 and 3240?.
Answers
Answer:
12500
Step-by-step explanation:
9260+3240==
12500
PLSSSS HELP IF YOU TULRY KNOW THISSS

Answers
Answer:
Step-by-step explanation:
Rearrange terms: -3x+4=6-3x
-3x+4=-3x+6
Subtract 4 from both sides
-3+4-4=-3x+6-4
Subtract the numbers
=3x==3x+2
Add 3x to both sides
=3x+3x=-3x+2+3x
-3x+3x=-3x+2+3x= 0=2 which is no solution
Which of the following is the radical expression of 2 times d to the seven tenths power?
Answers
Answer:
2d^0.7
Step-by-step explanation:
Wanda and Ted work together at a convenience store and are paid the same hourly rate. If Wanda works 22 hours and is paid $165 before taxes, and Ted works 13 hours and is paid a pre-tax amount of $97.50, what is their hourly salary
Answers
For Wanda:
Hourly salary = Total pre-tax earnings / Number of hours worked
Hourly salary = $165 / 22 hours
Hourly salary = $7.50/hour
For Ted:
Hourly salary = Total pre-tax earnings / Number of hours worked
Hourly salary = $97.50 / 13 hours
Hourly salary = $7.50/hour
Therefore, both Wanda and Ted have an hourly salary of $7.50.
rather than a t test whenever the null hypothesis makes a claim about
Answers
Instead of using a t-test, alternative statistical tests can be employed when the null hypothesis makes specific claims about the data. These tests are chosen based on the type of data and the specific hypothesis being tested.
When the null hypothesis makes claims about the data that go beyond a simple comparison of means, using a t-test may not be appropriate. Alternative statistical tests can be used in such situations to address specific hypotheses.
For example, if the null hypothesis involves comparing proportions, a chi-square test or Fisher's exact test can be used. These tests are suitable for analyzing categorical data and determining if there is a significant difference between observed and expected frequencies.
Similarly, if the null hypothesis involves comparing means across more than two groups, analysis of variance (ANOVA) or its non-parametric counterpart, the Kruskal-Wallis test, can be employed. These tests allow for the comparison of means across multiple groups and can provide valuable information about the overall differences between groups.
The selection of an appropriate test depends on various factors. The nature of the variables being analyzed, such as categorical or continuous, influences the choice of test. Sample size also plays a role, as some tests require larger sample sizes to yield accurate results. Additionally, each test has certain assumptions associated with it, and researchers need to ensure these assumptions are met before applying the test.
By using alternative tests that are suitable for the specific hypotheses being tested, researchers can gain a deeper understanding of their data. This approach allows for more accurate statistical inferences and can provide valuable insights into the relationships and differences present in the dataset.
To learn more about ANOVA click here, brainly.com/question/31809956
#SPJ11
Solve Eq. 7.5 and Eq. 7.6 ( 2 equations with 2 unknowns) for v1 in terms of: m,M,g,h. (20 pts) mv1=(m+M)V2(Eq.7.5)21(m+M)V22=(m+M)gh(Eq.7.6) 6. You shoot a ball, m=50.0 g, into a catcher, M=200.0 g, the center of mass rises 15.0 cm. Calculate vi. Refer to your answer for Question 5.
Answers
The initial velocity (vi) of the ball, when shot into the catcher, is approximately 367.5 m/s.
To solve Eq. 7.5 and Eq. 7.6 for v1 in terms of m, M, g, and h, we will substitute the given values of m, M, and h into the equations.
Eq. 7.5: mv1 = (m+M)V2
Eq. 7.6: (m+M)V22 = (m+M)gh
Given:
m = 50.0 g (mass of the ball)
M = 200.0 g (mass of the catcher)
h = 15.0 cm (rise in the center of mass)
First, let's solve Eq. 7.6 for V2 by dividing both sides by (m+M):
V22 = gh
Next, substitute the expression for V2 into Eq. 7.5:
mv1 = (m+M)(gh)
Now, solve for v1 by dividing both sides by m:
v1 = (m+M)(gh) / m
Substituting the given values:
v1 = (50.0 g + 200.0 g)(9.8 m/s²)(0.15 m) / (50.0 g)
Calculating the expression:
v1 = (250.0 g)(9.8 m/s²)(0.15 m) / (50.0 g)
v1 = 367.5 m/s
Therefore, the initial velocity (vi) of the ball, when shot into the catcher, is approximately 367.5 m/s.
Learn more about velocity here
https://brainly.com/question/25749514
#SPJ11