Winona has two jobs; one pays $10.00 per hour and the other pays $9.25 per hour plus an average of 70% of the hourly pay in tips and bonuses. Each pay
period, 20% of her total pay goes to taxes.
Part 1 of 2
(a) Write and simplify an equation that describes Winona's total take-home pay, P, in terms of the number of hours, x, worked at the first job and the
number of hours, y, worked at the second.
The total take-home pay from Winona's two jobs can be found using the equation
P=
Part 2 of 2
X
3
(b) If Winona is committed to work 30 hours per week at the first job, how many hours per week would she need to work at the second job if she needs
her biweekly take-home pay to be $800? Round the answer to one decimal place.
In order for her biweekly take-home pay to be $800, Winona needs to work
hours per week at her second job.
Answers
Answer:
76.5 hours
(If you like this answer i would appreciate if u give brainliest but otherwise, i hope this helped ^^)
Step-by-step explanation:
Part 1 of 2:
(a) The equation that describes Winona's total take-home pay, P, in terms of the number of hours, x, worked at the first job and the number of hours, y, worked at the second job can be written as:
P = (10x) + (9.25y + 0.7 * 9.25y) - 0.2[(10x) + (9.25y + 0.7 * 9.25y)]
Simplifying the equation:
P = 10x + 9.25y + 0.7 * 9.25y - 0.2(10x + 9.25y + 0.7 * 9.25y)
Part 2 of 2:
(b) If Winona is committed to working 30 hours per week at the first job, let's assume x = 30. We need to find the number of hours per week, y, she would need to work at the second job to reach a biweekly take-home pay of $800.
Using the simplified equation from part (a), we substitute x = 30 and solve for y:
800 = (10 * 30) + (9.25y + 0.7 * 9.25y) - 0.2[(10 * 30) + (9.25y + 0.7 * 9.25y)]
800 = 300 + (9.25y + 0.7 * 9.25y) - 0.2(300 + (9.25y + 0.7 * 9.25y))
Now, we can solve for y:
800 = 300 + 9.25y + 0.7 * 9.25y - 0.2(300 + 9.25y + 0.7 * 9.25y)
Simplify the equation:
800 = 300 + 9.25y + 0.7 * 9.25y - 0.2 * 300 - 0.2 * 9.25y - 0.2 * 0.7 * 9.25y
Combine like terms:
800 = 300 - 60 + 9.25y - 1.85y - 0.1295y
800 = 240 + 7.32y
Subtract 240 from both sides:
560 = 7.32y
Divide both sides by 7.32:
y ≈ 76.5
Therefore, Winona would need to work approximately 76.5 hours per week at her second job to reach a biweekly take-home pay of $800.
Related Questions
Multiply 2x(5x+8y+3)
Answers
Answer:
10x² + 16xy + 6x
Step-by-step explanation:
2x(5x+8y+3)
10x² + 16xy + 6x
Answer:
\(10x^{2} + 16xy + 6x\)
Hope this helped!
Calculate a high estimate for each. Show your work?81×37
Answers
Step-by-step explanation:
2997
81
×
37
=2997
it just a simple calculation just multiply the numbers
If 2a + 1=3a, then 5a=?
Answers
Answer:
5a =5
Step-by-step explanation:
Zach learns the same amount of money each week doing yard work. If he earns $36
at the end of 4 weeks, how much money does Zachlearn each week?
Answers
Answer:
$9 per week
Step-by-step explanation:
36/4 = 9
The ratio of length to width in a rectangle is 2:3. Find the length of the rectangle when area is 150in^3.
Show a quadratic model
Answers
Answer:
\(\fbox{length= 10 \: in}
\)
Step-by-step explanation:
Given:
Length to Width Ratio = 2:3
Area of Rectangle= 150 in²
To find:
Length of rectangle=?
Solution:
Assume the length of rectangle is 2x
and the width of rectangle is 3x
Now,
we know that,
\(\sf\: Area \: of \: Rectangle = Length \times Width \\ \sf \: 150 = 2x \: \times 3x \\ \sf \: 150 =6 {x}^{2} \\ \sf \: {x}^{2} = \frac{150}{6} \\ \sf {x}^{2} = \cancel\frac{150}{6} \\\sf {x}^{2} = 25 \\\sf \sqrt{ {x}^{2} } = \sqrt{ {25} } \\ \sf \sqrt{ {x}^{2} } = \sqrt{ {5}^{2} } \\ \sf \: \fbox{x = 5 \: in}\)
Substituting the value of x in assumption,
\( \sf \: length= 2x \\ \sf \: length= 2 \times 5 \\ \sf \: \fbox{length= 10 \: in}
\)
Thanks for joining brainly community!
Find the area of the regular hexagon:
(Just enter your numerical answer. Do not round!)
Area =
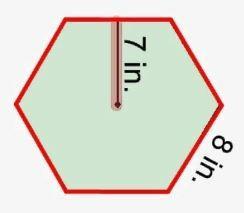
Answers
Answer:
168
Step-by-step explanation:
Area = 168
(Just typing since it needs at least 20 letters to post)
Given A = {10, 11, 12, 13}, B = {10, 12, 14, 16}, and C = {7, 8, 9, 10, 11}, find
A ∪ B
A ∩ B
A ∪ C
A ∩ C
B ∪ C
B ∩ C
Answers
The (A ∪ B) ∩ (A ∪ C) ∩ (B ∪ C) ∩ (B ∩ C) ∩ C is an empty set {}.To find the sets A ∪ B, A ∩ B, A ∪ C, A ∩ C, B ∪ C, and B ∩ C, we can perform the following operations:
A ∪ B: The union of sets A and B includes all unique elements from both sets, resulting in {10, 11, 12, 13, 14, 16}.
A ∩ B: The intersection of sets A and B includes only the common elements between the two sets, which are {10, 12}.
A ∪ C: The union of sets A and C combines all unique elements, resulting in {7, 8, 9, 10, 11, 12, 13}.
A ∩ C: The intersection of sets A and C includes only the common elements, which is {10, 11}.
B ∪ C: The union of sets B and C combines all unique elements, resulting in {7, 8, 9, 10, 11, 12, 14, 16}.
B ∩ C: The intersection of sets B and C includes only the common elements, which is an empty set {} since there are no common elements.
Finally, performing the remaining operations:
(A ∪ B) ∩ (A ∪ C): This is the intersection of the union of sets A and B with the union of sets A and C. The result is {10, 11, 12, 13} since these elements are common to both unions.
(B ∪ C) ∩ (B ∩ C): This is the intersection of the union of sets B and C with the intersection of sets B and C. Since the intersection of B and C is an empty set {}, the result is also an empty set {}.
For more such questions on empty set
https://brainly.com/question/1363314
#SPJ8
For the pair of functions, find the indicated sum, difference, product, or quotient. f(x) = x² + 1, g(x) = 9x + 5 Find (fg)(x).
Answers
Answer:The product of the two functions, f and g, is given by fg(x) = (x² + 1)(9x + 5). This can be expanded to give fg(x) = 9x³ + 14x² + 5x + 5.
Step-by-step explanation:
10. Higher Order Thinking Each of 5 friends has x action figures in
his or her collection. Each friend buys 11 more action figures. Now
the 5 friends have a total of 120 action figures.
a. Write an equation that models the problem.
b. Solve the equation to find the number of action figures, x, that
each friend had originally.
Answers
Each friend had originally 13 action figures before buying 11 more.
Given that there are 5 friends and each of them has x action figures in his or her collection. When they buy 11 more action figures, the total number of action figures they will have is 120.
a) We need to find an equation that models the problem. Let x be the original number of action figures that each friend had.
Therefore, the total number of action figures that each friend will have after purchasing 11 more is x + 11.
The total number of action figures will be 5(x + 11) = 5x + 55.
Now, according to the problem,5x + 55 = 120
This is our equation that models the problem.
b) We have to solve the equation 5x + 55 = 120 to find the original number of action figures, x, that each friend had before buying 11 more action figures.
5x + 55 = 120
5x = 120 - 55 (subtract 55 from both sides)
5x = 65x = 13
Therefore, each friend had originally 13 action figures before buying 11 more.
For more such questions on figures, click on:
https://brainly.com/question/24491627
#SPJ8
2x29(4x3)= what is the answer to this problem
Answers
Answer:
10,092
Step-by-step explanation:
841×12
A certain type of kickboard scooter comes in silver, red, 2
or purple with wheel sizes of 125 millimeters or 180
millimeters. Determine the total number of color-wheel size combinations.
(This is probability and I’m having such a hell of a time figuring it out pls help)
Answers
There are a total of 8 color-wheel size combinations for the kickboard scooter. This means that customers have 8 different options to choose from when selecting the color and wheel size for their scooter.
To determine the total number of color-wheel size combinations for the kickboard scooter, we need to multiply the number of color options by the number of wheel size options.
Given that there are 4 color options (silver, red, blue, and purple) and 2 wheel size options (125mm and 180mm), we can use the multiplication principle to find the total number of combinations:
Total combinations = Number of color options × Number of wheel size options
Total combinations = 4 colors × 2 wheel sizes
Total combinations = 8
There are a total of 8 color-wheel size combinations for the kickboard scooter. This means that customers have 8 different options to choose from when selecting the color and wheel size for their scooter.
For more such questions on combinations
https://brainly.com/question/28065038
#SPJ8
Which net represents this triangular prism?

Answers
Answer:
this one.............

Answer:
top right
Step-by-step explanation:
There are 3 types of PercentProblems.
a) Number compared to the Base is unknown: x/100 = ?/b
b) Base Number is unknown: a/?= x/100
c) Percent is unknown: a/b = ?/100
Solve the following percent problems using the appropriate percent equation. Describe
the steps used to calculate each answer.
1) What distance is 24% of 710 miles?
2) 62 hours is what percent of 3 days?
3) 16% of what number is 8?
Answers
Answer:A. x= .100
Step-by-step explanation:because of the distance in miles
Rewrite (10 + 6) + 5+ 3 using the Associative Law of Addition,
O 10+6+5+3
O 10+(6+5) + 3
O 3+5+ (10+6)
O (10+6) + 3+5
Answers
Rewriting (10 + 6) + 5+ 3 using the Associative Law of Addition will be 3+5+ (10+6).
How to compute the value?It should be noted that the Associative Law of Addition explains that a + (b + c) is the same as (a + b) + c.
Therefore, Rewriting (10 + 6) + 5+ 3 using the Associative Law of Addition will be 3+5+ (10+6).
It should be noted that this will still give same value.
Therefore, the correct option is B.
Learn more about addition on:
brainly.com/question/25421984
#SPJ1
The Venn diagram below represents the students in a particular high school.
How many female students have a part-time job and a driver's license?
Female
Part-time job
74
111
56
45
92
38
137
89
Driver's license
No

Answers
Answer:
C. 45
Step-by-step explanation:
Since the question is asking how many females have both a driver's license and a part-time job. So you need to find the number that reoresents all three of these categories. This category is shown in the very center with the number 45.
Hope it helps!
Answer:
C. 45
Step-by-step explanation:
Because its inside all three circles that you want.
Use Structure The area of a rectangular
playground has been extended on one side.
The total area of the playground, in square
meters, can be written as 352 +22x.
Rewrite the expression to give a possible set
of dimensions for the playground,
Answers
Answer:
The width and length of the rectangle are \(22\) and \(16+x\), for \(x > -16\), respectively.
Step-by-step explanation:
From Geometry, we remember that area of the rectangle (\(A\)) is defined by:
\(A = w\cdot l\) (1)
Where:
\(w\) - Width.
\(l\) - Length.
In addition, we know that area is described by a first-order polynomial:
\(A = 352+22\cdot x\) (2)
Meaning that is the product of another first-order polynomial and a constant. That is:
\(A = a\cdot (b+c\cdot x)\) (3)
Now we determine the Great Common Divisor of 352 and 22:
\(352 = 2\times 2\times 2 \times 2 \times 2 \times 11\)
\(22 = 2\times 11\)
The Great Common Divisor is 22.
Then, the area of the rectangle can be expressed by this expression:
\(A = 22\cdot (16+x)\) (3b)
According to this, the width and length of the rectangle are \(22\) and \(16+x\), for \(x > -16\), respectively.
Farmer Elentire started with 71 dubbles on his farm. Every month, he
got 6 more of them. Farmer Hopt started with 10 dubbles on her farm.
Every month, she purchased 10 more of them.
Write a system of equations, in slope-intercept form, to model each
farmer's dubbles (y) as months (x) increase.
y= 71+ 6x
y= 10+ 10x
What pair of numbers solves the system? Use values accurate to the
nearest tenth.
Answers
The pair of numbers that solves the system is (15.25, 164.5).
The system of equations, in slope-intercept form, for Farmer Elentire and Farmer Hopt are:
y = 6x + 71 (for Farmer Elentire)
y = 10x + 10 (for Farmer Hopt)
To find the pair of numbers that solves the system, we need to find the point where the two lines intersect.
We can do this by setting the equations equal to each other:
6x + 71 = 10x + 10
Solving for x, we get:
4x = 61
x = 15.25
Now that we know x = 15.25, we can plug this value into either equation to find y.
71 + 6x = 10 + 10x
Subtracting 6x and 10 from both sides, we get:
61 = 4x
Dividing both sides by 4, we get:
x = 15.25
Now we can use either of the original equations to find y. Let's use the first equation:
y = 71 + 6x = 71 + 6(15.25) ≈ 164.5
Using the first equation, we get:
y = 6(15.25) + 71
y = 163.5
Therefore,
The pair of numbers that solves the system is (15.25, 163.5), accurate to the nearest tenth.
For similar question on pair:
https://brainly.com/question/29122030
#SPJ11
PLEASE HELP ME
An article claimed the state's typical price of a gallon of gasoline is $2.69. The prices from 5 gas stations surveyed were $2.79, $2.99, $2.69, $2.89, and $2.69.
According to this data, what is wrong with the article's statement?
Answers
This statement in the article is wrong because $2.69 is not the mean price ($2.81)
How to determine what is wrong with the data?The prices from 5 gas stations surveyed are given as:
$2.79, $2.99, $2.69, $2.89, and $2.69.
There are no outliers in the dataset
So, the dataset can be summarized by the mean
The mean is
Mean = Sum/Count
So, we have
Mean = ($2.79+ $2.99+ $2.69+ $2.89+$2.69)/5
Evaluate
Mean = 2.81
The article quoted $2.69 as the typical price
This statement in the article is wrong because $2.69 is not the mean price ($2.81)
Read more about mean at:
https://brainly.com/question/20118982
#SPJ1
2. Thirty decreased by 6 times a number is the same as 16 more than the number. Find the number.
Answers
Answer:
x=2
Step-by-step explanation:
Let x be the number
30 - 6x = x+16
Add 6x to each side
30 -6x+6x = x+16+6x
30 = 7x+16
Subtract 16 from each side
30-16 = 7x+16-16
14 = 7x
Divide each side by 7
14/7 = 7x/7
2 =x
Answer:
2
Step-by-step explanation:
let the number be x
given, 30 decreased by 6 times the number=30-6x
that is same as 16 more than the number= x+16
equating these 2 equations since they are equal
30-6x=x+16
6x+x=30-16
7x=14
x=2
The req. number is 2
A used car dealer sells SUVs and cars. Of all the vehicles, 60% are cars. Of all the vehicles 15% are red cars. what is the probability that a car chosen at random is not a red car?
Answers
The probability that a car chosen at random is not a red car is 51%
How to determine the probability that a car chosen at random is not a red car?From the question, we have the following parameters that can be used in our computation:
Cars = 60%
Red cars = 15%
The probability that a car chosen at random is not a red car is calculated as
P = (1 - Red cars) * Cars
substitute the known values in the above equation, so, we have the following representation
P = (1 - 15%) * 60%
Evaluate
P = 51%
Hence, the probability that a car chosen at random is not a red car is 51%
Read more about probability at
https://brainly.com/question/31649379
#SPJ1
How do i write this quotient as a mixed number?

Answers
Answer:
I would write 8 3/4
right?
Answer:
8 3/4
Step-by-step explanation:
To write this as a mixed number you divide 35 by 4 to get 8 and if you out it in decimal form you get 8.75 so you take the remainder (75/100) and you simplify that to get 3/4 and after all of that you get 8 3/4. Hope this helps
labor-hours and its standard cost card per unit is as follows:
Direct material: $ pounds at $11.00 per pound
Direct labor: 3 hours at $12 per hour
Variable overhead: 3 hours at $7 per hour
Total standard variable cost per unit
The company also established the following cost formulas for its selling expenses:
sales salaries and commissions
shipping expenses
Fixed Cost per
Month
$ 280,000
$ 260,000
$ 55.00
36.00
$112.00
Variable
Cost per
Unit Sold
$ 20.00
$ 11.00
The planning budget for March was based on producing and selling 21,000 units. However, during March the company
actually produced and sold 26.600 units and incurred the following costs:
a Purchased 154.000 pounds of raw materials at a cost of $9.50 per pound. All of this material was used in production.
b. Direct laborers worked 63,000 hours at a rate of $13.00 per hour
e Total variable manufacturing overhead for the month was $510,930
d Total advertising sales salaries and commissions, and shipping expenses were $286,000, $495,000, and $195,000,
respectively
6 What direct labor cost would be included in the company's flexible budget for March?

Answers
The direct labor cost included in the Preble Company's flexible budget for March is $819,000.
How to compute Preble Company's direct labor cost?To find the direct labor cost included in the company's flexible budget for March, we shall estimate the actual direct labor cost incurred during the period.
Given:
Actual production and sales =n26,600 units
Actual direct labor rate = $13.00 per hour
Actual direct labor hours worked = 63,000 hours
Direct labor cost = Actual direct labor rate × Actual direct labor hours worked
Direct labor cost = $13.00/hour × 63,000 hours
Direct labor cost = $819,000
Hence, the direct labor cost included in the company's flexible budget for March would be $819,000.
Learn more about direct labor cost at brainly.com/question/29390449
#SPJ1
A tower on a college campus was built with a faulty foundation and is starting to lean. A student climbs to the tilted top and drops a rope down to the ground. The
end of the rope lands 3 feet from the base of the tower and measures 57 feet from
the top of the building to the ground. What is the angle that the tower is leaning?
Round your answer to the nearest degree, and enter the number only
Answers
Answer:
leaning at 3 degrees off of normal (to the nearest whole degree)
Step-by-step explanation:
To visualize, let's assume the tower is leaning to the right, (as viewed by us).
If the rope is dropped from the top to the ground, the tower, the rope and the ground (between the rope and the tower) form a right triangle.
The height and base of the triangle are given
Often we're looking for the angle near the ground, but here, we're looking for the angle between the rope and the tower because the angle is congruent to the angle that the tower forms with it's original vertical position (alternate interior angles). If the tower was standing straight up and hadn't been leaning at all, we wouldn't say that the tower was leaning 90°... we would say it was leaning 0°. Whereas if the tower fell over and was laying flat on the ground, we would say it was leaning at 90°. So, we're not measuring the angle between the tower and the ground, but rather the angle between the tower where it should be, and where it is... which is the same angle as the rope forms with the tower.
Recall that \(tan(\theta)=\dfrac {opp}{hyp}\)
Since we know the two legs of the right triangle, one can setup a tangent relationship with the two legs, but remember that the "opposite" side is going to be the ground, and the "adjacent side" will be the rope.
\(tan(\theta)=\dfrac {ground}{rope}\\tan(\theta)=\dfrac {3ft}{57ft}\\tan(\theta)=\frac {3}{57}\\\theta=tan^{-1}(\frac {3}{57})\\\theta=3.012787504^o\\\theta \approx 3^o\\\)
Consider this equation.
cos(0) = 4/41
If 0 is an angle in quadrant IV, what is the value of sin(0)
Answers
Answer: sin(0) = 40.804/41
Step-by-step explanation:

Natalie is selling fruit at the Saturday market. She has a
otal of 48 pears that she wants to sell. She makes bags of
pears and sells them for $5 per bag. In which equation
oes b represent the number of bags of pears?
Answers
If Natalie puts 4 pears in each bag, she will be able to sell a total of 12 bags of pears at the Saturday market.
To represent the number of bags of pears, b, that Natalie sells at the Saturday market, we can use the following equation:
b = total_number_of_pears / pears_per_bag
In this equation, "total_number_of_pears" represents the total quantity of pears Natalie has, and "pears_per_bag" represents the number of pears she puts in each bag.
Given that Natalie has a total of 48 pears, we can substitute the value into the equation:
b = 48 / pears_per_bag
Now, we need to determine the number of pears she puts in each bag. The information provided states that Natalie sells bags of pears, and each bag is sold for $5. However, the specific number of pears per bag is not given. To proceed, we need this information.
Let's assume that Natalie puts 4 pears in each bag. We can substitute this value into the equation:
b = 48 / 4
Simplifying the equation gives:
b = 12
So, if Natalie puts 4 pears in each bag, she will be able to sell a total of 12 bags of pears at the Saturday market.
It's important to note that the specific value of "pears_per_bag" will affect the final result. If Natalie puts a different number of pears in each bag, the equation will yield a different number of bags sold.
For more such questions on sell visit:
https://brainly.com/question/24951536
#SPJ8
In a lottery, the top cash prize was $632million, going to three lucky winners. Players pick four different numbers from 1 to 57 and one number from 1 to 44. A player wins a minimum award of $100 by correctly matching two numbers drawn from the white balls (1 through 57) and matching the number on the gold ball (1 through 44). What is the probability of winning the minimum award?
Answers
The probability of winning the minimum award of $100 in the lottery is \(\frac{113}{140448}\)
The probability of picking first winning number (out of 57 white balls) is \(\frac{1}{57}\)
Now, out of the remaining 56 white balls, the probability of picking second winning number is \(\frac{1}{56}\)
So total probability of the correctly matching two numbers drawn from the white balls is \(\frac{1}{57} + \frac{1}{56}\)
Next, to match the winning number on the gold ball, probability of this event is \(\frac{1}{44}\)
Thus overall probability of winning the minimum award is calculated by multiplying the individual probabilities of aforesaid two independent events as follows:
\((\frac{1}{44}) (\frac{1}{57}+\frac{1}{56})\)
= \(\frac{113}{140448}\)
Above value is the probability of winning the minimum award of $100.
To know more about "probability" click here
https://brainly.com/question/11234923
#SPJ1
Median of 3,6,9,7,4,6,7,0,7
Answers
The median, mean, range and mode will be 6, 5.4, 9 and 7.
What is median?
The median is the middle number in the data set when the data set is written from least to greatest.
The median is the number in the middle when arranged in an ascending order. The numbers will be:
0, 3, 4, 6, 6, 7, 7, 7, 9.
The median is 6.
The range is the difference between the highest and lowest number which is: = 9 - 0 = 9
The mode is the number that appears most which is 7.
The mean will be the average which will be:
= (0 + 3 + 4 + 6 + 6 + 7 + 7 + 7 + 9) / 9.
= 49/9
= 5.4
To know more about median check the below link:
https://brainly.com/question/14532771
#SPJ1
Please help me in this math problem
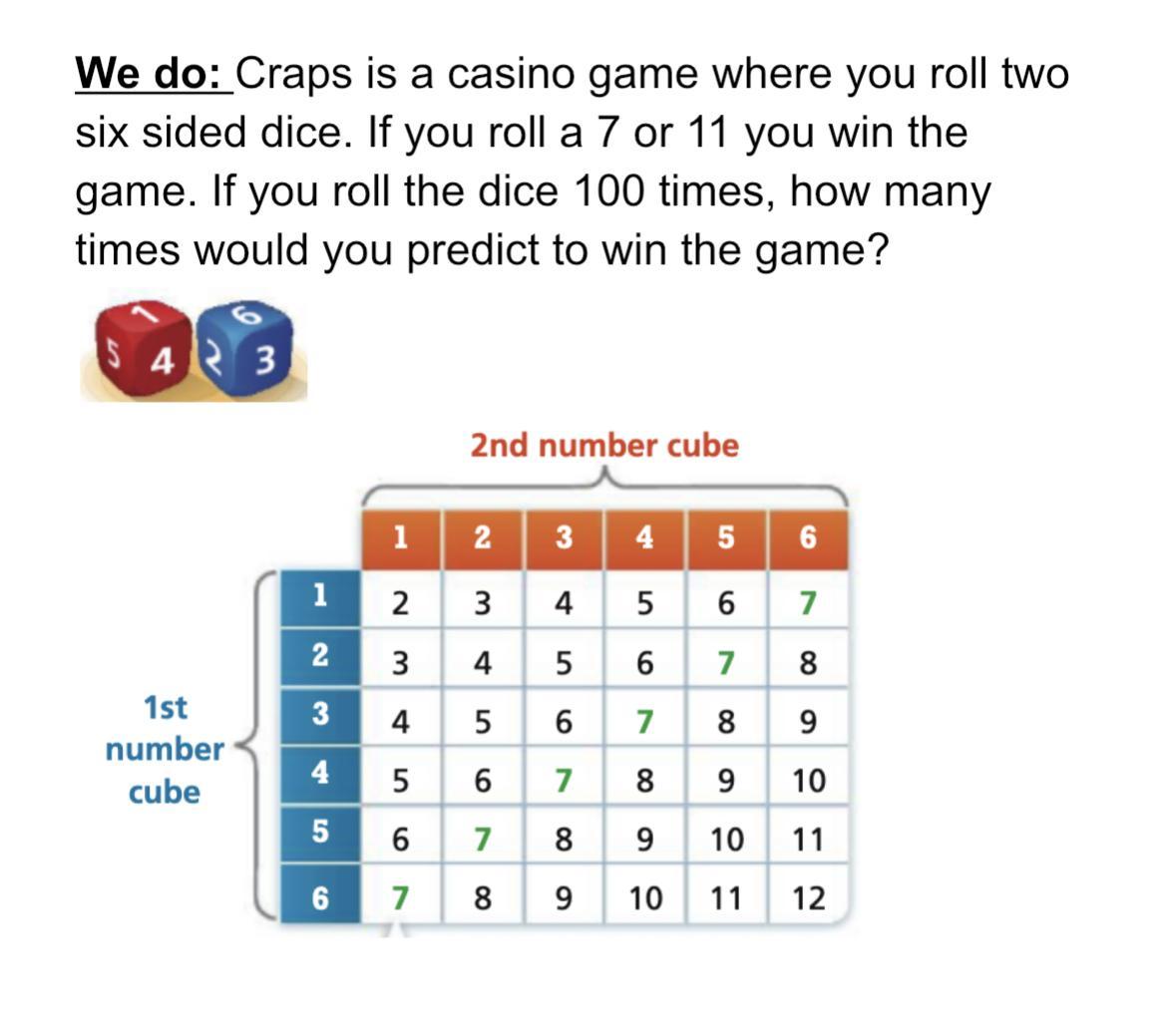
Answers
Answer:
Step-by-step explanation: well, you will more than likely get it at least 60%-80% of the time! If this doesn't help i can try to explain in a lot more detail unless someone does for me!
Pls help explain how to do this I need help
[(4r²t)³]²
Answers
Answer:
To solve the expression [(4r²t)³]², you need to follow the order of operations (PEMDAS):
Calculate the expression inside the outermost parenthesis first: (4r²t)³ = (4 * r^2 * t)^3, where r and t are variables.
Raise the result to the power of 3: (4 * r^2 * t)^3 = 64 * r^6 * t^3.
Raise the result to the power of 2: (64 * r^6 * t^3)^2 = 4096 * r^12 * t^6.
So, [(4r²t)³]² = 4096 * r^12 * t^6.
Step-by-step explanation:
Question 2 of 37
Suppose that F(x) =3/x and G(X) 3/x-10Which statement best compares
the graphs of F(x) and G(x)?

Answers
Answer:
F(x) = x^3 and G(x) = (4x)^3 − 5
so
G(x) = F(4x) -5
the visual effect is
1) shrink horizontally by 1/4
2) shift down by 5
therefore
the answer is the option
D.The graph of G(x) is the graph of F(x) compressed horizontally and shifted 5 units down.
Step-by-step explanation: