Answers
Answer:
All you have to do is turn x-2y=6 into y=mx+b form. That would mean you need to get y by itself. Subtract x from 6. Then you have -2y=6-x. Now you have to divide both sides of the equation by -2. So then you have -2y/-2 = 6-x/-2. That turns out to y=3-1/2x Which is easier read by: y=1/2x - 3
Related Questions
An ant needs to travel along a 20cm × 20cm cube to get from point A to point B. What is the shortest path he can take, and how long will it be (in cm)? WILL MARK BRAINLIEST
Answers
Answer:
The shortest path to take is \(20\sqrt{3}\ cm\) or \(34.64\ cm\)
Step-by-step explanation:
This question requires an attachment (See attachment 1 for question)
Given
Cube Dimension: 20cm * 20cm
Required
Shortest path from A to B
For proper explanation, I'll support my answer with an additional attachment (See attachment 2)
The shortest path from A to B is Line labeled 2
But first, the length of line labeled 1 has to be calculated;
This is done as follows;
Since, the cube is 20 cm by 20 cm
\(Line1^2 = 20^2 + 20^2\) (Pythagoras Theorem)
\(Line1^2 = 2(20^2)\)
Take square root of both sides
\(Line1 = \sqrt{2(20)^2}\)
Split square root
\(Line1 = \sqrt{2} * \sqrt{20^2}\)
\(Line1 = \sqrt{2} * 20\)
\(Line1 = 20\sqrt{20}\)
Next is to calculate the length of Line labeled 2
\(Line2^2 = Line1^2 + 20^2\) (Pythagoras Theorem)
Substitute \(Line1 = 20\sqrt{20}\)
\(Line2^2 = (20\sqrt{2})^2 + 20^2\)
Expand the expression
\(Line2^2 = (20\sqrt{2})*(20\sqrt{2}) + 20 * 20\)
\(Line2^2 = 400*2 + 400\)
Factorize
\(Line2^2 = 400(2+1)\)
\(Line2^2 = 400(3)\)
Take square root of both sides
\(Line2 = \sqrt{400(3)}\)
Split square root
\(Line2 = \sqrt{400} * \sqrt{3}\)
\(Line2 = 20 * \sqrt{3}\)
\(Line2 = 20 \sqrt{3}\)
The answer can be left in this form of solve further as follows;
\(Line2 = 20 * 1.73205080757\)
\(Line2 = 34.6410161514\)
\(Line2 = 34.64 cm\) (Approximated)
Hence, the shortest path to take is \(20\sqrt{3}\ cm\) or \(34.64\ cm\)


Answer:
44.72 cm
Step-by-step explanation:
1. This was marked correct by RSM
2. Unfold the cube, so that points A and B and on points diagonal from each other on a 40 cm x 20 cm rectangle. Now draw a line connecting points A to B. That is the hypotenuse of both triangles. Now according to the pythagorean theorem, the hypotenuse is √2000, which is equal to 5√20.
3. The answer is 44.72 cm
The line plot represents the wait time in line for a ride at a local fair.
A line plot titled Wait Time at the Fair. The horizontal line labeled Time in Minutes begins at 4, with every one unit labeled up to 10. There are 2 dots above 8. There are 3 dots above 5. There are 5 dots above 7. There are 6 dots above 6.
Which of the following best describes the shape of the data, and why?
The data is skewed and might mean that the wait times were lower than 5 minutes because the park was not busy.
The data is skewed and might mean that the wait times were higher than 7 minutes because the park was busy.
The data is symmetric and might mean that most rides had a wait of 6 to 7 minutes, which are the expected times for those rides.
The data is bimodal with peaks and might mean that the wait times were usually 5 or 7 minutes to ride, which is lower than the expected wait time for those rides.
Answers
The data being skewed and indicating higher wait times above 7 minutes due to a busy park is the most suitable description based on the given line plot.
The best description of the shape of the data is that it is skewed and might mean that the wait times were higher than 7 minutes because the park was busy.
Here's the explanation:
From the line plot, we can observe that there are 6 dots above 6, 5 dots above 7, 3 dots above 5, and 2 dots above 8.
The distribution is not symmetric, as the data points are not evenly spread around a central value.
The fact that there are more dots above 7 and 8 suggests that the wait times were higher than these values for a significant number of rides. This skewness in the data indicates that there were instances of longer wait times.
Additionally, the presence of dots above 5 and 6 suggests that there were some rides with shorter wait times as well.
However, the higher concentration of dots above 7 and 8 indicates that the park was likely busy, leading to longer wait times.
The option stating that the data is skewed and might mean that the wait times were higher than 7 minutes because the park was busy best aligns with the information provided by the line plot.
It acknowledges the skewness of the data towards higher wait times, suggesting that the park experienced increased demand and longer queues during the fair.
For similar question on data.
https://brainly.com/question/31089407
#SPJ8
what is 1 5/12 + 1 8/12 for iready
Answers
Answer:
3 1/12
Step-by-step explanation:
there are about 127 million workers in the u.s. about 14.4 million workers belong to unions. what is the approximate percentage of workers who are not protected by unions (round to the nearest percent)?
Answers
11% is the approximate percentage of workers who are not protected by unions.
What is percentage?Rather than being stated as a fraction, a percentage is a piece of a total expressed as a number between 0 and 100. Nothing is zero percent; everything is 100 percent; half of something is 50 percent, and nothing is zero percent. You divide the part of the whole by the whole and multiply the result by 100 to get the percentage.
In essence, percentages are fractions with 100 as the denominator. We place the percent sign (%) next to the number to indicate that the number is a percentage.
One-tenth of anything is one percent. Therefore, it may be expressed as a fraction as well as a decimal.
Given that,
there are about 127 million workers in the u.s.
among them 14.4 million workers belong to unions
Now, workers belong to the united states = (127 - 14.4) = 112.6 million
So, number of people protected by unions = (112.6 / 127) × 100 = 88.66%
Now, the approximate percentage of workers who are not protected by unions: (100 - 88.66) = 11.33% ≈ 11%
To know more about percentage refer to:
https://brainly.com/question/24877689
#SPJ4
Which of the following is the correct point-slope equation for the line that
passes through the point (-4,-2) and is parallel to the line given by
y = 5x + 44?
Ay+2= 5(x+4)
OB. y-4-5(x-2)
OC. y+4= 5(x+2)
OD. y-2= 5(x-4)
Answers
The correct point-slope equation for the line that passes through the point (-4,-2) and is parallel to the line given by y = 5x + 44 is: A. y + 2 = 5(x + 4)
How to determine an equation of this line?In Mathematics and Geometry, the point-slope form of a straight line can be calculated by using the following mathematical expression:
y - y₁ = m(x - x₁)
Where:
x and y represent the data points.m represent the slope.Since the line is parallel to y = 5x + 44, the slope is equal to 5.
At data point (-4, -2) and a slope of 5, a linear equation for this line can be calculated by using the point-slope form as follows:
y - y₁ = m(x - x₁)
y - (-2) = 5(x - (-4))
y + 2 = 5(x + 4)
Read more on point-slope here: brainly.com/question/24907633
#SPJ1
Can someone please help me it’s geometry

Answers
The surface are of the rectangular pyramid is 576.4 in².
What is a pyramid?
A pyramid is a three-dimensional shape. A pyramid has a polygonal base and flat triangular faces, which join at a common point called the apex.
To calculate the surface are of the rectangular pyramid, we use the formula below
Formula:
S.A = l'(l+w)+lw................. Equation 1Where:
S.A = Surface area of the pyramidl' = Slight height of the pyramidl = Length of the rectangular basew = Width of the rectangular baseFrom the question,
Given:
l = 11 inw = 11 inl' = 20.7 inSubstitute these values into equation 1
S.A = 20.7(11+11)+(11×11)S.A = 455.4+121S.A = 576.4 in²Learn more about pyramid here: https://brainly.com/question/22744289
#SPJ1
PLEASE HELP !!
What is the missing reason for line 6 in this proof?

Answers
CPCTC
This acronym stands for "corresponding parts of congruent triangles are congruent". The triangles being congruent leads to their corresponding pieces being the same length. It's like saying if two houses are identical, then the front doors must be identical as well. Notice how this builds off the fact the triangles are proven congruent in line 5.
Which choice is NOT a fillet type in SOLIDWORKS? constant size variable size faceangled
Answers
The correct option is (d) Angled. Among all (constant size, variable size, face, angled) The Angled is NOT a fillet type in SOLIDWORKS.
SOLIDWORKS has 3 types of fillets: constant size, variable size, and face. Constant size fillets have a constant radius applied to all edges of the selected face or faces. Variable size fillets allow the user to customize the radius of each edge. Face fillets are used to create a smooth transition between two faces.
All three types of fillets create a smooth transition between the edges and faces of a 3D model. Angled is not a type of fillet in SOLIDWORKS, but rather it is a type of chamfer, which is a type of edge treatment that creates an angled edge rather than a smooth transition. Chamfers can be used to create sharp corners or to break up the edges of a 3D model.
To know more about Chamfers:
brainly.com/question/883430
#SPJ4
A dam spillway is 40 ft long and has fluid velocity of 10 fus. Considering Weber number effects as minor, calculate the corre- sponding model fluid velocity for a model length of 5 ft.
Answers
The problem involves determining the corresponding model fluid velocity for a dam spillway with a given length and fluid velocity, considering Weber number effects as minor. The model length is provided, and we need to calculate the model fluid velocity.
To calculate the corresponding model fluid velocity, we can use the concept of geometric similarity. According to the Froude model law, which applies to open channel flows, the ratio of velocities in a prototype and its model is equal to the square root of the ratio of their lengths.
In this case, we have a prototype dam spillway with a length of 40 ft and a fluid velocity of 10 ft/s. The model length is given as 5 ft, and we need to determine the corresponding model fluid velocity.
Using the Froude model law, we can write the equation as follows:
(V_model / V_prototype) = \(\sqrt{(L_model / L_prototype)}\)
Substituting the given values, we have:
(V_model / 10 ft/s) = \(\sqrt{5 ft / 40 ft}\)
Simplifying the equation, we find:
V_model = 10 ft/s * \(\sqrt{5/40}\)
Calculating the square root and performing the multiplication, we obtain the corresponding model fluid velocity.
In summary, by applying the Froude model law and utilizing the given lengths and fluid velocities, we can determine the corresponding model fluid velocity for the dam spillway.
Learn more about length here:
https://brainly.com/question/32060888
#SPJ11
8 divided by 2/7 please :)
Answers
Answer:
28
Step-by-step explanation:
8÷2/7 = 8 x 7/2 = 56/2 = 28
^Its the same as this^
Hope this helps :D
Answer:
28
Step-by-step explanation:
8÷2/7 = 8 x 7/2 = 56/2 = 28
Hope this answers your question!
A can of soup has the shape of a cylinder.
The diameter of the base of the can is
3.4 inches, and the height of the can is
4.5 inches. What is the volume of the can
rounded to the nearest tenth of a cubic
inch?
Answers
Answer:
Step-by-step explanation:
Height h = 4.5 inches
Radius r = 3.4/2 = 1.7 inches
Area of base = πr² = 2.89π square inches
Volume of cylinder = πr²h = 2.89π4.5 = 13.0 cubic inches
Answer:
The answer is 40.8
Step-by-step explanation:
The volume of a cone equals the area of a circle multiplied by the height
\(v = \pi \times {r}^{2} \times h \\ = 3.14 \times 1.7 \times 1.7 \times 4.5 \\ = 40.8\)
Buys a new car for $25,300. The car depreciates exponentially at a rate of 7. 5% per year. How much is the car worth after six years?
Answers
The car is worth approximately $14,488.57 after six years of depreciation at a rate of 7.5% per year, given that it was bought for 25,300.
To find the value of the car after six years of depreciation at a rate of 7.5% per year, we can use the formula:
Value after t years = Initial value x \(e^(-rt)\)
where r is the annual depreciation rate as a decimal (in this case, 0.075), t is the number of years of depreciation (in this case, 6), and e is the mathematical constant approximately equal to 2.71828.
Substituting the values given in the problem, we get:
Value after 6 years = 25,300 x \(e^(-0.075 x 6)\)
Simplifying the expression inside the exponential, we get:
Value after 6 years = 25,300 x \(e^(-0.45)\)
Using a calculator to evaluate \(e^(-0.45)\), we get:
Value after 6 years = 14,488.57 (rounded to the nearest cent)
Therefore, after six years at 7.5% annual depreciation, the automobile is now valued roughly 14,488.57.
To learn more about depreciation please click on below link
https://brainly.com/question/17827672
#SPJ4
Please help me with this anyone

Answers
Answer:
Step-by-step explanation:
Begin by combining like terms and then factoring. Combining like terms will give you
\(10p^2-17p-20=0\) Using the "old-fashioned" way of factoring, the a times c method, our a = 10, b = -17 and c = -20.
a * c = 10(-20) = -200 and now we need the factors of 200 (don't worry about the negative) that combine to give us that middle term, -17p (here is where the negative matters). The factors of 200 are:
1. 200; 2, 100; 4. 50; 5, 40; 8, 25; 10, 20
The combination of those numbers that can be manipulated to give us a -17p is the 8, 25 as long as we say that the 25 is negative and the 8 is positive. Rewrite the original polynomial to reflect those factors:
\(10p^2-25p+8p-20=0\) and then factor by grouping:
\((10p^2-25p)+(8p-20)=0\) and factor out from each set of parenthesis what is common:
\(5p(2p-5)+4(2p-5)=0\) again factor out what is common:
(2p - 5)(5p+ 4) = 0. These are the factors; therefore the solutions are
2p - 5 = 0 so
2p = 5 and
p = 5/2 and
5p + 4 = 0 and
5p = -4 so
p = -4/5
which of the following bases is the weakest? the base is followed by its kb value. a) hoch2ch2nh2, 3.2 × 10-5 b) nh3, 1.76 × 10-5 c) c5h5n, 1.7 × 10-9 d) (ch3ch2)3n, 5.2 ×
Answers
The weakest base is C5H5N (pyridine) with Kb = 1.7 × 10^(-9). The correct answer is C.
The strength of a base is determined by its ability to accept or donate a pair of electrons. In general, a stronger base has a higher tendency to accept or donate electrons compared to a weaker base.
In the given options, the bases are characterized by their Kb values. Kb (base dissociation constant) represents the equilibrium constant for the reaction of a base with water to form the corresponding conjugate acid and hydroxide ions.
Comparing the Kb values provided:
(CH3CH2)3N with Kb = 5.2 × 10^(-4) C5H5N (pyridine) with Kb = 1.7 × 10^(-9) NH3 with Kb = 1.76 × 10^(-5)HOCH2CH2NH2 with Kb = 3.2 × 10^(-5)A smaller Kb value indicates a weaker base because it corresponds to a lower concentration of hydroxide ions in solution. Therefore, in this case, C5H5N (pyridine) with a Kb value of 1.7 × 10^(-9) is the weakest base among the given options. The correct answer is C.
Learn more about base at https://brainly.com/question/17464001
#SPJ11
Kelly is designing a logo for a company. She creates the four-sided
figure PBAC, as shown. Then, she creates an image of figure
PBAC by rotating it 90 degrees clockwise about point P to create
figure PB' A'C'. Then, she performs the same rotation to create
image PB''A''C'.
Answers
Solve for x over the real numbers:
x^2 - 10 x - 27 = 0
Hint: | Solve the quadratic equation by completing the square.
Add 27 to both sides:
x^2 - 10 x = 27
Hint: | Take one half of the coefficient of x and square it, then add it to both sides.
Add 25 to both sides:
x^2 - 10 x + 25 = 52
Hint: | Factor the left hand side.
Write the left hand side as a square:
(x - 5)^2 = 52
Hint: | Eliminate the exponent on the left hand side.
Take the square root of both sides:
x - 5 = 2 sqrt(13) or x - 5 = -2 sqrt(13)
Hint: | Look at the first equation: Solve for x.
Add 5 to both sides:
x = 5 + 2 sqrt(13) or x - 5 = -2 sqrt(13)
Hint: | Look at the second equation: Solve for x.
Add 5 to both sides:
Answer:
x = 5 + 2√(13) or x = 5 - 2√(13)
Triangle
A
′
B
′
C
′
is the image of
Triangle
A
B
C
under a rotation about point
Q
Determine the angle of rotation
A:-165 degrees
B:-120 degrees
C:165 degrees
D:120 degrees

Answers
Answer:
The correct answer is 120. Thank me later.
Step-by-step explanation:
Zane began this table to show the equalvalent ratios for x and y but realized that he made a mistake. He wants to change one number to make the table correct
Answers
Zane set out to create a table that would demonstrate equivalent ratios for x and y, but after completing it, he noticed an error.
As per the question given,
Determined to correct the mistake and provide accurate information, he took a closer look at each row and column of the table to identify the incorrect number.
Using his problem-solving skills, Zane was able to pinpoint the incorrect number and replace it with the correct one, ensuring that the table accurately represented the equivalent ratios for x and y. With this correction, Zane could rest easy knowing that he had provided reliable information for others to use and reference.
As the saying goes, "to err is human," but Zane's dedication to accuracy and attention to detail ensured that his mistake did not go unnoticed, and he was able to take the necessary steps to correct it.
For such more questions on Ratio
https://brainly.com/question/12024093
#SPJ4
Given a hashing function H(x) = y, where y is an n=128 bit output:
1. find the number of computations(hashes) required for finding a collision at 80% and 10% probabilities. Provide figures with 4 decimals.
2. you’ve access to a computer that can process a trillion hashes per second, how long will it take to find a collision at 10% probability?
Answers
1. The number of computations (hashes) required for finding a collision at 80% and 10% probabilities is 1.8447 x 10^19.
2. It will take approximately 47,891 seconds (or 13.3 hours) to find a collision at 10% probability.
The number of computations (hashes) required for finding a collision at 80% and 10% probabilities depends on the size of the hash function output. Let's assume a hash function output size of 128 bits for this example.
For finding a collision at 80% probability, we need to use the birthday attack algorithm. The number of hashes required can be calculated using the following formula:
N = sqrt((2^(n+1))*ln(1/(1-p)))
where n is the size of the hash output in bits (n=128 in this case), p is the desired probability of finding a collision (p=0.8 in this case), ln is the natural logarithm, and sqrt is the square root function.
Substituting the values, we get:
N = sqrt((2^(128+1))*ln(1/(1-0.8))) = 2^64.3155 = 1.8447 x 10^19
Therefore, we need 1.8447 x 10^19 hashes to find a collision at 80% probability.
For finding a collision at 10% probability, we can use the following formula:
N = sqrt((2^(n+1))*ln(1/(1-p)))
Substituting the values, we get:
N = sqrt((2^(128+1))*ln(1/(1-0.1))) = 2^55.9724 = 4.7891 x 10^16
Therefore, we need 4.7891 x 10^16 hashes to find a collision at 10% probability.
If we have a computer that can process a trillion hashes per second, we can calculate the time required to find a collision at 10% probability as follows:
time = N / rate
where N is the number of hashes required to find a collision (N=4.7891 x 10^16), and rate is the rate of hashes that the computer can process per second (rate=1 trillion = 1 x 10^12).
Substituting the values, we get:
time = (4.7891 x 10^16) / (1 x 10^12) = 4.7891 x 10^4 seconds
Therefore, it will take approximately 47,891 seconds (or 13.3 hours) to find a collision at 10% probability with a computer that can process a trillion hashes per second.
Know more about computations here:
https://brainly.com/question/29558206
#SPJ11
To determine a1 = (1,0,-1)", a₂ = (1, 1, 1)T and 93=(3, 1,-1)" are linearly dependent or linearly independent. Let us consider the matrix with columns as a₁ = (1,0,-1) a2 = (1, 1, 1) and 11 3 A = 01 1 1 -1 a3=(3, 1,-1) to be Now a1 = (1,0,-1)", a2 = (1,1,1) and a3=(3, 1,-1) are linearly dependent or linearly independent accordingly the determinant of the matrix A is zero or not equal to zero. 1 1 3 0 1 1 A 1 For we will get 1 3 |A| = 01 1 -1 1-1 |A| = 1[(1)(-1)-(1¹)(1)]1[(0)(-1)-(1)(-1)] +3[(0)(-1)-(1)(-1)] |A| = 1[-1-1] - 1[0 + 1] +3[0 + 1] |A|-2-1+3|A| = 0, As|A| = 0, so a1 = (1, 0, -1) a₂ = (1,1,1) and a3 = (3, 1,-1) are linearly dependent. Hence, a1 = (1,0,-1)", a₂ = (1, 1, 1) and a3 = (3, 1,-1) are linearly dependent.
Answers
The vectors a₁ = (1, 0, -1), a₂ = (1, 1, 1), and a₃ = (3, 1, -1) are linearly dependent.
We have,
To determine if the vectors a₁ = (1, 0, -1), a₂ = (1, 1, 1), and a₃ = (3, 1, -1) are linearly dependent or linearly independent, we can follow these steps:
Step 1:
Form the matrix A by arranging the vectors a₁, a₂, and a₃ as columns:
A = [1 1 3; 0 1 1; -1 1 -1]
Step 2: Calculate the determinant of matrix A:
|A| = 1[(1)(-1)-(1)(1)] - 1[(0)(-1)-(1)(-1)] + 3[(0)(-1)-(1)(-1)]
= 1[-1-1] - 1[0 + 1] + 3[0 + 1]
= -2 - 1 + 3
= 0
Step 3:
Analyze the determinant value. If the determinant |A| is equal to zero, it indicates that the vectors a₁, a₂, and a₃ are linearly dependent. If the determinant is non-zero, the vectors are linearly independent.
Therefore,
The vectors a₁ = (1, 0, -1), a₂ = (1, 1, 1), and a₃ = (3, 1, -1) are linearly dependent.
Learn more about vectors here:
https://brainly.com/question/24256726
#SPJ4
(GIVING BRAINLIEST!!!)

Answers
Answer:
5
Step-by-step explanation:
5/30 = 1/6
Jessie goes to the market every 64 days. Chris goes to the same market every 72 days
They met each other one day. How many days later ws they meet each other again? Pleaseee answer this question
Answers
Answer:
576
Step-by-step explanation:
64×9 = 576
72×8= 576
The LCM of both numbers is 576
Answer:
Step-by-step explanation:
It will be the least common multiple of 64 and 72 days
64 = 2 * 2 * 2 * 2 * 2 * 2 = 2⁶
72 = 2*2*2*3*3 = 2³ * 3²
LCM = 2⁶ * 3² = 64 * 9 = 576
Both will meet each other again 576 days
Marisol rents a bike in a city she is visiting. If
she pays $10.50 to rent the bike for 14 minutes,
what is the average price per minute?
Answers
Answer:
$10.50 ×14 = 150
Step-by-step explanation:
espero que te sirve
$0.75 is the average price per minute.
What is division?Division is the process of splitting a number or an amount into equal parts.
Division is one of the four basic operations of arithmetic, the ways that numbers are combined to make new numbers. The other operations are addition, subtraction, and multiplication.
here, we have,
she pays $10.50 to rent the bike for 14 minutes,
i.e. the average price per minute is,
$10.50 /14
=$0.75
Hence, $0.75 is the average price per minute.
To learn more on division click:
brainly.com/question/21416852
#SPJ2
i need help with this question

Answers
The number of hours that each person worked is given as follows:
Sophie: 7 hours.Addison: 4 hours.How to obtain the number of hours worked by each person?The number of hours worked by each person is obtained solving a system of equations, for which the variables are given as follows:
Variable x: number of hours worked by Sophie.Variable y: number of hours worked by Addison.They worked a total of 11 hours, hence:
x + y = 11
y = 11 - x.
They ironed 260 shirts, hence:
20x + 30y = 260.
Replacing the first equation into the second, the value of x is obtained as follows:
20x + 30(11 - x) = 260
10x = 70
x = 7.
Then the value of y is obtained as follows:
y = 11 - 7 = 4.
More can be learned about a system of equations at https://brainly.com/question/30915484
#SPJ1
PLEASE HELP ILL GIVE 53 POINTS

Answers
B: Yes
C: No (but, if the angles are 90°, then yes. Though in general no)
if 11 is subratecet from 4 times a number the result is 89 find the number
Answers
Answer:
25
Step-by-step explanation:
Answer:
25
Step-by-step explanation:
4x-11=89
4x=89+11
4x=100
x= 25
I hope this will help
PLEASE YALL I NEED HELP WITH EVERYTHING!!

Answers
Answer:
I'm not entirely sure how to help-
Step-by-step explanation:
Answer:
what do you mean by everything? do you have any pictures of the questions?
Step-by-step explanation:
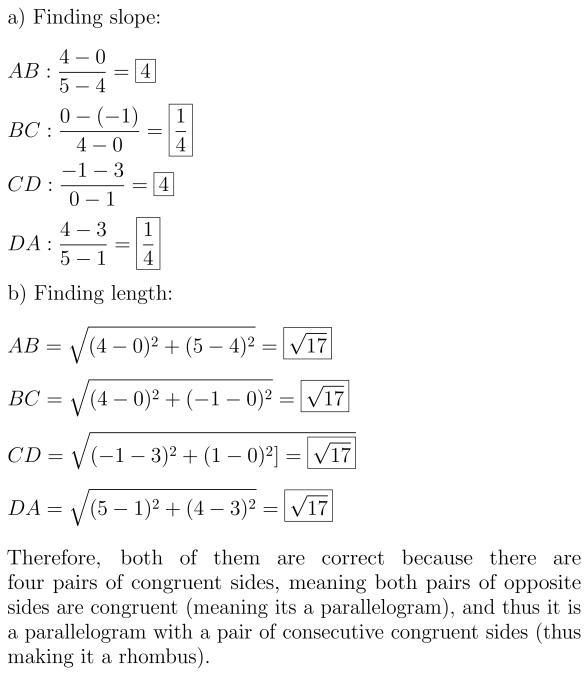
can anyone help me with this slope-length activity? whoever answers will get 5 stars and brainliest, i know its a bit of work so I'd really appreciate it, I've been rlly struggling with this unit. this is 10th grade geometry

Answers
Answer:
Step-by-step explanation:
a recent study shows that the amount of daily sugar intake by an american follows a normal distribution with a mean of 67 grams and standard deviation of 5 grams. let be a random variable representing the amount of daily sugar intake of an american. suppose you take a random sampe of size 5 people and calculate the sample average, . estimate the probability that is lower than 63 grams.
Answers
The probability that is lower than 63 grams is 95%
Given that,
X~ Normal ( 67 grams, 5 grams ) , n = 5
=> E( x ) = 67 grams , Var( X ) =5 grams
* ~ Normal ( 67 grams , 1 grams )
We know that, if X~ Normal ( E ( x) = a . Val (x ) = b)
Then X~ Normal ( E(x) = a, √Var (x)/n = √b/n)
E(x) = a, Var (x)/ = √b/n
Probability that sample mean X , is blw 65 and 70 grams
P ( 65< X<70 ) =
= P(Z<1. 76 ) - P(Z≤-2.65)
Using standard normal table values represent area
left of the Z- score, we get
= 0.96080 - 0.00402
= 0 .95678
=0.9568
So,The probability that is lower than 63 grams is 95%
To learn more about Probability click here:
brainly.com/question/11234923
#SPJ4
Find the range for the measure of the third side of a triangle when the measures of the other two sides are 6 ft and 19 ft.
Answers
The range of possibilities for the third side of the triangle, after using the triangle inequality theorem is 13 < n < 25.
According to the theorem of triangle inequality, the sum of any two of a triangle's sides must exceed the length of the third side. When we know the lengths of two sides, x and y, we can apply the triangle inequality theorem to estimate the length of the third side, which will be between x + y and x - y.
As a result,
x – y < n < x + y
19 – 6 < n < 19 + 6
13 ft < n < 25 ft
To learn more about triangle inequality theorem, click here:
https://brainly.com/question/30095626
#SPJ4
Order of operations
(6+5) +9 =

Answers
Answer:
(6+5)+9=20
Step-by-step explanation:
