Which expression is equivalent to13 p + 15? Btw happy almost halloween

The solution is Option C.

Number of squares n in a size number s is given by the equation n = 4s + 1

What is an Equation?

Equations are mathematical statements with two algebraic expressions flanking the equals (=) sign on either side.

It demonstrates the equality of the relationship between the expressions printed on the left and right sides.

Coefficients, variables, operators, constants, terms, expressions, and the equal to sign are some of the components of an equation. The "=" sign and terms on both sides must always be present when writing an equation.

Given data ,

Let the equation be represented as A

Now , the value of A is given by

Let the number of squares be = n

Let the size number be = s

Now ,

Figure 1 :

The size number s = 1

The number of squares in the figure 1 is = 5 squares

So , the equation will be n = 4s + 1

n = 4 x 1 + 1 = 5 squares

Figure 2 :

The size number s = 2

The number of squares in the figure 2 is = 9 squares

So , the equation will be n = 4s + 1

n = 4 x 2 + 1

n = 8 + 1 = 9 squares

Figure 3 :

The size number s = 3

The number of squares in the figure 2 is = 13 squares

So , the equation will be n = 4s + 1

n = 4 x 3 + 1

n = 12 + 1 = 13 squares

Hence , The equation is given by n = 4s + 1 , where n is the number of squares and s is the size number

To learn more about equations click :

https://brainly.com/question/19297665

#SPJ1

Answer:

20/3

Step-by-step explanation:

Answer:

15 cents right?

Step-by-step explanation:

3/20=.15

Step-by-step explanation:

To solve the system of equations:

5x + y = 9

10x - 7y = -18

We can use the elimination method by multiplying the first equation by 7 and subtracting it from the second equation:

35x + 7y = 63 (multiplying first equation by 7)

10x - 7y = -18

45x = 45

Dividing both sides by 45, we get:

x = 1

Substituting x = 1 into the first equation:

5(1) + y = 9

Simplifying, we get:

y = 4

Therefore, the solution to the system of equations is x = 1 and y = 4.

Answer:

nth term = tn = n� - 2n - 6

if i'm wrong i'm rlly rlly sorry!!

Answer:

Three chocolate bars will cost £1.95

Step-by-step explanation:

I divided £3.25 by 5, this told me they cost £0.65 each so i used that and multiplied it by 3 to get my answer £1.95

The open spaces in sculpture are called negative spaces.

In sculpture, negative space refers to the empty or void areas that exist between and around the solid forms or objects. It is the space that surrounds and defines the positive elements or shapes in a sculpture. Negative space plays a crucial role in creating balance, contrast, and harmony in sculptural compositions.

When an artist sculpts an object, they not only consider the physical mass and volume of the object itself but also pay attention to the spaces that are created as a result. These empty spaces are as important as the solid forms and contribute to the overall aesthetic and visual impact of the sculpture. By carefully manipulating the negative spaces, artists can enhance the perception of the positive elements and create a sense of depth, movement, and tension within the artwork.

In contrast, positive space refers to the solid or occupied areas in a sculpture, while the terms "literal" and "linear" do not specifically relate to the concept of open spaces in sculpture. Therefore, the correct answer is negative spaces.

to learn more about positive click here:

brainly.com/question/29546604

#SPJ11

Answer:

8

Step-by-step explanation:

8

Answer:

B

Step-by-step explanation:

2x=16

16/2=8

Answer is 8

The length of the diagonal outlined by rectangular deck of 8 ft by 15 ft is 17 ft.

A rectangle is the quadrilateral (has four sides and four angles) in which each angle measures 90 degrees. Opposite sides are parallel and equal.

The length of the diagonal (x) can be determined using Pythagoras, hence:

x² = 15² + 8²

x = 17 feet

The length of the diagonal outlined by rectangular deck of 8 ft by 15 ft is 17 ft.

Find out more on rectangle at: https://brainly.com/question/25292087

Therefore, the probability of being dealt a full house in a 5-card poker hand is approximately 0.00144 or 0.144%.

To calculate the probability of being dealt a full house in a 5-card poker hand, we need to consider the total number of possible hands and the number of favorable hands (full houses).

Total number of possible hands:

There are 52 cards in a standard deck, and we choose 5 cards for our hand. So, the total number of possible hands is given by the combination formula:

C(52, 5) = 52! / (5! * (52 - 5)!)

= 2,598,960

Number of favorable hands (full houses):

To form a full house, we need to consider the choices for the three cards of one denomination and the two cards of another denomination.

Number of choices for the denomination of three cards: We have 13 denominations (2, 3, 4, ..., 10, J, Q, K, A) to choose from.

Number of ways to choose the three cards of one denomination: We have 4 cards of each denomination in a deck. Therefore, we have C(4, 3) = 4 ways to choose three cards of one denomination.

Number of choices for the denomination of two cards: We have 12 remaining denominations (since we already used one for the three cards).

Number of ways to choose the two cards of another denomination: We have C(4, 2) = 6 ways to choose two cards of another denomination.

To calculate the total number of favorable hands, we multiply these choices:

Number of favorable hands = 13 * C(4, 3) * 12 * C(4, 2) = 13 * 4 * 12 * 6

= 3,744

Now we can calculate the probability by dividing the number of favorable hands by the total number of possible hands:

Probability of getting a full house = Number of favorable hands / Total number of possible hands

= 3,744 / 2,598,960

≈ 0.00144

To know more about probability,

https://brainly.com/question/29580548

#SPJ11

The events that are mutually exclusive are randomly selecting a number that is less than 0 and greater than -2

and randomly selecting a number that is less than 5 and greater than -5.

The group of whole numbers and negative numbers is known as an integer in mathematics. Integers, like whole numbers, do not include the fractional portion. Integers can therefore be defined as numbers that can be positive, negative, or zero but not as fractions. On integers, we can carry out all arithmetic processes, including addition, subtraction, multiplication, and division. Integer instances include 1, 2, 5, 8, -9, -12, etc. "Z" stands for a number. Let's now go over the definition of integers, their symbol, types, operations, rules, and properties, as well as how to represent them on a number line with numerous worked-out instances.

To know more about integers, visit

https://brainly.com/question/15276410

#SPJ1

Answer:

Area of shape =  187 cm²

Step-by-step explanation:

Given:

Length of rectangle = 15 cm

Width of rectangle = 9 cm

Height of triangle = (22-9) cm = 13 cm

Base of triangle = (15-7) cm = 8 cm

Find:

Area of shape

Computation:

Area of shape =  Area of rectangle  + Area of triangle

Area of shape =  (l)(b) + (1/2)(b)(h)

Area of shape =  (15)(9) + (1/2)(8)(13)

Area of shape =  135 + 52

Area of shape =  187 cm²

In order to find a critical value (maximum or minimum) we need to compute the first derivative, which is given by

Then, a critical value ocurrs when

which implies that

So by adding 6 to both side, we have

Therefore, there is a maximum or minimum at x=1.

In order to see if the point represents a maximum or minimum, we need to find the second derivative of our function, which is given by

We have that if the second derivative is positive, the point represents a minimum and if it is negative, the point represents a maximum. In our case, since the second derivative is greater than zero (positive number) there is a minimum point at x=1. Then, by substituting this values into the function, we get

so the minimum point is located at (1,2).

Therefore, with the above information, the answers are:

Does the function have a minimum or maximum values? Answer: Minimum

Where does the minimum or maximum value occur? Answer: x=1

Whats is the funtion's minimum or maximum values? Answer: 2

The marginal propensity to consume (MPC) for Australia in 2021, when total income (Y) is 10, is 0.3.

The consumption function for Australia in 2021 is given as C = 0.0052 + 0.3Y + 20, where C represents the total consumption and Y represents the total income. To calculate the MPC, we need to determine how much of an increase in income is consumed rather than saved. In this case, when Y = 10, we substitute the value into the consumption function:

C = 0.0052 + 0.3(10) + 20

C = 0.0052 + 3 + 20

C = 23.0052

Next, we calculate the consumption when income increases by a small amount, let's say ΔY. So, when Y increases to Y + ΔY, the consumption function becomes:

C' = 0.0052 + 0.3(Y + ΔY) + 20

C' = 0.0052 + 0.3Y + 0.3ΔY + 20

To find the MPC, we subtract the initial consumption (C) from the new consumption (C') and divide it by the change in income (ΔY):

MPC = (C' - C) / ΔY

MPC = (0.0052 + 0.3Y + 0.3ΔY + 20 - 23.0052) / ΔY

Simplifying the equation, we can cancel out the terms that don't involve ΔY:

MPC = (0.3ΔY) / ΔY

MPC = 0.3

Learn more about Marginal propensity

brainly.com/question/32676784

#SPJ11

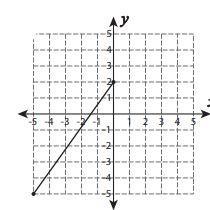
Answer:

Step-by-step explanation:

hello :

x-32>32+9x

means : x-9x>32+32

-8x >64

dvid by :8        -x >8  .......continu

The eigenvalues of the matrix H are λ₁ = 1 + 3i, λ₂ = 1 - 3i, and λ₃ = 5. The corresponding eigenvectors are v₁ = [7i, 0, 1], v₂ = [-7i, 0, 1], and v₃ = [0, 1, 0].

To find the eigenvalues and eigenvectors of the given matrix H, we will first perform block diagonalization. The matrix H can be written as:

H =\(BDB^(^-^1^)\),

where D is the diagonal matrix of eigenvalues and B is the matrix of eigenvectors. We can find B by solving the equation H·B = B·D.

Finding the eigenvalues

To find the eigenvalues, we solve the secular equation |H - λI| = 0, where I is the identity matrix. Substituting the values of H, we have:

|1 - λ   0      7i  |

|0       3 - λ   0   | = 0

|-7i     0       5 - λ|

Expanding the determinant, we get:

(1 - λ)[(3 - λ)(5 - λ) + 7i·(-7i)] - 7i[0 - (-7i)·(7i)] = 0

Simplifying further, we obtain:

(1 - λ)[(3 - λ)(5 - λ) + 49] + 49 = 0

Expanding and collecting terms, we get:

(λ - 1)λ² - 8λ - 250 = 0

Solving this quadratic equation, we find the eigenvalues λ₁ = 1 + 3i, λ₂ = 1 - 3i, and λ₃ = 5.

Finding the eigenvectors

To find the eigenvectors, we substitute each eigenvalue into the equation H·v = λv, where v is the eigenvector corresponding to the eigenvalue.

For λ₁ = 1 + 3i:

(1 - (1 + 3i))v₁₁ + 0v₁₂ + (7i)v₁₃ = 0

(0)v₁₁ + (3 - (1 + 3i))v₁₂ + (0)v₁₃ = 0

(-7i)v₁₁ + (0)v₁₂ + (5 - (1 + 3i))v₁₃ = 0

Simplifying each equation, we get:

-3iv₁₁ + 7iv₁₃ = 0

2v₁₂ = 0

-4iv₁₁ + 4iv₁₃ = 0

Solving these equations, we find v₁ = [7i, 0, 1].

Similarly, for λ₂ = 1 - 3i, we find v₂ = [-7i, 0, 1].

For λ₃ = 5, we find v₃ = [0, 1, 0].

Learn more about Block diagonalization

brainly.com/question/30705300

#SPJ11

The measurement of angle DBC is equal to 33°, here we have to know the meaning of angle.

Angle is the measurement distance between two straight line or ray when they meet and it can also say that their one part is opening and other part is joint.

We have given that, ∠ABD = 4x, ∠DBC = 3x and measure of angle ABC is equal to 77°.

So ∠ABD + ∠DBC = ∠ ABC

⇒  4x + 3x = 77°

⇒ 7x = 77°

⇒ x = 11°

So, ∠ ABD = 4x = 4 * 11 = 44°

and, ∠DBC = 3x = 3 * 11 = 33°

Therefore, angle DBC is equal to 33°.

To Learn more about Angle, visit:

https://brainly.com/question/1309590

#SPJ1

Complete Question:

What is the measure of angle DBC if the measure of angle ABD is represented by 4x, the measure of angle DBC is represented by 3x and the measure of angle ABC is 77° ?

Answer:

Rectangle B has a length of 4 inches.

Step-by-step explanation:

Rectangle A has a width of 3 inches and a length of 5 inches.

Let us find its perimeter:

Perimeter = 2(L + W)

where L = length

W = width

Its perimeter is therefore:

P = 2(5 + 3)

P = 2 * 8 = 16 inches

Rectangle B has the same perimeter as Rectangle A. Let us find the length of B.

Its width is 4 inches, therefore, perimeter is:

P = 2(L + W)

16 = 2(L + 4)

16 = 2L + 8

16 - 8 = 2L

8 = 2L

=> L = 8 / 2 = 4 inches

Rectangle B has a length of 4 inches.

The value of the function (f - g)(x) would be \((f-g)(x)=x^2-11x+4\).

Option (A) is correct.

What are composite functions?

A composite function is generally a function that is written inside another function. The composition of a function is done by substituting one function into another function. For example, f [g (x)] is the composite function of f (x) and g (x). The composite function f [g (x)] is read as “f of g of x”.'

Given:

                \(f(x)=4x^2-5x\\\\g(x)=3x^2+6x-4\)

Now by using the definition of composite functions we can write,

      \((f-g)(x)=f(x)-g(x)\\\\(f-g)(x)=4x^2-5x-(3x^2+6x-4)\\\\(f-g)(x)=4x^2-5x-3x^2-6x+4\\\\(f-g)(x)=x^2-11x+4\)

Hence, the value of the function (f - g)(x) would be \((f-g)(x)=x^2-11x+4\).

To learn more about composite functions, visit:

https://brainly.com/question/10687170

#SPJ1

Answer: 45 (depends on what the rightmost angle is)

Step-by-step explanation:

All angles of a triangle add up to 180 degrees.

Two angle measures are provided, 100 and 35 (?)

Add up the two to get 135, and subtract from 180

180 - 135 = 45 degrees.

I might be seeing the rightmost angle measure wrong but I think it's 35, if it's not you can still apply the same strategy, just add the two given angles and subtract that from 180 to find x.

a) This can't be correct because we will see that she found 6.7 animals, and that can't be.

b) in this case the fraction of plants ios 2/5, then the number of plants is 8.

a) We know that she found 20 species of animals and plants, so, if she says that a fraction of 1/3 of these are animals, then the number of animals will be:

A = (1/3)*20 = 20/3 = 6.7

That is not a whole number, so it isn't correct.

b) If 3/5 of the species were animals, then 2/5 were plants, so the number of plants is given by:

P = (2/5)*20 = 2*20/5 = 2*4 = 8

There are 8 plants in this case.

Learn more about fractions at:

https://brainly.com/question/11562149

#SPJ1

The sine of 0 radians is 0.

In trigonometry, the sine of an angle is defined as the ratio of the length of the side opposite to the angle to the length of the hypotenuse of a right-angled triangle.

When the angle is 0 degrees (or 0 radians), the opposite side has a length of 0, and thus the ratio is also 0. Therefore, the sine of 0 radians is 0.

In a circle with the radius r, the horizontal axis x, and the vertical axis y, 0 is the angle formed by the two sides x and r; r moving counterclockwise is the positive angle.

Note that, the sine function maps an angle to a value which is between -1 and 1, with a periodic nature, meaning that the values repeat in a cycle as the angle increases.

Know more about sine here:

https://brainly.com/question/12595712

#SPJ11

Answer:

B ?

Step-by-step explanation:

Answer:

The answer is A. they quickly illustrate measure of center. and C. they show trends in data that can be compared.

Answer:

6 centimeters

Step-by-step explanation:

60 millimeters

10 millimeters = 1 centimeter

How long is the picture in centimeters

______________________________

We know the scale of millimeters to centimeter which is when there are 10 millimeters, 1 centimeter is made. Now we're looking for 60 millimeters, let's see how many times it takes 10 to get to 60.

10 * 6 = 60

So you have to multiply by 6 to find you answer :

1 centimeter * 6

6 centimeters

Answer:

f = 3

g = -1

h = 6

r = 18

Step-by-step explanation:

Box 1

f(x) = \(2x^{4}\)-\(12x^{3}\)+\(16x^{2}\)+4x+15 with x = 3

f(3) = \(2(3^{4)}\) - \(12(3^{3} )\) + \(16(3^{2})\)+ 4(3) + 15

Reorder

Evaluate

Multiply

3f = 2 x 81 - 12 + 27 +16 x 9 + 12 +15

3f = 162 - 324 + 144 + 12 + 15

3f =  9

3  ÷  3

f = 3

Box 1

g(x) = \(3x^{3}\) - \(16x^{2}\) - 7x - 36 with x = 6

g(6) = \(3(6^{3} )\) - \(16(6^{2})\) - 7(6) - 36

g6 = 3 x 216 - 576 - 42 - 36

g6 = -6

6   ÷ 6

g = -1

Box 2

h(x) = \(5x^{3}\) - \(2x^{2}\) - 3 with x = -1

h(-1) = \(5(-1^{3} )\) - \(2(-1^{2})\) -3

h-1 = -5 + 2 -3

h-1 = -6

-1    ÷  - 1

h = -6

Box 2

r(x) = \(4x^{4}\) - \(9x^{2}\) + 5x - 2 with x = 2

r(2) = \(4(2^{4} )\) - \(9(2^{2} )\) + 5(2) - 2

r2 = 64 - 36 + 10 - 2

r2 = 36

2   ÷  2

r = 18

The area is 120 \(cm^{2}\).

Area is the size of a two-dimensional surface, usually expressed in square units, such as square feet or square meters. It is the amount of two-dimensional space enclosed within a given set of boundaries. Area can be calculated for various shapes, including squares, rectangles, triangles, circles, and more complex shapes. Area is also a measure of the amount of space an object occupies. In mathematics, area is used to measure the size of a shape or region in a plane. It can also be used to calculate the size of a figure, such as the area of a triangle, or to measure the area of an enclosed space, such as a garden or a room.

Given:

AB = CD = 8cm

AD = BC = 12cm

EF = DH = 4cm

So, the required area is A = Area (ABCEFD) = Area (ABCD) + Area (CEFD)

Area of ABCD = Area (ABCD) = AB × BC

⇒ 12 × 8 = 96 \(cm^{2}\)

Area of CEFD = Area (CEFD) = 0.5 (CD + EF) × HD

⇒ 0.5 × (8 + 4) × 4 = 24\(cm^{2}\)

Now, the required area is

⇒ A = 96 \(cm^{2}\) + 24 \(cm^{2}\) = 120 \(cm^{2}\)

Hence, the required area of the figure is 120 \(cm^{2}\).

To learn more about area from the given link:
https://brainly.com/question/25965491
#SPJ1

Given that \(\(T(n)\) = \(10n^2-3n\)\) if (\(\(n=1\)\)), you have to prove it by induction. So, we have proved it by induction that  \($$\(T(n)=10n^2-3n\)$$\)  if ( n= 1). The given statement is true for all positive integers n

Let's do it below: The base case (n=1) is given as follows: \(T(1)\) =\(10\cdot 1^2-3\cdot 1\\&\)=\(7\end{aligned}$$\). This implies that \(\(T(1)\)\) holds true for the base case.

Now, let's assume that \(\(T(k)=10k^2-3k\)\) holds true for some arbitrary \(\(k\geq 1\).\)

Thus, for n=k+1, T(k+1) = \(10(k+1)^2-3(k+1)\\&\) = \(10(k^2+2k+1)-3k-3\\&\)=\(10k^2+20k+7k+7\\&\) = \(10k^2-3k+20k+7k+7\\&\) = \(T(k)+23k+7\\&\) = \((10k^2-3k)+23k+7\\&\) =  \(10(k+1)^2-3(k+1)\).

Therefore, we have proved that the statement holds true for n=k+1 as well. Hence, we have proved it by induction that  \($$\(T(n)=10n^2-3n\)$$\)  if (n=1). Therefore, the given statement is true for all positive integers n.

For more questions on: integers

https://brainly.com/question/17695139

#SPJ8  

Rate of change of the angle of elevation = 96/845 radians / sec

To solve this related rate (of change) problem:

Attached is the solution picture.

You can learn more about rate from:

https://brainly.com/question/13324776

#SPJ4