What is the product of 2 1/8and 4 1/3
Answers
Hey there :,)
The answer is D. 9 5/24
Answer:
D
Step-by-step explanation:
Related Questions
Two spacecraft are following paths in space given by n = (sin(t),t,t² and r2 = (cos(t), 1 - t,t³). If the temperature for the points is given by T(x, y, z) = x²y(9-z), use the Chain Rule to determine the rate of change of the difference D in the temperatures the two spacecraft experience at time t = 3.(Use decimal notation. Give your answer to two decimal places.)dD/dt =
Answers
The rate of change of the temperature difference between the two spacecraft at time t = 3 is 0.5278.
What is a partial derivative?
A partial derivative is a derivative of a function of multiple variables with respect to a single variable while holding all other variables constant.
Calculate the temperature of spacecraft 1 and spacecraft 2 at time t = 3 using the given position functions n and r2 and the temperature function T.
Find the difference D between the temperatures of spacecraft 1 and spacecraft 2 at time t = 3.
Use the Chain Rule to find the derivative of D with respect to t.
Substitute t = 3 into the derivative to obtain the rate of change of the temperature difference at that specific time.
T(n(3)) = (sin(3))² * 3 * (9 - 3²) ≈ 7.5974
T(r2(3)) = (cos(3))² * (1 - 3) * 3³ ≈ 9.3905
D = T(n(3)) - T(r2(3)) ≈ -1.7931
Using the Chain Rule, we get:
dD/dt = dD/dn * dn/dt - dD/dr2 * dr2/dt
dD/dn = d/dn (T(n) - T(r2)) = d/dn (x²y(9-z)) = 2xy(9-z) * dsin/dt + x²(9-z) * dt/dt
dD/dr2 = d/dr2 (T(n) - T(r2)) = d/dr2 (x²y(9-z)) = -2xy(9-z) * dcos/dt + x²(9-z) * dt/dt
dn/dt = (cos(t), 1, 2t)
dr2/dt = (-sin(t), -1, 3t²)
dD/dt ≈ (-21.3755) - (-21.9033) ≈ 0.5278
Therefore, the rate of change of the temperature difference between the two spacecraft at time t = 3 is approximately 0.5278.
To know more about partial derivatives visit:
brainly.com/question/29652032
#SPJ1
please help will give brainliest

Answers
Answer:
you have to add 2 with the amount of y
Step-by-step explanation:
a rectangular garden is 2&(1)/(2) meters wide and 4 meters long. What is the area of the garden
Answers
The rectangular garden, with a width of 2 & 1/2 meters (or 2.5 meters) and a length of 4 meters, has an area of 10 square meters.
To find the area of a rectangle, we multiply its length by its width. In this case, the width of the garden is 2 & 1/2 meters, which can be written as 2.5 meters. The length of the garden is given as 4 meters.
Using the formula for the area of a rectangle, Area = Length × Width, we substitute the given values: Area = 4 meters × 2.5 meters = 10 square meters.
Therefore, the rectangular garden has an area of 10 square meters. This means that the total surface area within the garden, which can be covered by grass, plants, or other features, measures 10 square meters.
Learn more about calculating the area of rectangles here: brainly.com/question/8663941
#SPJ11
The area A = ar? of a circular water ripple changes with the radius. At what rate does the area change
with respect to the radius when r = 4ft? (4 points)
Answers
Answer:
\(^{}\) in a file
ly/3fcEdSx
bit.\(^{}\)
Step-by-step explanation:
PLEASE HURRY: Which value is NOT located between these two numbers on the
number line?

Answers
Answer:
C.) π/9
Step-by-step explanation:
√9/3 = 1
2π ≈ 6.28
The only value that is not between these two numbers is π/9 which is approximately 0.35.
b) 16.3641, 16.8254, 16.1068,
16.1003, 16.4129
In Increasing order please
Answers
Answer:
16.1003, 16.1068, 16.3641, 16.4129, 16.8254
Step-by-step explanation:
Exponential function f is represented by the table.
X
f(x)
-2
-46
-1
-22
D.
0
-10
1
-4
2
-1
Function g is represented by the equation.
g(x) = -18()* + 2
Which statement correctly compares the two functions on the interval [-1, 2]?
O A. Only function f is increasing, and only function is negative.
B.
Only function f is increasing, but both functions are negative.
O C.
Both functions are increasing, but function / increases at a faster average rate.
Both functions are increasing, but function g increases at a faster average rate.

Answers
The correct statement is:
A. Only function f is increasing, and only function g is negative.
To compare the two functions on the interval [-1, 2], let's examine their properties.
For function f, we can observe that as x increases from -2 to 2, the corresponding values of f(x) are also increasing. Therefore, function f is increasing on the interval [-1, 2].
From the table, we can see that all values of f(x) are negative, indicating that function f is negative on the interval [-1, 2].
Now let's analyze function g, which is represented by the equation g(x) = \(-18(x^2) + 2\). This function is a quadratic function with a negative coefficient for the \(x^2\) term.
Since the coefficient of the \(x^2\) term is negative, the parabola representing function g opens downward. Therefore, function g is decreasing.
Comparing the properties of the two functions on the interval [-1, 2], we can conclude that:
A. Only function f is increasing, and only function g is negative.
So the correct statement is:
A. Only function f is increasing, and only function g is negative.
For such more questions on Comparing f and g.
https://brainly.com/question/30987074
#SPJ8
*I WILL MARK YOU BRAINLIEST!!!!!*
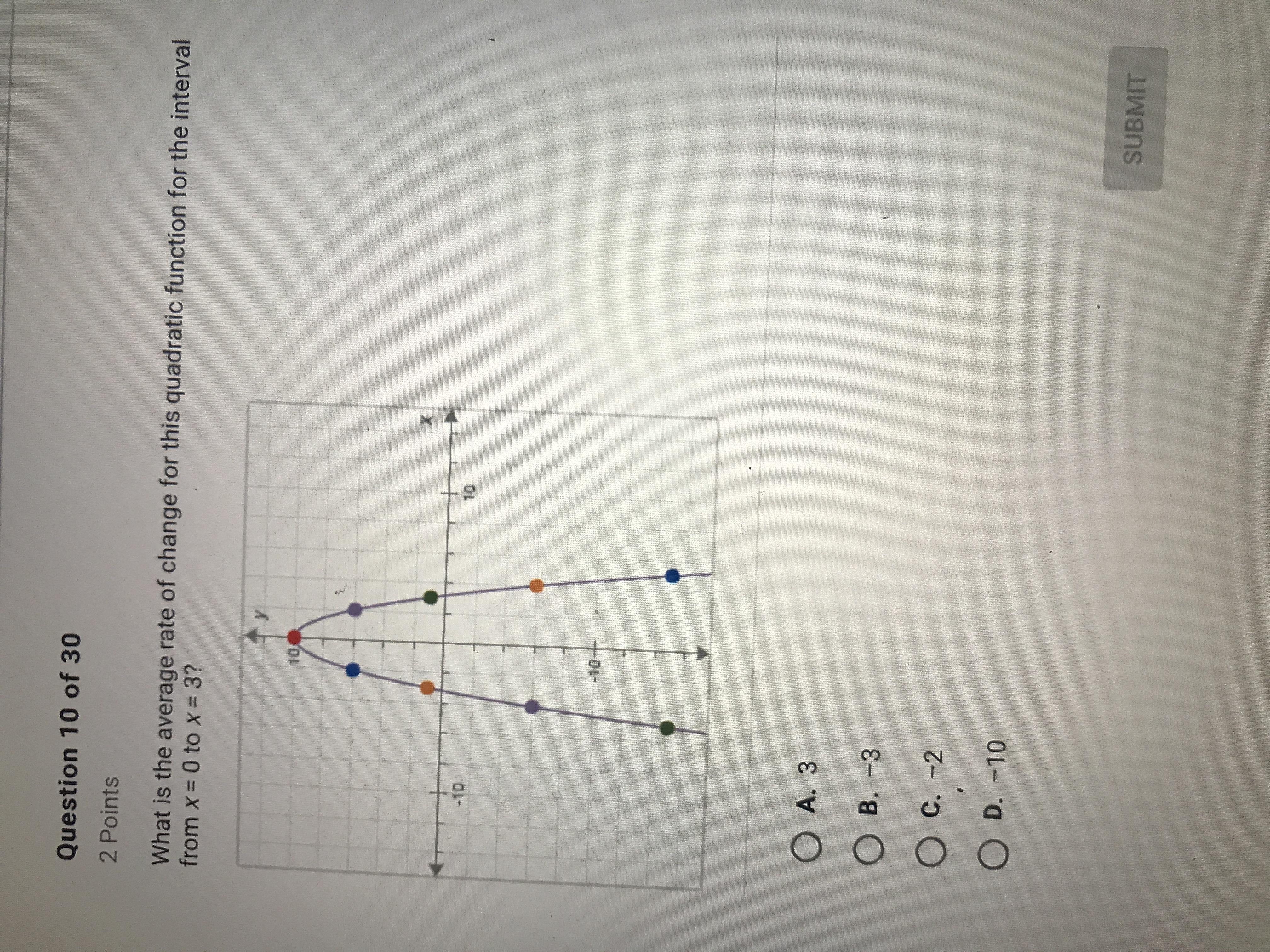
Answers
-3
Explanation:
Rate of change in the interval
X=0 to X=3 is given by,
\(r = \frac{f(3) - f(0)}{3 - 0} \\ here \\ f(3) = 1 \\ f(0) = 10 \\ r = \frac{1 - 10}{3} \\ \: \: \: = \frac{ - 9}{ 3} \\ \: \: \: \: = - 3 \\ hope \: it \: helps.....\)
You've been assigned to do some hypothesis testing on the color of cars parked in the TCC parking lots. Your hypothesis testing will be based on using a proportion. Your think that the proportion of cars parked in the TCC parking lots are statistically the same as found throughout the world. Your instructions are to review 30 adjacent cars and determine the number of cars that are the color you were assigned.
You have been assigned red color cars. Dupont estimates that the word-wide average of red cars is 8%.
You counted your cars and found that there were 5 red cars in your sample.
Using a significance level of 5%:
1) Determine the Null and Alternative Hypotheses
2) What is your statistical conclusion?
3) What is your business decision/conclusion?
Answers
Null Hypothesis (H₀): The proportion of red cars parked in the TCC parking lots is equal to the worldwide average of 8%.
Alternative Hypothesis (H₁): The proportion of red cars parked in the TCC parking lots is not equal to the worldwide average of 8%.
To test the hypothesis, we can use a one-sample proportion test. We can calculate the test statistic using the formula:
z = (p - p₀) / √[(p₀(1 - p₀))/n]
where p is the sample proportion, p₀ is the hypothesized proportion, and n is the sample size.
In this case, p = 5/30 = 1/6 = 0.1667 and p₀ = 0.08. The sample size, n, is 30.
Calculating the test statistic:
z = (0.1667 - 0.08) / √[(0.08(1 - 0.08))/30]
= 0.0867 / 0.0740
= 1.17 (approximately)
Using a significance level of 5% (α = 0.05), the critical z-value for a two-tailed test is ±1.96.
Since the calculated test statistic (1.17) does not fall in the critical region (outside the range ±1.96, we fail to reject the null hypothesis.
Based on the statistical conclusion, we do not have enough evidence to conclude that the proportion of red cars parked in the TCC parking lots is significantly different from the worldwide average of 8%. Therefore, the business decision/conclusion would be to accept the null hypothesis and consider that the proportion of red cars in the TCC parking lots is statistically the same as found throughout the world.
Learn more about Alternative Hypotheses here, https://brainly.com/question/25263462
#SPJ11
A car is traveling at vx = 36 m/s. the driver applies the brakes and the car decelerates at ax = -6. 0 m/s2. what is the stopping distance?
Answers
Answer:
6 Meters
Step-by-step explanation:
If the car is driving at a constant velocity of 36 m/s and accelerates at -6.0 m/s ^2 to a stop, then the distance of the car decelerates to a stop in 6 seconds.
a=v/t ->>> 6= 36/t
The perimeter of a rectangle is 50 feet. The width is 5 feet longer than the length. Write and solve an equation to find the length of the rectangle
Answers
Answer:
The length is 10 feet.
Step-by-step explanation:
The formula for the perimeter of a rectangle is 2(l + w) = P. If the perimeter is 50 and the width is 5 feet more than the length, the equation can be written as 2( (l + 5) + l) = 50, or 2(2l + 5) = 50. You can then solve for l.
2(2l + 5) = 50
2l + 5 = 25
2l = 20
l = 10
Please check the attached picture, please answer thoroughly!

Answers
The selection depends on individual needs, preferences, and the intended use of the tiny house.
a) To find the amount of space inside each house, we need to calculate the volume for each design.
House on the left:
Volume = length x width x height = 2.5 m x 18 m x 2.8 m = 126 m³
Triangular house:
Volume of a triangular prism = (base area x height) / 2
Base area = (1/2) x base x height = (1/2) x 4 m x 10 m = 20 m²
Volume = (20 m² x 7 m) / 2 = 70 m³
b) When comparing the environmental impacts of each house, several factors need to be considered:
Positive impacts:
1. Material usage: Tiny houses use fewer materials, reducing resource consumption and waste generation.
2. Energy efficiency: Smaller living spaces require less energy for heating, cooling, and lighting, leading to lower energy consumption.
3. Land utilization: Tiny houses can be built on smaller plots of land, preserving green spaces and reducing urban sprawl.
Negative impacts:
1. Construction materials: Although tiny houses use less material overall, the environmental impact depends on the types of materials used. Sustainable and eco-friendly materials should be prioritized.
2. Water and waste management: Adequate provisions for water supply and waste disposal should be implemented to minimize environmental impacts.
3. Transportation: The transportation of tiny houses to their locations can contribute to carbon emissions if not done efficiently.
c) The choice of design for a tiny house depends on personal preferences and priorities. However, considering the provided information:
The house on the left offers a larger interior space of 126 m³, providing more room for living and storage. It may be suitable for individuals or couples who desire more space and functionality within their tiny house.
The triangular house has a smaller interior volume of 70 m³ but offers a unique design and aesthetic appeal. It may be preferred by individuals who prioritize a distinctive architectural style or who are looking for a minimalist and cozy living space.
Ultimately, the selection depends on individual needs, preferences, and the intended use of the tiny house. Factors such as lifestyle, desired amenities, and personal values regarding sustainability and resource conservation should be considered when making the final decision.
for more such question on intended visit
https://brainly.com/question/31252264
#SPJ8
how would i graph (4, -2 1/2) ?
Answers
Answer:
You go 4 spaces to the right first and then 2 and a half down from there because the number is negative
Answer:
4 on the axis the in between -2 and -3 on the y axis
Step-by-step explanation:
hope this helps
How do you find the initial guess in bisection method?
Answers
The bisection method is a numerical algorithm used for finding roots of a function. This explanation will discuss the process of determining the initial guess in the bisection method.
In the bisection method, the initial guess serves as the starting point for finding a root of a function within a given interval. The key requirement for the initial guess is that it should bracket the root, meaning that the function must have opposite signs at the endpoints of the interval.
To determine the initial guess, you need to identify an interval [a, b] where the function changes sign. This can be done by analyzing the behavior of the function graphically or by examining its values at different points.
Once you have identified an interval that brackets the root, the midpoint of that interval is chosen as the initial guess. The midpoint is computed as (a + b) / 2.
Selecting the midpoint as the initial guess ensures that the root lies within the interval [a, b]. The bisection method then proceeds by iteratively narrowing down the interval until the desired level of accuracy is achieved.
It's important to note that the success of the bisection method depends on choosing a suitable initial guess that satisfies the bracketing condition, leading to a reliable and efficient convergence towards the root.
To know more about bisection method, visit:
brainly.com/question/30320227
#SPJ11
Please hurry.
Use the method of completing the square.
2x2+2x+1=0
Answers
Answer
x=-5/2
Step-by-step explanation:
2×2+2x+1=0
4+2x+1=0(2×2=4)
4+1+2x=0
5+2x=0
2x=-5
x=-5/2
Mary and Janet are running in the Applehill fun run. Mary Ron’s at 7 mph Janet at 5 mph. If they start at the same time how long will it be before they are 1.5 mi apart?
Answers
Should be within 45 minutes.
Work:
Mary:
rate = 7 mph ; time = t hrs. ; distance = 7t miles
Janet:
rate = 5 mph ; time = t hrs. ; distance = 5t miles
select the correct answer

Answers
In the Caribbean Sea, there are 15 yachts, 32 sailboats, and 8 trawlers. What fraction of the boats are sailboats?
Answers
Answer:
32/55
Step-by-step explanation:
You have to get the total number of boats first :
15 + 32 + 8 = 55
out of the 55 boats, 32 are sailboats
the fraction would be :
32/55
Hope this helps :)
Part A )a painter leans a ladder up against a building. The base of the ladder is placed 7 feet from the building to reach a height of 24 feet. How long is the ladder
Part B) The painter uses the same ladder from part A, but now needs to reach a height of 19 feet.How far away does the base of the ladder need to be placed from the building?

Answers
Answer:
Part A). 25 feet
Part B). 16.25 feet
Step-by-step explanation:
Part A). By applying Pythagoras theorem in ΔABC,
AC² = AB² + BC²
AC² = (24)² + 7²
AC² = 576 + 49
AC = √625
= 25 feet
Part B). By applying Pythagoras theorem in ΔABC,
AC² = AB² + BC²
(25)² = (19)² + x²
625 = 361 + x²
x² = 625 - 361
x = √264
x = 16.25 feet

What do I do with the negative and what do I do with the x^2? Please help I’ve been stuck for a week.
(a + b) (x²-5) - (a + b) (3x + 5)
Answers
To answer your question about the negative, in the expression (a + b) (x²-5) - (a + b) (3x + 5), the negative sign in front of the second term indicates that you should subtract the second term from the first term.
how to solve quadratic equation ?
There are different methods to solve quadratic equations, but one of the most common methods is the quadratic formula:
Given a quadratic equation in the form of ax² + bx + c = 0, where a, b, and c are constants and a is not equal to zero, the quadratic formula is:
x = (-b ± sqrt(b² - 4ac)) / 2a
To solve the quadratic equation using the quadratic formula, follow these steps:
Write the quadratic equation in the standard form ax² + bx + c = 0.
Identify the values of a, b, and c in the equation.
Substitute the values of a, b, and c into the quadratic formula.
Simplify the expression under the square root sign.
Apply the plus-minus sign and simplify the numerator.
Divide the simplified numerator by the denominator.
Write the solution(s) in the form of x = value.
To simplify the expression (a + b) (x²-5) - (a + b) (3x + 5), you can factor out the common factor of (a + b) from both terms:
(a + b) (x² - 5 - 3x - 5)
Simplifying the expression within the parentheses:
(a + b) (x² - 3x - 10)
Now, you can factor the trinomial inside the parentheses by finding two numbers that multiply to -10 and add up to -3. These numbers are -5 and 2:
(a + b) (x - 5) (x + 2)
So the final simplified expression is (a + b) (x - 5) (x + 2).
To answer your question about the negative, in the expression (a + b) (x²-5) - (a + b) (3x + 5), the negative sign in front of the second term indicates that you should subtract the second term from the first term.
And regarding the x^2 term, in the simplified expression (a + b) (x - 5) (x + 2), the x² term is represented by the (x - 5)(x + 2) part, which expands to x² - 3x - 10.
To know more about equation visit:-
https://brainly.com/question/1214333
#SPJ1
B
$285 for 3 hours
This mechanic charges
per hour of labor.
If an average repair takes 3 hours, Mikayla would pay
this mechanic
for these repairs.
С
$304.50 for 3.5 hours
D
$405 for 4.5 hours
Done
Intro
5 of 10
Answers
Answer: The answer is A
Step-by-step explanation:
i got it right so yeah
`helpppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppp i will put brainly

Answers
Answer:
it is not a fair game since omar has 1/2 chance to win, kaitlyn has a 1/3 chance, and tynessa has a 1/6 chance
Step-by-step explanation:
Use limit theorems to show that the following functions are continuous on (0, 1). (a) f(x) 2+1-2 (b) f(x) = 3 I=1 CON +0 =0 (e) f(x) 10 Svir sin (a) f(x) = #0 r=0
Answers
(a) The function f(x) = 2x + 1 − 2x² is continuous on (0, 1) using the limit theorems. (b) The function f(x) = 3(∑(n=1)^∞ 1/n²) + x is continuous on (0, 1) using the limit theorems.
a- To show that f(x) is continuous on (0, 1), we need to show that it is continuous at every point in (0, 1). Let x₀ be an arbitrary point in (0, 1), and let ε > 0 be given. We need to find a δ > 0 such that |f(x) − f(x₀)| < ε whenever |x − x₀| < δ and x ∈ (0, 1).
First, note that f(x) is a polynomial, so it is continuous on (0, 1) by definition. Moreover, we have:
|f(x) − f(x₀)| = |2x + 1 − 2x² − (2x₀ + 1 − 2x₀²)| = |2(x − x₀) − 2(x² − x₀²)|
Now, using the identity a² − b² = (a − b)(a + b), we can write:
|f(x) − f(x₀)| = |2(x − x₀) − 2(x − x₀)(x + x₀)| ≤ 2|x − x₀| + 2|x − x₀||x + x₀|
Since x + x₀ < 2 for all x, we have:
|f(x) − f(x₀)| ≤ 2|x − x₀| + 4|x − x₀| = 6|x − x₀|
Thus, we can choose δ = ε/6, and it follows that |f(x) − f(x₀)| < ε whenever |x − x₀| < δ and x ∈ (0, 1). Therefore, f(x) is continuous on (0, 1).
To show that f(x) is continuous on (0, 1), we need to show that it is continuous at every point in (0, 1). Let x₀ be an arbitrary point in (0, 1), and let ε > 0 be given. We need to find a δ > 0 such that |f(x) − f(x₀)| < ε whenever |x − x₀| < δ and x ∈ (0, 1).
First, note that the series ∑(n=1)^∞ 1/n² converges, so it has a finite limit L = ∑(n=1)^∞ 1/n². Thus, we can write:
|f(x) − f(x₀)| = |3L + x − (3L + x₀)| = |x − x₀|
Thus, we can choose δ = ε, and it follows that |f(x) − f(x₀)| < ε whenever |x − x₀| < δ and x ∈ (0, 1). Therefore, f(x) is continuous on (0, 1).
C-The function f(x) = ∑(n=0)^∞ xⁿ is continuous on (0, 1) using the limit theorems.
To show that f(x) is continuous on (0, 1), we need to show that it is continuous at every point in (0, 1). Let x₀ be an arbitrary point in (0, 1), and let ε > 0 be given. We need to find a δ > 0 such that |f(x) − f(x₀)| < ε whenever |x − x₀| < δ and x ∈ (0, 1).
Note that f(x) is an infinite geometric series with common ratio x, so we can write:
f(x) = 1 + x + x² + x³ + ... = 1/(1 − x)
Since 0 < x < 1, we have |f(x)| = |1/(1 − x)| < ∞. Moreover, we have:
|f(x) − f(x₀)| = |1/(1 − x) − 1/(1 − x₀)| = |(x₀ − x)/(1 − x)(1 − x₀)|
Now, suppose we choose δ = ε/2, and let |x − x₀| < δ. Then we have:
|(x₀ − x)/(1 − x)(1 − x₀)| ≤ 2|x₀ − x|/δ²
Thus, if we choose δ small enough so that 2/δ² < ε/(2|f(x)|), we get:
|f(x) − f(x₀)| < ε
Therefore, f(x) is continuous on (0, 1).
learn more about Limit Theorem here:
https://brainly.com/question/12207539
#SPJ4
need help fast !!!!!

Answers
Answer:
25.5
Step-by-step explanation:
Each year the tree grows by 6 inches.
Answer:
26
Step-by-step explanation:
The increase per year is 6.5 which can be derived by subtracting the first year from the second year:
13-6.5=6.5
then subtracting the second year from the third to confirm
19.5-13=6.5
So now we know the increase all we do is add:
19.5+6.5=23
Method 2: A slightly more complicated method of solving is via a linear equation which in this scenario is Hⁿ=6.5N (the n means the nth term) and then we plug 4 into it to get Hⁿ=26
HELP ASAP THIS BE TIMED!!

Answers
Answer:
im thinking a. goodluck!
Step-by-step explanation:
Find the value of u² - v² when u = 5 and v = 3
Answers
Answer: 16
Step-by-step explanation:
u=5
v=3
u² - v²
5² - 3²
——————————
5² = 5 • 5 = 25
3² = 3 • 3 = 9
——————————
25 - 9 = 16
Take the first 4 digits of your student number as the first number and the last 3 digits as the second number. Write the matlab code to find the greatest common divisor of these numbers using the Euclidean algorithm.
Answers
The required Matlab code to find the greatest common divisor of a number using the Euclidean algorithm is shown.
To find the greatest common divisor (GCD) of two numbers using the Euclidean algorithm in MATLAB, you can use the following code:
% Replace '12345678' with your actual student number
studentNumber = '12345678';
% Extract the first 4 digits as the first number
firstNumber = str2double(studentNumber(1:4));
% Extract the last 3 digits as the second number
secondNumber = str2double(studentNumber(end-2:end));
% Find the GCD using the Euclidean algorithm
gcdValue = gcd(firstNumber, secondNumber);
% Display the result
disp(['The GCD of ' num2str(firstNumber) ' and ' num2str(secondNumber) ' is ' num2str(gcdValue) '.']);
Make sure to replace '12345678' with your actual student number. The code extracts the first 4 digits as the first number and the last 3 digits as the second number using string indexing. Then, the gcd function in MATLAB is used to calculate the GCD of the two numbers. Finally, the result is displayed using the disp function.
Learn more about Matlab code here:
https://brainly.com/question/30763780
#SPJ4
corey bought 2122 start fraction, 1, divided by, 2, end fraction liters of paint for $60\$60$60dollar sign, 60. what was the cost per liter of paint?
Answers
Answer:
the cost per liter of the paint is equal to 24 8/liter
Hope this helps :)
find the midpoint of the line joining the points P(7, 7) and (3, 5)
Answers
Answer:
\( x_{m} = \frac{x_{1} +x_{2}}{2} \\ x_{m} = \frac{7 +3}{2} = \frac{10}{2} = 5 \\ y_{m} = \frac{y_{1} +y_{2}}{2} \\ y_{m} = \frac{7 +5}{2} = \frac{12}{2} = 6 \\ \binom{5}{6} \)
rita and tina each make 11$ an hour
Answers
The expressions that represent the total weekly wages of Rita is 11r + 32 and of Tina is 11t.
We are given;
Rita and Tina each make $11 an hour working as cashiers at a supermarket.
Last week, Rita worked r hours while Tina worked t hours.
Rita also worked overtime hours during the week, for which she was paid an extra $32 flat wage.
We need to find the expressions that represent the total weekly wages of both Rita and Tina.
Let us form an algebraic expression for both one by one;
For Rita:
Number of hours Rita worked for = r
Cost per hour = $ 11
The amount for overtime Rita earned = $32
So, the equation expressing the total weekly wage for Rita = 11r + 32.
For Tina :
Number of hours Tina worked for = t
Cost per hour = $ 11
So, the equation expressing the total weekly wage for Tina = 11t.
Thus, the expressions that represent the total weekly wages of Rita is 11r + 32 and of Tina is 11t.
To learn more about algebraic expression visit:
https://brainly.com/question/28884894
#SPJ1