What is the product of −2 1/4 and −4 1/2?
Answers
Related Questions
the average college student in the united states spends 145 minutes per day studying, according to a 2014 research study of national student engagement. suppose students at glendale college test the hypothesis that glendale students spend more than 145 minutes per day studying. suppose students distribute a survey to a random sample of 25 students enrolled at the college. suppose the students have the results shown in the plot below:dotplot with a central peak and a left tail that is only slightly longer than the right tailonce they have collected the data, the students get into a disagreement about the next steps for their research analysis.true or false? the students should use a t-test to analyze the significance of the data they collected.
Answers
The students should use a t-test to analyze the significance of the data they collected is a true statement.
In this scenario, the population standard deviation is unknown and the sample size is small (n=25). Therefore, a t-test should be used instead of a z-test. The t-test is a statistical hypothesis test that is used to determine if there is a significant difference between the means of two groups when the sample size is small and/or the population standard deviation is unknown.
Using a t-test will allow the students to analyze the significance of their data in a more appropriate and accurate way, taking into account the small sample size and the fact that they do not know the population standard deviation.
Learn more about Hypothesis:
brainly.com/question/30665946
#SPJ11
PLEASE HELP, SOLVE THIS PROBLEM AND GIVE ME THE ANSWER!!!

Answers
Answer:
The answer is option B
f(x) = 4x + 8
g(x) = 9x + 7
(fg)(x) = (4x + 8)(9x + 7)
= 36x² + 28x + 72x + 56
= 36x² + 100x + 56
Hope this helps
Answer:
B
Step-by-step explanation:
Determine if the two functions f and g are inverses of each other
algebraically. If not, why not?

Answers
Answer:
Step-by-step explanation:

Carl's BBQ is increasing the amount of fries in their family value meal by 10%. If there were originally 16 ounces of fries in the family value meal, how many are there after the increase?
Answers
Answer:
17.6
Step-by-step explanation:
For a given type of trial, after the sentence is rendered, 45% of defendants go to prison. Among the defendants who go to prison, 40% pleaded guilty, and among those who do not go to prison, 57% pleaded guilty. Someone decides to plead not guilty at his trial; what is the probability that he will not have to go to prison?
Answers
The probability that a defendant who pleads not guilty at their trial will not have to go to prison is equal to 1 minus the probability of going to prison, which is 1 - 0.45 = 0.55 or 55%.
The probability that a defendant who pleads not guilty at their trial will not have to go to prison can be calculated using conditional probability.
Let's denote the events as follows:
A: Defendant goes to prison
B: Defendant pleads guilty
We are given the following information:
P(A) = 0.45 (45% of defendants go to prison after the sentence)
P(B|A) = 0.40 (among the defendants who go to prison, 40% pleaded guilty)
P(B|A') = 0.57 (among those who do not go to prison, 57% pleaded guilty)
We need to find P(A'|B), which represents the probability that the defendant does not go to prison (A') given that they plead not guilty (B).
Using Bayes' theorem, we have:
P(A'|B) = P(B|A') * P(A') / P(B)
To calculate P(B), we can use the law of total probability:
P(B) = P(B|A) * P(A) + P(B|A') * P(A')
Given that the defendant pleads not guilty, we can assume that P(B|A) is 0, as they are not pleading guilty. Thus, we have:
P(B) = P(B|A') * P(A')
Substituting the values:
P(B) = 0.57 * (1 - P(A))
Now we can calculate P(A'|B):
P(A'|B) = P(B|A') * P(A') / P(B)
= 0.57 * (1 - P(A)) / (0.57 * (1 - P(A)))
Simplifying the expression:
P(A'|B) = 1 - P(A)
Therefore, the probability that a defendant who pleads not guilty at their trial will not have to go to prison is equal to 1 minus the probability of going to prison, which is 1 - 0.45 = 0.55 or 55%.
Learn more about probability here
https://brainly.com/question/25839839
#SPJ11
What is the probability that a defendant who pleads not guilty at their trial will not have to go to prison?
A rectangular mural measures 238 centimeters by 435 centimeters. raegan creates a mural that is 27 centimeters longer. what's is the perimeter of ragans new mural
Answers
The perimeter of Ragans new mural is 530cm.
Rectangle:
A rectangle is a type of quadrilateral, whose opposite sides are equal and parallel. It is a four-sided polygon that has four angles, equal to 90 degrees. A rectangle is a two-dimensional shape.
Here it is given that the measure of the rectangle is 238 cm and 435cm.
The Raegan creates a mural that is 27cm.
The formula to calculate the perimeter of a rectangle:
Perimeter = 2(length + breadth)
Earlier the length is 238cm and the breadth is 435cm.
Now replace the breath 435cm to 27cm.
length = 238cm
breadth = 27cm
Therefore we get the perimeter of the rectangle:
Perimeter = 2( 238 + 27)
= 2( 265)
=530cm
Therefore the perimeter is 530cm.
To know more about the rectangle refer to the link given below:
https://brainly.com/question/15612913
#SPJ4
Answer:
1400
Step-by-step explanation:
Z23w1120х23YWhat is the measure of angle Z?
Answers
We are given a trapezoid and we are asked to determine one of its angles. To do that we need to have into account that the following angles are equal:
\(\begin{gathered} \angle W=\angle X \\ \angle Z=\angle Y \end{gathered}\)Also, the sum of two different angles must be equal to 180, therefore, we have the following relationship:
\(\angle W+\angle Z=180\)Replacing the value of angle W:
\(112+\angle Z=180\)Subtracting 112 to both sides:
\(\begin{gathered} \angle Z=180-112 \\ \angle Z=68 \end{gathered}\)Therefore, the measure of angle Z is 68.
Which expression shows the value of a $7300 investment after it has grown by 2. 3% per year for 5 years?
Answers
The problem asks for the expression that represents the value of a $7300 investment after it has grown by 2.3% per year for 5 years.
To find the value of the investment after 5 years with a growth rate of 2.3% per year, we can use the formula for compound interest:
A = P(1 + r)^n
Where:
A is the final amount,
P is the principal amount (initial investment),
r is the interest rate (in decimal form), and
n is the number of years.
In this case, the principal amount is $7300, the interest rate is 2.3% (or 0.023 as a decimal), and the number of years is 5. Plugging these values into the formula, we get:
A = 7300(1 + 0.023)^5
Simplifying the expression gives us the value of the investment after 5 years with a growth rate of 2.3% per year.
To learn more about investment click here:
brainly.com/question/17252319
#SPJ11
Expand 3(x+4) I tried this question but I cant get it quite right. Could you help me?
Answers
Unless there’s further instruction on what to do that should be correct
A bookstore had copies 180 of a magazine. Yesterday the bookstore sold 5/6 of these copies. How many copies were sold yesterday?
Answers
Answer:
150
Step-by-step explanation:
180 divide by six is 30 and 30 times 5 is 150
OA and OB are congruent. CD is a chord of both circles.If a radius is 15 ft and CD = 24 ft, how long is AB?
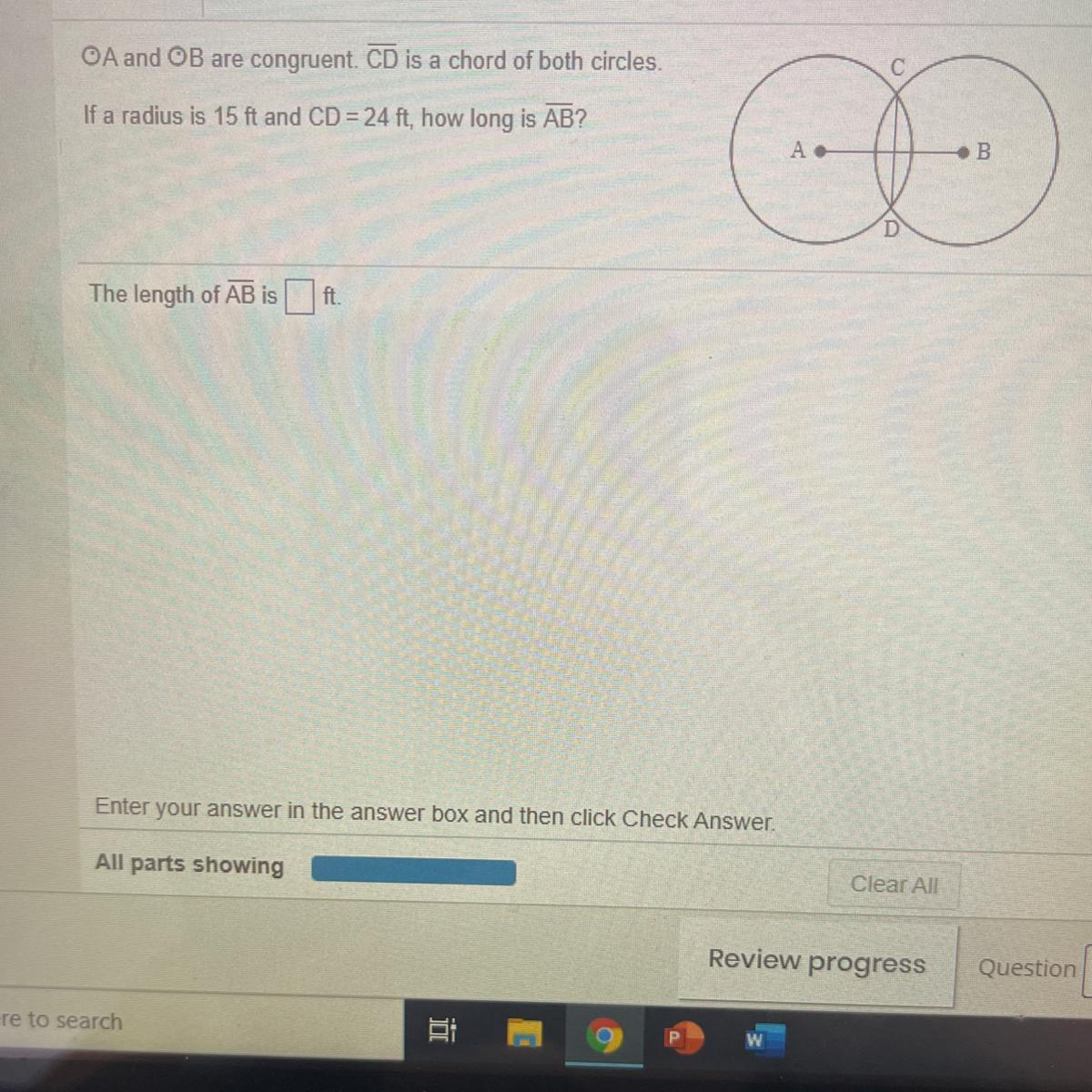
Answers
First, we have to identify the right triangle formed
As you can observe, segment AB is divided into two equal parts. We can find one part of AB using the Pythagorean's Theorem
\(c^2=a^2+b^2\)Where c = 15 and b = 12. Let's find a
\(\begin{gathered} 15^2=a^2+12^2 \\ 225=a^2+144 \\ 225-144=a^2 \\ a=\sqrt[]{81} \\ a=9 \end{gathered}\)Then, we know that AB = 2a, so
\(AB=2\cdot a=2\cdot9=18ft\)Hence, AB is 18 feet long.
Help me I don’t know if it -7 and -12

Answers
Answer:
I think it's-12
I am not sure
Please ask to some one else please
X < -7
Explain:
Please answer correctly !!!!!!!!!!!!!!!! Will mark brainliest !!!!!!!!!!!!!!!!!!!!!

Answers
Answer:
x = 123°
General Formulas and Concepts:
Geometry
Corresponding AnglesStep-by-step explanation:
The angles on the transversal and parallel lines are Corresponding Angles. Therefore, according to the definition, they are congruent.
given the graphs of f(x) and g(x), evaluate h'(3) if h(x) = f(x) xg(x)
Answers
To find h'(3) given h(x) = f(x) xg(x), we use the product rule of differentiation:
h'(x) = f'(x) xg(x) + f(x) g(x) + f(x) xg'(x)
We are not given the functions f(x) and g(x), but we can use the given graphs to estimate their values near x = 3. Let's say that f(3) = 2 and g(3) = 5. We also need to estimate f'(3) and g'(3) in order to calculate h'(3). We can estimate these values using the slopes of the tangent lines to the graphs at x = 3.
Let's say that the slope of the tangent line to the graph of f(x) at x = 3 is 1, and the slope of the tangent line to the graph of g(x) at x = 3 is 3. Then we have:
f'(3) ≈ 1
g'(3) ≈ 3
Substituting these values into the product rule for h'(x), we get:
h'(x) = f'(x) xg(x) + f(x) g(x) + f(x) xg'(x)
h'(3) = f'(3) 3 g(3) + f(3) g(3) + f(3) 3
h'(3) = (1)(3)(5) + (2)(5) + (2)(3)
h'(3) = 19
Therefore, h'(3) is approximately equal to 19, based on the given graphs and our estimates of f'(3) and g'(3).
To learn more about differentiation refer below
https://brainly.com/question/31492852
#SPJ11
How do you compare fractions with like numerators?
Answers
To compare fractions with like numerators always compare denominators.
We can calculate the LCM of the numerators and then multiply the numerators by the corresponding values to make the numerators of two or more fractions the same or similar. If the numerators are similar or the same, comparisons become simple. Verify the denominators of fractions with comparable or identical numerators. The fraction decreases as the denominator increases. They are referred to as having like or the same numerators when two or more fractions have the same numerators but distinct denominators. While they are referred to as having like or the same denominators when the denominators of two or more fractions are the same.
Learn more about like numerators
brainly.com/question/16435840
#SPJ4
jen smith has decided to become her own boss after spending 5 years as an assistant manager for a restaurant. the owner of a local sandwich store wants to sell the store to jen for $65,000 to be paid in installments of $13,000 in each of the next 5 years. according to the current owner, the store brings in revenue of about $110,000 per year and incurs operating costs of about 63% of sales. thus, once the store is paid for, jen should make about $35,000 -$40,000 per year before taxes. until the store is paid for, she will make substantially less-but she will be her own boss. realizing that some uncertainty is involved in this decision, jen wants to simulate what level of net income she can expect to earn during the next 5 years as she operates and pays for the store. in particular, she wants to see what could happen if sales are allowed to vary based on a normal distribution with mean of $100,000 and standard deviation of $10,000, and if operating costs are allowed to vary uniformly between 60% and 65% of sales. assume that jen's payments for the store are not deductible for tax purposes and that she is in the 28% tax bracket. a. create a spreadsheet model to simulate the annual net income jen will receive during each of the next five years if she decides to buy the store. (3 points) b. given the money she has in savings; jen thinks she can get by for the next five years if she can make at least $12,000 from the store each year. run 100 simulation (replication) and find the probability that jen will make at least $12,000 in each of the next five years? (1 point) c. what is the probability (based on 100 simulation) that jen will make at least $60,000 total over the next five years? (1 point)
Answers
Jen simulated her net income over 5 years assuming sales vary based on a normal distribution $100,000 and operating costs 60% and 65% of sales. keeping the loan payment be fixed at $13,000. The probability of making at least $12,000 each year is the number of successful simulations divided by 100. and at least $60,000 total over 5 years based as the number of successful simulations divided by 100.
To simulate Jen's annual net income over the next 5 years, a spreadsheet model can be created with columns for year, sales, operating costs, loan payment, and net income.
For each year, the sales can be generated from a normal distribution with mean $100,000 and standard deviation $10,000, and the operating costs can be generated from a uniform distribution between 60% and 65% of sales.
The loan payment can be fixed at $13,000, and the net income can be calculated as the difference between the sales, operating costs, loan payment, and taxes (28% of net income).
To find the probability that Jen will make at least $12,000 in each of the next five years, 100 simulations can be run using the model created in part a. For each simulation, the net income for each of the next five years can be calculated, and if the minimum net income is at least $12,000 for each year, then the simulation is counted as a success.
The probability of success can be calculated as the number of successful simulations divided by 100.
To find the probability that Jen will make at least $60,000 total over the next five years, 100 simulations can be run using the model created in part a. For each simulation, the total net income over the next five years can be calculated, and if it is at least $60,000, then the simulation is counted as a success.
The probability of success can be calculated as the number of successful simulations divided by 100.
To know more about Probability:
https://brainly.com/question/11234923
#SPJ4
A skier is trying to decide whether or not to buy a season ski pass. A daily pass costs $80. A season ski pass costs $300. The skier would have to rent skis with either pass for $25 per day. How many days would the skier have to go skiing in order to make the season pass less expensive then the daily passes?
Answers
Answer: I think it's 9 days.
Step-by-step explanation:
True/False
1) The nullspace of a 3x4 matrix cannot consist of only the zero vector.
2) The nullspace of a 4x3 matrix cannot consist of only the zero vector.
3) The set of all vectors of the form 1
x
y
where x and y range over all real numbers, is a subspace of R^3.
4) 3) The set of all vectors of the form 0
x
y
where x and y range over all real numbers, is a subspace of R^3.
Answers
Null space is defined as the set of vectors 'x' in Rn that are solutions to the matrix equation A*x = 0. False: The set of all vectors of the form 1 x y where x and y range over all real numbers is a subspace of R3. True: The set of all vectors of the form 0 x y where x and y range over all real numbers is not a subspace of R3.
1) False Null space can consist of zero vector only. A null space is defined as the set of vectors 'x' in R^n that are solutions to the matrix equation A*x = 0.
2) True The number of columns can never be less than the number of rows of the matrix in order for a null space to exist, hence a 4x3 matrix may have the null space of only the zero vector.
3) True The set of all vectors of the form 1 x y where x and y range over all real numbers, is a subspace of R^3. This set contains zero vector because for x=0 and y=0, we get the vector as [0,0,0]. Hence it is a subspace of R^3.
4) False The set of all vectors of the form 0 x y where x and y range over all real numbers, is not a subspace of R^3 as it doesn't contain zero vector. To have a subspace of R^3, it should contain a zero vector.
So, the correct options are:1) False2) True3) True4) False
To know more about matrix equation Visit:
https://brainly.com/question/27572352
#SPJ11
What is the equation of the line that passes through the point (-6,1) and has a
slope of 1?
Answers
Answer:
y = x + 7
Step-by-step explanation:
If the slope is 1, the original equation is y = mx + b, with m being 1.
Now substitute -6 and 1 for x and y and solve for b
1 = 1(-6) + b
1 = -6 + b
7 = b
Put it all together:
y = x + 7
Given g(x)=-2x^(2)+1, find the indicated values. g(0)
Answers
Answer:
\(g(x) = 2 {x}^{2} + 1 \\ g(0) = 2 ({0})^{2} + 1 \\ = 2(0) + 1 \\ = 1\)
Zachary has some dimes and some quarters. He has no more than 20 coins worth a minimum of $2.75 combined. If Zachary has 11 dimes, determine the minimum number of quarters that he could have. If there are no possible solutions, submit an empty answer.
Answers
Answer:
7 Quarters
Step-by-step explanation:
Let's assume Zachary has x quarters.The total value of the quarters is 25x cents.
The total value of the dimes is 11*10 = 110 cents.Together, the total value of the coins is 25x + 110 cents. We know that this total value is at least $2.75, which is equal to 275 cents.So we can set up the following inequality:25x + 110 >= 275Solving for x, we get:25x >= 165
x >= 6.6Since x must be a whole number, the smallest possible value for x is 7.Therefore, Zachary must have at least 7 quarters.
Zachary has 11 dimes worth $1.10 and needs to make up $1.65 for a total of $2.75. He would need 7 quarters to do this, giving him a total of 18 coins.
Explanation:To find out the minimum amount of quarters, one would need to understand that he only has 20 coins and $2.75 minimum. Considering a dime is worth 10 cents and Zachary has 11 dimes, they collectively amount to $1.10.
This leaves $1.65 to reach the minimum of $2.75. We know a quarter is worth 25 cents. To find the number of quarters needed to reach $1.65, we would divide 165 (representing $1.65) by 25 (a quarter's worth), which equals 6.6. However, you can't have 0.6 of a quarter, so we would round up to 7 quarters.
To check this answer, the total count of coins which is dimes and quarters should not exceed 20. Indeed, 7 quarters and 11 dimes equals 18 coins, which is less than or equal to 20. Therefore, the minimum number of quarters Zachary could have is 7.
Learn more about Minimum Number of Coins here:https://brainly.com/question/33722425
#SPJ2
Use the fact that the tower was 184.5 feet tall when itstood upright to answer the following questions:(a) The article states that when work began the tower Leaned 6° or 13 feet, off the perpendicular. Draw a sketch and label the two measurements.(b) How high is the tower with a lean of 6 degrees?(c) The article goes on to say that the tower now leans 16 inches less. Draw a new sketch that shows this measurement.(d) What is the degree measure of this lean?(e) What is the height of the tower with this lean?(f) Comment on the fact that sin 4° =13/184.5 = 0.07046 while sin 6° = 0.10453.Can you reconcilethis seeming discrepancy?
Answers
184.04 ft high the tower with a lean of 6 degrees, the degree measure of this lean is 3.6 degrees and most likely because either the 13ft lean has not been measured to the top edge of the tower or the side of the tower isn't straight.
In the given question, the tower was 184.5 feet tall.
(a) If the article states that when work began the tower Leaned 6° or 13 feet, off the perpendicular then we have to draw a sketch and label the two measurements.
According to given statement the sketch is given below:
(b) We have to find how much high is the tower with a lean of 6 degrees.
From the graph using the Pythagorean Theorem:
H^2 = 184.5^2- 13^2
H^2 =34040.25-169
H^2 = 33871.25
Taking square root on both side
H=184.04 ft
(c) The article goes on to say that the tower now leans 16 inches less we have to draw a new sketch that shows this measurement.
The graph is given below:
(d) Now we have to find the degree measure of this lean.
We know that the tower leans 16 in less.
So 16in= 1 1/3ft = 4/3 ft = 1.33 ft
Consequently the lean after the work is: 13ft - 1.33= 11.67 ft
Then we can find the "lean" after the work as:
sin ("lean") =11.67/184.5
sin ("lean")= 0.0633
Angel of ("lean") after work = 3.6degrees.
(e) Now we have to find the height of the tower with this lean.
Height with reduced lean is found by using Pythagorean Theorem again:
H^2 = (184.5)^2 - (11.67)^2
H^2 = 34040.25 - 136.19
H^2 = 33904.06
Taking square root on both side, we get
H = 184.13
(f) Now comment on the fact that sin 4° =13/184.5 = 0.07046 while sin 6° = 0.10453. We have to check can we reconcile this seeming discrepancy.
Most likely because either the 13ft lean has not been measured to the top edge of the tower or the side of the tower isn't straight.
To learn more about Pythagorean Theorem link is here
brainly.com/question/28361847
#SPJ4


The angles of a triangle are 5a/2, 3a/4, 7a/4. Find the value of the largest and smallest angle
Answers
The largest angle is \(\frac{7a}{4}\) and the smallest angle is \(\frac{3a}{4}\).
In this case, we have the angles \(\frac{5a}{2}, \frac{3a}{4}, and \frac{7a}{4}\). So we can set up the equation:
\(\frac{5a}{2} + \frac{3a}{4} + \frac{7a}{4} = 180\)
To solve for "a", we can simplify the left side of the equation by finding a common denominator:
\(\frac{(10a + 3a + 7a)}{4} = 180\)
Simplifying the left side gives:
\(\frac{20a}{4} = 180\)
And further simplifying gives:
5a = 180
Dividing both sides by 5 gives:
a = 36
Now that we know the value of "a", we can substitute it back into each angle expression to find their actual values:
\(\frac{5a}{2} = \frac{5(36)}{2} = \frac{903a}{4} = \frac{3(36)}{4} = \frac{277a}{4} = \frac{7(36)}{4} = 63\)
Therefore, \(\frac{7a}{4} = 63\) is the largest angle and \(\frac{3a}{4} = 27\) is the smallest angle.
To learn more about angle follow the link: https://brainly.com/question/28451077
#SPJ4
Will give brainlist if correct! Thanks if you do help!

Answers
Answer:
D is the answer
Step-by-step explanation:
20 points :3 please help
what is the equation of the line that passes through the point (5,6) and has a slope of -3/5?
Answers
Answer: y - 6 = -3/5 ( x - 5 )
Step-by-step explanation: ask for explanation, and ill give it!
Answer:
Step-by-step explanation:
Y=3x-9
A local store charges $1.97 per pound for bananas and $4.49 for a gallon of apple juice what is the cost of 1.5 lb of bananas and 1 gallon of apple juice
Answers
The expense of 1.5 lbs of bananas and 1 gallon of apple juice, according to the provided statement, is $7.45.
What does arithmetic multiple mean?Multiplication is one of the four basic operations in mathematics, along with adding, subtracting, and division. Multiply in mathematics refers to the continual adding of sets of identical size.
The cost of 1.5 lb of bananas can be found by multiplying the price per pound by the number of pounds:
Cost of 1.5 lb of bananas = 1.5 x $1.97 = $2.96 (rounded to the nearest cent)
The cost of 1 gallon of apple juice is $4.49.
So, the total cost of 1.5 lb of bananas and 1 gallon of apple juice is:
Total cost = Cost of bananas + Cost of apple juice
Total cost = $2.96 + $4.49
Total cost = $7.45
Therefore, the cost of 1.5 lb of bananas and 1 gallon of apple juice is $7.45.
To know more about Multiply visit:
https://brainly.com/question/233244
#SPJ1
the number of times a variable appears in a data set is called _____
Answers
Answer:
The number of times a variable appears in a date set is called a constant.
b) The number when 75% of the number is 12
Answers
Answer:
16
Step-by-step explanation:
(75/100)*x=12
75x=12*100
75x=1200
x=1200/75
x=16
Answer:
16
Step-by-step explanation:
divide 75 by 100
multiply 12 by 100
which is 1200
divide 1200 by 75
and your answer is 16
WILL GIVE BRAINLIST FOR FIRST ANSWER
A building covers an area of 600 square ft. the eight is 45 feet what is the volume?
Answers
Answer:
27000
Step-by-step explanation:
600(45)=27000
Answer:
27000
Step-by-step explanation:
600(45)=27000
What type of symmetry does each figure have? Check all of the boxes that apply.
An oval.
line symmetry
rotational symmetry
no symmetry
Two rectangles combine to form the shape of an L.
line symmetry
rotational symmetry
no symmetry
A smiley face.
line symmetry
rotational symmetry
no symmetry
Answers
Answer:
The oval is a line and rotational symmetry.
The line shapes rectangle has no symmetry.
The smiling face is a line symmetry.
Step-by-step explanation:
Answer:
The first oval - line symmetry, rotational symmetry
The L shape - no symmetry
The smiley face - line symmetry
Step-by-step explanation:
I just took the quiz on egde2020