Answers
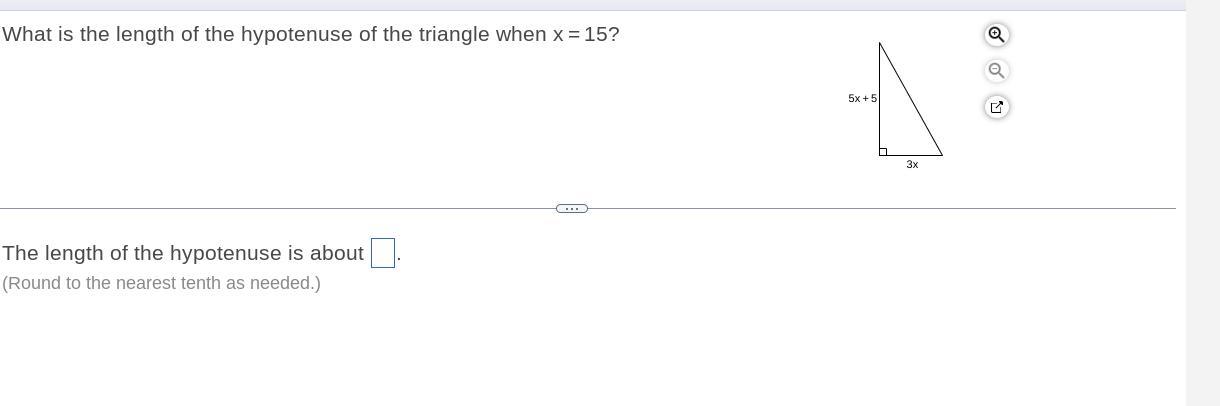
Answer: 114.24
Step-by-step explanation: 7(15)+6= 1053(15)= 45, now we plug it in the formula, so now we square root it= 114.24
Related Questions
how do you determine the quadratic equation having roots that are real numbers and equal
Answers
Answer:
To determine the nature of roots of quadratic equations (in the form ax^2 + bx +c=0) , we need to calculate the discriminant, which is b^2 - 4 a c. When discriminant is greater than zero, the roots are unequal and real. When discriminant is equal to zero, the roots are equal and real.
ANSWER:
when the value of (b^2-4ac) is positive, then the roots are real and distinct.
when it is 0 the roots are equal and if it is negative than its root is not real.
\(3/4x+3-2x = -1/4+1/2x+5\)
Answers
x = -1
Explanation:
3/4X +3-2X = 19/4+1/2X
-5X +12 = 19+ 2X
-5X -12 X = 19-12
-5X-2X=19-12
-7X = 7x=-1
help please :)))))))))))))))))))

Answers
Answer:
21600cm^3
Step-by-step explanation:
7200cm^3÷30cm=24cm
24cm×30cm×40cm
=21600cm^3
name the quadrant in which the angle is in cos theta>0, csc theta<0
Answers
Answer:
Quadrant 4
Step-by-step explanation:
Csc = 1/sin
Cos is positive in quadrants 1 and 4
Sin is negative in quadrants 3 and 4
Solve for x.
17b + bx = 34x – a
Answers
Answer:
17b + bx + a over 34 =x
Answer:
Shoot I don't know but this is as far as I got until I got confused.
Step-by-step explanation:
17b + bx = 24x - a
-17b = - 17b
-24x = -24x
------------------------------
-24x + bx = -17b - a
-24x + bx/b = -17b/b - a/b
-24x + x = -17b - a/b
-23x = -17b - a/b
-23x/-23 = -17b/-23 - a/b/-23
x =
A random sample of n = 1,000 observations from a binomial population contained 380 successes. You wish to show that p < 0.4. n = 1,000 and x = 380. You wish to show that p < 0.4. A button hyperlink to the SALT program that reads: Use SALT. Calculate the appropriate test statistic. (Round your answer to two decimal places.) z = Calculate the p-value. (Round your answer to four decimal places.) p-value = ?
Answers
The test statistic is given as follows:
z = -1.29.
The p-value is given as follows:
0.0985.
How to obtain the test statistic?The equation for the test statistic is given as follows:
\(z = \frac{\overline{p} - p}{\sqrt{\frac{p(1-p)}{n}}}\)
In which:
\(\overline{p}\) is the sample proportion.p is the proportion tested at the null hypothesis.n is the sample size.The parameters for this problem are given as follows:
\(\overline{p} = \frac{380}{1000} = 0.38, p = 0.4, n = 1000\)
Hence the test statistic is calculated as follows:
\(z = \frac{0.38 - 0.4}{\sqrt{\frac{0.4(0.6)}{1000}}}\)
z = -1.29.
Looking at the z-tabe with z = -1.29, the p-value is given as follows:
0.0985.
More can be learned about the z-distribution at https://brainly.com/question/25890103
#SPJ1
can you please help me with this 2 part problem ? there is another image to go with this I'll send to you

Answers
Question 13.
The estimated monthly cost of an energy-efficient refrigerator is $57, meaning the yearly cost is
\(57\cdot12=\$684\)Question 14.
The least energy-efficient refrigerator costs $72 per month, meaning the annual cost is
\(\$72\cdot12=\$864\)Therefore, the amount you will save on an energy-efficient refrigerator be
\(\$864-\$684=\$180\)Hence, the amount saved on an energy-efficient refrigerator will be $180.
52/4 + 8z^2
I need help solving this equation?
Answers
Answer:
8
2
+
1
3
Step-by-step explanation:
Answer = 13+8z2
David made 4/3 of a quart of fruit juice. Each mug he has holds 1/3 of quart. How many mugs will David be able to fill?

Answers
Answer:
D) 4
Step-by-step explanation:
4/3 divided by 1/3
4/3 * 3/1= 4
Based on the information given on the fraction, the number of mugs will be 4.
Solving fractionsFrom the information, David made 4/3 of a quart of fruit juice and each mug he has holds 1/3 of quart.
Therefore, the number of mugs that can be filled will be;
= 4/3 ÷ 1/3
= 4/3 × 3/1
= 4
In conclusion, the correct option is 4.
Learn more about fractions on:
https://brainly.com/question/24304697
-6<2x+8<16 compound inequality
Answers
Answer:
- 7 < x < 4
Step-by-step explanation:
- 6 < 2x + 8 < 16 ( subtract 8 from each interval )
- 14 < 2x < 8 ( divide each interval by 2 )
- 7 < x < 8
Given points A(-1,4) and B(x,7), determine the value(s) of x if AB=5cm
Answers
The value of x is either 3 or -5 based on the distance formula.
What is a co-ordinate system?
In pure mathematics, a coordinate system could be a system that uses one or additional numbers, or coordinates, to uniquely confirm the position of the points or different geometric components on a manifold like euclidean space.
Main body:
according to question
Given points A(-1,4) and B(x,7)
Also AB = 5 cm
Formula of distance = \(\sqrt{(y1-y2)^{2}+(x1 -x2)^{2} }\)
here by using points ,
5 = \(\sqrt{(x+1)^{2} +(7-4)^{2} }\)
taking square on both side ,'
25 = \((x+1)^{2} +3^{2}\)
25-9 = (x+1)²
16 = (x+1)²
taking square root on both sides,
x+1= ±4
x = 4-1 = 3 or x = -4-1 = -5
Hence value of x is either 3 or -5.
To know more about point , visit:
https://brainly.com/question/26310043
#SPJ1
Does anyone know the question to this ?? :(

Answers
Answer:
The ratio of the new area to the old area will be 16:1
Step-by-step explanation:
I remember learning this in class. The radius is quadrupled, which means the radius is 4 times bigger. This will make the new area 16 times bigger than the old area.
Let's check
Area of circle: 3.14 x r^2
Area of the circle when radius 6:
3.14 (6)^2 = 113.04 ft^2
Area of the new circle when the radius is quadrupled:
3.14 (24)^2 = 1808.64 ft^2
1808.64 divided by 113.04 = 16
So, the ratio of the new area to the old area will be 16:1
if the proportions of nests occupied is the same for golf and nongolf sites, what would be the expected count of birdhouses with 1 nest in nongolf locations?
Answers
The estimated number of birdhouses with one nest in non-golf locations would depend on the overall number of birdhouses in the non-golf locations if the proportion of nests occupied is the same for golf and non-golf sites.
Let's assume that there are a total of N birdhouses in the non golf locations, and that the proportion of nests occupied in both golf and non golf sites is p. The proportion of birdhouses with one nest in the non-golf locations would likewise be p since the proportions of nests occupied are the same for both locations.
As a result, the anticipated number of birdhouses with one nest in areas other than golf courses would be:
Expected count = proportion of birdhouses with 1 nest x total number of birdhouses
Expected count = p x N
So, if we know the value of p and N, we can calculate the expected count of birdhouses with 1 nest in nongolf locations.
To learn more about proportion follow the link:
https://brainly.com/question/12235587
#SPJ1
Answer:
1
Step-by-step explanation:
1=1
4/16 in reduced fraction
Answers
Answer:1/4
Step-by-step explanation:
Answer:
1/4th
Step-by-step explanation:
Q2
How
many cubic metres of earth must be dug to construct a well 7
m deep and of diameter 2.8 m?
Answers
Answer:
43.103m³
Step-by-step explanation:
This is just finding the volume of a cylinder problem.
Area of a circle = πr²
Area of circle * length of cylinder = volume
3.14*(1.4)² = 6.158m²
6.158*7 = 43.103m³
HURRY I NEED HELPPPP!!

Answers
Answer:
AB and GF
Step-by-step explanation:
The answer is AB and GF ;)
3) A moving target at a police academy target range can be hit 88% of the time by a particular individual. Suppose that as part of a training exercise, eight shots are taken at a moving target. a) What 3 characteristics of this scenario indicate that you are working with Bernoulli trials? b) What is the probability of hitting the 6
th
target (Hint: think of this as a single trial)? c) What is the probability that the first time hitting the target is not until the 4 th shot?
Answers
a. The probability of success (hitting the target) is constant for each trial (88% or 0.88).
b. The probability of hitting the 6th target is:
P(X = 1) = C(1, 1) * 0.88^1 * (1 - 0.88)^(1 - 1) = 0.88
c. Using the binomial probability formula as before, with p = 0.88 and n = 3:
P(X = 1) = C(3, 1) * 0.88^1 * (1 - 0.88)^(3 - 1)
P(X = 2) = C(3, 2) * 0.88^2 * (1 - 0.88)^(3 - 2)
P(X = 3) = C(3, 3) * 0.88^3 * (1 - 0.88)^(3 - 3)
a) The three characteristics of this scenario that indicate we are working with Bernoulli trials are:
The experiment consists of a fixed number of trials (eight shots).
Each trial (shot) has two possible outcomes: hitting the target or missing the target.
The probability of success (hitting the target) is constant for each trial (88% or 0.88).
b) To find the probability of hitting the 6th target (considered as a single trial), we can use the binomial probability formula:
P(X = k) = C(n, k) * p^k * (1 - p)^(n - k)
where:
P(X = k) is the probability of getting exactly k successes,
C(n, k) is the binomial coefficient or number of ways to choose k successes out of n trials,
p is the probability of success in a single trial, and
n is the total number of trials.
In this case, k = 1 (hitting the target once), p = 0.88, and n = 1. Therefore, the probability of hitting the 6th target is:
P(X = 1) = C(1, 1) * 0.88^1 * (1 - 0.88)^(1 - 1) = 0.88
c) To find the probability that the first time hitting the target is not until the 4th shot, we need to consider the complementary event. The complementary event is hitting the target before the 4th shot.
P(not hitting until the 4th shot) = P(hitting on the 4th shot or later) = 1 - P(hitting on or before the 3rd shot)
The probability of hitting on or before the 3rd shot is the sum of the probabilities of hitting on the 1st, 2nd, and 3rd shots:
P(hitting on or before the 3rd shot) = P(X ≤ 3) = P(X = 1) + P(X = 2) + P(X = 3)
Using the binomial probability formula as before, with p = 0.88 and n = 3:
P(X = 1) = C(3, 1) * 0.88^1 * (1 - 0.88)^(3 - 1)
P(X = 2) = C(3, 2) * 0.88^2 * (1 - 0.88)^(3 - 2)
P(X = 3) = C(3, 3) * 0.88^3 * (1 - 0.88)^(3 - 3)
Calculate these probabilities and sum them up to find P(hitting on or before the 3rd shot), and then subtract from 1 to find the desired probability.
Learn more about probability from
https://brainly.com/question/30390037
#SPJ11
3 and 4 I need help with so confusing

Answers
Answer: 3. (3,-3) and 4. (-3,4)
Step-by-step explanation: Number 4 is self explanatory of what they are and number 3, you need to figure out what minus 6 equals -3 and that happens to be 3.
The graph of f(x) and g(x) are shown below. How many solutions does the system of equations have?
Click pic to see whole problem

Answers
Answer:
Step-by-step explanation:
Solving systems of equations gives the points of intersection when the equations are graphed.
The answer is 3.
What is the surface area of the cylinder if the height is 11in and the radius is 4in?
Answers
Answer:
Around 376.99
Step-by-step explanation:
the formula is 2πrh+2πr²
so if we plug in everything we get 2π(4)(11)+2π(4)²
When we simplify that, we would get 376.99
Help :
(1 + 2 + 3 .... + 5) - 4 = ?
(1 + 2 + 3 .... + 4) - 1 = ?
(1 + 2 + 3) - 2 = ?
Interpret the answer with some logic
Answers
Answer:
whats ..... representating
is it a continuous series?
Plot the two points on the graph:(0,-4) and (-8,2)graph the line that goes through the two pointsFind the slope by drawing a slope triangle between the two points. Make sure it's simplifiedWrite the equation of the line in slope-intercept form

Answers
We are given the following two points
\((0,-4)\: \text{and}\: (-8,2)\)Let us plot these two points on the graph and draw a line through them.
Now let us find the slope by drawing a slope triangle between the two points.
As you can see, the rise (-6) is the vertical distance between the two points on the line.
The run (8) is the horizontal distance between the two points on the line.
So, the slope of the line is
\(slope=m=\frac{\text{rise}}{\text{run}}=\frac{-6}{8}=-\frac{3}{4}\)Write the equation of the line in slope-intercept form.
The slope-intercept form is given by
\(y=mx+b_{}\)Where m is the slope and b is the y-intercept of the line.
The y-intercept is the point where the line crosses the y-axis.
From the above graph, we see that the line crosses the y-axis at -4
So, the y-intercept is -4
Therefore, the equation of the line in slope-intercept form is
\(\begin{gathered} y=mx+b \\ y=-\frac{3}{4}x-4 \end{gathered}\)

You and your friend decide to sell cookies to raise money for a local fundraiser. After d days, you have raised (6d+7)dollars and your friend has raised (10d+10) dollars. Write an expression that represents the total amount of money that you raised together
Answers
So…
6d+7+10d+10
We add like terms which would be (7 and 10) and (6d and 10d)
7+10 is 17
6d+10d is 16d
So the answer would be
16d +17
If w divided by x = 3 what does x divided by w equal?
Help
Answers
The value of x/w is 1/3.
...........
Write an example from daily life that uses each type of real number.
rational numbers
Answers
Write an example from daily life that uses rational numbers .
Real numbers that may be expressed as p/q, where p, q are integers, and q 0, are rational numbers. The money we have is a rational number. If we spend some sum out of it, it is subtraction of rational number.The running race involves rational numbers if you're an athlete.Rational numbers include the following:the distance runthe time needed to complete the distance,the number of competitors placing first, second, or thirdthe number of heartbeats you take per minute.We use fractions to represent taxes.If you split a pizza or any other food.When you finish a piece of your work, you indicate that you have done half, or 50%.Loan and mortgage interest rates.Savings account interest.To learn more about rational numbers, refer:
https://brainly.com/question/12088221
#SPJ4
hii pls help i’ll give you brainliest if you give a correct answer.

Answers
Answer:
1. 19.375 inches taller
2. 8.808 km farther
Step-by-step explanation:
Answer:
Step-by-step explanation:
1.19.375
2.8.808
for the reasoning you just show that you subtracted
Solve the following expression when
f = 3
7 + 4 + f2 - 5f + 2
Answers
Answer:
7+4+2f-5f+2.
substitute the f.
7+4+2(3)-5(3)+2
Do the multiplication.
7+4+6-15+2.
11+6-15+2
17-15+2
2+2
4.
Help me please I’d really appreciate it right now .

Answers
Answer:
sales tax is $0.6
total cost is $20.60
Step-by-step explanation:
20*0.03=0.6
20*1.03=20.6
[15 points] Use Green's theorem to evaluate the line integral \[ \int_{C}\leftarctan x+y^{2}\right) d x+\left(e^{y}-x^{2}\right) d y \] where \( C is the path enclosing the annular region shown in the figure
Answers
The line integral of the given function can be solved using Green's theorem. Green's theorem helps to convert line integrals to double integrals of the region bounded by the path.
The line integral can be written as follows:
∫C Pdx + Qdy = ∬ R (Q_x - P_y) dA. ... (1)
Where R is the region enclosed by C, and P and Q are functions of x and y that have continuous partial derivatives in R. In this given function, P = x + y² and Q = e^y - x².The annular region enclosed by C can be divided into two regions by another simple closed curve as shown below. {drawing}By Green's theorem, we have:
∫C Pdx + Qdy = ∬ R (Q_x - P_y) dA. ... (1)
By evaluating (Q_x - P_y), we get, (Q_x - P_y) = -2x + 2y.We know that the double integral over the entire region enclosed by the two simple closed curves, C1 and C2 is equal to the difference of double integrals over the regions enclosed by these curves. Thus, the line integral over C can be written as follows:
∫C Pdx + Qdy = ∬ R1 (Q_x - P_y) dA - ∬ R2 (Q_x - P_y) dA = ∬ R (Q_x - P_y) dA,
where R = R1 - R2.By using the equation (1), the line integral of the given function over C can be expressed as follows:
∫C Pdx + Qdy = ∬ R (Q_x - P_y) dA = ∬ R (-2x + 2y) dA.
The double integral can be written as follows:
∬ R (-2x + 2y) dA = 2∬ R (y - x) dA = 2(∬ R y dA - ∬ R x dA).
The line integral of the given function can be solved using Green's theorem. Green's theorem helps to convert line integrals to double integrals of the region bounded by the path. By using Green's theorem, the given function's line integral over the simple closed curve C can be expressed in terms of a double integral over the annular region enclosed by C. The double integral can be evaluated by converting it into two integrals over two simple closed curves, C1 and C2, that enclose the annular region. These two curves intersect each other at two points, say A and B. These points divide the region into two regions, R1 and R2, where R1 is enclosed by C1 and R2 is enclosed by C2. The line integral over C can be written as the difference between the double integrals over R1 and R2. By solving these double integrals, we get the required line integral of the given function over the path C.
Therefore, by using Green's theorem, we can easily evaluate line integrals over simple closed curves. Green's theorem helps to convert line integrals to double integrals over the region bounded by the path. The double integrals can be evaluated by dividing the region into two regions enclosed by two simple closed curves. By evaluating the double integrals over these regions, we can easily evaluate the required line integral of the given function over the simple closed curve.
To learn more about Green's theorem visit:
brainly.com/question/32644400
#SPJ11
The solution of: 4.5x-100>125
Answers
Answer:
x>50
Step-by-step explanation:
I hope this helps you