What is the additive inverse of the complex number 9-412
-9-41
-9 +41
9 - 41
9 + 41
Answers
Additive inverse means the sum should be 0
so all you gotta do is multiply the complex number by -1
(the question isn't clear so I can't state the option)
Related Questions
Troy uses colored sand to make sand art. The storage container for his sand is shaped like a right square prism. He pours some of the sand into a display container shaped like a right triangular prism. When he is done, the height of the sand left in the storage container is 4 in. What is the height of the sand in the display container?

Answers
The height of the sand in the display container is 12 inches when the display container is right triangular prism.
What is right triangular prism ?
A right triangular prism is a three-dimensional geometric shape with two parallel triangular bases and three rectangular faces. The rectangular faces connect the corresponding vertices of the triangular bases.
To find the height of the sand in the display container, we need to use the fact that the volume of sand in the storage container is equal to the volume of sand in the display container.
The storage container has a length, width, and height of 6 inch, 6 inch, and 6inche, respectively. Then, the volume of the storage container is:
\(V_{storage} = L * W * H = 6 * 6 * 6 = 216 inch^3\)
Similarly, let's assume that the display container has a base of length 4 inch and height 9 inches and height of sand be H.
Then, the volume of the display container is:
\(V_{display} = (1/2) * b * h * H = (1/2) * 4 * 9 * H = 18H\)
where (1/2) is the area of the triangle base of the display container.
Since the volumes of sand in both containers are equal, we can set these expressions equal to each other and solve for h:
216 = 18H
H = 12 inches
Therefore, the height of the sand in the display container is 12 inches.
To learn more about volume from the given link :
https://brainly.com/question/22023329
#SPJ1
Order from least to greatest 1/3, 2/7, 3/14, 1/6
Answers
Answer:
1/6(0.16), 3/14(0.21), 2/7(0.28), 1/3(0.33)
Step-by-step explanation:
Your answer should be: \(\frac{1}{6} < \frac{3}{14} < \frac{2}{7} < \frac{1}{3}\)
Otherwise known as \(\frac{1}{6}, \frac{3}{14}, \frac{2}{7}, \frac{1}{3}\)
---------------------------------------------
Hope this helps!
Have a great day and God bless! :)
An environmental research firm wants to estimate the proportion of cars in Jackson County, Missouri that would be considered SUVs. Using the county's vehicle registration records they randomly select 220 vehicles. From that list they determined that 90 of them were SUVs. The sample proportion is 0.409, and the 95% confidence interval for the proportion of cars in Jackson County that are SUVs is (0.344, 0.474).
Select all of the following which would produce a confidence interval with a larger margin of error.
If you created a 90% confidence interval instead of the 95% confidence interval the margin of error would? Answer 1
If you had a random sample of 98 vehicles instead of 220 the margin of error would? Answer 2
If you created a 99% confidence interval instead of the 95% confidence interval the margin of error would?
Answer
If you had a random sample of 320 vehicles instead of 220 the margin of error would?
Answers
Answer:
If you had a random sample of 98 vehicles instead of 220.
If you created a 99% confidence interval instead of the 95% confidence interval.
Step-by-step explanation:
In a sample with a number n of people surveyed with a probability of a success of \(\pi\), and a confidence level of \(1-\alpha\), we have the following confidence interval of proportions.
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which
z is the zscore that has a pvalue of \(1 - \frac{\alpha}{2}\).
The margin of error is given by:
\(M = z\sqrt{\frac{\pi(1-\pi)}{n}}\)
This means that a higher confidence level(higher value of z) leads to a larger margin of error, and a larger sample(higher value of n) leads to a lower margin of error.
Select all of the following which would produce a confidence interval with a larger margin of error.
Higher confidence level or smaller sample. So the correct options are:
If you had a random sample of 98 vehicles instead of 220.
If you created a 99% confidence interval instead of the 95% confidence interval.
A 99% confidence interval for the mean sugar content of a shipment of strawberries is (5.9, 8.3) grams per 5 oz. serving. Which of the following is the best interpretation of the 99% confidence level for the mean sugar content of the shipment. (A) There is a 99% probability that the interval 5.9 to 8.3 has captured the true mean sugar content of the shipment. (B) There is a 99% probability that the true mean sugar content of the strawberries in the shipment is between 5.9 and 8.3 grams. (C) If we constructed many, many confidence intervals from random samples of this size, 99% of the intervals we constructed would capture the sample mean. (D) If we constructed many, many confidence intervals from random samples of this size, 99% of the intervals we constructed would capture the population mean.
Answers
The best interpretation of the 99% confidence level for the mean sugar content of the shipment is given as follows:
(D) If we constructed many, many confidence intervals from random samples of this size, 99% of the intervals we constructed would capture the population mean.
What is the interpretation of a x% confidence interval?It means that it is x% likely that the population parameter(mean/proportion/standard deviation) is between a and b, which are the bounds of the confidence interval.
This also means that over a large number of intervals, x% would contain the population parameter.
More can be learned about confidence intervals at https://brainly.com/question/17097944
#SPJ1
3 2/6+8 2/9-1 1/2 ?????????
Answers
Answer:
10 1/18
Step-by-step explanation:
3 2/6 + 8 2/9 - 1 1/2 = ?
First, we need to find the common denominator, which I will choose to be 18, which means I will need to convert all the fractions.
3 6/18 + 8 4/18 - 1 9/18 = ?
Now, we solve.
3 6/18 + 8 4/18 - 1 9/18 = 10 1/18
I will mark brainliest pls help

Answers
Answer:
15
Step-by-step explanation:
You can buy 20 pens for $8 or 30 pens for $10. Which is the better deal?
Answers
Answer: It would be the first one (20 pens for 8$)
Step-by-step explanation: If you divide 20 by 8 then each pencil will be 2.50$. However if you divide 30 by 10 it will be 3 dollars for each pencil so the first deal will be better since you spend less money.
emisphere is placed on top of a cylinder with the same radius. The heig sed in.

Answers
When given this kind of composite figures, what we have to do is split it into individual figures. In this case, we would have a cylinder and half a sphere.
For the cylinder:
Remember that the formula for the volume of a cylinder is:
\(V_c=\pi r^2h\)Where r is the radius of the base and h is the height of the cylinder.
Using this and the data given, we get that:
\(V_c=\pi(3^2)(10)\rightarrow V_c=282.74\)The volume of the cyllinder is 282.74 cubic inches.
For the semisphere:
Remember that the formula for the volume of a sphere is:
\(V=\frac{4}{3}\pi r^3\)Now, notice that we have half a sphere, so the formula we'll use is:
\(V_s=\frac{4}{6}\pi r^3\)This way,
\(V_s=\frac{4}{6}\pi(3^3)\rightarrow V_s=56.55\)Adding up both volumes,
\(282.74+56.55=339.29\)This way, the volume of the composite figure, rounded to the nearest hundredth is 336.29 cubic inches
Determine the indicated probability for a binomial experiment with the given number of trials n and the given success probability p. Round your final answer to three decimal places. Intermediate calculations should be rounded to a minimum of four places. n = 15, p = 0.4 a. Find P(2). Round to three decimal places. b. Find P(2 or fewer). Round to the three places.
Answers
a. The value of P(2) is 0.022
b. The value of P(2 or fewer) is 0.027
From the question; n = 15, p = 0.4
a. We have to determine P(2).
P(X = x) = ⁿCₓ·Pˣ·(1 - P)ⁿ⁻ˣ
P(X = 2) = ¹⁵C₂·(0.4)²·(1 - 0.4)¹⁵⁻²
We can write ⁿCₓ = \(\frac{n!}{x!(n - x)!}\)
P(X = 2) = \(\frac{15!}{2!(15 - 2)!}\) · (0.16) · (0.6)¹³
P(X = 2) = \(\frac{15\times14\times13!}{2\times1\times13!}\) · (0.16) · (0.6)¹³
P(X = 2) = (15 × 7) · (0.16) · (0.6)¹³
After simplification
P(X = 2) = 0.022(approx)
b. We have to determine P(2 or fewer).
P(x ≤ 2) = P(x = 0) + P(x = 1) + P(x = 2)
P(x ≤ 2) = ¹⁵C₀·(0.4)⁰·(1 - 0.4)¹⁵⁻⁰ + ¹⁵C₁·(0.4)¹·(1 - 0.4)¹⁵⁻¹ + ¹⁵C₂·(0.4)²·(1 - 0.4)¹⁵⁻²
After simplification like above
P(x ≤ 2) = 0 + 0.005 + 0.022
P(x ≤ 2) = 0.027
To learn more about binomial experiment link is here
brainly.com/question/15351475
#SPJ4
ali graphed -3 and 4 on the number line. which is further from 0? explain.
Answers
Answer:
4
Step-by-step explanation:
because if you count how many numbers are from 0-4 you get 4 but if you count from 0 to -3 youget three
Answer:
the answer for this question is 4
Step-by-step explanation:
write and solve an equation to find the value of x so the graph is 161 fegree its like the 90 percent degree and there a x
Answers
The equation for x is:
\(x+161=180\)solving for x we have:
\(\begin{gathered} x=180-161 \\ x=19 \end{gathered}\)Therefore, x=19°
Help fast plz 100 points!

Answers
No because root(3)(x+8+1) is equal to x^3 -9 and (x-1)^3+8 is equal to root (3)(√x−8−1)
Answer:
yes!! brainliest please?
Step-by-step explanation:
Hello everyone-SOLVING nonlinear system of equations- ALGEBRA 1

Answers
The solution to the nonlinear system of equations is (x, y) = (-3, -2) and (x, y) = (1, 6). These points represent the coordinates where the two equations intersect and satisfy both equations simultaneously.
To solve the nonlinear system of equations:
Equation 1: \(y = -x^2 + 7\)
Equation 2: y = 2x + 4
We can equate the right sides of both equations since they both represent y.
\(-x^2 + 7 = 2x + 4\)
To simplify the equation, we can rearrange it to be in the standard quadratic form:
\(x^2 + 2x - 3 = 0\)
Now, we can solve this quadratic equation by factoring, completing the square, or using the quadratic formula. In this case, let's use factoring:
(x + 3)(x - 1) = 0
From this equation, we get two possible solutions:
x + 3 = 0 => x = -3
x - 1 = 0 => x = 1
Now, we substitute these x-values back into either equation to find the corresponding y-values.
For x = -3:
y = 2(-3) + 4
y = -6 + 4
y = -2
For x = 1:
y = 2(1) + 4
y = 2 + 4
y = 6
For more such questions on coordinates
https://brainly.com/question/29660530
#SPJ8
Find the height of the tower using the information given in the illustration.

Answers
using SOH CAH TOA
Tan 85.144 =h/130
h=tan 85.144*130
h=1530.19 fr

Given that a+b = 10 and a square - b square = 40 find the value of a-b
Answers
Answer:
the value of a - b is 4.
Step-by-step explanation:
We have been given the following two equations:
a + b = 10 ------------(1)
a² - b² = 40 -------(2)
We can factor the left-hand side of equation (2) using the difference of squares identity:
(a + b)(a - b) = 40
Substituting equation (1) into this equation, we get:
10(a - b) = 40
Dividing both sides by 10, we get:
a - b = 4
Therefore, the value of a - b is 4.
Step-by-step explanation:
if I understand this correctly :
a + b = 10
a² - b² = 40
(a² - b²) = (a + b)(a - b) = 40
10(a - b) = 40
(a - b) = 4
1.
State the missing steps and reasons to this solution of 3(x + 4) = 18.
a) 3(x + 4) = 18
b)
c) 3x + 12 - 12 = 18 - 12
d) 3x + 0 = 18 - 12
e) 3x = 18 - 12
f
h) 1x
IN
) X=2
Answers
Answer: B) 3x+12=18; Distributive Property
F) 3x=6; Simplify
Step-by-step explanation:
A group of college students is volunteering for Help the Homeless during their spring break. They are putting the finishing touches on a house they built. Working alone, Irina can paint a certain room in 9 hours. Paulo can paint the same room in 8 hours. Write an equation that can be used to find how long it will take them working together to paint the room. How many hours will it take them to paint the room? If necessary, round your answer to the nearest hundredth.
A. \(\frac{x}{9} + \frac{x}{8} = 1\); 2.18 hours
B. \(\frac{1}{9} + \frac{1}{8} = \frac{1}{x}\); 3.73 hours
C. \(\frac{9}{x} + \frac{8}{x} = 1\); 8.5 hours
D. \(\frac{x}{9} + \frac{x}{8} = 1\); 4.24 hours
Answers
Answer:
D 4.24
Step-by-step explanation:
Assume the area of room be A
Irina painting speed be x, 8x = A
Paulo painting speed be y, 9y = A
Together painting speed = x + y = A/8 + A/9
Assume together painting time = t
(A/8 + A/9) t = A
(1/8 + 1/9)t = 1
17t = 72
t = 4.24
what is 10 + 8x = 10003
Answers
Answer:
Step-by-step explanation:
10 + 8x =10003
8x = 10003 - 10
8x = 9993
X = 9993 ÷ 9
Step-by-step explanation:
Rearrange = 10 + 8 x =10003
8x + 10 = 10003
subtract both= 8x + 10 = 10003
8x + 10 -10= 10003-10
simplify= subtract the number 8x = 999
Divide the both number = 8x = 9993
8x/8 =993/8
And them simplify it again = x = 9993/8
What’s the probability that a randomly selected sheet will be blue if there are only 2 out of 12?
Answers
Answer:1/6
Step-by-step explanation:
Which graph represents the solution set to the system of inequalities?
{ Y ≤ 1/4X-2
Y ≥ −54X+2
ANSWER Down Below
Answers
The graph of the system of inequalities:
y ≤ (1/4)*x - 2
y ≥ −(5/4)x +2
Is in the image at the end.
Which is the graph of the system of inequalities?Here we have the system of inequalities:
y ≤ (1/4)*x - 2
y ≥ −(5/4)x +2
To graph this, we just need to graph both of the linear equations, and we need to shade the region below the first line (the one with positive slope) and the region above the second line, the one with negative slope.
Then the graph of the system of inequalities is the graph you can see in the image at the end of the answer.
Learn more about system of inequalities at.
https://brainly.com/question/9774970
#SPJ1

Help I don’t understand at all

Answers
Answer:
\(1)\ \ 4h^2-13h+6\\2)\ \ 7x^3y^2-x^2y+1\\3)\ \ -7n+2\\4)\ \ -8m+4\)
Step-by-step explanation:
1.
Simplify the expression by combining like terms. Remember, like terms have the same variable part, to simplify these terms, one performs operations between the coefficients. Please note that a variable with an exponent is not the same as a variable without the exponent. A term with no variable part is referred to as a constant, constants are like terms.
\(2h^2-7h+2h^2-h+6+4h-9h\)
\((2h^2+2h^2)+(-7h-h+4h-9h)+(6)\)
\(4h^2-13h+6\)
2.
Use a very similar method to solve this problem as used in the first. Please note that all of the rules mentioned in the first problem also apply to this problem; for that matter, the rules mentioned in the first problem generally apply to any pre-algebra problem.
\(8x^3y^2-7x^2y+8x-4-x^3y^2+2x^2y+4x^2y-8x+5\)
\((8x^3y^2-x^3y^2)+(-7x^2y+2x^2y+4x^2y)+(8x-8x)+(-4+5)\)
\(7x^3y^2-x^2y+1\)
3.
Use the same rules as applied in the first problem. Also, keep the distributive property in mind. In simple terms, the distributive property states the following (\(a(b+c)=(a)(b)+(a)(c)=ab+ac\)). Also note, a term raised to an exponent is equal to the term times itself the number of times the exponent indicates. In the event that the term raised to an exponent is a constant, one can simplify it. Apply these properties here,
\(-2(8n+1)-(5-9n)+3^2\)
\(-2(8n+1)-(5-9n)+(3*3)\)
\(-2(8n+1)-(5-9n)+9\)
\((-2)(8n)+(-2)(1)+(-)(5)+(-)(-9n)+9\)
\(-16n-2-5+9n+9\)
\((-16n+9n)+(-2-5+9)\)
\(-7n+2\)
4.
The same method used to solve problem (3) can be applied to this problem.
\(\frac{1}{2}(10-8m+6m^2)-(3m^2+4m-7)-2^3\)
\(\frac{1}{2}(10-8m+6m^2)-(3m^2+4m-7)-(2)(2)(2)\)
\((\frac{1}{2})(10)+(\frac{1}{2})(-8m)+(\frac{1}{2})(6m^2})+(-)(3m^2)+(-1)(4m)+(-1)(-7)-8\)
\(5-4m+3m^2-3m^2-4m+7-8\)
\((-3m^2-3m^2)+(-4m-4m)+(5+7-8)\)
\(-8m+4\)
Match the function with its graph.
1)y=-csc x
2)y=-sec x
3)y=-tan x
4)y=-cot x
Answers
Answer:
The answer is B
Step-by-step explanation:
1B, 2D, 3C, 4A
on Edge this is the correct answer
Brainliest would be awesome
Does anyone know how to do this?

Answers
Answer:
A
Step-by-step explanation:
\(\dfrac{f(x)}{g(x)}=\dfrac{x+1}{x^{2}-x}\\f(x) = \dfrac{x+1}{x^{2}-x}*g(x)\\\\\dfrac{2}{x}*\dfrac{x^{2}-x}{x+1}=g(x)\\\\\\\dfrac{2}{x}*\dfrac{x(x-1)}{x+1}=g(x)\\\\\\\dfrac{2(x-1)}{x+1}=g(x)\\\\\\)
help with horizontal asymptotes


Answers
Answer:
y = 3. last option is correct answer for 2nd question.
Step-by-step explanation:
f(x) just means y.
horizontal asymptote is y = 'something.'
the graph crosses y = 4, so y = 4 cannot be asymptote.
y = 3 is since the graph never quite touches it.
as for ∞, we see that as x approaches -∞, y almost reaches 3. but not quite. also, as x approaches +∞, y approaches +∞.
so the last option is the correct answer.
What is the translation from
a) shape X to shape Z?
b) shape Z to shape X?

Answers
The translation form of each shape is given as follows:
a) X to Z: (x, y) -> (x + 5, y + 2).
b) Z to X: (x, y) -> (x - 5, y - 2).
What are the translation rules?The four translation rules are defined as follows:
Left a units: x -> x - a.Right a units: x -> x + a.Up a units: y -> y + a.Down a units: y -> y - a.For the composition of translations, we add the coordinates, hence:
-2 + 7 = 5.5 - 3 = 2.Hence the rule from X to Z is given as follows:
(x, y) -> (x + 5, y + 2).
From Z to X, we have the inverse rule, hence:
(x, y) -> (x - 5, y - 2).
More can be learned about translation at brainly.com/question/29209050
#SPJ1
may i please get help for this please and thank you
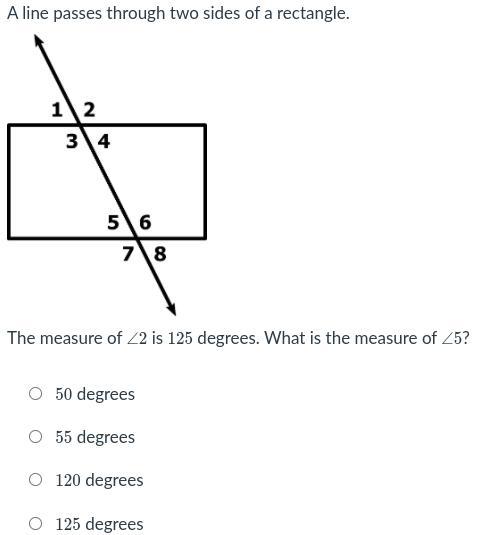
Answers
Because 2= 125 that means that 7 is also 125 and 6 so you subtract 125-180= 55
HELP HELP I WILL GIVE ALL MY POINTS JUST HELP ME PLEASE

Answers
Answer:
A
Step-by-step explanation:
x = 0
y = -3
Answer:
My best bet is (0,-3) sorry if I'm wrong
1.5.2For the telephone usage model of Example 1.18, let Bm denote the event that a call is billed for m minutes. To generate a phone bill, observe the duration of the call in integer minutes (rounding up). Charge for M minutes M = 1,2,3,... if the exact duration T is M-1
Answers
(a) The probability that a call is classified as long (L) is \($P[L] = 1 - P[B_3] = 1 - \alpha (1-\alpha)^2 = 0.829$.\)
(b) The probability that a call will be billed for nine minutes or less is
\(P[B1] + P[B2] + P[B3] + P[B4] + P[B5] + P[B6] + P[B7] + P[B8] + P[B_9] = \alpha + \alpha(1-\alpha) + \alpha(1-\alpha)^2 + \alpha(1-\alpha)^3 + \alpha(1-\alpha)^4 + \alpha(1-\alpha)^5 + \alpha(1-\alpha)^6 + \alpha(1-\alpha)^7 + \alpha(1-\alpha)^8 = 0.958\)
Probability is a branch of mathematics that deals with the likelihood of an event occurring. It is expressed as a number from 0 to 1, with 0 indicating that an event is impossible and 1 indicating that it is certain to occur. Probability is used to calculate the chance of a random event happening or to predict the outcome of a situation. Probability theory is also used to describe the underlying mechanics and dynamics of random phenomena
Learn more about probability:
https://brainly.com/question/24756209
#SPJ4
Complete question:
For the telephone usage model of Example 1.18, let \($B_m$\) denote the event that a call is billed for $m$ minutes. To generate a phone bill, observe the duration of the call in integer minutes (rounding up). Charge for M minutes \($M=1,2,3, \ldots$\) if the exact duration T is \($M-1 < t \leq M$\). A more complete probability model shows that for $m=1,2, \ldots$ the probability of each event \($B_m$\) is
\($$P\left[B_m\right]=\alpha(1-\alpha)^{m-1}$$\)
where \($\alpha=1-(0.57)^{1 / 3}=0.171$\)
(a) Classify a call as long, L, if the call lasts more than three minutes. What is \($P [L]$\) ?(b) What is the probability that a call will be billed for nine minutes or less?Pls Help! Worth 8 Points!

Answers
- BRAINLIEST answerer
Step-by-step explanation:
Hope it helps....
Can you give me BRAINLIEST ANSWER
isabel drove 335 miles in 5 hours at the same rate how any miles would she drive in 8 hours
Answers
335/5=67
Therefore, if she was to drive eight hours she would drive 536 miles
Answer=536 miles