wendy earns a 7 and 5/8% commission on her total sales, She had a total of $10,524.68 in sales. How much commission did Wendy earn?
Answers
Answer:
The amount of commission earned by Wendy is $6,577.93
Step-by-step explanation:
Commissions can be described as a type of variable remuneration that is paid to an employee based on the value of services rendered or products sold.
Commission is calculated by multiplying the commission rate by the value of services rendered or products sold.
From the question, we have:
Fixed earnings = $7
Commission rate = 5/8% = 0.625%
Total sales = $10,524.68
Amount of commission = Commission rate * Total sales = 0.625% * $10,524.68 = $6,577.93
Therefore, the amount of commission earned by Wendy is $6,577.93.
Note that the fixed earning of $7 will be earned by Wendy whether she made sales or not. Therefore, the fixed earning is not part of the commission and should not be added to the commission.
Related Questions
A garden is to designed with a rectangular part in the middle with two semi-circles on the ends.
The dimensions of the rectangular portion are 18.4 feet long and 8.6 feet wide.
a) What is the area of one semi-circle at one end?
b) What is the area of the garden?
c) Find the area in square metres.
Answers
Given statement solution is :- a) The area of one semi-circle at one end is 58.09 square feet.
b) The area of the garden is 274.42 square feet.
c) The area in square metres is approximately 58.09 square feet.
The area of the garden is approximately 274.42 square feet, and the area in square meters is approximately 25.49 square meters.
a) To find the area of one semi-circle at one end, we need to calculate the area of a complete circle and then divide it by 2. The formula for the area of a circle is A = πr², where A represents the area and r is the radius.
Since the diameter of the semi-circle is equal to the width of the rectangular portion, which is 8.6 feet, the radius will be half of that, which is 8.6 / 2 = 4.3 feet.
Now we can calculate the area of the semi-circle:
A = (π * 4.3²) / 2
A ≈ 58.09 square feet
b) To find the area of the garden, we need to sum the area of the rectangular portion with the areas of the two semi-circles.
Area of the rectangular portion = length * width
Area of the rectangular portion = 18.4 feet * 8.6 feet
Area of the rectangular portion ≈ 158.24 square feet
Area of the two semi-circles = 2 * (area of one semi-circle)
Area of the two semi-circles ≈ 2 * 58.09 square feet
Area of the two semi-circles ≈ 116.18 square feet
Total area of the garden = area of the rectangular portion + area of the two semi-circles
Total area of the garden ≈ 158.24 square feet + 116.18 square feet
Total area of the garden ≈ 274.42 square feet
c) To convert the area from square feet to square meters, we need to know the conversion factor. Since 1 foot is approximately 0.3048 meters, we can use this conversion factor to convert the area.
Area in square meters = Total area of the garden * (0.3048)²
Area in square meters ≈ 274.42 square feet * 0.3048²
Area in square meters ≈ 25.49 square meters
Therefore, the area of one semi-circle at one end is approximately 58.09 square feet. The area of the garden is approximately 274.42 square feet, and the area in square meters is approximately 25.49 square meters.
For such more questions on Garden Area Calculations
https://brainly.com/question/2516802
#SPJ8
What should you substitute for x in the second equation (bottom equation) in order to solve the system by the substitution method?

Answers
We substitute -1+1/6y for x in the second equation (bottom equation) in order to solve the system by the substitution method.
The given system of equations are 2x-1/3y = -2...(1)
3x+y=1...(2)
We solve this system of equations by using substitution method.
Let us solve for x in equation to substitute it in equation (2).
2x=-2+1/3y
Divide both sides of equation by 2.
x=-1+1/6y
Now plug in the value of x in equation 2.
Hence, we substitute -1+1/6y for x in the second equation (bottom equation) in order to solve the system by the substitution method.
To learn more on Equation:
https://brainly.com/question/10413253
#SPJ1
A goat is tired to a pole at the center of a square of length of side 20m. If the rope is 10m long. Calculate the (I) area of the square that can't be grazed by the goat (ii) fraction of grazed portion to the whole area
Answers
Answer:
percentage = 78.54%
Step-by-step explanation:
The area of a square of side length x is:
\(A_s=x^2\)
The area of a circle of radius r is:
\(A_c=\pi r^2\)
The goat is tied to a pole at the center of a square of a side length of x=20 cm.
The area of the square is:
\(A_s=20^2\)
\(A_s=400~m^2\)
The rope is 10 m long. The goat is able to graze an area of:
\(A_c=\pi 10^2\)
\(A_c=100\pi~m^2\)
(i)
Since the diameter of the circle (20 m) is equal to the length of the square, the area of the circle is contained in the square and the area of the square not grazed by the goat is:
\(A = A_s-A_c=400~m^2-100\pi~m^2\)
\(A=100(4-\pi)~m^2\)
Calculating:
\(A = 85.84~m^2\)
(ii)
The percentage can be calculated as:
\(\displaystyle \frac{A_c}{A_s}*100=\frac{100\pi}{400}*100=25\pi\)
Calculating:
percentage = 78.54%
Write an equation of the line that passes through the point (4, –5) with slope 2.
A. y−4=2(x+5)
B. y−4=−2(x+5)
C. y+5=2(x−4)
D. y+5=−2(x−4)
Answers
After answering the provided question, we can conclude that The slope answer is not listed as a choice, but this is the correct equation.
what is slope?In mathematics, slope is the slope of the regression of a line or curve. It is a measure of the degree to which a function's y-value varies once the x-value changes. A line's slope is usually expressed as a letter m and is able to be calculated as follows: m = (y2 - y1) / (x2 - x1) (x1, y1) and (x2, y2) is some two important points on the line. The slope of a line can be favorable, negative, equal to 0, or unknown. A positive slope means the line rises up from left to right, whereas a slope means the line falls back from left to right.
To find the equation of the line passing through the point (4,-5) with slope 2, we can use the point-slope form of the equation of a line.
y - y1 = m(x - x1)
y - (-5) = 2(x - 4)
y + 5 = 2x - 8
y = 2x - 13
y = 2x - 13
The answer is not listed as a choice, but this is the correct equation.
To know more about slope visit:
https://brainly.com/question/3605446
#SPJ1
Barbara buys
3 boxes of eggs costing £1.20 each
1 jar of mayonnaise costing £1.80
2 loaves of bread
She pays with a £10 note and gets £1.60 change.
Barbara works out the cost of 1 loaf of bread as £1.40
Is she correct?
You must show your working.
Answers
Answer:
Actually, a loaf of bread costs 1.5. Barbara was wrong but only by 10 pence
Step-by-step explanation:
1 box of eggs = 1.2
3 boxes of eggs =3*1.2=3.6
1 jar of mayonnaise =1.8
2 loves of bread=2x
Total price of her purchases =10-1.6=8.4
Setting up the equation:
3.6+1.8+2x=8.4
Solving for x
2x=8.4-3.6-1.8
2x=3
x=3/2=1.5
Which of the following is a perfect square trinomial?
a. x2 + 10x + 25
b. x2 + 5x + 10
c. XP + 10x + 20
d. x2 + 10x + 50
Answers
Answer:
a. x2 + 10x + 25 should be the correct one
If b = -2, what is 3b-7 ?
Answers
3 x -2 = -6
-6 -7 = -13
Write this in a Algebraic expression. (Use x as your variable) The sum of x squared and y
Answers
Answer:
x^2+y
Step-by-step explanation:
simply because you have x squared and a variable y that needs to be added.
The temperature during the day is 5.4 Celsius, after sunset the temperature drops 7.5 Celsius. Wind chill makes the temperature after sunset feel 6.3 Celsius colder. What temperature does it feel like after sunset
Answers
Temperature during the day = 5.4 C
After sunset drops = 7.5 C
5.4 - 7.5 = -2.1 C
Wind chill drops 6.3 C
-2.1 - 6.3 = -8.4°C
Two integers , c and d , have a product of -6. What is the greatest possible sum of c and d?
Answers
-6 * 1 = -6
-1 * 6 = 6
-2 * 3 = -6
-3 * 2 = -6
now, we can replace the * sign with an addition sign to determine what the greatest sum will be.
-6 + 1 = -5
-1 + 6 = 5
-2 + 3 = 1
-3 + 2 = -1
the expression -1 * 6 will create the greatest sum of 5.
hope this helps!
Based on a weather record, the probability of snowfall in a certain town in New York on January 1 is 0.230. Find the probability that next year there will be no snowfall in that town on January 1.
1. 0.770
2. 4.348
3. 0.299
4. 1.230
Answers
The probability that next year there will be no snowfall in that town on January 1 is,
P (no snowfall) = 0.770
The term probability refers to the likelihood of an event occurring. Probability means possibility. It is a branch of mathematics that deals with the occurrence of a random event. The value is expressed from zero to one.
We have to given that;
Based on a weather record, the probability of snowfall in a certain town in New York on January 1 is 0.230.
Now, WE get;
the probability that next year there will be no snowfall in that town on January 1 is,
P (no snowfall) = 1 - P (snowfall)
P (no snowfall) = 1 - 0.230
P (no snowfall) = 0.770
Thus, The probability that next year there will be no snowfall in that town on January 1 is,
P (no snowfall) = 0.770
Learn more about the probability visit:
https://brainly.com/question/13604758
#SPJ1
Find the number of playing cards in a standard deck of 52 cards that are either black cards or cards with even numbers on them.
Answers
There are 36 cards in the deck of cards that are either black or even number .
A deck of cards consists of 52 cards equally divided into 4 suits: clubs , hearts , spades and diamond.
Clubs and spades are black in color and diamond and heart is red .Each suit has 13 cards : 9 are number cards 2 to 10 and 4 face cards ace, jack, queen and king. The deck of cards is widely used in the field of probability to understand various conceptsNow even numbers are numbers that can be divided by 2 completely .
So according to the given statement:
number of black cards = 13 spades + 13 clubs = 26 cards
even number cards ={2 ,4 ,6 , 8,10} = 5 cards in each suit
Therefore in the hearts and diamonds there are 5 × 2 =10 cards.
Therefore the total number of cards that are black or even is :
26 + 10 = 36
To learn more about probability in a deck of cards visit:
https://brainly.com/question/19202591
#SPJ1
Each figure shows a triangle with one of its angle bisectors
Pls help asap

Answers
The measures of the required angles formed by the angle bisectors are found by using the angle addition property and angle bisector are as follows;
15) x = 9
16) x = 2
17) x = 6
18) x = 4
What is an angle bisector?An angle bisector is a ray that divides an angle into two equal angles.
15) The given parameters are;
m∠2 = 2·x - 3
∠GEF = 2·x + 12
∠GEF = m∠1 + m∠2; angle addition postulate
m∠1 = m∠2 definition of angle formed by the bisecting segment EP
Which gives;
∠GEF = m∠1 + m∠2 = ∠GEF = m∠2 + m∠2 = 2 × m∠2
Plugging in the values of ∠GEF and m∠2, gives;
2·x + 12 = 2×(2·x - 3) = 4·x - 6
2·x + 12 = 4·x - 6
4·x - 2·x = 12 + 6 = 18
2·x = 18
x = 18 ÷ 2 = 9
x = 916) Given m∠1 = -1 + 16·x
m∠XYZ = 31·x
m∠XYZ = m∠1 + m∠2 angle addition postulate
m∠1 = m∠2 angles formed by the bisector line XP
Therefore, m∠XYZ = 2 × m∠1
Which gives; 31·x = 2 ×(-1 + 16·x) = -2 + 32·x
32·x - 31·x = x = 2
x = 217) m∠1 = 9·x - 6
∠XVW = 17·x - 6
∠XVW = m∠1 + m∠2
m∠1 = m∠2
∴ ∠XVW = 2 × m∠1
17·x - 6 = 2×( 9·x - 6) = 18·x - 12
18·x - 17·x = x = 12 - 6 = 6
x = 618) m∠1 = 6·x + 6
m∠CAB = 16·x - 4
m∠CAB = m∠1 + m∠2
m∠1 = m∠2
m∠CAB = 2 × m∠1
∴ 16·x - 4 = 2 × (6·x + 6) = 12·x + 12
16·x - 4 = 12·x + 12
16·x - 12·x = 12 + 4 = 16
4·x = 16
\(x = \dfrac{16}{4} = 4\)
x = 4Learn more about angle bisectors here:
https://brainly.com/question/21752287
#SPJ1
What is the starting term of 64?
Answers
Answer:
1
Step-by-step explanation:
One is the starting term
1, 2, 4, 8, 16, 32, 64, 128, 256,
The first few steps in solving the quadratic equation 9x2 + 49x = 22 − 5x by completing the square are shown. 9x2 + 49x = 22 − 5x 9x2 + 54x = 22 9(x2 + 6x) = 22 Which is the best step to do next to solve the equation by completing the square? 9(x2 + 6x + 3) = 25 9(x2 + 6x + 3) = 49 9(x2 + 6x + 9) = 31 9(x2 + 6x + 9) = 103
Answers
Answer:
The Answer is : 9(x2 + 6x + 9) = 103
Step-by-step explanation:
Option D on edge2020 i got a 100 on the quiz
The resulting quadratic equation is \((x+3)^2-103/9=0\)
Quadratic equationGiven the quadratic equation \(9x^2 + 49x = 22 - 5x\)
Write in standard form:
\(9x^2 + 49x = 22 - 5x\\ 9x^2 + 49x + 5x -22= 0\\ 9x^2+54x-22=0\)
Divide through by 9
\(x^2+6x-22/9=0\\ \)
complete the square to have:
\(x^2+6x-22/9+3^2-3^2=0\\ x^2+6x+3^2-9-22/9 = 0\\ (x+3)^2-103/9=0\)
Hence the resulting quadratic equation is \((x+3)^2-103/9=0\)
Learn more on completing the square here: https://brainly.com/question/1596209
I need help urgent plz someone help me solved this problem! Can someone plz help I’m giving you 10 points! I need help plz help me! Will mark you as brainiest!
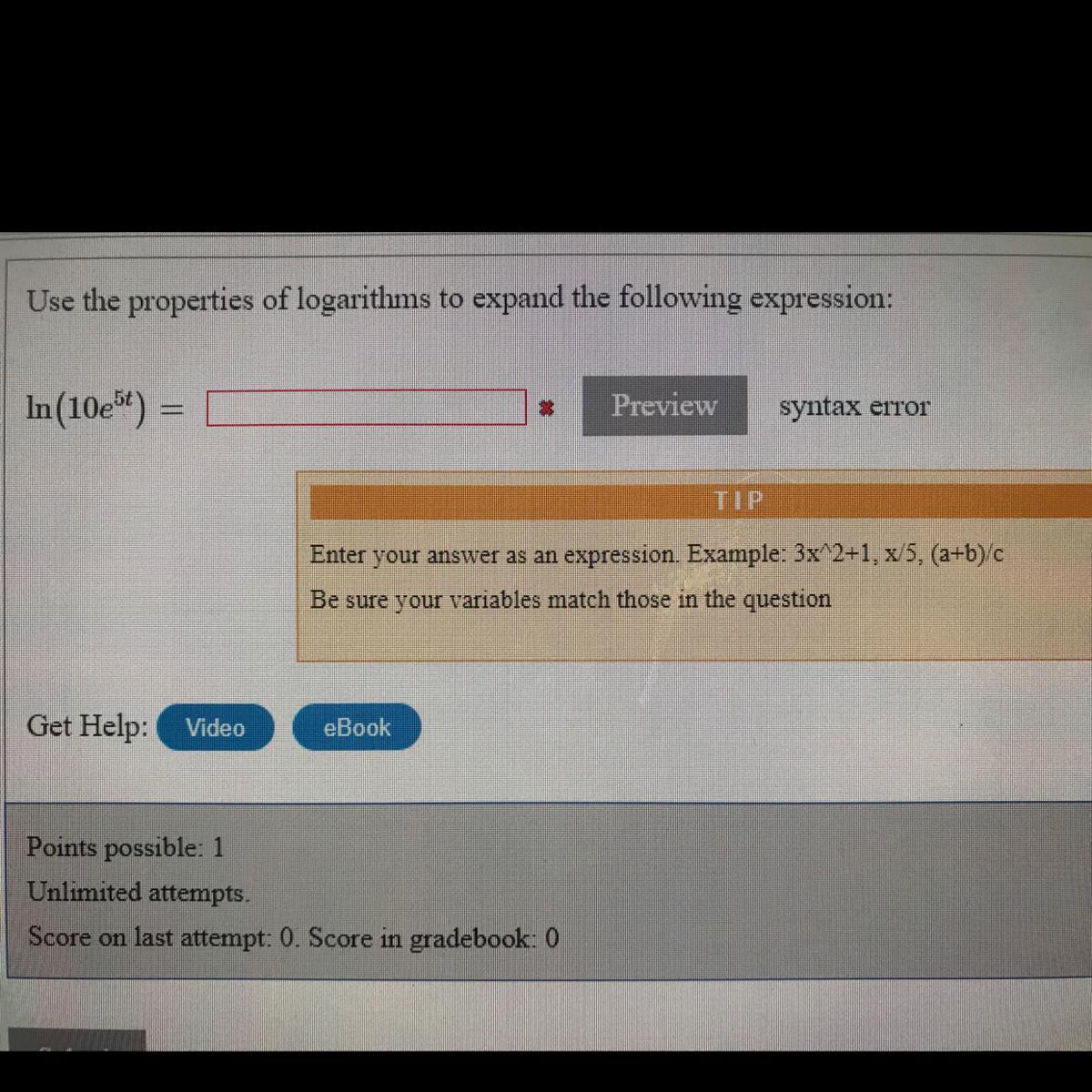
Answers
Answer: ln 20 + 5t
Step-by-step explanation:
ln (ab) = lna + lnb
ln (10e⁵ˣ) = ln 10 + ln e⁵ˣ → (ln e cancels out)
= ln 10 + 5t
El mayor es Novecientos mil cuatrocientos ochenta y nueve , y cuarenta mil dos
Answers
El número "Novecientos mil cuatrocientos ochenta y nueve" se representa como 900,489 en notación numérica.
Por otro lado, el número "cuarenta mil dos" se representa como 40,002.
Si estamos buscando determinar cuál de estos dos números es mayor, podemos comparar las cifras en cada posición.
Comenzando desde la izquierda, el primer dígito de 900,489 es 9, mientras que el primer dígito de 40,002 es 4.
Dado que 9 es mayor que 4, podemos concluir que 900,489 es mayor que 40,002.
En general, al comparar números, se debe observar cada posición en orden de mayor a menor importancia.
Esto significa que el primer dígito a la izquierda es el más significativo y tiene más peso en el valor total del número.
Si los dígitos en la posición más significativa son iguales, se debe pasar a la siguiente posición hasta que se encuentre una diferencia.
En este caso, dado que el primer dígito de 900,489 es mayor que el primer dígito de 40,002, no es necesario comparar los dígitos en posiciones posteriores.
Por lo tanto, podemos concluir que el número "Novecientos mil cuatrocientos ochenta y nueve" (900,489) es mayor que "cuarenta mil dos" (40,002).
For similar questions on Novecientos
https://brainly.com/question/1753221
#SPJ8
write 7x+9 in fractional form
Answers
The solution of the equation 7x+9 =11 as a fraction in its simplest form is x = 2/7
Simple fraction is in the form of a/b. a is called the numerator and b is called the denominator. In simple fraction both numerator and denominators are integers expressed as a ratio
The equation is 7x+9 =11
we have to rearrange the terms
Move 9 to the right hand side
7x+9 = 11
7x = 11-9
Subtract it
7x = 2
Divide both sides by 7
x = 2/7
Hence, the solution of the equation 7x+9 =11 as a fraction in its simplest form is x = 2/7
Learn more about simple fraction here
brainly.com/question/2115640
#SPJ1
solve the PDE using separation of variables method Uxx = 1/2 Ut 0< X <3 with U(0,t) = U(3, t)=0, U(0, t) = 5sin(4πx)
Answers
The general solution of the partial differential equation is:
U(x, t) = Σ [Aₙ*sin((nπ/3)x)]*e^(-(nπ/3)²t)
How to solve Partial Differential Equations?The partial differential equation (PDE) is given as:
Uxx = (1/2)Ut with the boundary and initial conditions as 0< X <3 with U(0,t) = U(3, t)=0, U(0, t) = 5sin(4πx)
Assume that the solution can be written as a product of two functions:
U(x, t) = X(x)T(t)
Substituting this into the PDE, we have:
X''(x)T(t) = (1/2)X(x)T'(t)
Dividing both sides by X(x)T(t), we get:
(X''(x))/X(x) = (1/2)(T'(t))/T(t)
Since the left side only depends on x and the right side only depends on t, both sides must be equal to a constant, denoted as -λ²:
(X''(x))/X(x) = -λ²
(1/2)(T'(t))/T(t) = -λ²
Simplifying the second equation, we have:
T'(t)/T(t) = -2λ²
Solving the second equation, we find:
T(t) = Ce^(-2λ²t)
Applying the boundary condition U(0, t) = 0, we have:
U(0, t) = X(0)T(t) = 0
Since T(t) ≠ 0, we must have X(0) = 0.
Applying the boundary condition U(3, t) = 0, we have:
U(3, t) = X(3)T(t) = 0
Again, since T(t) ≠ 0, we must have X(3) = 0.
Therefore, we can conclude that X(x) must satisfy the following boundary value problem:
X''(x)/X(x) = -λ²
X(0) = 0
X(3) = 0
The general solution to this ordinary differential equation is given by:
X(x) = Asin(λx) + Bcos(λx)
Applying the initial condition U(x, 0) = 5*sin(4πx), we have:
U(x, 0) = X(x)T(0) = X(x)C
Comparing this with the given initial condition, we can conclude that T(0) = C = 5.
Therefore, the complete solution for U(x, t) is given by:
U(x, t) = Σ [Aₙsin(λₙx) + Bₙcos(λₙx)]*e^(-2(λₙ)²t)
where:
Σ represents the summation over all values of n
λₙ are the eigenvalues obtained from solving the boundary value problem for X(x).
To find the eigenvalues λₙ, we substitute the boundary conditions into the general solution for X(x):
X(0) = 0: Aₙsin(0) + Bₙcos(0) = 0
X(3) = 0: Aₙsin(3λₙ) + Bₙcos(3λₙ) = 0
From the first equation, we have Bₙ = 0.
From the second equation, we have Aₙ*sin(3λₙ) = 0. Since Aₙ ≠ 0, we must have sin(3λₙ) = 0.
This implies that 3λₙ = nπ, where n is an integer.
Therefore, λₙ = (nπ)/3.
Substituting the eigenvalues into the general solution, we have:
U(x, t) = Σ [Aₙ*sin((nπ/3)x)]*e^(-(nπ/3)²t)
where Aₙ are the coefficients that can be determined from the initial condition.
Read more about Partial Differential Equations at: https://brainly.com/question/28099315
#SPJ1
PLEASE HELP MEEEE!!!!!!!

Answers

NO LINKS! Please help me with this vertex problem
Show work please: step by step

Answers
Answer:
\(f(x)=6(x-2)^2+18\)
Step-by-step explanation:
Given quadratic function:
\(f(x)=6x^2-24x+42\)
To complete the square, begin by grouping the x terms within parentheses:
\(\implies f(x)=(6x^2-24x)+42\)
Factor out the coefficient of x²:
\(f(x)=6\left(x^2-4x\right)+42\)
Add the square of half the coefficient of the term in x inside the parentheses, and subtract the distributed value outside the parentheses:
\(\implies f(x)=6\left(x^2-4x+\left(\dfrac{-4}{2}\right)^2\right)+42-6\left(\dfrac{-4}{2}\right)^2\)
Simplify:
\(\implies f(x)=6\left(x^2-4x+\left(-2\right)^2\right)+42-6\left(-2\right)^2\)
\(\implies f(x)=6\left(x^2-4x+4\right)+42-6\left(4)\)
\(\implies f(x)=6\left(x^2-4x+4\right)+42-24\)
\(\implies f(x)=6\left(x^2-4x+4\right)+18\)
Factor the perfect square trinomial inside the parentheses:
\(\implies f(x)=6(x-2)^2+18\)
\(\boxed{\begin{minipage}{5.6 cm}\underline{Vertex form of a quadratic equation}\\\\$y=a(x-h)^2+k$\\\\where:\\ \phantom{ww}$\bullet$ $(h,k)$ is the vertex. \\ \phantom{ww}$\bullet$ $a$ is some constant.\\\end{minipage}}\)
Comparing the derived equation with the vertex formula, the vertex of the derived equation is (2, 18). Hence the completion of the square is correct.
In Bridget's class, 35% of the students chose pizza as their favorite food. If 14 of the students chose pizza as their favorite food, how many students are in the class?
Answers
Answer:
40 students are in the class :)
Step-by-step explanation:
35%=14
1%=14/35=0.4
0.4*100=40=100%
Benny has 72 teddy bears at his toy store. He wants to put them on 8 shelves with
the same number of bears on each shelf. How many teddy bears are on each shelf?
Answers
Answer: Answer below
Step-by-step explanation: So you would simply have to divide 8 into 72 which would be 9.
we can verify our answer by doing 9x8 which equals 72.
Answer: 9
Step-by-step explanation:
bears divided by shelves = number of bears per shelf
72 bears divided by 8 shelves= 9 bears per shelf
An automobile manufacturer produces production parts with 98% accuracy. What is the probability that in a production run of 25 parts, at most 2 are found to be defective?
Answers
An automobile manufacturer produces production parts with 98% accuracy. the probability is mathematically given as
P(X\leq2) = 0.9868
What is the probability that in a production run of 25 parts, at most 2 are found to be defective?Generally, the equation for the probability is mathematically given as
\(P(X\leq2) = P(X=0) + P(X=1) + P(X=2)\)
Therefore
\(P(X=0)=\binom{25}{0}* 0.98^{25}* 0.02^{0}=1* 0.6035* 1\\\\P(X=0)=0.6035\)
Hence
P(X=1)=0.3079
P(X=0)=0.07554
In conclusion, bringing the equation into view
\(P(X\leq2) = P(X=0) + P(X=1) + P(X=2)\)
\(P(X\leq2) = 0.6035 +0.3079 + 0.07554\)
P(X\leq2) = 0.9868
Read more about probability
https://brainly.com/question/795909
#SPJ1
Cole and Bryson went to Video Game Land. Cole won 152 tickets. Bryson won 84 tickets. They want to put their tickets together to get a large toy monkey that costs 300 tickets. How many more tickets do they need?
Answers
Answer:
64
Step-by-step explanation:
152+84=236
300-236=64
fraction that is equivalent to 3/9 and has a denominator of 3."
Answers
, The fraction equivalent to 3/9 with denominator 3 is the proper fraction p=1/3
What is a fraction in math?A fraction is a part of a whole. In arithmetic, the number is expressed as a quotient, in which the numerator is divided by the denominator. In a simple fraction, both are integers. A complex fraction has a fraction in the numerator or denominator. In a proper fraction, the numerator is less than the denominator.
Given here: The fraction 3/9
Now we can simplify the fraction further by dividing both the numerator and denominator by 3
let p=3/9
then p=3/3 / 9/3
p= 1/3
Hence, The fraction equivalent to 3/9 with denominator 3 is the proper fraction p=1/3
Learn more about fractions here:
https://brainly.com/question/29264210
#SPJ1
Which table represents a linear function?

Answers
Area of the base, B = 42 square meters
and height = 3 meters
Answers
Answer:
14
Step-by-step explanation:
all i did was divided 42 by 3 and got 14
3) g(x) = 4x
h(x) = -3x^2 + 4
Find (g + h)(x)
Answers
Answer:
The answer is the last line below.
Step-by-step explanation:
g(x) = 4x
h(x) = -3x^2 + 4
(g + h)(x) = 4x - 3x^2 + 4
Example # 2:
Find
Za
for the confidence level 90%.
2
Example # 3:
Find 2, for the confidence level 99%.
Answers
Answer:
(a) 1.645
(b) 2.5758
Step-by-step explanation:
The complete question is: Find Z(\(\alpha\)/2) for the confidence level 90% and for the confidence level 99%.
Firstly, as we know that \(\alpha\) represent the level of significance, i.e;
Level of significance = 1 - confidence level
(a) We are given the confidence level of 90%, so;
Level of significance = 1 - 90%
= 1 - 0.90 = 0.10 or 10%
Now, \(Z_(_\frac{\alpha}{2}_)\) = \(Z_(_\frac{0.10}{2}_)\) = \(Z_(_0_._0_5_)\)
This means that we have to look for a critical value in the z table at a 5% level of significance which gives us a value of 1.645.
(a) We are given the confidence level of 99%, so;
Level of significance = 1 - 99%
= 1 - 0.99 = 0.01 or 1%
Now, \(Z_(_\frac{\alpha}{2}_)\) = \(Z_(_\frac{0.01}{2}_)\) = \(Z_(_0_._0_0_5_)\)
This means that we have to look for a critical value in the z table at a 0.5% level of significance which gives us a value of 2.5758.