Triangle 1 undergoes four different transformations.
The results of these transformations are shown.
1
4
2
5
3
Which statement best describes one of these
transformations?
Triangle 1 is rotated to result in triangle 2.
O Triangle 1 is dilated to result in triangle 3.
O Triangle 1 is reflected to result in triangle 4.
Triangle 1 is stretched to result in triangle 5.
Answers
time tables on the map mannnnn
Related Questions
Free Branliest Quick and Correct!


Answers
A solid figure is separated into 2 rectangular prisms. The volume of rectangular prism A is 75 cubic yards. Rectangular prism
B has a length of 7 yards and a width of 3 yards. The total volume of the solid figure is 180 cubic yards. What is the height of
rectangular prism B?
Answers
The height of rectangular prism B is 5 yards.
Let's first find the volume of rectangular prism B:
The volume of the solid figure = Volume of prism A + Volume of prism B
180 = 75 + length × width × height of prism B
105 = length × width × height of prism B
We know that the length of prism B is 7 yards and the width is 3 yards,
Substitute those values:
105 = 7 × 3 × height of prism B
105 = 21 × height of prism B
height of prism B = 105/21
height of prism B = 5
Therefore, the height of rectangular prism B is 5 yards.
To know more about rectangular prism follow
https://brainly.com/question/30799424
#SPJ1
Fahrenheit femperature can be obtained from Celsius (centigrade) temperature by multiplying by 1and adding 32 degrees What Fahrenheit temperature corresponds to a Celsius temperature of 85
Answers
Answer:
117
Step-by-step explanation:
85 times 1 plus 32 = 117
How many green jolos are there in balans colony?

Answers
Answer: B. 280
Step-by-step explanation:
ur welcome
Answer:
B) 280
Step-by-step explanation:
The length of a rectangle is four meters less than twice it's width. If the area of the rectangle is 96 square meters what is the width?
Answers
Answer: (I'm sorry if this is not the right answer)
The width = 8 meters
Step-by-step explanation:
The length: a (m)
The width: b (m)
We cannot have a negative length and a negative width: a>b>0
Eq. I: a=2b-4
Eq. II: ab=96
Substitute the value of a from Eq. I into Eq. II: (2b-4).b=96
Solve for b:
(2b-4).b=96
2\(b^{2}\)-4b-96=0
\(b^{2}\)-2b-48=0
\(b^{2}\)+6b-8b-48=0
b.(b+6)-8.(b+6)=0
(b+6).(b-8)=0
b=8; b=-6
As mentioned above, b>0. Therefore, b=8
Conclusion: if the area of the rectangle is 96 square meters then its width is 8 meters.
The width of the given rectangle is 8 units.
What is a rectangle?A rectangle is a geometrical figure in which opposite sides are equal.
The angle between any two consecutive sides will be 90 degrees.
Area of rectangle = length × width.
Perimeter of rectangle = 2( length + width).
Let's say the length of the rectangle is L and the width is W
Given that,
The length of a rectangle is four meters less than twice its width.
So,
L = 2W - 4
And,
Area = 96 square meter
LW = 96
Now by substituting
2W² - 4W = 96
W² - 2W - 48 = 0
W² -8W + 6W - 48 = 0
W(W - 8) + 6(W - 8) = 0
(W + 6)(W - 8) = 0
W = 8, -6 since distance cannot be negative so it will be 6 meters.
Hence "The width of the given rectangle is 8 units".
For more about rectangles,
https://brainly.com/question/15019502
#SPJ2
determine the slope (m) and y-intercept (b) of a line that passes through the points (2,9)
and (5,21). Use a table with at least three other points and draw a graph to justify your answers. Finally, construct a
story problem that would use these conditions in the real world.
Answers
Answer:
y = mx + b is the slope-intercept form of the equation of a straight line. In the equation y = mx + b, m is the slope of the line and b is the intercept. x and y represent the distance of the line from the x-axis and y-axis, respectively. The value of b is equal to y when x = 0, and m shows how steep the line is.
HELP!!! Which equation below shows the relationship for the graph?

Answers
10(2)=20
10(3)=30
10(4)40
Write the next three terms of the geometric sequence 81, -27, 9, -3,...
Answers
1, -1/3, 1/9
it is divided by negative 3 or -3
To find that you need to divide the the first term with the next term
81÷-27=-3
16. Given that k is a real constant such that 0 < k < 1. Show that the roots of the equation kx2 + 2x + (1 – k) = 0, are С (i) Always real (ii) Always negative
Answers
Answer:
Discriminant
\(b^2-4ac\quad\textsf{when}\:ax^2+bx+c=0\)
\(\textsf{when }\:b^2-4ac > 0 \implies \textsf{two real roots}\)
\(\textsf{when }\:b^2-4ac=0 \implies \textsf{one real root}\)
\(\textsf{when }\:b^2-4ac < 0 \implies \textsf{no real roots}\)
Given equation: \(kx^2+2x+(1-k)=0\)
\(\implies a=k,\:b=2,\:c=(1-k)\)
\(\begin{aligned}\implies b^2-4ac & =(2)^2-4(k)(1-k)\\ & =4-4k(1-k)\\ & =4-4k+4k^2\\ & = 4k^2-4k+4 \end{aligned}\)
Complete the square:
\(\begin{aligned}\implies 4k^2-4k+4 & =4(k^2-k+1)\\ & = 4\left[k^2-k+\left(\dfrac{-1}{2}\right)^2+1-\left(\dfrac{-1}{2}\right)^2\right]\\ & = 4\left[\left(k-\dfrac12\right)^2+\dfrac34\right]\\ & = 4\left(k-\dfrac12\right)^2+3\end{aligned}\)
\(\textsf{As }\left(k-\dfrac12 \right)^2 \geq 0 \implies 4\left(k-\dfrac12 \right)^2+3\geq 3\)
Therefore, the roots are always real.
Mark wants to buy a new pair of shoes that cost $95. He has $20 and plans to save $5 each week. How many weeks will it take him to save the money?
Answers
Answer:
it would take him 15 weeks
Step-by-step explanation:
cost of shoes= 95
he has 20 so 95-20= 75
he needs 75 more dollars so 75 divided by 5= 15 weeks
Find the investment value when compounded anually.
P = $120,000, r= 5.3%, t = 8 yr
Answers
Given:
\(P=\$120,000\)
\(r=5.3\%\)
\(t=8\text{ years}\)
To find:
The value of the investment when the interest is compounded annually.
Solution:
The formula for amount is:
\(A=P\left(1+\dfrac{r}{n}\right)^{nt}\)
Where, P is the principal, r is the rate of interest in decimal, n is the number of time interest compounded in an years, and t is the number of years.
The interest is compounded annually. So, \(n=1\).
Substituting \(P=120000, r=0.053, n=1, t=8\) in the above formula, we get
\(A=120000\left(1+\dfrac{0.053}{1}\right)^{1(8)}\)
\(A=120000\left(1.053\right)^{8}\)
\(A=181387.85936\)
\(A\approx 181387.86\)
Therefore, the value of the investment after 8 years is $181,387.86.
If f(x) = 5x2 – X + 2, then what is the remainder when f(x) is divided by x + 1?
Answers
Answer:
The answer is 8Step-by-step explanation:
f(x) = 5x² - x + 2
Using the remainder theorem
To find the reminder substitute the value of x into the above function and solve
That's
x + 1 = 0
x = - 1
So we have
f(-1) = 5(-1)² - ( - 1) + 2
f(-1) = 5 + 1 + 2
f(-1) = 6 + 2
We have the final answer as
8Hope this helps you
If f(x) = 3x - 1 and g(x) = x + 2, find (f- g)(x).
I can’t find it
Answers
Answer:
\((f-g)(x)=2x-3\)
Step-by-step explanation:
We are given the two functions:
\(f(x)=3x-1\text{ and } g(x)=x+2\)
And we want to find:
\((f-g)(x)\)
This is equivalent to:
\(=f(x)-g(x)\)
By substitution:
\(=(3x-1)-(x+2)\)
Simplify. Distribute:
\(=(3x-1)+(-x-2)\)
Rearrange:
\(=(3x-x)+(-1-2)\)
Therefore:
\((f-g)(x)=2x-3\)
Which value of x is a solution to this equation? 2x2 + 7x – 30
Answers
simplify these numbers
1. 125 5. 384
2. 458 6. 15710
3. 438 7. 2549
4. 10512 8. 8920
Answers
the shortest side of a right triangle measures 7m. The lengths of the other two sides are Consecutive integers. What is the length of the other two sides?
Answers
The lengths of the other two sides of the right triangle are 24m and 25m, respectively.
Let's assume the consecutive integers representing the lengths of the other two sides of the right triangle are x and x + 1, where x is the smaller integer. We are given that the shortest side measures 7m. Now, we can use the Pythagorean theorem to solve for the lengths of the other two sides.
According to the Pythagorean theorem, in a right triangle, the square of the length of the hypotenuse (the longest side) is equal to the sum of the squares of the lengths of the other two sides.
Using this theorem, we have the equation:
\(7^2 + x^2 = (x + 1)^2\)
Expanding and simplifying this equation, we get:
\(49 + x^2 = x^2 + 2x + 1\)
Now, we can cancel out \(x^2\) from both sides of the equation:
49 = 2x + 1
Next, we can isolate 2x:
2x = 49 - 1
2x = 48
Dividing both sides by 2, we find:
x = 24
Therefore, the smaller integer representing the length of one side is 24, and the consecutive integer representing the length of the other side is 24 + 1 = 25.
Hence, the lengths of the other two sides of the right triangle are 24m and 25m, respectively.
For more question on lengths visit:
https://brainly.com/question/28322552
#SPJ8
PLEASE PLEASE HELP
The vertices of quadrilateral LMNP are L(-1 , 7), M(4,9), N(8, -1), and P(3,-3). Using the distance formula, determine the most precise classification of LMNP.
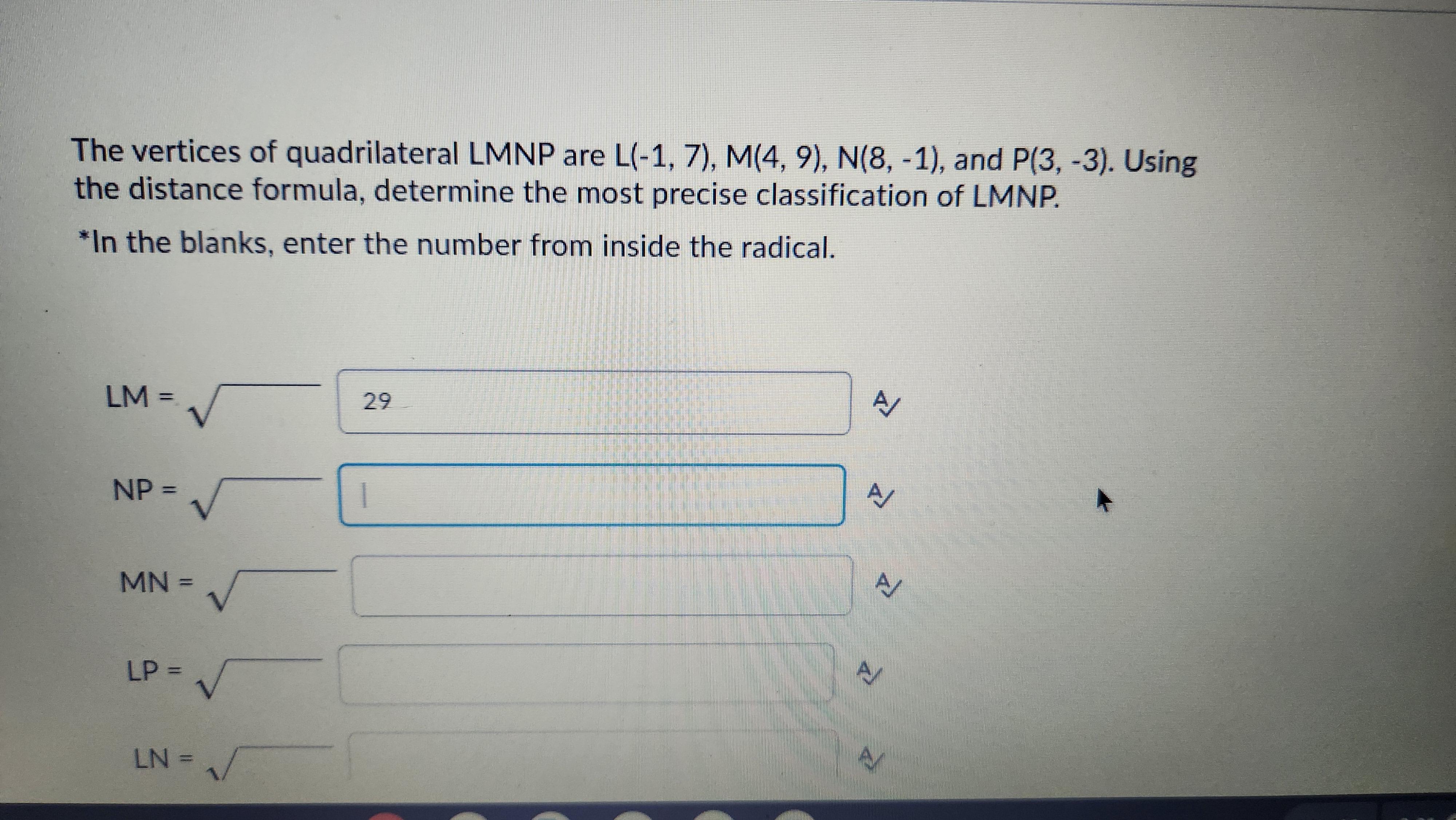

Answers
So, the most precise classification of quadrilateral LMNP is a kite.
What is equation?In mathematics, an equation is a statement that two mathematical expressions are equal. It typically contains variables, constants, and mathematical operations such as addition, subtraction, multiplication, and division. The goal of solving an equation is to find the values of the variables that make the equation true. Equations are used in many areas of mathematics, science, and engineering to model relationships between quantities and to solve problems.
Here,
To classify the quadrilateral LMNP, we need to calculate the length of all four sides using the distance formula and then use those values to determine the shape of the quadrilateral.
The distance formula is given by:
d = √[(x₂ - x₁)² + (y₂ - y₁)²]
Using this formula, we can calculate the length of each side of the quadrilateral as follows:
LM = √[(4 - (-1))² + (9 - 7)²] = √[5² + 2²] = √29
MN = √[(8 - 4)² + (-1 - 9)²] = √[4² + (-10)²] = √116
NP = √[(3 - 8)² + (-3 - (-1))²] = √[(-5)² + (-2)²] = √29
PL = √[(-1 - 3)² + (7 - (-3))²] = √[(-4)² + 10²] = √116
Now, we can use the values we have calculated to determine the shape of the quadrilateral. We can see that LM = NP and MN = PL, which means that opposite sides are congruent. Also, LM and MN are not equal to PL and NP, which means that opposite sides are not parallel. Therefore, LMNP is a kite.
To know more about equation,
https://brainly.com/question/28243079
#SPJ1
Jim is twice old as paul
Answers
Answer:
2x
Step-by-step explanation:
If Jim is twice as old as Paul,
we can use algebraic notation to express their ages.
Let's let "x" be Paul's age.
Then, Jim's age would be "2x" since he is twice as old as Paul
can anyone help me i have to find a equation of the line below it's a graph can anyone help me?
Answers
The equation of a line given two points A and B is calculated by the formula
\(\begin{gathered} A(x_1,y_1);B(x_2,y_2) \\ \text{the equation of the line AB is} \\ \frac{y-y_1}{x-x_1}=\frac{y_2-y_1}{x_2-x_1} \end{gathered}\)From the graph given, we have two points.
\(A(-5,3);B(5,-2)\)Substituting the values of the given points into the formula to get the equation of line
\(x_1=-5;y_1=3;x_2=5;y_2=-2\frac{\square}{\square}\)\(\begin{gathered} \frac{y-3}{x-(-5)}=\frac{-2-3}{5-(-5)} \\ \frac{y-3}{x+4}=\frac{-5}{5+5} \\ \frac{y-3}{x+4}=-\frac{5}{10} \end{gathered}\)\(\begin{gathered} \frac{y-3}{x+4}=\frac{-1}{2} \\ 2(y-3)=-1(x+4) \\ 2y-6=-x-4 \\ 2y+x=-4+6 \\ 2y+x=2 \end{gathered}\)\(\begin{gathered} 2y=2-x \\ y=\frac{2}{2}-\frac{x}{2} \\ y=1-\frac{x}{2} \end{gathered}\)Hence, the equation of the line is y=1-x/2
A truck weighs 16,000 pounds. What is the weight range in tons?
Answers
Answer:
8
Step-by-step explanation:
uh idrk all ik is that 16,000 punds = 8 tons
16,000 pounds equals 8 tons
674 is 40% of what number
Answers
start by writing the percentage as a relationship
clear the relationship for x
\(\begin{gathered} x=\frac{674\cdot100}{40} \\ x=1685 \end{gathered}\)
Determine what fraction of the figure is shaded.

Answers
Answer:
I would say that it would be 1/3 because there are 3 parts
Answer:
3/7
Step-by-step explanation:
There are 4 small squares inside the figure.
The partial squares are all half squares, so each two triangles have the same are as 1 small square. There are 6 small triangles which add to 3 squares.
4 + 3 = 7
Total area = 7 squares
Shaded area = 2 squares + 2 half squares = 3 squares
Fraction of shaded area = 3/7
9. Find the mean of the data set: 2, 3, 4, 5, 5
Step 1:
Add up all the numbers.
Step 2:
Divide by the number of items
in your data set.

Answers
Answer: Step 1 box= 19 (2+3+4+5+5)
Step 2 box= 3.8 (19 divided by 5)
Step-by-step explanation:
You add together the numbers to get 19, the you divide the number by five because you added 5 numbers together.
You had it just right on the bottom. Your equation was perfect. It doesn't matter if you get a decimal number.
Your answers are right too, I double checked.
Hope I was helpful.
which of the following is a true statement?

Answers
Answer:
3/8 less than 1/2 is correct
Step-by-step explanation:
Explanation: Multiply right side with 4
Lynne needs to borrow $9250 for cosmetic surgery she obtains a loan from her grandmother for 24 months at a simple interest rate of 7.1%. What is the loans future value?
Answers
The loan's future value, FV = $10563.5
Explanations:Let the amount borrowed by Lynned be the principal, P
P = $9250
The interest rate , r = 7.1%
r = 7.1 / 100
r = 0.071
Time, t = 24 months
12 months = 1 year
24 months = 2 years
t = 2 years
The future value is given by the formula:
FV = P ( 1 + rt)
FV = 9250 ( 1 + 0.071(2) )
FV = 9250 ( 1 + 0.142)
FV = 9250 ( 1.142)
FV = $10563.5
The school is planning to add 2 new playgrounds. Each playground will be in the shape of a square that measures by 24m by 24m.What will be the area of the playgrounds?
Answers
Answer: 1152 sq m
Step-by-step explanation: 24x24=576+ 24x24=576 so 576+576
If one angle of a set of alternate interior angles on parallel lines measures 77°, then the other angle also equals 77° _____.
always
sometimes
never
Answers
Answer:
I think is sometimes
Step-by-step explanation:
Answer:
The answer is always
What is the solution to the equation x + 14 = 63? (Input a whole number only.) (3 points) Blank 1:
Answers
Hey there!
This is a one-step equation, meaning that we can solve it in one step.
This is the step:
Subtract 14 from both sidesGoal:
To find out the value of xSubtract 14:
x+14-14=63-14
the 14's on the left cancel:
x=49
Hence, the answer is
\(\boxed{\boxed{\bold{x=49}}}\)
Hope everything is clear.
Let me know if you have any questions!
#LearningDoesn'tEnd
:-)
\(\rule{200}{2}\)
find the distance between the following pairs of points (-1,5)and(-7,-3)
Answers
The distance between the points (-1, 5) and (-7, -3) is 10 units.
What is the distance between the given points?The distance formula used in finding the distance between two points is expressed as;
D = √( ( x₂ - x₁ )² + ( y₂ - y₁ )² )
Point 1 (-1,5)
x₁ = -1y₁ = 5Point 2 (-7,-3)
x₂ = -7y₂ = -3Plug the given values into the distance formula and simplify.
D = √( ( x₂ - x₁ )² + ( y₂ - y₁ )² )
D = √[(-7 - (-1))² + (-3 - 5)²]
D = √[(-7 + 1)² + (-3 - 5)²]
D = √[-6² + (-8)²]
D = √[36 + 64]
D = √100
D = 10
Therefore, the distance is 10 units.
Learn more about the distance formula here: brainly.com/question/24509115
#SPJ1
Which equation represents a line which is perpendicular to the line y=-1/7x-3
Answers
Answer:
Step-by-step explanation:
To be perpendicular m1(m2)=-1
-1m/7=-1
m=7
So the perpendicular line will have 7 as the linear coefficient
y=7x+b