Timothy drinks ⅗ of a 16 ounce glass of juice in 30 minutes. How much would he drink in 2 ½ hours?

Answer:

5 : 7

Step-by-step explanation:

groom's side: 40

bride's side: 56

ratio groom to bride = 40/56 = 20/28 = 10/14 = 5/7

Answer: 5 : 7

Answer:

10.19

Step-by-step explanation:

Use the Pythagorean Theorem:

\(a^{2}+b^{2}=c^{2}\)

Substitute the numbers given:

\(11^{2}+b^{2}=15^{2}\)

\(121+b^{2}=225\\ b^{2}=225-121\\ b^{2}=104\\ \sqrt b^{2}= \sqrt{104} \\ b=10.19\)

Answer:

The quadratic x2 − 5x + 6 factors as (x − 2)(x − 3). Hence the equation x2 − 5x + 6 = 0

has solutions x = 2 and x = 3.

Similarly we can factor the cubic x3 − 6x2 + 11x − 6 as (x − 1)(x − 2)(x − 3), which enables us to show that the solutions of x3 − 6x2 + 11x − 6 = 0 are x = 1, x = 2 or x = 3. In this module we will see how to arrive at this factorisation.

Polynomials in many respects behave like whole numbers or the integers. We can add, subtract and multiply two or more polynomials together to obtain another polynomial. Just as we can divide one whole number by another, producing a quotient and remainder, we can divide one polynomial by another and obtain a quotient and remainder, which are also polynomials.

A quadratic equation of the form ax2 + bx + c has either 0, 1 or 2 solutions, depending on whether the discriminant is negative, zero or positive. The number of solutions of the this equation assisted us in drawing the graph of the quadratic function y = ax2 + bx + c. Similarly, information about the roots of a polynomial equation enables us to give a rough sketch of the corresponding polynomial function.

As well as being intrinsically interesting objects, polynomials have important applications in the real world. One such application to error-correcting codes is discussed in the Appendix to this module.

Answer:

\(2^x-4=32\quad :\quad x=\frac{2\ln \left(6\right)}{\ln \left(2\right)}\quad \left(\mathrm{Decimal}:\quad x=5.16992\dots \right)\\\\2^x-4=32 \\\mathrm{Add\:}4\mathrm{\:to\:both\:sides}\\2^x-4+4=32+4\\\mathrm{Simplify}\\2^x=36\\\\apply exponential rule\\x\ln \left(2\right)=\ln \left(36\right)\\x\ln \left(2\right)=\ln \left(36\right)\\x=\frac{2\ln \left(6\right)}{\ln \left(2\right)}\)

hope it helps:)

Answer:

Step-by-step explanation:

First confirm that x = 1 is one of the zeros.

f(1) = 2(1)^3 - 14(1)^2 + 38(1) - 26

f(1) = 2 - 14 + 38 - 26

f(1) = -12 + 38 = + 26

f(1) = 26 - 26

f(1) = 0

=========================

next perform a long division

x -1  || 2x^3 - 14x^2 + 38x - 26 || 2x^2 - 12x + 26

          2x^3 - 2x^2

          ===========

                    -12x^2 + 28x

                     -12x^2 +12x

                     ==========

                                  26x -26

                                  26x - 26

                                 ========

                                      0

Now you can factor 2x^2 - 12x + 26

                                 2(x^2 - 6x + 13)

The discriminate of the quadratic is negative. (36 - 4*1*13) = - 16

So you are going to get a complex result.

x = -(-6) +/- sqrt(-16)

     =============

                 2

x  = 3 +/- 2i

f(x) = 2*(x - 1)*(x - 3 + 2i)*(x - 3 - 2i)

The zeros are

1

3 +/- 2i

Answer:

Step-by-step explanation:

y= money earned , x is the number of hours

k is the constant=y/x=12

b- if she worked 18 hours:

y=12(18)

c- if she earned 330 dollars:

330=12x

x=330/12

Answer:

Our problem is \(x^2-2x+6=0\), but as we can see, we are unable to factor. We have to use the quadratic equation to solve.

\(x^2-2x+6=0\)

\(\frac{-b+-\sqrt{b^2-4ac}}{2a}\)

Positive Quadratic Formula:

\(\frac{-(-2)+\sqrt{(-2)^2-4(1)(6)}}{2(1)}\)

\(=\frac{2+\sqrt{4-24}}{2}\\=1+\frac{\sqrt{-20}}{2}\)

Negative Quadratic Formula:

\(\frac{-(-2)-\sqrt{(-2)^2-4(1)(6)}}{2(1)}\)

\(=\frac{2-\sqrt{4-24}}{2}\\=1-\frac{\sqrt{-20}}{2}\)

Since both of our answers are the square root of a negative number, we know that the quadratic equation has no real solution.

*We could have also used the Discriminant Test to determine whether the quadratic equation has real roots or not. However, for our means, the quadratic equation seems enough.

Answer:

D. No real Solution

Step-by-step explanation:

Hello!

Let's use the quadratic formula: \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\)

In our equation:

This comes from the standard form of a quadratic: \(ax^2 + bx + c\)

Now, solve:

Since the radicand (-5) is negative, there are no real solutions. The correct answer is Option D.

Given

LM = 9, MN = 6x, and LN = 9x

Find

LN

Explanation

as we see LN = LM + MN

so ,

so , LN = 9x = 9 * 3 = 27

Final Answer

Therefore , the length of LN is 27

Answer:

yall help out alot

Step-by-step explanation:

Answer:

0.4

Step-by-step explanation:

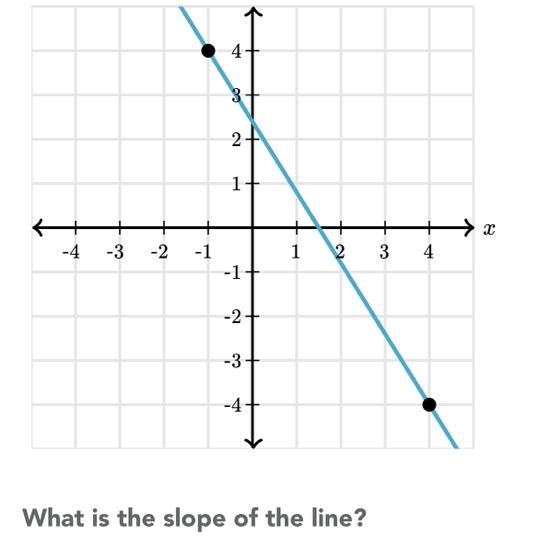
Answer:

\(slope = - \frac{8}{5} \)

Step-by-step explanation:

So it looks like on the line, we have two dots.

Let's find those points, starting from the left one.

The left point is: (-1, 4) --- (x1, y1)

The right point is: (4, -4) --- (x2, y2)

**These are in (X, Y) form**

Now to find the slope, you use this equation

\(m = \frac{y2 - y1}{x2 - x1} \)

Now let's plug our numbers into this equation

\(m = \frac{ - 4 - 4}{4 - ( - 1)} = \frac{ - 8}{5} = - \frac{8}{5} \)

Two negatives make a positive, so 4-(-1) is the same as 4+1.

Answer:

D I think

Step-by-step explanation:

one dozen is 12 so you reduce it so you make it smaller

This is approximately 0.283 hours, or 17 minutes. Therefore, it will take the boat approximately 17 minutes to cross the river.

The key to solving this problem is to understand the concept of relative velocity. In this case, the boat's speed relative to the water is 5 km/hr, and the water's speed relative to the shore is also 5 km/hr. Therefore, the boat's speed relative to the shore is the vector sum of these two velocities, which is 0 km/hr. This means that the boat will not make any progress toward the other side of the river unless it angles its course slightly upstream.
To determine the angle required, we need to use trigonometry. Let θ be the angle the boat makes with the direction perpendicular to the river. Then sin θ = 5/5 = 1, so θ = 45 degrees. This means that the boat needs to head upstream at a 45-degree angle to make progress across the river.
Now we can use the Pythagorean theorem to find the distance the boat travels:
d = √(1² + 1²) = √(2) km
Since the boat's speed relative to the shore is 0 km/hr, the time required for the crossing is simply the distance divided by the boat's speed relative to the water:
t = d / 5 = √(2) / 5 hours
This is approximately 0.283 hours or 17 minutes. Therefore, it will take the boat approximately 17 minutes to cross the river.

Learn more about trigonometry here:

https://brainly.com/question/12068045

#SPJ11

Answer:

-26

Step-by-step explanation:

Order of Operations: BPEMDAS

Step 1: Multiply

-6 - 20

Step 2: Subtract

-26

Answer:

-26

Step-by-step explanation:

You would first have to multiply 4 by -5 which is -20 so the equation now is, -6+(-20), the addition sign and the negative would also make a negative so it would be -6-20 which is -26. I hope you understand :*)

Answer: 4th option (the one already selected)

Step-by-step explanation: as the X variable changes by 7 the Y variable changes by 1

Answer:

3 : 7

Step-by-step explanation:

24/56 = 3 / 7

Answer:

3:7 helpful answer to your question have a nice evening

Step-by-step explanation:

Area of the large trapezoid :

   height * average of bases

     area = 8 * (6+11)/2 = 68 cm^2

  MINUS the area of the parallelogram   4x3 =12 cm^2

68 - 12 = 56 cm^2 = shaded region

Answer:

7g-13

Step-by-step explanation:

Answer:

-13 +17g

Step-by-step explanation:

−3 + 6g + 11g −10

-13 + 6g + 11g

-13 + 17g

Answer: 69.08 in

Step-by-step explanation:

The formula for circumference is:

2πr

If we substitute 3.14 for π, the formula is:

2(3.14)(11) = 69.08 in

Answer:

2386.0232

Step-by-step explanation:

Find the are, and then find the circumference.

Among the following values of r, 0.75 represents the strongest relationship. So, the correct option is D.

The value of r represents the strength of the correlation between two variables. The value of r ranges between -1 and 1, where a value of -1 represents a perfect negative correlation, 0 represents no correlation, and 1 represents a perfect positive correlation.

Therefore, among the given options, the value of 0.75 represents the strongest relationship. A value of 0.75 indicates a strong positive correlation between the two variables, meaning that as one variable increases, the other variable also tends to increase.

A correlation of this strength is often considered a strong relationship in many fields, such as psychology, economics, and engineering.

In contrast, a correlation of 0.50 indicates a moderate positive correlation, while a correlation of 0 indicates no correlation between the variables. A correlation of -1 indicates a perfect negative correlation, where the variables move in opposite directions.

To learn more about relationship click on,

https://brainly.com/question/29460656

#SPJ4

The value of b = -3/2 and c = -5/4

To write the quadratic x² - 3x + 1 in the form (x + b)² + c, we can expand (x + b)² and compare it to the given expression:

(x + b)² = x² + 2bx + b²

Comparing this to the given expression x² - 3x + 1, we see that we need:

2bx = -3x, so b = -3/2

b² + c = 1, so substituting b = -3/2 gives:

(9/4) + c = 1

so c = 1 - 9/4

= 4/4 - 9/4

= -5/4

The quadratic x² - 3x + 1 can be written in the form (x - 3/2)² + ( - 5/4).

Therefore, the value of b = -3/2 and c = -5/4

Learn more about Quadratic Equation here

https://brainly.com/question/30098550

#SPJ4

Given question is incomplete, the complete question is below

the quadratic x²-3x + 1 can be written in the form (x +b)² + c, where b and c are constants. what is b, c?

Assuming that the histogram for the sample is bell-shaped, approximately 68% of the sample values are within one standard deviation of the mean, 95% of the sample values are within two standard deviations of the mean, and 99.7% of the sample values are within three standard deviations of the mean.

Therefore, to estimate the percentage of sample values between 70 and 82, we need to find the number of standard deviations away from the mean that corresponds to these values.

Once we have the mean and standard deviation, we can use the formula:

z = (x - μ) / σ

where z is the number of standard deviations away from the mean,

x is the sample value,

μ is the mean,

and is the standard deviation.

The percentage of sample values between 70 and 82 will be the difference between these two percentages.

To learn more about “standard deviations” refer to the https://brainly.com/question/475676

#SPJ11

Answer:

$8225

Step-by-step explanation:

329 (amount for each ipad mini) x 25 (amount of ipad minis Missbailey is going to buy) = 8225.

Answer:

Move all terms that don't contain  x  to the right side and solve.

x =  5 /3 −  4 y /3

Answer:4y+3x=5

Step 1: Add -4y to both sides.

3x+4y+−4y=5+−4y

3x=−4y+5

Step 2: Divide both sides by 3.

3x

3

=

−4y+5

3

x=

−4

3

y+

5

3

Step-by-step explanation:

O no real solutions

hope it's helpful

Answer:

Below

Step-by-step explanation:

Value will be original value * ( 1 + i)^n      i = decimal annual interest

                                                                      n = years

  Value = 300 ( 1 + .07)^10 = 590.15  (dollars ?)

Answer:

Tn = 8n - 2

Step-by-step explanation:

Given the arithmetic sequence

6, 14, 22, 30, 38

The nth term is expressed as;

Tn = a +(n-1)d

a is the first term = 6

d is the common difference = 14 - 6 = 22 - 14 = 8

Substitute into the formula;

Tn = 6 + (n-1)(8)

Tn = 6 + 8n-8

Tn = 8n - 2

Hence the nth term of the sequence is 8n-2

Answer: equation y = 1/2 x -4

Step-by-step explanation:

Since u have the slope just keep working with it 1 down 2 left and u will arrive at the y intercept

Answer:

40.56

Step-by-step explanation:

2pir

8÷2=r=4

2pi4=25.13

25.13÷2=12.565

10+10+8+12.56=40.565