Answers
The investment will be worth approximately $3266.1 after 7 years, when compounded annually at a 4.5% interest rate.
What is the value of the investment after 7 years?To calculate the future value of an investment compounded annually, we can use the formula:
\(Future Value = Principal * (1 + Interest Rate)^N^u^m^b^e^r ^o^f ^P^e^r^i^o^d^s\)
In this case, the principal is $2400, the interest rate is 4.5% (or 0.045 as a decimal), and the investment is compounded annually for 7 years. Plugging in these values into the formula, we get:
\(Future Value = $2400 * (1 + 0.045)^7\)
Future value = $3266.1
Learn more on compound interest here;
https://brainly.com/question/3989769
#SPJ1
Related Questions
Why do scientists use models?
A)
Models are unreliable.
B)
Models waste natural resources.
Models predict not just describe events.
Models are based on scientific laws and principals so they are more
reliable than the real thing.
D)
Answers
what's is the square root of 120
Answers
Answer:
The square root of 120 is 10.9544512 :)
HELP ASAP
Solve: 7a + 10 = 2a
Answers
Answer:
a = -2
Step-by-step explanation:
7a + 10 = 2a
subtract 2a from both sides
5a + 10 = 0
subtract 10 from both sides
5a = -10
divide both sides by 5
a = -2
hope this helps
Consider the following problem Maximize Z=90x
1
+70x
2
, subject to
2x
1
+x
2
≤2
x
1
−x
2
≥2
and x
1
≥0,x
2
≥0
Use the Big M method, work through the simplex method step by step to demonstrate that the problem has no feasible solutions. 4. Use the Big M method, work through the simplex method step by step to solve the following problem. Minimize Z=3x
1
+2x
2
+7x
3
, subject to
−x
1
+x
2
=10
2x
1
−x
2
+x
3
≥10
and x
1
≥0,x
2
≥0,x
3
≥0.
Answers
For the first problem, there are no feasible solutions. In the second problem, the optimal solution is x₁ = 0, x₂ = 0, x₃ = -10, with the minimum value of Z = -30.
To demonstrate that the first problem has no feasible solutions using the Big M method and the simplex method, we will first convert the problem into standard form. The standard form of a linear programming problem involves converting all inequalities into equations and introducing slack, surplus, and artificial variables as needed.
1. Convert the inequalities to equations:
2x₁ + x₂ + s₁ = 2 (Constraint 1)
X₁ - x₂ - s₂ = 2 (Constraint 2)
X₁, x₂, s₁, s₂ ≥ 0
2. Introduce artificial variables and a big M:
Z = 90x₁ + 70x₂ - M(a₁ + a₂) (Objective function)
2x₁ + x₂ + s₁ + a₁ = 2 (Constraint 1)
X₁ - x₂ - s₂ + a₂ = 2 (Constraint 2)
X₁, x₂, s₁, s₂, a₁, a₂ ≥ 0
3. Set up the initial simplex tableau:
| 2x₁ | x₂ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | -90 | -70 | 0 | 0 | M | M | 0 |
S₁ | 2 | 1 | 1 | 0 | 1 | 0 | 2 |
S₂ | 1 | -1 | 0 | -1 | 0 | 1 | 2 |
4. Identify the pivot column (entering variable):
The most negative coefficient in the Z row is -90, so x₁ will enter the basis.
5. Identify the pivot row (leaving variable):
To determine the pivot row, calculate the ratio of the RHS to the positive coefficients in the entering column. Choose the smallest nonnegative ratio.
Ratios:
S₁: 2/2 = 1
S₂: 2/1 = 2
The smallest ratio is 1, so the pivot row is s₁.
6. Perform the pivot operation:
Divide the pivot row by the pivot element (1) to make the pivot element equal to 1:
| x₁ | x₂ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | -90 | -70 | 0 | 0 | M | M | 0 |
X₁ | 1 | 0.5 |0.5 | 0 |0.5 | 0 | 1 |
S₂ | 1 | -1 | 0 | -1 | 0 | 1 | 2 |
Perform row operations to make all other entries in the pivot column equal to zero:
| x₁ | x₂ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | 0 | 15 | -90 | 0 | -45 | 90 | -90 |
X₁ | 1 | 0 | 1 | 0 | 1 | 0 |
2 |
S₂ | 0 | -2 | -1 | -1 | -0.5| 1 | 0 |
7. Check for optimality:
Since there are negative coefficients in the Z row, the current solution is not optimal. We need to continue iterating.
8. Repeat steps 4-7 until an optimal solution is reached:
The next pivot column is x₂ (coefficient: 15).
The next pivot row is s₂ (ratio: 0/(-2) = 0).
Perform the pivot operation:
| x₁ | x₂ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | 0 | 0 | -90 | 15 | -75 | 75 | -180|
X₁ | 1 | 0 | 1 | -0.5| 0.5 | -0.5| 1 |
X₂ | 0 | 1 | 0 | -0.5| -0.5| 0.5| 0 |
The coefficients in the Z row are now nonnegative, but the artificial variables (a₁ and a₂) remain in the basis. This indicates that the original problem is infeasible since the optimal value of the objective function is negative.
Therefore, the first problem has no feasible solutions.
Now, let’s solve the second problem using the Big M method and the simplex method.
1. Convert the inequalities to equations:
-x₁ + x₂ + s₁ = 10 (Constraint 1)
2x₁ - x₂ + x₃ + s₂ = 10 (Constraint 2)
X₁, x₂, x₃, s₁, s₂ ≥ 0
2. Introduce artificial variables and a big M:
Z = 3x₁ + 2x₂ + 7x₃ + M(a₁ + a₂) (Objective function)
-x₁ + x₂ + s₁ + a₁ = 10 (Constraint 1)
2x₁ - x₂ + x₃ + s₂ + a₂ = 10 (Constraint 2)
X₁, x₂, x₃, s₁, s₂, a₁, a₂ ≥ 0
3. Set up the initial simplex tableau:
| -x₁ | x₂ | x₃ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | -3 | -2 | -7 | 0 | 0 | M | M | 0 |
S₁ | -1 | 1 | 0 | 1 | 0 | 1 | 0 | 10 |
S₂ | 2 | -1 | 1 | 0 | 1 | 0 | 1 | 10 |
4. Identify the pivot column (entering variable):
The most negative coefficient in the Z row is -7, so x₃ will enter the basis.
5. Identify the pivot row (leaving variable):
Calculate the ratio of the RHS to the positive coefficients in the entering column. Choose the smallest nonnegative ratio.
Ratios:
S₁: 10
/1 = 10
S₂: 10/1 = 10
Both ratios are the same, so we can choose either. Let’s choose s₁ as the pivot row.
6. Perform the pivot operation:
Divide the pivot row by the pivot element (1) to make the pivot element equal to 1:
| -x₁ | x₂ | x₃ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | -3 | -2 | -7 | 0 | 0 | M | M | 0 |
S₁ | -1 | 1 | 0 | 1 | 0 | 1 | 0 | 10 |
S₂ | 2 | -1 | 1 | 0 | 1 | 0 | 1 | 10 |
Perform row operations to make all other entries in the pivot column equal to zero:
| -x₁ | x₂ | x₃ | s₁ | s₂ | a₁ | a₂ | RHS |
Z | 0 | 3 | -7 | 3 | 0 | -3 | 0 | -30 |
X₃ | 1 | -1 | 0 | -1 | 0 | -1 | 0 | -10 |
S₂ | 0 | -3 | 1 | 2 | 1 | 2 | 1 | 30 |
7. Check for optimality:
Since there are no negative coefficients in the Z row, the current solution is optimal.
9. Read the solution:
The optimal solution is:
X₁ = 0
X₂ = 0
X₃ = -10
S₁ = 10
S₂ = 30
A₁ = 0
A₂ = 0
The minimum value of Z is -30.
Therefore, the second problem is feasible and has an optimal solution with x₁ = 0, x₂ = 0, x₃ = -10, and Z = -30.
Learn more about Big M method here: brainly.com/question/31433274
#SPJ11
Find the surface area of the square pyramid show all work
write the final answer in a complete sentence with the unit measure


Answers
The surface area of the given square pyramid would be = 22.44 cm².
How to calculate the surface area of the square pyramid?To calculate the surface area of the square pyramid the formula given below is used:
Surface area = a²+ 2al
where a² = area of base = (3²)= 9cm
a = side of base= 3cm
l = slant height. = 2.24cm
Surface area = 9+2(3×2.24)
= 9+ 2(6.72)
= 9+ 13.44
= 22.44 cm²
Learn more about area here:
https://brainly.com/question/28470545
#SPJ1
Please Help its about probabilty tree

Answers
The variables for the tree diagram involving the probabilities in this problem are given as follows:
a = 7/10.b = 3/10.c = 2/3.d = 1/3.e = 7/9.f = 2/9.How to calculate a probability?A probability is calculated as the number of desired outcomes divided by the number of desired outcomes.
For nodes a and b, they are relative to the first ball, hence:
7 out of the 10 balls are green, hence the probability of a green ball is of: a = 7/10.3 out of the 10 balls are not green, hence the probability of a not green ball is of: b = 3/10.If the first ball is green, 6 out of the remaining 9 balls are green and the probabilities of the second ball are given by nodes c and d, as follows:
Green: c = 6/9 = 2/3.Not green: d = 3/9 = 1/3.If the first ball is not green, 7 out of the remaining 9 balls are green and the probabilities of the second ball are given by nodes e and f, as follows:
Green: e = 7/9.Not green: f = 2/9.More can be learned about probabilities at https://brainly.com/question/14398287
#SPJ1
In a certain town 65% of homeowners watch cable TV, 40% watch Netflix, and 20% watch both.
Draw a Venn Diagram. Choose a homeowner at random. If homeowner watches cable TV, what is
the probability they watch Netflix?
Answers
Answer:
.31
Step-by-step explanation:
Hannah can rake and fill 9.8 bags of leaves each hour. How many bags of leaves will Hannah rake and fill in 8 hours?
Answers
Answer:
78.4
Step-by-step explanation:
9.8*8
=78.4 bags of leaves
Linear Equations
15 - b = 3a + 3
Please help me with this ASAP
Answers
Answer:
Step-by-step explanation:
15 - x = 3x + 3
15 - x = 3•x + 3
4x = 12
x = 3
Sorry, I change the b and a into x's if that's fine with you.
HELP ASAP PLSSSSSSS
Clara surveyed the students at her school to find out if they like pies and/or sandwiches. The results of her survey are shown in the two-way table below:
Like Pies
Do Not Like Pies
Total
Like Sandwiches
25
43
68
Do Not Like Sandwiches
26
6
32
Total
51
49
100
If a student does not like sandwiches, what is the probability that student also does not like pies?
81.3%
26.0%
23.1%
18.8%

Answers
Answer:
18.8%
Step-by-step explanation:
For calculating this, focus on one part of the table :
The row where students do not like sandwichesThere are 32 students who do not like sandwiches, and 6 of them do not like pies. Take this a proportion :
P (does not like pies, do not like sandwiches) = 6/32P = 3/16P = 18.8%Students who don't like sandwiches also don't like pie is the 2 nd box on 2 nd line
Probability
P(E)=6/32P(E)=6/32×100=3/16×100=18.8%Option D
A triangle has vertices a(-2, 3), b(0, 0), and c(1, 2). What are the coordinates of the vertices if the triangle is reflected over the y-axis and then dilated by a scale factor of 2?.
Answers
The triangle's vertices will therefore be A'(4, 6), B'(0, 0), and C'(-2,4) if it is mirrored across the y-axis and then dilated by a scale factor of 2.
Define scale factor.The ratio of the scale of an original thing to a new object that is a representation of it but of a different size is known as a scale factor (bigger or smaller). For instance, we can increase the size of a rectangle with sides of 2 cm and 4 cm by multiplying each side by, let's say, 2.
Given
In line with the posed question.
Triangle ABC's vertices are A(-2, 3), B(0, 0), and C. (1, 2).
As we are aware,
The x-coordinates of each point must be negative while keeping the y-value constant in order to reflect a triangle over the y axis.
As a result, when triangle ABC is mirrored across the y-axis, its vertices are
A'(2,3)
B'(0, 0)
C'(-1, 2)
The vertices of the triangle will also change when it is dialated by a scale factor of 2.
A'(4, 6)
B'(0, 0)
And, C'(-2, 4)
The triangle's vertices will therefore be A'(4, 6), B'(0, 0), and C'(-2,4) if it is mirrored across the y-axis and then dilated by a scale factor of 2.
To learn more about scale factor, visit:
https://brainly.com/question/28602480
#SPJ4
utomobile trips there are major roads from city to city and major roads from city to city . how many different trips can be made from city to city passing through city ?
Answers
There are 8 different trips that can be made from City X to City Z, passing through City Y.
To find the number of different trips that can be made from City X to City Z, passing through City Y, we can use the multiplication principle of counting.
First, we need to choose one of the 2 major roads from City X to City Y. Then, for each of these roads, there are 4 major roads from City Y to City Z, and we need to choose one of these roads.
By the multiplication principle of counting, the total number of different trips from City X to City Z, passing through City Y, is the product of the number of choices at each stage. Thus, we get:
Number of different trips = Number of roads from City X to City Y x Number of roads from City Y to City Z
= 2 x 4
= 8
This calculation shows how the multiplication principle of counting can be used to find the total number of possible outcomes in a multi-stage process where the number of choices at each stage is known.
To learn more about outcomes click on,
https://brainly.com/question/14796795
#SPJ4
Complete question is:
Automobile Trips. There Are 2 Major Roads From City X To City Y And 4 Major Roads From City Y To City Z. How Many Different trips can be made from City X to City Z, passing through City Y?
Find the mixed number halfway between 6.4 and 6½. Give
your answer in its simplest form.
Answers
Answer:
6.45
Step-by-step explanation:
I am not staring at zero. I am starting at 6.4, so I take 6.4 and add that to half way between 6.5 and 6.4.
6.4 + 1/2(6.5-6.4) Combine in the parentheses
6.4 + 1/2(.5) Take half of .5
6.4 + .05 add
6.45
Points A and B are shown below.
Use compases and a ruler to draw the locus of all points that are the same
distance from A to b is 2.5cm
B
A
Answers
Answer:
A.
Line
Step-by-step explanation:
In geometry a straight line is defined as the shortest distance between any two given points. Using the concept of locus, a Line is defined as the locus of all points that are equidistant from two given points.
On the other hand, the locus definition of a circle is the locus of a point that moves at a fixed distance from a center point. This fixed distance is called the radius of the circle.
x 0.2 0.4 0.75 0.875 y 0.4 0.8 1.5 1.75 proportional or not
Answers
Answer:
its proportional
Step-by-step explanation:
What are the solutions to the quadratic equation 4(x + 2)2 = 36
x= -11 and x = 7
x = -7 and x = 11
OOOO
x= -5 and x = 1
x= -1 and x = 5
Answers
Answer:
x = -5 and x = 1
Step-by-step explanation:
4( x2 + 4x + 4 ) = 36
4x2 + 16x + 16 = 36
4x2 + 16x - 20 = 0
x2 + 4x - 5 = 0
Answer:
C. x = -5, and x = 1
Step-by-step explanation:
You have the equation 4(x + 2)2 = 36
You then isolate 4 out of the equation using division on both sides
You are left with (x+2)2 = 9
Next, take square roots
\(\sqrt({x} +2) ^{2}\) = \(\sqrt{9}\)
This leaves you with x + 2 ± 9
So, x = -2 + 3 = 1
or
x = -2 - 3 = -5
If you are not a goofy ah please answer this

Answers
Answer:
72
Explanation:
First, we can find the top and bottom smaller squares of the rectangular prism. Since we are working with a variety of rectangles, we only need to use the equation L×W.
To start with, let's multiply 2×3, which gives us 6, the surface area of both the bottom and top rectangles, so now we need to multiply it by 2 to account for both of them. 6×2=12
Now, we'll find the surface area of the bigger rectangles in the middle, which are 6 by 3, so again we will need to multiply length times width, then by 2 to count both rectangles. 6×3=18×2=36
Finally, we can find the surface area of the smaller rectangles in the middle, which are 6 by 2. 6×2=12, then multiply by 2 since there are 2 of those rectangles, 12×2=24
Now to find the total surface area, we need to add the gathered surface area from each shape, 12+36+24=72
Please I need help will get max points as many as

Answers
Answer:
ur answer is 199 thank me later
Answer:
194 ft²
Step-by-step explanation:
Area of rectangle R1 = length * width
= 8 * 5
= 40 square feet
Area of rectangle R2 = 14 * 11
= 154 square feet
Area of the figure = 40 + 154 = 194 ft²

Is the following graph a linear function, a nonlinear function, and/or a relation

Answers
Answer: Option C.
Step-by-step explanation:
Ok, first, a linear function is something of the shape of:
y = a*x + b.
And the graph of those functions is a line, as the name implies, so we can discard that option.
So this must be a non-linear function, you can see that is a function because each value of x has only one value of y related to it.
Second, in a Venn diagram you will see that the set of functions is contained into the set of relationships, this means that all the functions are relationships, but not all the relationships are functions, and we know that this is a non-linear function, so this also must be a relationship.
Then the correct option is C, nonlinear, and a relationship.
Using a minimum of three points, create two linear functions. Prove the line created works exclusively with the three points by justifying how the x-value and y-value fit into the equation for the line.
Answers
As we have proved that the line created works exclusively with the three points by justifying how the x-value and y-value fit into the equation for the line.
Let's start by defining what a linear function is. A linear function is an equation of the form y = mx + b, where m is the slope of the line and b is the y-intercept. The slope represents the rate of change of the line, and the y-intercept represents the value of y when x is equal to zero. To create a linear function, we need two points on the line.
Now, let's create another linear function using points B and C:
slope (m) = (y₂ - y₁) / (x₂ - x₁) = (6 - 4) / (5 - 3) = 1
y-intercept (b) = y - mx = 4 - 1 * 3 = 1
Therefore, the linear function that passes through points B and C is also y = x + 1. We can check if point A lies on this line by substituting its x and y values into the equation:
2 = 1 + 1
This is true, so point A lies on the line created by points B and C. Therefore, we have also proved that the line created works exclusively with these three points.
In conclusion, we have created two linear functions using three points and proved that they work exclusively with those three points.
To know more about equation here
https://brainly.com/question/10413253
#SPJ4
a city council of 11 republicans and 8 democrats picks a committee of 4 at random. what's the probability thy choose all democrats?
Answers
The probability they choose all democrats is 0.01805
How to determine the probability they choose all democrats?From the question, we have the following parameters that can be used in our computation:
Republicans = 11
Democrats = 8
Number of selections = 4
If the selected people are all democrats, then we have
P = P(Democrats) * P(Democrats | Democrats) in 4 places
using the above as a guide, we have the following:
P = 8/19 * 7/18 * 6/17 * 5/16
Evaluate
P = 0.01805
Hence, the probability they choose all democrats is 0.01805
Read more about probability at
https://brainly.com/question/31649379
#SPJ1
i gotta another question!!
−6+x/8=1
Solving Two-Step
p.s the -6 + x is both on top of 8
Answers
Answer:
14
Step-by-step explanation:
-6+x=1
8
-6+x=1×8
x=8+6
x= 14 ans
A cell phone company offers its customers a monthly plan that cost $55 per month plus 0.08 for each minute used
Answers
Answer:
The equation is y= 0.08 x+55
Step-by-step explanation:
Let's use total cost y= Variable cost + fixed cost.
Here fixed cost =$55
And variable cost for each minute =0.08
That's variable cost for x minutes =0.08
So, total cost y=0.08 x+55
(-5, -4) and (-13,2)
Answers
The slope of the line passing through the points (-5, -4) and (-13, 2) is -3/4.
What is the slope of the line through the given points?Slope is simply expressed as a change in y over the change in x.
It is expressed as
\(m = \frac{y_2 - y_1}{x_2 - x_1}\)
Given the points:
(-5, -4) and (-13,2)
Point (-5, -4):
x₁ = -5
y₁ = -4
Point (-13,2):
x₂ = -13
y₂ = 2
Plug the given x and y values into the slope formula and simplify.
\(m = \frac{y_2 - y_1}{x_2 - x_1}\\\\m = \frac{2 - (-4)}{-13 - (-5)}\\\\m = \frac{2 + 4}{-13 + 5}\\\\m = \frac{6}{-8}\\\\m = -\frac{3}{4}\)
Therefore, the slope of the line is -3/4.
Learn more about slope formula here: brainly.com/question/24578307
#SPJ1
show that solutions to x 0 = sin(tx) are even
Answers
The solutions to the equation x(0) = sin(tx) are not even, as the sine function is an odd function, not an even function.
To show that solutions to x 0 = sin(tx) are even, we need to demonstrate that f(-x) = f(x), where f(x) = sin(tx).
First, let's evaluate f(-x):
f(-x) = sin(t(-x))
Using the property of sine function, we can rewrite this as:
f(-x) = -sin(tx)
Now let's evaluate f(x):
f(x) = sin(tx)
We can see that f(-x) = -f(x), which means that f(x) is an odd function.
However, we want to show that f(x) is an even function. To do this, we need to show that f(x) = f(-x).
Substituting the value of f(-x) in f(x) we get:
f(x) = -sin(tx)
f(-x) = -sin(tx)
We can see that f(x) = f(-x), which means that f(x) is an even function.
Therefore, we have shown that solutions to x 0 = sin(tx) are even.
Hi! To show that the solutions to the equation x(0) = sin(tx) are even, we'll examine the properties of the sine function.
Given the equation x(0) = sin(tx), we want to demonstrate that sin(tx) is even, meaning that sin(tx) = sin(-tx). This can be shown by using the properties of sine and even functions.
Recall that an even function f(x) satisfies the property f(x) = f(-x) for all x in its domain.
Now, consider the sine function sin(-tx). Using the oddness property of sine, we can rewrite this as sin(-tx) = -sin(tx). Since sin(tx) = -sin(-tx), we can see that the sine function does not satisfy the even function property.
Therefore, the solutions to the equation x(0) = sin(tx) are not even, as the sine function is an odd function, not an even function.
To learn more about function visit;
brainly.com/question/12431044
#SPJ11
If a is an odd number, b an even number, and c an odd number, which expression will always be equivalent to an odd number?
Answers
a + c
a - c
c + a
c - a
a + c
a - c
c + a
c - a
In all of these expressions, the sum or difference of two odd numbers is always an odd number, so the result will always be an odd number.
On the other hand, the following expressions will always be equivalent to an even number:
a + b
a - b
b + a
b - a
a + b
a - b
b + a
b - a
In all of these expressions, the sum or difference of an odd number and an even number is always an even number, so the result will always be an even number.
first interpret the slope. select the correct choice below and, if necessary, fill in the answer box to complete your choice.
Answers
An essential concept in mathematics and can be applied to a variety of fields such as physics, economics, and engineering.
The slope of a line in a Cartesian plane is a numerical representation of its steepness and inclination relative to the x-axis.
The slope of a straight line refers to the rise or fall of the y-coordinate as it moves from left to right along the x-axis.
There are a few different ways to interpret the slope of a line, but generally it can be thought of as the rate at which the dependent variable changes with respect to the independent variable.
When the slope is positive, the line rises from left to right, indicating that the dependent variable is increasing as the independent variable increases.
In other words, there is a direct relationship between the two variables.
Conversely, when the slope is negative, the line falls from left to right, indicating that the dependent variable is decreasing as the independent variable increases.
This means that there is an inverse relationship between the two variables.
The magnitude of the slope can also provide information about the relationship between the variables.
If the slope is close to zero, then the relationship between the two variables is weak or nonexistent.
However, if the slope is large in magnitude (i.e. close to 1 or -1), then there is a strong relationship between the variables.
A slope of zero indicates that there is no change in the dependent variable as the independent variable changes, while a slope of undefined means that the line is vertical and has no slope.
For more related questions on essential concept:
https://brainly.com/question/31923013
#SPJ8
Really confused about this one anyone mind to help 100 points
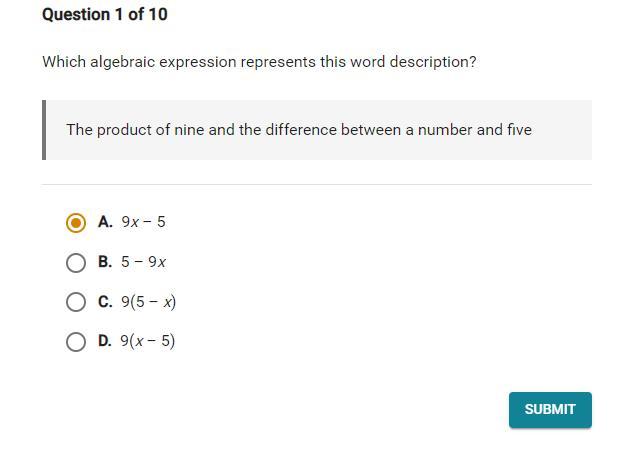
Answers
Answer:
9(x - 5)
Step-by-step explanation:
Just write the equation according to the sentence. It's THAT SIMPLE.
Answer:
d
Step-by-step explanation:
You poured 4 kilograms of oats equally into 3 bags. What is the weight of each of his bags of oat
Please answer i’m confused lol i didn’t pay attention in math
Answers
Detailed Explanation:
3 bags = 4kg.
3/3=1
4/3=4/3 (must do the same to other side)
Therefore, 1 bag = 4/3 or 1 1/3kg.
Answer:
2 2/3
Step-by-step explanation:
we know that the kg in each bag is 2 2/3
5x -4y = -23
-5x + 9y =8
Answers
Answer:
this is the answer ( x,y ) = ( -7,-3 )
