thirty-two people are going to lunch. each lunch table seats 4 people.how many tables are needed for 32 people?
Answers
We know that 8 tables will be needed to place 32 people using simple mathematical operations.
What are mathematical operations?A mathematical function known as an operation converts zero or more input values into a precisely defined output value. The quantity of operands affects the operation's arity. You solve mathematical expressions in the following order: parentheses, exponents, multiplication, division, addition, and subtraction. In this order, all expressions should be condensed.So, we know that:
There is a total of 32 people.
Each table can have 4 people on it.
So, a number of tables needed to place 32 people:
32/4
8
Therefore, we know that 8 tables will be needed to place 32 people using simple mathematical operations.
Know more about mathematical operations here:
https://brainly.com/question/28937023
#SPJ4
Related Questions
I need help on these!
Answers
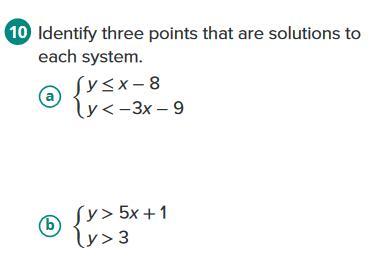
Three points that are solutions to the system are:
(0, -9)
(3, -5)
(4, -4)
b.
Three points that are solutions to the system are:
(3, 2)
(2, 1)
(1, 0)
Philip owns 100 shares of a stock that is trading at $97. 55 and pays an annual dividend of $2. 74. How much should he receive in quarterly dividends? What's the annual yield on this stock?
Answers
Philip should receive $68.50 in quarterly dividends and the annual yield on this stock is about 2.81%.
To calculate the quarterly dividend that Philip need to acquire, we need to first calculate the quarterly dividend per share:
Quarterly dividend in step with share = Annual dividend per percentage / 4
In this situation, the once a year dividend in line with proportion is $2.74, so the quarterly dividend per proportion is:
Quarterly dividend per proportion = $2.74 / 4 = $0.685
For the reason that Philip owns 100 shares, his quarterly dividend should be:
Quarterly dividend = Quarterly dividend per share * number of stocks
Quarterly dividend = $0.685 * 100 = $68.50
Therefore, Philip should receive $68.50 in quarterly dividends.
To calculate the once a year yield on this inventory, we want to divide the yearly dividend in line with proportion by the present day stock price, after which multiply by way of 100 to specific the result as a percentage:
Annual yield = (Annual dividend per share / inventory price) * 100
In this case, the annual dividend per percentage is $2.74, and the inventory charge is $97.55. Plugging those values into the components, we get:
Annual yield = ($2.74 / $97.55) * 100
Annual yield ≈ 2.81%
Therefore, the annual yield on this stock is about 2.81%.
Learn more about stock dividend:-
https://brainly.com/question/29803016
#SPJ4
what is the absolute value of |4.7|
Answers
Answer:
4.7
Step-by-step explanation:
Answer:
4.7
Step-by-step explanation:
The Phillipses traveled 717.2 miles to pick up their new boat. At the end of the trip, the odometer read
23,518.9 miles.
Answers
Answer:
what is the question?
Step-by-step explanation:
ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok ok
Find the area of the rectangle.
1 1/2 yd
58/ 8 yd
Answers
The area of the rectangle is calculated by L*B
The area of the rectangle is 10.875 yards^2.
How to calculate the area?l = 58/ 8 yd = 7.25yd
b = 1 1/2 yd = 1.5yd
Solution:
The area of the rectangle is calculated by L*B
Therefore,
L*B = 7.25* 1.5
= 10.875 yards^2.
The area of the rectangle is 10.875 yards^2.
To learn more about the area of the rectangle, refer
https://brainly.com/question/25292087
#SPJ13
Mrs. Bergstedt teaches four classes. Each class has 15 students. Her first class has 9 juniors, her second class has 12 juniors, her third class has 6 juniors, and her fourth class has 3 juniors. If Mrs. Bergstedt chooses four students at random, what is the probability that exactly two of the students are juniors (rounded to the nearest thousandth)?
Answers
The probability that exactly two of the students are juniors is given as follows:
0.270 = 27%.
What is the hypergeometric distribution formula?The mass probability formula is presented as follows:
\(P(X = x) = h(x,N,n,k) = \frac{C_{k,x}C_{N-k,n-x}}{C_{N,n}}\)
\(C_{n,x} = \frac{n!}{x!(n-x)!}\)
The parameters are:
x is the number of successes.N is the size of the population.n is the size of the sample.k is the total number of desired outcomes.The values of these parameters for this problem are given as follows:
\(N = 60, n = 4, k = 18\)
Hence the probability that exactly two of the students are juniors is calculated as follows:
\(P(X = x) = h(x,N,n,k) = \frac{C_{k,x}C_{N-k,n-x}}{C_{N,n}}\)
\(P(X = 2) = h(2,60,4,18) = \frac{C_{18,2}C_{42,2}}{C_{60,4}} = 0.270\)
More can be learned about the hypergeometric distribution at https://brainly.com/question/14741653
#SPJ1
What is 38581194 + 2484103 -12345779?
Answers
38581194+2484103-12345779=28719518
28719518
Answer:
28719518
Step-by-step explanation:
Plz mark me the brainlist
the weekly sales at two movie theaters were recorded for a random sample of 25 weeks. a 95 percent confidence interval for the difference in mean weekly sales for the two movie theaters was calculated as
Answers
To calculate the 95% confidence interval for the difference in mean weekly sales for the two movie theaters, follow these steps:
Step 1: Gather the data for the random sample of 25 weeks.
Step 2: Calculate the mean and standard deviation for each movie theater's weekly sales.
Step 3: Calculate the difference in mean weekly sales for the two movie theaters (subtract the mean of theater 2 from the mean of theater 1).
Step 4: Calculate the standard error of the difference by taking the square root of [(standard deviation of theater 1^2 / number of weeks) + (standard deviation of theater 2^2 / number of weeks)].
Step 5: Determine the critical value for a 95% confidence interval using a t-table or calculator (for a two-tailed test with 24 degrees of freedom, the critical value is approximately 2.064).
Step 6: Multiply the critical value by the standard error to get the margin of error.
Step 7: Calculate the lower and upper bounds of the confidence interval by subtracting and adding the margin of error from the difference in mean weekly sales.
The result will be the 95% confidence interval for the difference in mean weekly sales for the two movie theaters.
To know more about Standard deviation refer here:
https://brainly.in/question/35932722
#SPJ11
Arc length for xy I’ve been stuck on this for a while

Answers
Answer:
12.22
Step-by-step explanation:
There are 360° in a full circle
The circumference of a circle is c = 2πr
----------------------------------
The lenght of the arc will be the portion of the circumferece included in the angle
l = (∅/360) * 2πr
plug in the knowns
l = (70/360) * 2π(10)
l ≈ 12.22
Citrix Apps Apps CANVAS > Home EPB Intranet 7. -/2 points RogaCalcET4 13.5.017.Tutorial. Find r(t) and v(t) given a(t) and the initial velocity and position. a(t) = tk, v(0) = 4i, r(0) = 2; v(t) = r(t) = Additional Materials Tutorial +-12 points RogaCalcETA 19 rann
Answers
The position value, r(t) is equals to the (t³/6)k + 2j and velocity value, v(t) is equals to ( t²/2 )k + 4i , for a(t) = tk, v(0) = 4i, r(0) = 2j.
Acceleration is defined as the rate of change of the velocity of an object with respect to time. Accelerations are vector quanty.
a = dv/dt
We have the following informations are available,
Initial velocity, v(0) = 4i
Initial position, r(0) = 2j
Acceleration at any time "t",
a(t) = tk
we have to determine the value of v(t) and r(t).
As we know, a(t) = dv(t)/dt = tk
integrating the above equation ,
v(t) = ∫tk dt = ( t²/2 )k + c
at t = 0 , v(0) = 0 + c = 4i ( since, v(0) = 4i
=> c = 4i
So, v(t) = ( t²/2 )k + 4i
Also, velocity is calculated by derivative of postion (r) with respect to time.
=> v(t) = dr(t) /dt
=> r(t) = ∫ v(t) dt
=> r(t) = ∫ ( t²/2 )k dt
integrating value of the right hand side,
r(t) = ( t³/2×3 )k +d
= (t³/6)k + d
At t = 0, r(0) = (0/6)k + d
=> r(0) = d = 2j
so, r(t) = (t³/6)k + 2j
Hence, the required position and velocity are
(t³/6)k + 2j and ( t²/2 )k + 4i.
To learn more about Accelation , refer:
https://brainly.com/question/10307723
#SPJ4
Sony released 15,000 PS5’s online, 95% of them were purchased by bots. How many PS5’s were purchased by bots? How many PS5’s were left for everyone else?
Answers
Answer:
14250 purchased by bots, 750 purchased by humans.
Step-by-step explanation:
Answer:14250 were purchased by bots and 750 were left
Step-by-step explanation:95% of 15000 is 14250 so that's how many bots had bought ps5 so to find out how many people bought the ps5 you minus 14250 from 15000
4 inches 6 inches and 9 inches
v= in3
Answers
Answer:
what are you asking the total or what
In a certain sock drawer, there are 4 pairs of black socks, 3 pairs of gray socks and 2 pairs of orange socks. If socks are removed at random without replacement, what is the minimum number of socks that must be removed in order to ensure that two socks of the same color have been removed
Answers
Answer:
4 socks.
Step-by-step explanation:
There are three different colors of socks in the drawer: black, gray and orange.
The worst possible scenario for making a pair would be getting a different color of sock with the first three picks (1 black, 1 gray, 1 orange). Since we now have one of each, the next sock drawn from the drawer will form a pair with one of our three socks. Therefore, the minimum number of socks that must be removed in order to ensure that two socks of the same color have been removed is 4.
how many solutions can be found for the equation −4x − 11 = 2(x − 3x) 13? none one two infinitely many
Answers
Answer:
One x = 11/48
Step-by-step explanation:
Solve for x:
-4 x - 11 = 2×13 (x - 3 x)
x - 3 x = -2 x:
-4 x - 11 = 2×13×-2 x
2 (-2) = -4:
-4 x - 11 = -4×13 x
-4×13 = -52:
-4 x - 11 = -52 x
Add 52 x to both sides:
52 x - 4 x - 11 = 52 x - 52 x
52 x - 52 x = 0:
52 x - 4 x - 11 = 0
Grouping like terms, 52 x - 4 x - 11 = (-4 x + 52 x) - 11:
((-4 x + 52 x) - 11) = 0
52 x - 4 x = 48 x:
48 x - 11 = 0
Add 11 to both sides:
48 x + (11 - 11) = 11
11 - 11 = 0:
48 x = 11
Divide both sides of 48 x = 11 by 48:
(48 x)/48 = 11/48
48/48 = 1:
Answer: x = 11/48
Answer:
None.
Step-by-step explanation:
The lines do not intersect, so this equation has no solution.
The price of an item has been reduced by 20%. The original price was $20. What is the price of the item now?
Answers
Answer:
what type of work is this?
Can someone help me ?

Answers
Answer:
Can you please show the questions? It's way more easier
The table shows a linear function. What is the rate of change?
Hours
Pay
1
18
1.5
23
2
28
2.5
33
3
38

Answers
Answer:
rate of change = 5
Step-by-step explanation:
the slope m gives the measure of the rate of change of the function
calculate the slope m using the slope formula
m = \(\frac{y_{2-y_{1} } }{x_{2-x_{1} } }\)
with (x₁, y₁ ) = (3, 38) and (x₂, y₂ ) = (1, 18) ← 2 ordered pairs from the table
m = \(\frac{18-38}{1-3}\) = \(\frac{-20}{-2}\) = 5
then rate of change = 5
Using the results from the regression analysis in the Excel
document (Question 10), what is the estimated milk production
rounded to the nearest whole number?
A. 105,719 gallons of milk
B. 53 gallons
Answers
Based on the information provided, the estimated milk production rounded to the nearest whole number is 105,719 gallons of milk.
The estimated milk production value of 105,719 gallons is obtained from the regression analysis conducted in the Excel document. Regression analysis is a statistical technique used to model the relationship between a dependent variable (in this case, milk production) and one or more independent variables (such as time, weather conditions, or other relevant factors). The analysis likely involved fitting a regression model to the available data, which allows for estimating the milk production based on the variables considered in the analysis.
To know more about statistical technique here: brainly.com/question/32688529
#SPJ11
i need help I am haveing trouble understanding

Answers
Answer: y= x + 4
Step-by-step explanation:
What is monomial representations and symmetric presentations?
Answers
Answer: A monomial representation is a way to express a polynomial as a product of powers of its variables, where each power is a non-negative integer. For example, the polynomial 2x^3 + 4x^2 - 6x + 8 can be represented as a monomial representation of (2x^3)(x^2)(-6x)(8).
Symmetric polynomials are polynomials that are invariant under permutation of their variables. A symmetric presentation is a way of expressing a symmetric polynomial as a sum of elementary symmetric polynomials, which are defined as the sum of all possible products of variables taken i at a time, where i ranges from 1 to the number of variables. For example, the symmetric polynomial x^3 + y^3 + z^3 can be expressed as a symmetric presentation of x + y + z.
Step-by-step explanation:
17
The product of two positive numbers is
Calculate the numbers.
5/6
And their quotient is 15/8 calculate the number
Answers
Answer:
Let "x" and y" be the two numbers.
We have
xy = 5/6, (1) and x/y = 15/8. (2)
Multiply (1) and (2) (both sdes). You will get
x^2 = (5*15)/(6*8) = 25/16.
Hence, x = +/- 5/4.
Next, divide (1) by (2) (both sides). You will get
y^2 = 5/6:15/8 =
(5*8)/(6*15) = 4/9.
Hence, y=+/-2\/3.
Answer. There are two solutions: (x, y)=(5\/4,2\/3) and (x,y) = (-5/4,-2/3).
// We should exclude the second solution, since the numbers are negative.
Hi I really need help with this question please i really need Help (correct only)
Which data display could be used to represent the data set?
106, 94, 83, 115, 134, 122, 108, 99, 113, 125, 141, 137, 136, 144
line plot
stem-and-leaf plot
circle graph
bar graph
Answers
Answer:
I think (B) - Stem-and-Leaf Plot
Step-by-step explanation:
Answer:
Stem and leaf plot
Step-by-step explanation:
A Stem and Leaf Plot is a special table where each data value is split into a "stem" (the first digit or digits) and a "leaf" The "stem" values are listed down, and the "leaf" values go right (or left) from the stem values.
The "stem" is used to group the scores and each "leaf" shows the individual scores within each group.
List the sample space for rolling a fair eight-sided die.
S = {1}
S = {8}
S = {1, 2, 3, 4, 5, 6}
S = {1, 2, 3, 4, 5, 6, 7, 8}
Answers
The sample space for rolling a fair eight-sided die is {1, 2, 3, 4, 5, 6, 7, 8}.
We have to find the sample space for rolling a fair eight-sided die.
The sample space for rolling a fair eight-sided die consists of all the possible outcomes of a single roll of the die.
As the die has eight sides numbered from 1 to 8, the sample space can be represented as:
S = {1, 2, 3, 4, 5, 6, 7, 8}
Therefore, the sample space for rolling a fair eight-sided die is {1, 2, 3, 4, 5, 6, 7, 8}.
To learn more on probability click:
https://brainly.com/question/11234923
#SPJ1
In 2014, a survey stated that 51% of 650 randomly sampled North Carolina residents planned to set off fireworks on July 4th. a) Determine the margin of error for the 95% confidence interval for the proportion of North Carolina residents that plan to set off fireworks. Give your answer to three decimal places. Margin of Error = _____% b) How many randomly sampled residents do we need to survey if we want the 95% margin of error to be less than 3%? Sample size > _____ People
Answers
To find the required sample size for a margin of error less than 3%, we can rearrange the formula for the margin of error:
\(n = (Z^2 * p * (1 - p)) / (E^2)\)
Here, Z represents the critical value, p is the estimated proportion (0.51), and E is the desired margin of error (0.03)
To determine the margin of error for the 95% confidence interval, we need to use the formula:
Margin of Error = Critical value * Standard error
The critical value for a 95% confidence level can be obtained from the standard normal distribution table, which corresponds to 1.96. The standard error can be calculated using the following formula:
Standard error = \(\sqrt{(p * (1 - p) / n)}\)
Given that the proportion of North Carolina residents planning to set off fireworks is estimated to be 51% (0.51) based on the survey, we can substitute the values into the formula. However, the sample size (n) is not provided in the question, so we need to determine it in the next part.
To find the required sample size for a margin of error less than 3%, we can rearrange the formula for the margin of error:
\(n = (Z^2 * p * (1 - p)) / (E^2)\)
Here, Z represents the critical value, p is the estimated proportion (0.51), and E is the desired margin of error (0.03). Substituting these values into the formula, we can solve for the required sample size.
Learn more about standard normal distribution here:https://brainly.com/question/15103234
#SPJ11
Which of the equations shown will have infinitely many solutions? Select the TWO that apply. A 3x−1=3x+13 x minus 1 is equal to 3 x plus 1 B 2x−1=1−2x2 x minus 1 is equal to 1 minus 2 x C 3(x−1)=3x−33 times open paren x minus 1 close paren is equal to 3 x minus 3 D 2x+2=2(x+1)2 x plus 2 is equal to 2 times open paren x plus 1 close paren E 3(x−2)=2(x−3)
Answers
Answer:
Step-by-step explanation:
Am equation with infinite number of solution means that the equation has uncountable solutions.
Let's analyse each options
For the expression 3x-1 = 3x+1
Collect like terms
3x-3x = 1+1
0x = 2
Divide both sides by 0
x = 2/0
x = infinity
This shows that the expression 3x-1 = 3x+1 has infinite number of solution
Also considering the third expression
3(x-1) = 3x+3
Open the parenthesis
3x-3 = 3x+3
Collect like terms
3x-3x = 3+3
0x = 6
x = 6/0.
x = infinity
Thus also shows that expression 3(x-1) = 3x+3 has infinite number of solution.
3x-1 = 3x+1
3(x-1) = 3x+3
Two observers are 300 ft apart on opposite sides of a flagpole. The angles of
elevation from the observers to the top of the pole are 20°
and 15°. Find the
height of the flagpole.
Answers
A jar contains 56 quarters and pennies. The total value of the coins is $3.92. Which system of equations can be used to find p, the number of pennies, and q, the number of quarters?
Answers
Answer:
let x = the number of nickels
the number of pennies can be represented by (56 - x)
.05x + .01(56 - x) = 1.52
5x + (56 - x) = 152 if in the above line we multiply both sides of the equation by 100
4x + 56 = 152
4x = 96
x = 24
There are 24 nickels and 56 - 24 = 32 pennies
24 nickels are worth 24 * 5 = $1.20 and 32 pennies are obviously worth 32 cents, so the total is 1.20 + 0.32 = $1.52
Step-by-step explanation:
Find the value of x.
7
D
3
B В
х
С
X =

Answers
Answer:
x = \(\sqrt{30}\)
Step-by-step explanation:
Using the Altitude- on- Hypotenuse theorem
(leg of Δ ABC )² = (part of hypotenuse below it ) × ( whole hypotenuse )
x² = 3 × (3 + 7) = 3 × 10 = 30 ( take the square root of both sides )
x = \(\sqrt{30}\)
Ill give brainlest if right

Answers
Answer: The Car is moving at a Constant positive speed
Step-by-step explanation:
the car is going in a positive direction at a time in which doesn't change so it is at a constant speed
Find the equation of clean pulsations for a
left-mounted beam (for x=0) and simple pressed on the right (for
x=l) Take into account that: (sinx)^2+(cosx)^2=1
(chx)^2-(shx)^2=1
Answers
We can conclude that there are no nontrivial clean pulsations for the given left-mounted beam with a simple support on the right.
To find the equation of clean pulsations for a left-mounted beam with a simple support on the right, we can use the differential equation that describes the deflection of the beam. Assuming the beam is subject to a distributed load and has certain boundary conditions, the equation governing the deflection can be written as:
d^2y/dx^2 + (chx)^2 * y = 0
Where:
y(x) is the deflection of the beam at position x,
d^2y/dx^2 is the second derivative of y with respect to x,
ch(x) is the hyperbolic cosine function.
To solve this differential equation, we can assume a solution in the form of y(x) = A * cosh(kx) + B * sinh(kx), where A and B are constants, and k is a constant to be determined.
Substituting this assumed solution into the differential equation, we get:
k^2 * (A * cosh(kx) + B * sinh(kx)) + (chx)^2 * (A * cosh(kx) + B * sinh(kx)) = 0
Simplifying the equation and applying the given identity (chx)^2 - (shx)^2 = 1, we have:
(A + A * chx^2) * cosh(kx) + (B + B * chx^2) * sinh(kx) = 0
For this equation to hold for all values of x, the coefficients of cosh(kx) and sinh(kx) must be zero. Therefore, we get the following equations:
A + A * chx^2 = 0
B + B * chx^2 = 0
Simplifying these equations, we have:
A * (1 + chx^2) = 0
B * (1 + chx^2) = 0
Since we are looking for nontrivial solutions (A and B not equal to zero), the expressions in parentheses must be zero:
1 + chx^2 = 0
Using the identity (sinx)^2 + (cosx)^2 = 1, we can rewrite this equation as:
1 + (1 - (sinx)^2) = 0
Simplifying further, we get:
2 - (sinx)^2 = 0
Solving for (sinx)^2, we find:
(sin x)^2 = 2
Since the square of the sine function cannot be negative, there are no real solutions to this equation. Therefore, we can conclude that there are no nontrivial clean pulsations for the given left-mounted beam with a simple support on the right.
Learn more about simple support from
https://brainly.com/question/31510469
#SPJ11