There are 8 soccer teams for the 110 players in the league. The league wants the same number of players on each team. Which explains whether or not it is possible?
Answers
Answer:13 on each team but there wouldn’t be the Same amount
Step-by-step explanation:
Because you would divid 110 dived by 8 equals 13.75
Related Questions
System analysts define an object's attributes during the systems design process. true or false?
Answers
The statement "System analysts define an object's attributes during the systems design process" is true because defining object attributes is an essential part of the systems design process to ensure that the system meets the desired functional requirements.
In systems design, objects are used to represent real-world entities that are relevant to the system being developed. These objects have attributes that describe their characteristics or properties, which are used to identify and manipulate them within the system. System analysts define these attributes during the systems design process to ensure that the system meets the desired functional requirements.
For example, in a library system, a book object may have attributes such as title, author, publisher, and ISBN. Defining these attributes helps ensure that the system can properly manage and retrieve books as needed. Object-oriented design is a popular approach to systems design that relies heavily on defining objects and their attributes.
Learn more about Object-oriented design here
brainly.com/question/28731103
#SPJ4
PLEASE HELP!! (15 points!)

Answers
\( ({5}^{ - 8} )( {5}^{ - 10} ) = {5}^{ - 8 - 10} = {5}^{ - 18} \)
I Hope This Can Help You ^_^
Graph the reflection of the polygon in the given line #11
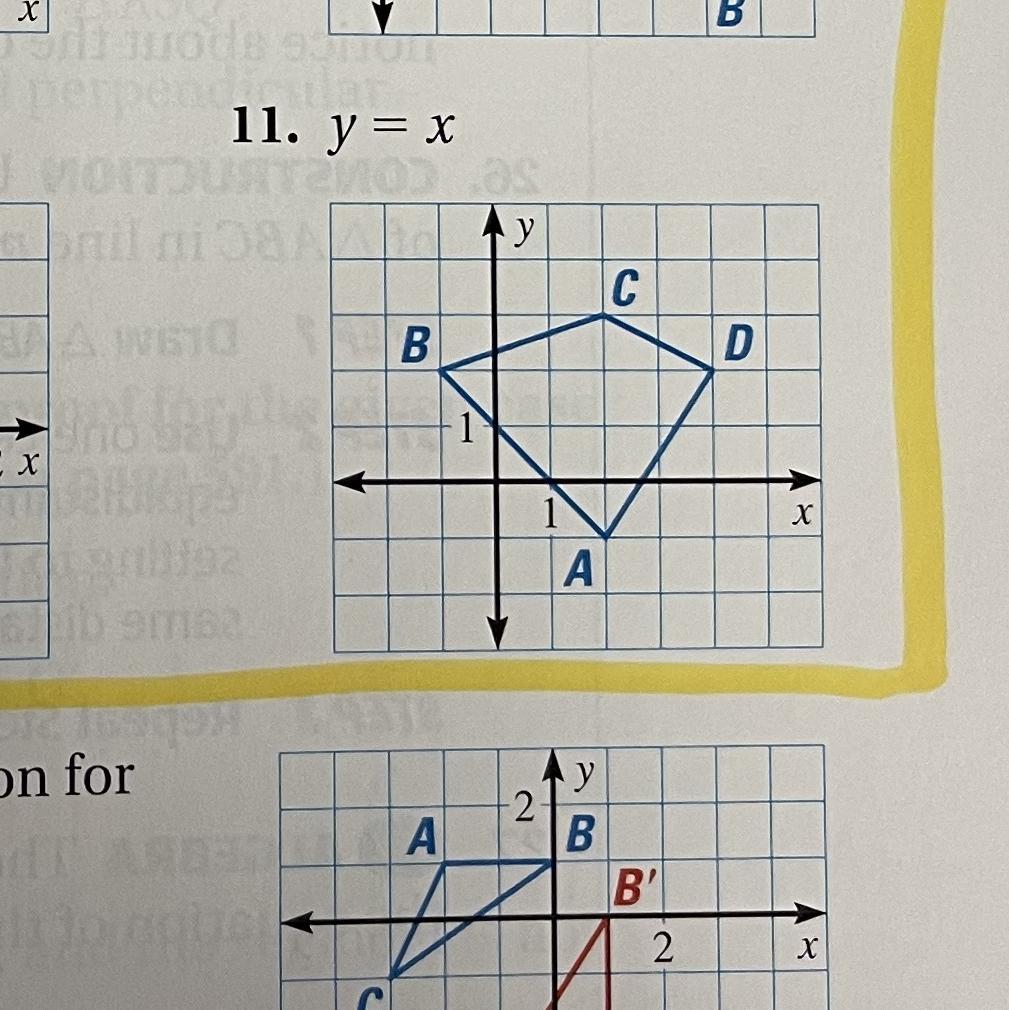
Answers
Let:
\(\begin{gathered} A=(2,-1) \\ B=(-1,2) \\ C=(2,3) \\ D=(4,2) \end{gathered}\)After a reflection across the line y = x:
\(\begin{gathered} A->(y,x)->A^{\prime}=(-1,2) \\ B->(y,x)->B^{\prime}=(2,-1) \\ C->(y,x)->C^{\prime}=(3,2) \\ D->(y,x)->D^{\prime}=(2,4) \end{gathered}\)
Mike had $70 of credit at an electronics store. He bought a package of DVDs for $20. a computer mouse for $17 and a programming book for $15. How much store credit does Mike have left? *
Answers
Answer:
18
Step-by-step explanation:
20+17+15=52
70-52=18
Hope it Helps
Answer:
70-(20+17+15)=$18
$18 of store credit left
2. The function ln(x)2 is increasing. If we wish to estimate √ In (2) In(x) dx to within an accuracy of .01 using upper and lower sums for a uniform partition of the interval [1, e], so that S- S < 0.01, into how many subintervals must we partition [1, e]? (You may use the approximation e≈ 2.718.)
Answers
To estimate the integral √(ln(2)) ln(x) dx within an accuracy of 0.01 using upper and lower sums for a uniform partition of the interval [1, e], we need to divide the interval into at least n subintervals. The answer is obtained by finding the minimum value of n that satisfies the given accuracy condition.
We start by determining the interval [1, e], where e is approximately 2.718. The function ln(x)^2 is increasing, meaning that its values increase as x increases. To estimate the integral, we use upper and lower sums with a uniform partition. In this case, the width of each subinterval is (e - 1)/n, where n is the number of subintervals.
To find the minimum value of n that ensures the accuracy condition S - S < 0.01, we need to evaluate the difference between the upper sum (S) and the lower sum (S) for the given partition. The upper sum is the sum of the maximum values of the function within each subinterval, while the lower sum is the sum of the minimum values.
Since ln(x)^2 is increasing, the maximum value of ln(x)^2 within each subinterval occurs at the right endpoint. Therefore, the upper sum can be calculated as the sum of ln(e)^2, ln(e - (e - 1)/n)^2, ln(e - 2(e - 1)/n)^2, and so on, up to ln(e - (n - 1)(e - 1)/n)^2.
Similarly, the minimum value of ln(x)^2 within each subinterval occurs at the left endpoint. Therefore, the lower sum can be calculated as the sum of ln(1)^2, ln(1 + (e - 1)/n)^2, ln(1 + 2(e - 1)/n)^2, and so on, up to ln(1 + (n - 1)(e - 1)/n)^2.
We need to find the minimum value of n such that the difference between the upper sum and the lower sum is less than 0.01. This can be done by iteratively increasing the value of n until the condition is satisfied. Once the minimum value of n is determined, we have the required number of subintervals for the given accuracy.
Learn more about integral here : brainly.com/question/31059545
#SPJ11
a cyclist travels 8km in the same time that a walker travels 3km. the speed of the cyclist is 8km more than the speed of the walk. find the speed of the cyclist and the speed of the walker.
Answers
Conclusion: The speed of the cyclist is 11 km/hr and the speed of the walker is 3 km/hr.
The speed of the cyclist is 11 km/hr and the speed of the walker is 3 km/hr.
To calculate the speed of the cyclist and the speed of the walker,
we need to use the formula for speed, which is distance divided by time.
The cyclist travels 8 km in the same time that the walker travels 3 km, so the time taken is the same for both. Let's call this time t.
The speed of the cyclist is 8 km/t and the speed of the walker is 3 km/t. To find the speed of the cyclist, we need to add 8 km to the speed of the walker.
Therefore, the speed of the cyclist is 11 km/hr and the speed of the walker is 3 km/hr.
for such more questions speed of the cyclist
https://brainly.com/question/28828724
#SPJ11
mitchell drives a taxi cab in new york. he charges people .75 for every mile and $3 upon entering the taxi. which equation best represents this situation?
Answers
The initial condition is $3.
The constant rate of change is 0.75 for every mile.
Based on the given information, we can express the following
\(y=0.75x+3\)Where x is miles, and y is the cost.
Write the equation of the line with the slope of -6 passing through the point ( − 8 , 0 ).
Answers
Answer:
y=-6x+48
Step-by-step explanation:
Given the set of all even integers between and including -18 to -6,
what is the probability of choosing a multiple of -6 from this set?
Answers
Answer:
3/7
Step-by-step explanation:
Given set is {-18,-16,-14,-12,-10,-8,-6}
Probability = Number of favorable outcome / Number of possible outcomes
Favorable outcomes ={ -18, -12, -6}
probability of choosing a multiple of -6 = 3/7
i need help solving this system of equations with elimination method
8x-5y=17
-8x+5y=-21
Answers
it becomes -8x+5y=17
then you add them to each other which makes
-8x-8x+5y-5y=17-21
-16x=-4
x=0.25
8x=2
5y=15
y=-3
A coin is flipped five times. find the number of possible sets of heads and tails that have 2 heads.
Answers
The number of possible sets of heads and tails that have 2 heads is 11.
Given,
There is one coin and it is flipped five times.
First, we have to list out the outcome when we toss the coin five times.
(HHHHH), (HHHHT), (HHHTT), (HHTTT), (HTTTT), (TTTTT), (TTTTH), (TTTHH), (TTHHH), (THHHH), (THTHT), (HTHTH), (THHTT), (THHHT), (HTTTH), (HTTHH), (HTHHH), (THTTT), (TTHTH), (TTTHT), (TTHHT), (HHTHH), (TTHTT), (HHTTH), (HHTHT), (HTHTT), (THTHH), (THTTH), (HTHHT), (HHHTH)
Now, we can list out the possible sets with 2 heads.
(HHTTT), (TTTHH), (THTHT), (HTTHT), (HTTTH), (THHTT), (TTHHT), (TTHTH), (HTHTT), (THTTH), (HTHTT)
So, the number of possible sets of heads and tails that have 2 heads is 11.
Learn more about coin here: https://brainly.com/question/24158973
#SPJ4
h(x) = x²- 5x + 7
a). h(2)
b).h(-5)
c). h(-8)
Answers
describe transformation of \(y= \frac{x^{4} }{6x+6}\)
Answers
The transformation of \(\(y= \frac{x^{4} }{6x+6}\)\) graph is compressed by a factor of 2.
The function is a rational function in which the numerator is a degree 4 polynomial, and the denominator is a degree 1 polynomial.
Rational functions are in the form of \(\(f(x) = \frac{p(x)}{q(x)}\)\), where p(x) and q(x) are polynomial functions and the degree of p(x) is less than the degree of q(x).
To transform a graph of a function, one can translate, stretch, or reflect it.
Here are some of the transformations of the function \(\(y= \frac{x^{4} }{6x+6}\)\)
Vertical Shift: To get the new graph, we add or subtract a constant from the function.
When f(x) is replaced with f(x) + k, we get a graph that is k units above the graph of f(x). In this case, if we subtract 2 from the equation, we get the following equation:
\(y = (x^4) / (6x+6) - 2\)
This means that the graph of the function is shifted 2 units downwards.
Horizontal Shift: To get the new graph, we add or subtract a constant to the variable.
When f(x+c) is replaced with f(x), the graph is shifted c units to the left.
When f(x-c) is replaced with f(x), the graph is shifted c units to the right.
In this case, we can shift the graph by substituting x-2 for x in the function.
The new function is: \(y = ((x-2)^4) / (6(x-2)+6)\)
This means that the graph is shifted 2 units to the right.
Vertical Stretch/Compression: When a constant is multiplied to the function, the graph is vertically stretched or compressed.
In this case, if the function is multiplied by 2, we get the following function:
\(y = 2(x^4) / (6x+6)\)
This means that the graph is compressed by a factor of 2.
It can also be stretched by dividing the function by a constant.
Horizontal Stretch/Compression:
When a constant is multiplied to the variable of the function, the graph is horizontally stretched or compressed. In this case, if the variable is divided by 2, we get the following function:
\(y = (x^4) / (3x+3)\)
This means that the graph is compressed by a factor of 2.
It can also be stretched by multiplying the variable by a constant.
For more related questions on transformation:
https://brainly.com/question/11709244
#SPJ8
f(x)=x+5
g(x)=\sqrt 3x+1
find f/g and f-g then give their domains using interval notation
Answers
\( \frac{f(x)}{g(x)} = \frac{x + 5}{ \sqrt{3x + 1} } \)
\(x ∈ \: \: ] \frac{ - 1}{3} ,∞[\)
\(f(x) - g(x) = x + 5 - \sqrt{3x + 1} \)
\(x ∈ \: \: [ \frac{ - 1}{3} ,∞[\)
The levine family gas 10 gallons of gas in the car. The car uses 1 5/8 of a gallon each hour. How long can they drive on 10 gallons of gas?.
Answers
Answer:
6 hours
Step-by-step explanation:
6 * 1 5/8
6* 13/8=9.75
Answer:
6 hours
Step-by-step explanation:
help me Please!!!!!!

Answers
Answer:
Step-by-step explanation:
Angle QRS is an inscribed angle. The rule is that an inscribed angle is half the measure of the arc it cuts off; in other words, the measure of the arc is double the measure of its inscribed angle. If the angle is 84, then the arc is
84(2) = 168.
Jake needs 5 cents to buy a tootsie roll. His piggy bank contains 5 quarters, 7 nickels, 14 pennies, and 6 dimes. What is the probability that, without looking, Jake grabs a nickel from his piggy bank?
P(nickel)=
options:
0.02
0.61
0.22
0.16
Answers
The probability that Jake grabs a nickel from his piggy bank without looking is 7/32 or approximately 22%.
To find the probability, we need to determine the total number of coins in Jake's piggy bank and the number of nickels.
Total number of coins = 5 quarters + 7 nickels + 14 pennies + 6 dimes
= 5 + 7 + 14 + 6
= 32
Number of nickels = 7
The probability of Jake grabbing a nickel without looking can be calculated as follows:
Probability = (Number of favorable outcomes) / (Total number of possible outcomes)
In this case, the number of favorable outcomes is the number of nickels, which is 7.
The total number of possible outcomes is the total number of coins, which is 32.
Probability of grabbing a nickel = 7 / 32
Therefore, the probability that Jake grabs a nickel from his piggy bank without looking is 7/32 or approximately 22%.
Learn more about probability click;
https://brainly.com/question/32117953
#SPJ1
BRAINLY IF CORRECT!!
In △ A B C , A B = 7 , B C = 12 , and m ∠ A B C = 82 ∘
In △ D E F , D E = 7 , E F = 12 , and m ∠ D E F = ( 2 x − 6 ) ∘
A C > D F.
What is the range of possible values for x?
(a) 44 < x < 180
(b) 3 < x < 44
(c) 0 < x < 44
d) none of these
(e) x > 44
(f) 44 < x < 93
(g) x < 44
Answers
The possible range of angle x is x < 44
How to solve for angle xgiven data
In △ A B C , A B = 7 , B C = 12 , and m ∠ A B C = 82 ∘
In △ D E F , D E = 7 , E F = 12 and m ∠ D E F = ( 2 x − 6 ) ∘
A C > D F
Solution for angle x is gotten from the clue in A C > D F
This means that angle included by m ∠ A B C = 82 ∘ is greater than the angle included by m ∠ D E F = ( 2 x − 6 ) ∘
We therefore say that
82 ∘ > ( 2 x − 6 ) ∘
82 + 6 > 2 x
88 > 2 x
divide both sides by 2
88 / 2 > 2 x / 2
44 > x
x < 44 degrees
Hence option g is the correct answer
Read more on inequalities here: https://brainly.com/question/25944814
#SPJ1
the right triangle shown below with hypotenuse 13 inches long and vertical leg 5 inches long is rotated 360c around the vertical leg to form a right circular cone. what is the volume of this cone, in cubic inches?
Answers
The cone has a volume of (D) 288in3.
What do we mean by volume?A volume is a unit of measure of occupied three-dimensional space. It is frequently quantified numerically using SI-derived units (such as cubic meters and liters) or various imperial modules (such as the gallon, quart, and cubic inch). The definition of volume is related to the definition of length (cubed). The volume of a container is generally recognized to be its capacity; that is, the quantity of fluid (gas or liquid) that it can hold, rather than the amount of space that it occupies.To find the volume:
Given:
Radius = 6 inheight =?g = 10 inVolume =?Formula:
Volume of a cone = πr²h / 3The Pythagorean theorem is used to calculate height.
height² = 10² - 6² = 100 - 36 = 64 height = 8The volume of the cone:
Volume = π(6)²(8) Volume = π(36)(8) Volume = 288π in³Therefore, the volume of the cone is (D) 288in³.
Know more about volumes here:
https://brainly.com/question/1972490
#SPJ4
The complete question is given below:
Right triangle ABC, shown, has a vertical leg 6 inches long and a hypotenuse 10 inches long. If the triangle was rotated 360 degrees around the vertical leg to form a right circular cone, what would be the volume of this cone, in cubic inches?
A. 32
B. 96
C. 128
D. 288
E. 384

Range of F(x) = x^2 + x: {-1.0.2}
Answers
Answer:
hugufydyfud5sdjfud5pdjf6dufjchfhcgx
Why tan(π/4) = 1 can you explain it simply? Also can we use it with cos, cot, sin, sec and cosec? Thanks! please, please dont write random answers it may be reported.
Answers
Answer:
1) tan π/4 = 1
2) cos π/4 = 0.7071
3) cot π/4 = 1
4) sin π/4 = 0.7071
5) sec π/4 = 1.4142
6) cosec π/4 = 1.4142
Step-by-step explanation:
Angle π is in radians
But converting to degree, it is;
π = 180˚
Thus; π/4 = 180/4 = 45°
Thus;
1) from calculator; tan π/4 = tan 45° = 1
2) from calculator, cos π/4 = cos 45° = 0.7071
3) cot is equivalent to 1/tan
Thus; cot π/4 = 1/(tan π/4) = 1/tan 45 = 1/1 = 1
4) from calculator, sin π/4 = sin 45° = 0.7071
5) sec in trigonometry is equivalent to 1/cos.
Thus;
sec π/4 = 1/cos π/4 = 1/cos 45° = 1/0.7071 = 1.4142
6) cosec in trigonometry is equivalent to 1/sin
Thus;
cosec π/4 = 1/sin π/4 = 1/sin 45° = 1/0.7071 = 1.4142
What is the approximate value of b, rounded to the nearest tenth? Use the law of sines to find the answer. Law of sines: answers- 1. 1.9 units 2. 4.7 units 3. 5.0 units 4. 5.7 units
Answers
Answer:
\(\boxed{\text{2. 4.7 units}}\)
Step-by-step explanation:
Assume your triangle has the dimensions shown below.
Before we can use the Law of Sines, we must find ∠C.
1. Calculate ∠C
\(\begin{array}{rcl}A + B + C & = & 180^{\circ}\\66^{\circ} + 76^{\circ} + C & = & 180^{\circ}\\142^{\circ} + C & = & 180^{\circ}\\C & = & 38\, ^{\circ}\\\end{array}\)
2. Calculate b
Use the Law of Sines
\(\begin{array}{rcl}\dfrac{b}{\sin B } & = & \dfrac{c}{\sin C}\\\\\dfrac{b}{\sin 76^{\circ}} & = & \dfrac{3}{\sin 38^{\circ}}\\\\\dfrac{b}{0.9703} & = & \dfrac{3}{0.6157}\\\\ & = & 4.873\\b & = & 0.9703\times 4.873\\& = & \boxed{\mathbf{4.7}}\\\end{array}\)

p.557(3-6 all): Similar Triangles and Indirect Measurement
I need helppp :/ please

Answers
Answer:
3. 200ft
4. 21ft
5. 37.5m
6. 4.2ft
Step-by-step explanation:
3. use proportions:
\(\frac{h}{50} = \frac{50}{12.5}\)
50(50) = 12.5h
h = 200ft
4.
\(\frac{h}{6} =\frac{7}{2}\)
6(7) = 2h
h = 21ft
5.
\(\frac{25}{8} =\frac{x}{12}\)
25(12) = 8x
x = 37.5m
6.
\(\frac{9}{15} =\frac{h}{7}\)
9(7) = 15h
h = 4.2ft
Can you please help on this question below

Answers
5/8=x/y so cross multiply and 5y=8x
x+102=y because there are 102 more blue beads than green
use substitution for y
5(x+102)=8x
5x+510=8x
510=3x
x=170 y=272
check answer by dividing 170/272=5/8 and it is correct
Solve the following equation. Then place the correct number in the box provided. Leave answer in terms of a mixed number.
6(2P - 1) > 5P + 2
Answers
Answer:
Since theres no box provided, all I can say Is the answer is p> 7/8
Step-by-step explanation:
Rearrange the equation by subtracting what is to the right of the greater than sign from both sides of the inequality :
6*(2*p-1)-(5*p+2)>0
STEP 1:
Equation at the end of step 1
6 • (2p - 1) - (5p + 2) > 0
STEP 2:
Equation at the end of step 2
7p - 8 > 0
STEP 3:
3.1
Divide both sides by 7
p-(8/7) > 0
Answer:
the correct answer is p> 1 1/7
Step-by-step explanation:
Jill borrows a pencil and returns it after the Warm Up. She then borrows a different pencil to show work on her CFA. The probability of selecting a mechanical pencil is 20%. The probability of selecting a wooden pencil is 40%. The probability of selecting a colored pencil is 30% What is the probability that Jill selects a wooden pencil and then a mechanical pencil?
Answers
Probabilities are used to determine the chances of events
The probability that Jill selects a wooden pencil and then a mechanical pencil is 8%
How to calculate the probabilityRepresent the events as follows:
A represents mechanical pencilB represents wooden pencilC represents colored pencilSo, we have
P(A) = 20%
P(B) = 40%
P(C) = 30%
The probability that Jill selects a wooden pencil and then a mechanical pencil is then calculated as:
P(B n A) = P(B) * P(A)
This gives
P(B n A) = 40% * 20%
Evaluate the product
P(B n A) = 8%
Hence, the probability that Jill selects a wooden pencil and then a mechanical pencil is 8%
Read more about probabilities at:
https://brainly.com/question/9385303
Whitch expression has greatest value 8 3/2 4 5/2 100 1/2 9 3/2 8 2/3
Answers
The expression "100 1/2" has the greatest value among the given options.
To determine which expression has the greatest value among the given options, we need to evaluate each expression and compare the results.
Let's calculate the value of each expression:
8 3/2 = 8 + 3/2 = 16/2 + 3/2 = 19/2
4 5/2 = 4 + 5/2 = 8/2 + 5/2 = 13/2
100 1/2 = 100 + 1/2 = 200/2 + 1/2 = 201/2
9 3/2 = 9 + 3/2 = 18/2 + 3/2 = 21/2
8 2/3 = 8 + 2/3 = 24/3 + 2/3 = 26/3
Now, let's compare the values:
19/2 ≈ 9.5
13/2 ≈ 6.5
201/2 ≈ 100.5
21/2 ≈ 10.5
26/3 ≈ 8.67
From the calculations, we can see that the expression with the greatest value is "100 1/2," which is approximately 100.5.
For similar question on expression.
https://brainly.com/question/1859113
#SPJ8
Translate this sentence into an equation.28 is the product of Mai's savings and 2.Use the variable m to represent Mai's savings.N+ローロロメロx 6?

Answers
2m = 28
Explanations:Given the following parameter
Mai's savings = m
The product of Mai's savings and 2 is given as:
\(\text{Product of Mai's savings and 2 = 2m}\)If 28 is equal to the product of Mai's savings and 2, the resulting expression will be given as:
28 = 2m
Hence the required equation is 2m = 28
a measurable part of a line consisting of two endpoints is called___
Answers
Answer:
Line Segment
Step-by-step explanation:
11/50 as a percentage
Answers
22% is the answer
Step-by-step explanation:
4. Ito ay baluktot na gawaing may kinalaman sa pagpapayaman nang
ilegal.
