The sum of 3 consecutive numbers is 126. Find the numbers.
Answers
Answer:
Hello!!
The sum of 3 constructive numbers is 126. The three constructive numbers are...
\(41+42+43=126\)
Hope this helps!!
Related Questions
Given that f(x) = x + 4 and g(x) = x + 7, find (g - 4(x).
Answers
Answer: The value of \((g - f)(x)=4 .\)
Step-by-step explanation:
Given functions : \(f(x) = x + 4\) and \(g(x) = x + 7\)
To find : \((g - f)(x)\)
Difference between two functions: \((u-v)(x)=u(x)-v(x)\)
Then, \((g-f)(x)=g(x)-f(x)\)
\(=(x+7)-(x+4)=x+7-x-4\\\\=7-4=3\)
Hence, the value of \((g - f)(x)=4 .\)
Help me ASAP plsssssssssssssssss

Answers
Answer:
4 and 9 hundreths
Step-by-step explanation:
1. A school gym has a width of 270 ft. Which of the following is equivalent to 270 ft?
90 yd
810 yd
9 yd
Not here

Answers
Answer:
It should be 90
Step-by-step explanation:
Because 270 divided by 3 is 90
Which of the following equations are equivalent? Select three options. 2 + x = 5 x + 1 = 4 9 + x = 6 x + (negative 4) = 7 Negative 5 + x = negative 2
Answers
The three equivalent equations are 2 + x = 5, x + 1 = 4 and -5 + x = -2. So, correct options are A, B and E.
Two equations are considered equivalent if they have the same solution set. In other words, if we solve both equations, we should get the same value for the variable.
To determine which of the given equations are equivalent, we need to solve them for x and see if they have the same solution.
Let's start with the first equation:
2 + x = 5
Subtract 2 from both sides:
x = 3
Now let's move on to the second equation:
x + 1 = 4
Subtract 1 from both sides:
x = 3
Notice that we got the same value of x for both equations, so they are equivalent.
Next, let's look at the third equation:
9 + x = 6
Subtract 9 from both sides:
x = -3
Since this value of x is different from the previous two equations, we can conclude that it is not equivalent to them.
Now, let's move on to the fourth equation:
x + (-4) = 7
Add 4 to both sides:
x = 11
This value of x is also different from the first two equations, so it is not equivalent to them.
Finally, let's look at the fifth equation:
-5 + x = -2
Add 5 to both sides:
x = 3
Notice that we got the same value of x as the first two equations, so this equation is also equivalent to them.
So, correct options are A, B and E.
To learn more about equations click on,
https://brainly.com/question/29266004
#SPJ1
Complete question is:
Which of the following equations are equivalent? Select three options.
2 + x = 5
x + 1 = 4
9 + x = 6
x + (- 4) = 7
- 5 + x = - 2
what is the expanded form of 5.629
Answers
Answer:
5
+ 0.6
+ 0.02
+ 0.009
Step-by-step explanation:
5 × 1
+ 6 × 0.1
+ 2 × 0.01
+ 9 × 0.001
5 × 100
+ 6 × 10-1
+ 2 × 10-2
+ 9 × 10-3
all of them are correct
The scatter plot shows the relationship between the number of coffee drinks sold and the total expenses of a coffee shop.
The slope of the line is....
The intercept of the line is.....
The regression equation is
Answers
For the linear function built from the scatter plot, it is found that:
The slope of the line is of: 5.The intercept of the line is of: b = 500.The equation is of: y = 5x + 500.What is a linear function?The slope-intercept representation of a linear function is the rule presented as follows:
y = mx + b
The coefficients of the function and their meaning are listed as follows:
m is the slope of the function, representing the change in the numeric value of the output variable y when the input variable x increases by one.b is the y-intercept of the function, which is the numeric value of the output variable y when the input variable x has a value of 0.From the graph, we have that when x = 0, y = 500, hence the intercept of the line is:
b = 500.
When the input increases by 200, the output increases by 1000, hence the slope is:
m = 1000/2 = 5.
Thus the equation is:
y = 5x + 500.
Missing InformationThe graph is given by the image at the end of the answer.
More can be learned about linear functions at https://brainly.com/question/24808124
#SPJ1

The circle below is centered at the point (4, 1) and has a radius of length 2.
What is its equation?
10
A. (x-4)² + (v-1)2 = 4
B. (x-1)2 + (v-4)2 = 4
C. (x + 1)2 + (v-4)² =
22
D. (x+4)2 + (y- 1)²2 = 4
Answers
Answer:
A
Step-by-step explanation:
the equation of a circle in standard form is
(x - h)² + (y - k)² = r²
where (h, k ) are the coordinates of the centre and r is the radius
here (h, k ) = (4, 1 ) and r = 2 , then
(x - 4)² + (y - 1)² = 2² , that is
(x - 4)² + (y - 1)² = 4 ← equation of circle
A polygon with an area of 10 square inches is dilated by a scale factor of 4, what is the new area?
Answers
The new area would be 40 square inches. When a scale factor dilates a polygon, the area is multiplied by the square of the scale factor. If the original area is 10 square inches and the scale factor is 4, the new area would be 10 x 4^2 = 10 x 16 = 40 square inches.
On its own, 5 � � 5ab5, a, b is the product of
Answers
The product of 5 × 5ab × 5, a, b is 125ab5 when written in its most Simple form
The expression 5 × 5ab × 5, a, b is equal to 125ab5. The reasoning is that when we multiply 5 by 5ab, we get 25ab, which we then multiply by 5 to get 125ab. Since a, b are variables, the product 125ab5 is the product of 5 × 5ab × 5, a, b, when written in its most simple form.
The expression 5 × 5ab × 5, a, b is an algebraic expression, which consists of numbers, variables, and operators. Variables are usually represented by letters or other symbols, while operators such as +, -, ×, ÷, ^ are used to indicate mathematical operations such as addition, subtraction, multiplication, division, and exponentiation. In algebra, expressions can be simplified by combining like terms, expanding brackets, and applying other rules of arithmetic.
The most simple form of an expression is the one that cannot be further simplified.In the given expression, 5 × 5ab × 5, a, b, we can see that the number 5 appears three times, so we can simplify the expression by multiplying the numbers together.
Similarly, we can multiply the variables a and b together to get the term ab. Finally, we write the result as 125ab5, which is the product of 5 × 5ab × 5, a, b, in its most simple form.
Therefore, the product of 5 × 5ab × 5, a, b is 125ab5 when written in its most simple form.
For more questions on Simple form.
https://brainly.com/question/27624273
#SPJ8
A spinner is spun `40` times for a game.
This graph shows the fraction of games that are wins.
Based on the graph, what is the probability of a spin winning this game?

Answers
(i) In decimals: 0.65
(ii) In percentage: 65%
The graph shows that after the spinner is spun after 40 times. The Fraction of wins is 0.65 out of 1. Look at the very end of the graph to look at the conclusion for probability. Similar cases when, after the spinner is spun after 20 times, the probability is 0.65.
Answer:
0.65 or 65%
Step-by-step explanation:
Approximate 5.7255 to the nearest thousand
Answers
round 5.7255 to thousands place
place after thousands place (5) rounds up the 5 before it
therefore 5.726 ur ans
MARK above ANS as branliest
y=3x-11
y-3x=-13
Solving using systems of equations
Answers
Answer: There are no possible solutions because
If you were to plot these two equations on the graph you would notice they are parallel because both have the same slope (3x) but still have different intercepts. This means there are no possible solutions as in order to have a solution for x and y they need to intercept and the point of interception is the solution for x and y but that cannot happen in a system of equations that result in parallel lines ( they’ll never intercept)

Answer:
Ø
Step-by-step explanation:
These equations are parallel, meaning they have SIMILAR rate of changes [slopes]. To prove it, convert the second equation from Standard Form to Slope-Intercept Form:
\(\displaystyle y - 3x = -13 \hookrightarrow y = 3x - 13 \\ \\ \left \{ {{y = 3x - 13} \atop {y = 3x - 11}} \right.\)
As you can see, both equations have a rate of change of 3, meaning we CANNOT obtain a solution here, therefore we have no solution.
I am joyous to assist you at any time.
Tell whether x and y show direct variation, inverse variation or - y/4 = 2x
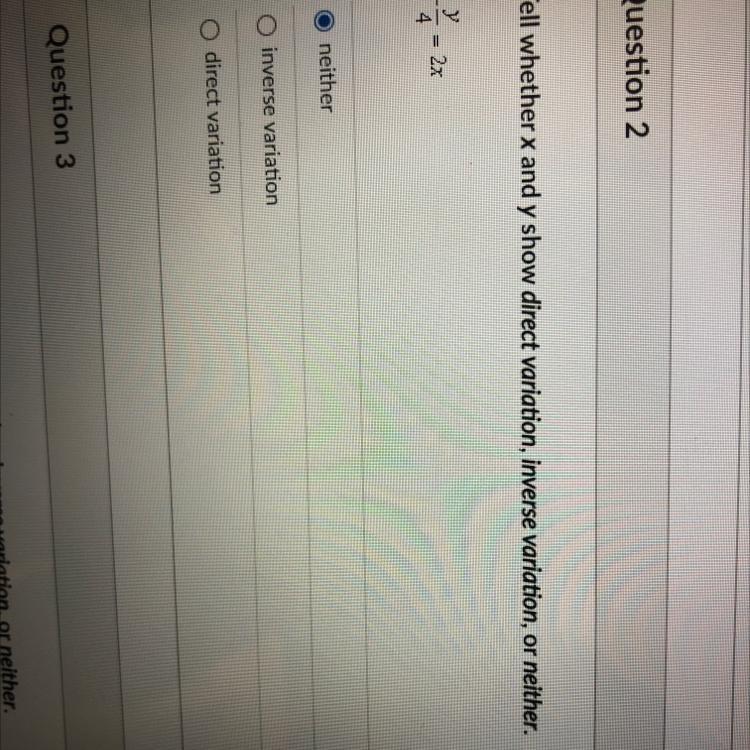
Answers
9514 1404 393
Answer:
direct variation
Step-by-step explanation:
The given equation can be written in the direct variation form ...
y = kx
y = -8x . . . . rewrite of the given equation (multiply by -4)
This is an example of direct variation.
Which value is a solution of 7/4x^2-2=-0.5+4?
Answers
Answer:
x=√154 over 7 , −√154 over 7
Step-by-step explanation:

Answer:
the answer is that x=positive or negative square root of 22 over square root of 7
Step-by-step explanation:
A rectangle has a length of 26 inches and a width of 14 inches. If 1 inch = 2.54 centimeters, find the area of the rectangle in centimeters. Round the answer to the nearest tenth.
HURRY PLEASE
560.4 cm2
56.4 cm2
2,348.4 cm2
924.6 cm2
Answers
The area of the rectangle of length 26 inches and width of 14 inches is 2348.4 cm²
What is area?Area is the total region bounded by a plane shape.
To calculate the area of the rectangle, we use the formula below.
Formula:
A = LW......... Equation 1Where:
A = Area of the rectangleL = Length of the rectangleW = Width of the rectangleFrom the question,
Given:
L = 26 inches = (26×2.54) = 66.04 cmW = 14 inches = (14×2.54) = 35.56 cmSubstitute these values into equation 1
A = (66.04×35.56)A = 2348.4 cm²Hene, the area of the rectangle is 2348.4 cm²
Learn more about area here: https://brainly.com/question/25292087
#SPJ1
1
7. There are 17 more boys than girls at Juab Junior High School. If there are
a total of 981 students there, how many students are girls?
Answers
Would appreciate brainliest!
help i need a answer asap

Answers
The number of times Jennifer rolls a number less than 3 in a number cube when she rolls it 60 times is 40.
How to find the probability of an event?The probability of an event is the ratio of number of favorable outcome to the total number of outcome of that event.
The probability of rolling a number less than 3 on a number cube is 2/6.
\(P( > 3)=\dfrac{2}{6}\)
Here, the Jennifer rolls a number cube 60 times. Thus, the number of times she rolled the number less than 3 in this cube is,
\(n=\dfrac{2}{3}\times60\\n=40\)
Thus, the number of times Jennifer rolls a number less than 3 in a number cube when she rolls it 60 times is 40.
Learn more about the probability here;
https://brainly.com/question/24756209
#SPJ1
an application is using a two-dimensional list defined as follows: write a statement that creates an empty two-dimensional list named values with 4 rows and 3 columns. write nested loops that get an integer value from the user for each element in the list, example: values
Answers
Complete Question :
An application is using a two-dimensional list defined as follows: 1. Write a statement that creates an empty two-dimensional list named values with 4 rows and 3 columns. 2. Write nested loops that get an integer value from the user for each element in the list, for example: values= [[1, 2, 3], [10, 20, 30], [100, 200, 300],[1000, 2000, 3000]] 3. Write a function named row_values that accepts values as argument and returns the sums of each row and displays the result as a list named row 4. Write a function named column_values that accepts values as arguments and returns the sums of each column and displays the result as a list named column 5. Write a function called sum_values that sums all the elements of the array and displays the result.
Sum of rows : [ 6, 60, 600, 6000 ]
Sum of columns : [ 1111, 2222, 3333]
Sum of all elements : 6666
The nested loops are :
Enter a number at row 0, and column 0 : 1
Enter a number at row 0, and column 1 : 2
Enter a number at row 0, and column 2 : 3
Enter a number at row 1, and column 0 : 10
Enter a number at row 1, and column 1 : 20
Enter a number at row 1, and column 2 : 30
Enter a number at row 2, and column 0 : 100
Enter a number at row 2, and column 1 : 200
Enter a number at row 2, and column 2: 300
Enter a number at row 3, and column 0 : 1000
Enter a number at row 3, and column 1 : 2000
Enter a number at row 3, and column 2 : 3000
To read more about nested loops, visit https://brainly.com/question/29973787
#SPJ4
4 ft
He wants to cover the entire model, including the base, with
gray paper.
How many square feet of paper will he need to cover the
model?

Answers
The square feet of paper that will cover the model is 56 ft squared.
How to find the surface area of a pyramid?The model above is a square base pyramid. Therefore, the square feet of papers he will need to cover the model is the surface area of the model.
Therefore,
surface area of the square base pyramid = A + 1 / 2 ps
where
A = surface area of the pyramidp = perimeter of the bases = height of the pyramidTherefore,
p = 4 × 4 = 16 ft
s = 5 ft
A = 4² = 16 ft²
Therefore,
surface area of the square base pyramid = 16 + 1 / 2 × 16 × 5
surface area of the square base pyramid = 16 + 40
surface area of the square base pyramid = 56 ft²
learn more on surface area here: brainly.com/question/31380742
#SPJ1
218-2
For what value of n does
= 2162
3r
1
36

Answers
Answer: n=1
Step-by-step explanation:

The value of n such that the given expression equals 216 is n = 1.
What are Expressions?Expressions are mathematical statements which consist of two or more terms and terms are connected to each other using mathematical operators like addition, multiplication, subtraction and so on.
Given expression is,
(216)ⁿ⁻² / (1/36)³ⁿ.
We have to find the value of n such that the expression equals to 216.
Let,
(216)ⁿ⁻² / (1/36)³ⁿ = 216
[(216)ⁿ (216)⁻²] / [(1/36)³ⁿ] = 216
[(216)ⁿ / (216)²] / [(1)³ⁿ / (36)³ⁿ] = 216
Taking the reciprocal and sign changes to multiplication,
[(216)ⁿ (36)³ⁿ] / (216)² = 216
[(216)ⁿ (36)³ⁿ] = (216)³
(216)ⁿ (36³)ⁿ = (216)³
(6³)ⁿ ((6²)³)ⁿ = (6³)³
(6³)ⁿ (6⁶)ⁿ = (6³)³
(6)³ⁿ (6)⁶ⁿ = (6)⁹
(6)³ⁿ⁺⁶ⁿ = (6)⁹
So, 3n + 6n = 9
9n = 9
n = 1
Hence the value of n is 1.
Learn more about Expressions here :
https://brainly.com/question/14083225
#SPJ7
A circle has a radius of 30 cm and a central angle that measures 312 degrees. Find the length of the arc defined by this central angle
Answers
The length of the arc defined by the central angle of 312 degrees is 26π cm.
The formula for the arc length of a circle is given by:
L = (θ/360) × 2πr
where L is the length of the arc, θ is the central angle in degrees, and r is the radius of the circle.
Substituting the given values, we get:
L = (312/360) × 2π(30)
L = (26/30) × π × 30
L = 26π cm
Therefore, the length of the arc defined by the central angle of 312 degrees is 26π cm.
To learn more on Circles click:
https://brainly.com/question/11833983
#SPJ1
Decide whether the rates are equivalent. Maria saves $50 in 4 months.
Ralph saves $60 in 5 months
Answers
Answer:
The rates are not equivalent since Maria saves $0.50 more per month than Ralph.
Step-by-step explanation:
We can determine if two rates are equivalent by comparing the rates at which they save per month.
Maria's savings per month:
Both 50 and 4 can be divided by 2, which gives us 25/2. As a regular number, this becomes 12.5/1 which means Maria saves $12.5 per month.
Ralph's savings per month:
Both 60 and 5 can be divided by 5, which gives us 12. Thus, Ralph saves $12 per month.
Thus, the rates are not equivalent as Maria saves $0.50 more per month than Ralph.
3(g-8)=18 solve for g
Answers
Step-by-step explanation:
1.: Distribute the 3: 3g-24=18
2.: Add 24 to both sides: 3g=42
3.: Divide by 3: 3g/3=42/3
4.: Answer: g=14
Answer:g=14
Step-by-step explanation:
3(g-8)=18
3g-24=18
3g-24+24=18+24
3g=42
\(\frac{3g}{3} =\frac{42}{3}\)
g = 14
Write the polynomial as a product of a difference and a sum: b^2-4/9
Answers
Answer:
\( \sf \huge \boxed{ \boxed{(b + \frac{2}{3} )(b - \frac{2}{3} )}}\)
Step-by-step explanation:
to understand thisyou need to know about:algebraPEMDAStips and formulas:\( {x}^{2} - {y}^{2} = (x + y)(x - y)\)let's solve:\( \sf \: rewrite : \\ ( {b})^{2} - ( \frac{2}{3} {)}^{2} \)\( \sf use \: the \: formula : \\ (b + \frac{2}{3} )(b - \frac{2}{3} )\)Answer:
Solution given:
\(b^{2} -\frac{4}{9}\)=\(b^{2} -\frac{2^2}{3^2}=b^2 -(\frac{2}{3})^2\)
it is in the form of x²-y²=(x+y)(x-y)
so
\(b^2 -(\frac{2}{3})^2=(b+\frac{2}{3})(b-\frac{2}{3})\) is A required polynomial.
Step-by-step explanation:
How many solutions does 6(4s+12)=8(3s-14) have?
Answers
9514 1404 393
Answer:
0 (no solutions)
Step-by-step explanation:
Eliminating parentheses, the equation becomes ...
24s +72 = 24s -112
184 = 0 . . . . . . . . . . . add 112 -24s to both sides
There is no value of the variable s that will make this true. The equation has no solutions.
Based on the information in the two-way table, what is the probability that a person
selected at random both bikes and runs?
Round your answer to the nearest tenth of a percent.

Answers
Answer:
Step-by-step explanation:

10. Prime numbers from 1 to 100 are running a restaurant - PRIME SPOT, near a tourist point. On a winter holiday, 1 and the composite numbers up to 100 enter the restaurant for dinner after their picnic at the same point. The dining hall has tables with seating capacity 15 for each. If they occupy tables without leaving any chair free, how many tables are required? If each prime number attender has to serve equal number of customers, how many customers should each one get to serve?
Answers
6 tables are required. Each prime number attender should serve 3 customers each.
The prime numbers between 1 and 100 are: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.
All the numbers other than prime numbers are composite numbers.
The composite numbers from 1 to 100 are: 1, 4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20, 21, 22, 24, 25, 26, 27, 28, 30, 32, 33, 34, 35, 36, 38, 39, 40, 42, 44, 45, 46, 48, 49, 50, 51, 52, 54, 55, 56, 57, 58, 60, 62, 63, 64, 65, 66, 68, 69, 70, 72, 74, 75, 76, 77, 78, 80, 81, 82, 84, 85, 86, 87, 88, 90, 91, 92, 93, 94, 95, 96, 98, 99, 100.
Now, as there are 25 primes and 75 composites in the group that visited the restaurant, we can calculate the number of tables required by dividing the number of people by the seating capacity of each table.
Each table has a seating capacity of 15, so the number of tables required will be: Number of tables = (Number of customers)/(Seating capacity of each table)Number of customers = 25 (the number of primes) + 75 (the number of composites) = 100Number of tables = 100/15 = 6 tables
Therefore, 6 tables are required.
Now, as each prime number attender has to serve an equal number of customers, we need to calculate how many customers each one should serve.
Each prime attender has to serve 75/25 = 3 customers each, as there are 75 composites and 25 primes.
Thus, each prime number attender should serve 3 customers each.
For more questions on prime number
https://brainly.com/question/145452
#SPJ8
A soda factory makes 9 flavours of soda. Each case of soda has 7 cans of each flavour. How many cans of soda does the factory put in 7 cases?
Answers
The factory will put 441 cans of soda in 7 cases.
If each case of soda contains 7 cans of each flavor, and the factory is putting 7 cases, we can calculate the total number of cans by multiplying the number of flavors, cans per flavor, and cases.
Given:
Number of flavors = 9
Cans per flavor = 7
Number of cases = 7
To calculate the total number of cans, we can multiply these values:
Total cans = Number of flavors × Cans per flavor × Number of cases
Total cans = 9 × 7 × 7
Total cans = 441
To arrive at this answer, we multiplied the number of flavors (9) by the number of cans per flavor (7) to get the number of cans per case (63). Then, we multiplied the cans per case (63) by the number of cases (7) to obtain the total number of cans (441).
Hence, the factory will put 441 cans of soda in 7 cases.
For more such questions on soda
https://brainly.com/question/29111121
#SPJ8
You are washing cars over the summer. It costs you $3 to wash each car and a one-time cost of $35 for some supplies to market your car wash. You plan to charge $10 per wash. Write an equation and solve to determine the amount of cars you need to break even.
Answers
The amount of cars you need to break even is 5.
To determine the number of cars you need to break even, we can set up an equation where the revenue equals the total cost.
Let's denote the number of cars as x.
Revenue = Number of cars × Price per wash
= x × $10
Total cost = Cost per car × Number of cars + One-time cost
= $3x + $35
To break even, the revenue should be equal to the total cost:
x × $10 = $3x + $35
Now, let's solve this equation to find the value of x:
10x = 3x + 35
Subtract 3x from both sides:
10x - 3x = 3x + 35 - 3x
7x = 35
Divide both sides by 7:
x = 35 / 7
x = 5
For similar questions on amount
https://brainly.com/question/24644930
#SPJ11
The measure of the supplement of an angle is in a 2:3 ratio to the measure of the angle. What is the measure of each angle?
Answers
Answer:
angle1 : angle 2:
72 108
Step-by-step explanation:
Supplementary angles add to 180
angle1 : angle 2:
2 3
Multiply each term by x
2x 3x
The total is 180
2x+3x= 180
5x= 180
Divide by 5
5x/5 =180/5
x =36
angle1 : angle 2:
2*36 3*36
72 108