the m 8 =m 1 true false will mark brainiest angles
Answers
Answer:
true
Step-by-step explanation:
trueeeeeeeeeeeeeeee
Related Questions
Describe in words the transformations of the graph of the parent function f(x) = x2 that
would result in the graph of
g(x) = 3(x - 5)^2 + 2.
Describe in words the transformations of the graph of the parent function f(x) = x2 that
would result in the graph of
g(x) = 1/3(x + 6)^2- 4.

Answers
2. The transformations are given as follows:
Vertical stretch by a factor of 3.Translation right 5 units.Translation up 2 units.3. The transformations are given as follows:
Vertical compression by a factor of 3.Translation left 6 units.Translation down 4 units.How to define the transformations?For item 2, the transformations in this problem are given as follows:
Vertical stretch by a factor of 3, due to the multiplication by 3.Translation right 5 units, as x -> x - 5.Translation up 2 units, as y -> y + 2.For item 3, the transformations are given as follows:
Vertical compression by a factor of 3, due to the multiplication by 1/3.Translation left 6 units, as x -> x + 6.Translation down 4 units, as y -> y - 4.More can be learned about translations at brainly.com/question/28174785
#SPJ1
the perimeter of a semicircle protractor is 14.8cm,find it's radius
Answers
The radius of the semicircle protractor is approximately 4.693 cm.
Given,Perimeter of a semicircle protractor = 14.8 cm.
To find:The radius of a semicircle protractor.Solution:We know that the perimeter of a semicircle protractor is the sum of the straight edge of a protractor and half of the circumference of the circle whose radius is the radius of the protractor.
Circumference of a circle = 2πrWhere, r is the radius of the circle.If the radius of the semicircle protractor is r, then Perimeter of a semicircle protractor = r + πr [∵ half of the circumference of a circle =\((1/2) × 2πr = πr]14.8 = r + πr14.8 = r(1 + π) r = 14.8 / (1 + π)r ≈ 4.693\) cm.
for more questions on semicircle protractor
https://brainly.com/question/30188078
#SPJ8
Solve the problem.
A landscaping team plans to build a rectangular garden that is between 240 yd² and 360 yd2 in area. For aesthetic reasons, they also want the
length to be 1.2 times the width. Determine the restrictions on the width so that the dimensions of the garden will meet the required area. Give exact
values and the approximated values to the nearest tenth of a yard.
Answers
The restrictions on the width is that it must be between 14.1 yards and 17.3 yards
How to determine the restrictions on the widthFrom the question, we have the following parameters that can be used in our computation:
Area = between 240 yd² and 360 yd²
Also, we have
Length = 1.2 times the width
This means that
l = 1.2w
The area is then calculated as
Area = lw
So, we have
Area = 1.2w²
This means that
240 < 1.2w² < 360
Divide through by 1.2
200 < w² < 300
Take the square roots
14.14 < w < 17.32
Approximate
14.1 < w < 17.3
Hence, the width must be between 14.1 yards and 17.3 yards
Read more about areas at
https://brainly.com/question/25292087
#SPJ1
Please help!!!!
Find the surface area of the composite figure below to
the nearest tenth.

Answers
Answer:
16801.2cm²
Step-by-step explanation:
Cone :
SA = πrl
SA = π · 28 · 45 90-45 = 45
SA = 3958.406744
Cylinder :
SA = 2 · π · r · ( r+h)
SA = 2 · π · 28 ( 28 +45 )
SA = 2 · π · 28 (73)
SA = 12842.83077
12842.83077 + 3958.406744 =
16801.2cm²
Show your work please help me it’s due tomorrow!!!!

Answers
Answer: Consider me the brainiest. The answer is... Decimal form =4.083
Exact form= 49/12
The mixed number form=4 1/12
How do we solve fractions step by step?
Conversion a mixed number 2 1/3 to a improper fraction: 2 1/3 = 2 1/3 = 2 3 + 1/3 = 6 + 1/3 = 7/3
To find a new numerator
A; Multiply the whole number 2 by the denominator 3. Whole number 2 equally 2 * 3/3 = 6/3
b) Add the answer from the previous step 6 to the numerator 1. The new numerator is 6 + 1 = 7
c) Write a previous answer (new numerator 7) over the denominator 3.
Two and one-third are seven-thirds.
Conversion a mixed number 1 3/4 to a improper fraction: 1 3/4 = 1 3/4 = 1 · 4 + 3/4 = 4 + 3/4 = 7/4
To find a new numerator:
a) Multiply the whole number 1 by the denominator 4. Whole number 1 equally 1 * 4/4 = 4/4
b) Add the answer from the previous step 4 to the numerator 3. The new numerator is 4 + 3 = 7
c) Write a previous answer (new numerator 7) over the denominator 4. One and three quarters are seven quarters.
Add: 7/3 + 7/4 = 7 · 4/3 · 4 + 7 · 3/4 · 3 = 28/12 + 21/12 = 28 + 21/12 = 49/12 It
is suitable to adjust both fractions to a common (equal, identical) denominator for adding, subtracting, and comparing fractions. The common denominator you can calculate as the least common multiple of both denominators - LCM(3, 4) = 12. It is enough to find the common denominator (not necessarily the lowest) by multiplying the denominators: 3 × 4 = 12. In the following intermediate step, it cannot further simplify the fraction result by cancelling. In other words - seven thirds plus seven quarters is forty-nine twelfths.
Derive an exact closed form bound in terms of n on the number of times function F called in each code segment. Use floor/ceilings as needed. Clearly explain your answer and show your work. (a) 1: function COUNT1 (n) 2: for i = 1 to 2n ^ 2 do for 1 to i do 3: k = 1 4: 5: while k <= n do 6: k = k + 8 7: F(i, j) (b) 1: function COUNT2(n) 23 for i = 1 ton do for j = 1 to i do 4: 6: for k = j ton do 5: 2966 F(i, j) F(j, k) 7: F(k, i) (c) The initial call is F(n ^ 2) 1: function F(i) 2: if i < 4 then 3: return 4: while i > 1 do 5: i leftarrow i / 4 6: F(i)
Answers
In order to calculate the exact closed form bound of the number of times function F is called in each code segment, we need to consider the number of times F is called in each line of code.
For COUNT1, line 3 calls F once, line 5 calls F n times, and line 7 calls F once more, for a total of n+2 calls.For COUNT2, line 4 calls F once, line 6 calls F n(n+1)/2 times, and line 7 calls F n(n-1)/2 times, for a total of n*(2n+1)/2 calls.For the initial call of F(n^2), the number of times F is called depends on the value of n. To calculate the exact number of calls for any value of n, we can use a recursive approach, starting from the top line of code and working our way down.
For example, for n=4, the initial call of F(16) will call F(4), which will then call F(1) four times, for a total of 5 calls. For n=8, the initial call of F(64) will call F(16), which will call F(4) four times and F(1) sixteen times, for a total of 20 calls.
Thus, for any value of n, the exact closed form bound of the number of times function F is called is given by the formula [1]:
F(n^2) + 4 * F(n/4) + 16 * F(n/16) + …Where the number of terms in the summation is equal to the number of times n is divided by 4 before it reaches 1.
For example, for n=16, the summation will have four terms (n=16, n=4, n=1), and for n=32, the summation will have five terms (n=32, n=8, n=2, n=1).
Learn more about programming:
https://brainly.com/question/28338824
#SPJ4
the population full-time equivalent number of students (ftes) at lake tahoe community college for 2005-2006 through 2010-2011 was given in an updated report. the data are reported here. year 2005-06 2006-07 2007-08 2008-09 2009-10 2010-11 total ftes 1,585 1,690 1,735 1,935 2,021 1,890 calculate the mean, median, standard deviation, the first quartile, the third quartile and the iqr. round to one decimal place. mean _____
median _____
Standard deviation ______
first quartile _____
third quartile ____
IQR _____
Answers
The correct answer for the required values is 1825.5, 1,812.5, 165.2, 1,690, 1,935, and 245 respectively.
To find the mean, median, standard deviation, first quartile, third quartile, and interquartile range (IQR) of the full-time equivalent number of students (FTES) at Lake Tahoe Community College for the given years, we can solve it using the following formulas for various aspects:
Mean: μ = (Σx)/n
Median: Middle value of the data set
Standard deviation: σ = sqrt[Σ(x-μ)²/n]
First quartile (Q1): Median of the lower half of the data set
Third quartile (Q3): Median of the upper half of the data set
IQR: Q3 - Q1
Year FTES
2005-06 1,585
2006-07 1,690
2007-08 1,735
2008-09 1,935
2009-10 2,021
2010-11 1,890
1. μ = (Σx)/n = (1,585 + 1,690 + 1,735 + 1,935 + 2,021 + 1,890)/6 = 1,825.2
Therefore the mean is 1,825.2
2. To find the median, arrange the data in ascending order:
1,585, 1,690, 1,735, 1,890, 1,935, 2,021
Since there are an even number of values, the median is the average of the two middle values, which are 1,812.5 and 1,812.5. So, the median is:
Median = (1,812.5 + 1,812.5)/2 = 1,812.5
3. σ = sqrt[Σ(x-μ)²/n]
= sqrt[((1,585-1,825.2)² + (1,690-1,825.2)² + (1,735-1,825.2)² + (1,935-1,825.2)² + (2,021-1,825.2)² + (1,890-1,825.2)²)/6]
= 165.2
4. To find the first quartile (Q1), find the median of the lower half of the data set, which consists of the values 1,585, 1,690, and 1,735.
Since there are an odd number of values, the median is the middle value, which is 1,690. Therefore, Q1 = 1,690.
5. To find the third quartile (Q3), we need to find the median of the upper half of the data set, which consists of the values 1,890, 1,935, and 2,021.
Since there are an odd number of values, the median is the middle value, which is 1,935. Therefore, Q3 = 1,935
6. IQR = Q3 - Q1
Substituting the calculated values in the equation
IQR = 1935 - 1690
= 245
Read more about Distribution from:
https://brainly.com/question/28941367
#SPJ4
2/3 minus 4/9 what is the fraction
Answers
Answer:
2/9. Decimal form is 0.222....
Step-by-step explanation:
Malcolm has $50 gift card to a local car wash and order is the ultimate car wash each visit is $8.95
Answers
The amount cheaper is the car washes Malcolm orders than the car washes Martha's order is $13.
The correct answer choice is option B.
How much cheaper is the car washes Malcolm orders than the car washes Martha's order?Malcolm's gift card = $50.
Cost Malcolm's car wash per visit = $7
Martha's gift card = $180
Cost Martha's car wash per visit = Difference between gift card balance of first and second visit
= $180 - $160
= $20
How cheap is the car washes Malcolm orders than the car washes Martha's order = $20 - $7
= $13
Therefore, Malcolm's car wash is cheaper than Martha's car wash by $13
The complete question is attached in the diagram.
Read more on graphs:
https://brainly.com/question/19040584
#SPJ1

A motorist completes a journey in 45 minutes covering a distance of 75km at what speed did he travel
Answers
Answer:
100KM PER HOUR
Step-by-step explanation:
Have a nice day
Answer:
He traveled at a speed of 1.6 miles per minute
Step-by-step explanation:
75/45=1.6
Please help I will give brainleist

Answers
Explained:-
-4.8 x -5 = 24
5. A submarine is searching for underwater features. It is accompanied by a small
At one time the aircraft is 200 m above the surface, the submarine is 55 m below
aircraft and an underwater robotic vehicle,
the surface, and the underwater robotic vehicle is 227 m below the surface
What is the difference in height between the submarine and the aircraft?
1.
2. What is the distance between the underwater robotic vehicle and the
submarine?
Answers
Answer: The required difference is 255 m .
Step-by-step explanation: Since we have given that
Distance at which the aircraft is above the surface = 200 m
Distance at which the submarine is below the surface = 55 m
Above the surface is represented as positive number i.e. (+) 200 m
Below the surface is represented as negative number i.e. (-) 55 m
Hence, the required difference is 255 m .
(sorry if im wrong)
:(
Find the difference
(2/7x-3) - (5/2+1/5y)
Answers
Step-by-step explanation:
Distribute the negative sign:
\(\frac{2}{7} x-3 -5/2-\frac{1}{5}y\)
From the given, we can only find the difference with the integers.
Subtract the integers:
\(\frac{2}{7} x-\frac{1}{5}y-0.5\)
You have two pitchers and each can contain 600 ml of liquid. One pitcher is one-third full and contains vinegar. The other pitcher is two-fifths full of vinegar. Oil is added to fill each pitcher completely. The contents of each pitcher are poured into one large container. What fraction of the mixture in the large container is vinegar?
Answers
Answer:
answera c
Step-by-step explanation:
Mid-West Publishing Company publishes college textbooks. The company operates an 800 telephone number whereby potential adopters can ask questions about forthcoming texts, request examination copies of texts, and place orders. Currently, two extension lines are used, with two representatives handling the telephone inquiries. Calls occurring when both extension lines are being used receive a busy signal; no waiting is allowed. Each representative can accommodate an average of 15 calls per hour. The arrival rate is 30 calls per hour.
How many extension lines should be used if the company wants to handle 90% of the calls immediately?
fill in the blank 1
lines should be used
What is the average number of extension lines that will be busy if your recommendation in part (a) is used? Round your answer to four decimal places.
L = fill in the blank 2
What percentage of calls receive a busy signal for the current telephone system with two extension lines? Round your answer to two decimal places.
fill in the blank 3
%
Answers
The various answers to the question are:
To answer 90% of calls instantly, the organization needs four extension lines.The average number of extension lines that will be busy is FourFor the existing phone system with two extension lines, 34.25 % of calls get a busy signal.How many extension lines should be used if the company wants to handle 90% of the calls immediately?a)
A number of extension lines needed to accommodate $90 in calls immediately:
Use the calculation for busy k servers.
\($$P_{j}=\frac{\frac{\left(\frac{\lambda}{\mu}\right)^{j}}{j !}}{\sum_{i=0}^{k} \frac{\left(\frac{\lambda}{\mu}\right)^{t}}{i !}}$$\)
The probability that 2 servers are busy:
The likelihood that 2 servers will be busy may be calculated using the formula below.
\(P_{2}=\frac{\frac{\left(\frac{20}{12}\right)^{2}}{2 !}}{\sum_{i=0}^{2} \frac{\left(\frac{20}{12}\right)^{t}}{i !}}$$\approx 0.3425$\)
Hence, two lines are insufficient.
The probability that 3 servers are busy:
Assuming 3 lines, the likelihood that 3 servers are busy may be calculated using the formula below.
\(P_{j}=\frac{\frac{\left(\frac{\lambda}{\mu}\right)^{j}}{j !}}{\sum_{i=0}^{2} \frac{\left(\frac{\lambda}{\mu}\right)^{i}}{i !}}$ \\\\$P_{3}=\frac{\frac{\left(\frac{20}{12}\right)^{3}}{3 !}}{\sum_{i=0}^{3} \frac{\left(\frac{20}{12}\right)^{1}}{i !}}$$\approx 0.1598$\)
Thus, three lines are insufficient.
The probability that 4 servers are busy:
Assuming 4 lines, the likelihood that 4 of 4 servers are busy may be calculated using the formula below.
\(P_{j}=\frac{\frac{\left(\frac{\lambda}{\mu}\right)^{j}}{j !}}{\sum_{i=0}^{k} \frac{\left(\frac{\lambda}{\mu}\right)^{t}}{i !}}$ \\\\$P_{4}=\frac{\frac{\left(\frac{20}{12}\right)^{4}}{4 !}}{\sum_{i=0}^{4} \frac{\left(\frac{20}{12}\right)^{7}}{i !}}$\)
Generally, the equation for is mathematically given as
To answer 90% of calls instantly, the organization needs four extension lines.
b)
The probability that a call will receive a busy signal if four extensions lines are used is,
\(P_{4}=\frac{\left(\frac{20}{12}\right)^{4}}{\sum_{i=0}^{4} \frac{\left(\frac{20}{12}\right)^{1}}{i !}} $\approx 0.0624$\)
Therefore, the average number of extension lines that will be busy is Four
c)
In conclusion, the Percentage of busy calls for a phone system with two extensions:
The likelihood that 2 servers will be busy may be calculated using the formula below.
\(P_{j}=\frac{\left(\frac{\lambda}{\mu}\right)^{j}}{j !}$$\\\\$P_{2}=\frac{\left(\frac{20}{12}\right)^{2}}{\sum_{i=0}^{2 !} \frac{\left(\frac{20}{12}\right)^{t}}{i !}}$$\approx 0.3425$\)
For the existing phone system with two extension lines, 34.25 % of calls get a busy signal.
Read more about signal
https://brainly.com/question/14699772
#SPJ1
39÷702=
What is the remainder
Answers
The answer is 18 with a remainder of 0.
Answer:
= 18 R 0
= 18
Step-by-step explanation:
YAY SO MANY POINTS
Evaluate the expression (x+3)(3x^2-4x-5) ?
Answers
3x³ + 5x² - 17x - 15
Step-by-step explanation:Here are the steps for solving your problem:1. Distribute
(x + 3)(3x² - 4x - 5)
x(3x² - 4x - 5) + 3(3x² - 4x - 5)
2. Distribute
x(3x² - 4x - 5) + 3 (3x² - 4x - 5)
3x³ - 4x² - 5x + 3 (3x² -4x - 5)
3. Distribute
3x³ - 4x² - 5x + 3 (3x² -4x - 5)
3x³ - 4x² -5x + 9x² - 12x - 15
4. Combine like terms
3x³ - 4x² -5x + 9x² - 12x - 15
3x³ + 5x² - 5x - 12x - 15
5. Combine like terms
3x³ + 5x² - 5x - 12x - 15
3x³ + 5x² - 17x - 15
Solution:
3x³ + 5x² - 17x - 15
I hope my answer helped you! If you need more information or help, comment down below and I will be sure to respond if I am online. Have a wonderful rest of your day!
How to make 3 dimensional object become 4 dimensional object
Answers
Using Hinton's method;
Draw two ordinary 3D cubes in 2D space, one encompassing the other, separated by an "unseen" distanceThen draw lines between their equivalent vertices.The eight lines connecting the vertices of the two cubes in this case represent a single direction in the "unseen" fourth dimension.What is a 4 dimensional shape?A four-dimensional shape (4D) is a mathematical extension of a three-dimensional or 3D space.
Three-dimensional space is the simplest possible abstraction of the observation that one only needs three numbers, called dimensions, to describe the sizes or locations of objects.
Using Hinton's method;
Draw two ordinary 3D cubes in 2D space, one encompassing the other, separated by an "unseen" distanceThen draw lines between their equivalent vertices.The eight lines connecting the vertices of the two cubes in this case represent a single direction in the "unseen" fourth dimension.Learn more about dimensional shapes here:
https://brainly.com/question/12280037
#SPJ1
There are two bags of counters, bag X and bag Y.
There are 20 counters in bag X.
11 of the counters are blue and the rest are red.
There are 16 counters in bag Y.
9 of the counters are blue and the rest are red.
Arkady takes at random a counter from bag X and takes at random a counter from bag Y.
(a) Complete the probability tree diagram.
Answers
The probability tree diagram of various possibilities is given:
Bag X = 20 counters
Blue counters: 11/20Red counters: 9/20Bag Y = 16counters
Blue counters: 9/16Red counters: 7/16(Refer to the image attached below)
What is a probability tree diagram?You can quickly and easily calculate the probabilities of dependent and independent events using a probability tree diagram. Multiply the probabilities of the connected branches to determine the likelihood of various outcomes. Add the probabilities together to determine the likelihood of multiple outcomes.So, tree diagram:
Bag X = 20 counters
Blue counters: 11/20Red counters: 9/20Bag Y = 16counters
Blue counters: 9/16Red counters: 7/16(Refer to the probability tree diagram given below)
Therefore, the probability tree diagram of various possibilities is given:
Bag X = 20 counters
Blue counters: 11/20Red counters: 9/20Bag Y = 16counters
Blue counters: 9/16Red counters: 7/16Know more about a probability tree diagram here:
https://brainly.com/question/27891044
#SPJ9

Write the following equation in LOGARITHMIC terms do not simplify your answer
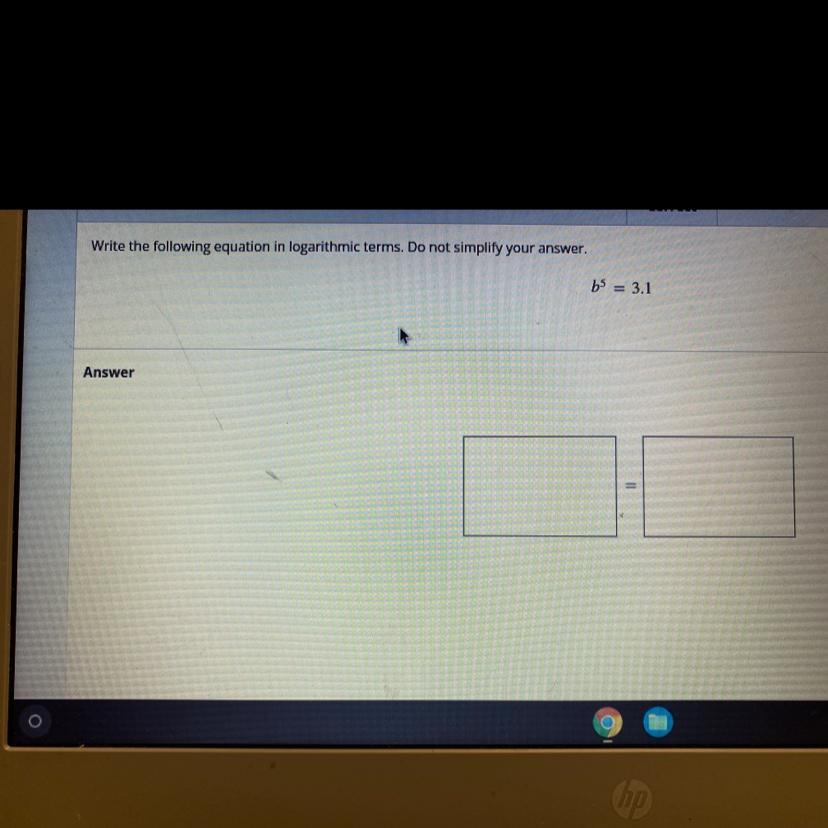
Answers
Solution
- The solution steps are given below:
\(\begin{gathered} b^5=3.1 \\ \text{ Take the logarithm of both sides} \\ \log b^5=\log3.1 \end{gathered}\)Final Answer
The answer is
\(\log b^5=\log3.1\)please help me thank you in advance!

Answers
Answer:
x = √6/2
Step-by-step explanation:
Side a = 1.73205 --> √3
Side b = 1.22474 --> √6/2
Side c = 1.22474 --> √6/2
I can prove that 2=1, where is the error?
X = 1
X+X = 1+X
2x = 1+X
2x = X+1
2X-2 = X+1-2
2x-2 = X-1
2 (x-1)/(x-1) = X-1/X-1
2 times 1 = 1 1-1 / 1-1
2 = 1
I subtracted -2
because thats the # I chose to subtract with.
Answers
There is no error in steps while proving 2=1
We have to prove 2=1
Let x=1
Add X on both sides
X+X = 1+X
2x = 1+X
2x = X+1
Subtract 2 from both sides
2X-2 = X+1-2
2x-2 = X-1
2(X-1)=x-1
Divide both sides by x-1
2 (x-1)/(x-1) = X-1/X-1
We get 2=1
Hence, while proving 2=1 there is no error in steps
To learn more on Number system click:
https://brainly.com/question/22046046
#SPJ1
For each of the 4 walls of the house, John will need 9 large
planks of wood. If each plank of wood needs 8 pieces of nails
to be secured, how many nails does John need for each wall
of the house?
Answers
is (5, 20) a solution of the equation y = 4x explain.
A. Yes, 5 = 5. B. No, 5 ≠ 4.
C. Yes, 20 = 20 D. No, 5 ≠ 80.
Answers
Answer:
C. yes, 20 = 20
Step-by-step explanation:
the equation is y=4x and from the coordinates (5,20) we can see that x =5 and y = 20
substituting in the original equation we must check if
20 = 4(5)
20 = 20
left = right
yes it is a solution
The cost of renting a boat is a $3 deposit and $5.25 for each hour. Find the total cost to rent the boat for 6 hours.
Answers
Answer:34.5
Step-by-step explanation:
5.25 x 6 + 3.
You multiple 5.25 however many hours it says and then you have the 3$ for the fee.
I don't understand this

Answers
Answer:
tytytytyt
Step-by-step explanation:
Answer:
i don't either
Step-by-step explanation:
which function will have the greatest value at
\(x = 16 \)
\( y = {10}^{16} \)
\(y = {x}^{2} - 17x + 182\)
\(y = {1.17}^{x} \)
Answers
The function that would have the greatest value at x = 16 include the following: B. y = x² - 17x + 182.
How to determine the corresponding output value for the given function?In Mathematics and Geometry, a function is a mathematical equation which defines and represents the relationship that exists between two or more variables such as an ordered pair in tables or relations.
In this exercise, we would determine the corresponding output value for this function of y based on the x-value (x = 16) in simplified form as follows;
\(y = 10^{16}\)
y = 10,000,000,000,000,000.
y = x² - 17x + 182
y = 16² - 17(16) + 182
y = 256 - 272 + 182
y = 166.
\(y = 1.17^x\\\\y = 1.17^{16}\)
y = 12.330304108137675851908392069373.
Read more on function here: brainly.com/question/10687170
#SPJ1
Sal is tiling his entryway. The floor plan is drawn on a unit grid. Each unit length represents 1 foot. Tile costs $1.95 per square foot. How much will Sal pay to tile his entryway? Round your answer to the nearest cent.
Answers
Sal will pay $84.83 to tile his entryway
Solution:
The image of the question is attached below.
Given each unit length = 1 foot
Tile cost per square feet = $1.95
To find the area, split the floor shape into two shape.
One is trapezoid and the other is parallelogram.
Let's count the grid given.
The parallels sides of trapezoid are 7 ft and 4 ft, height is 7 ft.
The base and height of the parallelogram are 4 ft and 4 ft respectively.
Area of the trapezoid \(=\frac{1}{2}*(\)\(sum\) \(of\) \(parallel\) \(sides)*height\)
\(=\frac{1}{2}*(7+4)*5\)
\(=\frac{1}{2} *11*5\)
Area of the trapezoid = 27.5 sq.ft
Area of the parallelogram = base × height
= 4 ft × 4 ft
Area of the parallelogram = 16 sq. ft
Total area = 27.5 sq. ft + 16 sq. ft
= 43.5 sq. ft
Cost of area per sq. ft = $1.95
Cost of area 43.5 sq. ft = 43.5 × 1.95
= $84.825
= $84.83
Hence Sal will pay $84.83 to tile his entryway.

please help i will give brainliest to one who answers
You and a friend work at a job, The job paid $20 and you and the friend have to split it evenly, except your friend must make $1.00 more. How much will each of you get?5 sentences, please help i will give brainliest to one who answers
Answers
Answer:
20 ÷ 2 is 10. and since your friend you smoke $1 more they would get 11 dollars and you would get $9
What is f_x (0,0) if
Hint: use Limit

Answers
\(f_x(0,0)\) is the partial derivative of \(f\) with respect to \(x\) evaluated at the origin. By definition of derivative, this is the limit
\(\displaystyle f_x(0,0) = \lim_{x\to0} \frac{f(x,0) - f(0,0)}{x-0} = \lim_{x\to0} \frac{f(x,0)}x\)
We have by definition of \(f\),
\(f(x,0) = \dfrac{x^5}{x^4+0^4} = \dfrac{x^5}{x^4}\)
so
\(\displaystyle f_x(0,0) = \lim_{x\to0} \frac{\frac{x^5}{x^4}}x = \lim_{x\to0} \frac{x^5}{x^5} = \lim_{x\to0} 1 = \boxed{1}\)