Ten granola bars and twelve bottles of water cost $23. Five granola bars and four bottles of water cost $10. How much do one granola bar and one bottle of water cost?$ Part BFor a fundraiser, a group plans to sell granola bars and bottles of water at the same prices as described in Part A. The group wants the income from the fundraiser to be at least $150.Choose the inequality to show the number of granola bars x and the number of bottles of water y that must be sold.A.1.4x + 0.75y > 150B.1.4x + 0.75y ≥ 150C.1.4x + 0.75y < 150D.1.4x + 0.75y ≤ 150

Answers
We want to find the cost of a granola bar and a water bottle. Let x be the cost of the granola bar and y the cost of the water bottle. We are told that 10 granola bars and 12 bottles of water cost 23. As x is the cost of one granola bar, 10 x is the cost of 10 granola bars. So if we add the cost of the granola bars and the cost of the water bottles we get the total cost. This leads to the equation
\(10x+12y=23\)Now, if we calculate the equation for the other situation (5 granola bars and 4 bottles are 10) we get the equatio
\(5x+4y=10\)Let us multiply the second equation by 2. So we get
\(10x+8y=20\)Now, we can subtract the second equation from the first one, so we ge t
\(10x+12y\text{ - (10x+8y)=4y=23-20=3}\)so if we divide both sides by 4, we get
\(y=\frac{3}{4}=0.75\)so the cost of one water bottle is 0.75
Now we replace this value in the first equation, so we get
\(10x+12\cdot\frac{3}{4}=23=10x+9=23\)If we subtract 9 from both sides we get
\(10x=23\text{ -9=14}\)So if we divide both sides by 10, we get
\(x=\frac{14}{10}=1.4\)so the cost of a granola bar is 1.4
Now, we know that a granola bar costs 1.4 and a water bottle costs 0.75. If we sell x granola bars, the total income for x granola bars would be 1.4x. In the same manner, the total income for y water bottles would be 0.75y. If we add this two quantities we get
\(1.4x+0.75y\)as this quantity should be at least 150, this means that it is greater than or equal to 150, so we get the inequality
\(1.4x+0.75y\ge150\)which is option B
Related Questions
n automatic machine in a manufacturing process is operating properly if the lengths of an important subcomponent are normally distributed with a mean of 116 cm and a standard deviation of 5.4 cm. A. Find the probability that one selected subcomponent is longer than 118 cm. Probability
Answers
Answer:
0.3557 = 35.57% probability that one selected subcomponent is longer than 118 cm.
Step-by-step explanation:
Normal Probability Distribution:
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Normally distributed with a mean of 116 cm and a standard deviation of 5.4 cm.
This means that \(\mu = 116, \sigma = 5.4\)
Find the probability that one selected subcomponent is longer than 118 cm.
This is 1 subtracted by the pvalue of Z when X = 118. So
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{118 - 116}{5.4}\)
\(Z = 0.37\)
\(Z = 0.37\) has a pvalue of 0.6443
1 - 0.6443 = 0.3557
0.3557 = 35.57% probability that one selected subcomponent is longer than 118 cm.
When 1370 subjects were asked, "On how many days in the past 7 days have you felt lonely?" the mean was 1.5 and the standard deviation was 2.16. The margin of error for a 95% confidence interval for the population mean is 0.12. Construct that confidence interval, and interpret it. The confidence interval goes from nothing to nothing. (Round to two decimal places as needed.)
Answers
Answer:
Confidence Interval = (1.41, 1.59)
Step-by-step explanation:
To construct a confidence interval for the population mean, we can use the following formula:
Confidence Interval = sample mean ± (margin of error * standard error)
where the standard error is equal to the standard deviation divided by the square root of the sample size.
Given the information provided, we can calculate the standard error as:
standard error = 2.16 / sqrt(1370) = 0.058
Then, we can calculate the margin of error as 0.12.
Substituting these values into the formula, we get:
Confidence Interval = 1.5 ± (0.12 * 0.058)
Confidence Interval = (1.41, 1.59)
Therefore, we can interpret the 95% confidence interval as follows: we are 95% confident that the true population mean of the number of days that people feel lonely in the past 7 days is between 1.41 and 1.59. This means that if we were to take many samples of 1370 subjects and compute the confidence intervals for each sample using the same method, we would expect about 95% of the intervals to contain the true population mean.
Which is the equation of the line with slope 0 passing through the point (-3, -1)?
Answers
An analyst notices that a CEO has consistently achieved 5% growth in profits from one yearto the next. The CEO's company currently has annual profits of $955,000. If the trendcontinues, what will the annual profits be in 9 years?If necessary, round your answer to the nearest cent.
Answers
EXPLANATION
This is a Growth Function Model, thus we can use the following expression:
\(f(t)=a(1+r)^t\)Where r represents the rate, t the time and a the initial amount.
Substituting terms:
\(f(t)=955,000(1+0.05)^9\)Adding numbers:
\(f(t)=955,000\cdot1.05^9\)Computing the power:
\(f(t)=955,000\cdot1.5513\)Multiplying numbers:
\(f(t)=1481518.45\)In conclusion, the annual profit in 9 years will be of $ 1,481,518.45
PLS HELP Use the net to find the lateral area of the prism. 8 15 17 9
answer choices 473 440 275 352
Answers
The lateral area of the prism is the area of the three rectangles in the middle of the net. (The two triangles are the bases of the prism, which don't count toward lateral area.) The total length of the three rectangular prism faces is (8 +15 +17) cm = 40 cm. The width of those rectangles is 9 cm. The lateral area will be the product of this length and width:
... lateral area = (40 cm)(9 cm) = 360 cm²
Help-_--_-__- - _____________________--

Answers
Answer:
Opinion C
Step-by-step explanation:
hope that will help you
If x= -5
-3(-5) +15 =30
15+15=30
So it is true
can someone help please, it wont give me the last mark

Answers
Answer:
The explanation is:
All interior angles in an equilateral triangle are congruent, making them all 60° by the sum of angles in a triangle. Because alternate interior angles of parallel lines are congruent, x = 60°.
find the product of ((-25ac) and (-4t))
Answers
\(\large\huge\green{\sf{Answer:-}}\)
\( = (-25ac) \times (-4t)) \\ = 100 \times a \times c \times t. \\ =100a \times c \times t \)
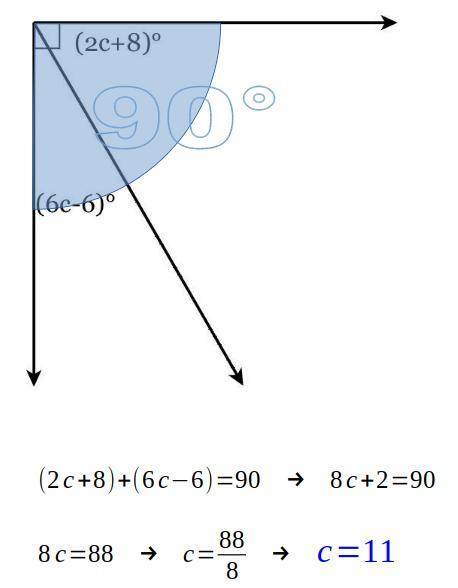
Whats (c) ????????????? ????

Answers
Answer:
11
Step-by-step explanation:
yeah-ya............ right?
Check the picture below.
What is 4 1/4 take away 2 2/4
Answers
Answer:
1 3/4
Step-by-step explanation:
4 - 2 = 2
2 1/4 - 2/4 is the new equation
2 1/4 - 1/4 = 2
2 - 1/4 = 1 3/4
1 3/4
:D
FCPS Bookmarks
Launch realize. 8-2: Find Volume of Cylinders (LMS graded)
8.2.PS-13
The cylinder shown has a volume of 824 cubic inches.
a. What is the radius of the cylinder? Use 3.14 for .
b. If the height of the cylinder is changed, but the volume
stays the same, then how will the radius change? Explain.
a. The radius of the cylinder is about 8.2 in³.
(Type an integer or decimal rounded to the nearest tenth as needed.)
10.7 in.
Question Help
◇
Answers
To find the radius of the cylinder, we can use the formula for the volume of a cylinder:
V = πr²h
Given that the volume is 824 cubic inches, we can rearrange the formula to solve for the radius:
824 = πr²h
Since the height is not given, we cannot find the exact radius. However, we can still determine the relationship between the height and radius if the volume remains the same.
b. If the height of the cylinder is changed, but the volume stays the same, the radius will change inversely proportional to the height. This means that as the height increases, the radius will decrease, and vice versa. This relationship ensures that the volume remains constant.
Therefore, we cannot determine the exact radius without knowing the height, but we can conclude that the radius and height have an inverse relationship.
Use the following information to answer the next question. In the process, what is the total distance that Jeremy covers?Use the following information to answer the next question.
In the process, what is the total distance that Jeremy covers?
Answers
The total distance covered by Jeremy is 1320 m
What is a line segment ?
A line segment in geometry is bounded by two separate points on a line. Another way to describe a line segment is as a piece of the line that joins two points. A line segment has two fixed or distinct endpoints while a line has no endpoints and can stretch in both directions indefinitely.
For sapling 1 = No distance is covered.
For sapling 2, distance covered = 10 m
Return distance=10 m
For sapling 3, distance covered =20 m
Return distance=20 m and so on
Thus the distances covered for saplings can be represented as : 0,2(10), 2(20),...i.e. 0,20, 40,...
Therefore total distance covered by Jeremy for planting 12 saplings and coming back to original position = S 12
Total distance covered = 122 [2(0) + (12 − 1) 20 ]
Total distance covered = 6 (11)(20) = 1320 m
To learn more about the line segment from the given link
https://brainly.com/question/2437195
#SPJ1
Complete question:
There are 12 saplings to be planted in a straight line at an interval of 10 m between
two consecutive saplings. Jeremy can carry only one sapling at a time and he has to
come back to the original point to take the next sapling. He plants 12 saplings in this
manner, planting the first sapling at the original point. After planting all the saplings,
he comes back at the original point,
In the process, what is the total distance that Jeremy covers?
What is the value of two in 0.259?
Answers
The value of two in 0.259 is 0.2 because it represents two hundredths or 2/100 of the total value of the number.
In the decimal number 0.259, the value of two depends on its position or place within the number. Each digit in a decimal number represents a different place value, starting from the left with the tenths place, hundredths place, thousandths place, and so on.
Since two is in the hundredths place in the number 0.259, its value is 0.02. This means that the digit two contributes two hundredths to the total value of the number. The digit in the tenths place (5 in this case) represents five tenths, and the digit in the thousandths place (9 in this case) represents nine thousandths.
To calculate the total value of 0.259, we add up the contributions from each place value: 0.2 (for the tenths place), 0.05 (for the hundredths place), and 0.009 (for the thousandths place). Adding these values together gives us a total value of 0.259.
In summary, the value of two in 0.259 is 0.2 because it represents two hundredths or 2/100 of the total value of the number.
For more such questions on value , Visit:
https://brainly.com/question/843074
#SPJ11
Solve for x
1(x+6) - 3 =4(x + 2)
Answers
Answer:
I think the answer to x is 1
Step-by-step explanation:
1 ( x + 6 ) - 3 = 4 ( x + 2 )
1x + 6 - 3 = 4x + 12
+3 +3
1x + 6 = 4x + 15
- 6 - 6
1x = 4x + 9
-4x -4x
-3 = 9
Then divide the -3 with -3 then put an equal sign then put 9 divided by -3.
X = 1
I hope this helps.
Answer:
the answer is
\(x = - \frac{5}{3} \)



What is the solution to |2x-8|<2?

Answers
Answer:
A) 3<x<5
Step-by-step explanation:
Given: |2x-8|<2
Make two inequalities:
First inequality:
2x-8<2
2x<10
x<5
Second inequality:
2x-8>-2
2x>6
x>3
Therefore, your solution is 3<x<5 since the two inequalities pass each other, making an "and" inequality.
What is the distance from Pto Q?
5432
V
AN
-21
de
0-14.4
OA. 25 units
OB. 1 unit
OC. 5 units
OD. 7 units
2345
P(3-1)

Answers
The distance from point P to point Q is equal to: C. 5 units.
How to calculate the distance between the two points?Mathematically, the distance between two (2) points that are on a coordinate plane can be calculated by using this formula:
Distance = √[(x₂ - x₁)² + (y₂ - y₁)²]
Where:
x and y represents the data points (coordinates) on a cartesian coordinate.
Substituting the given parameters into the distance formula, we have the following;
Distance = √[(x₂ - x₁)² + (y₂ - y₁)²]
Distance = √[(-1 - 3)² + (-4 - (-1))²]
Distance = √[(-4)² + (-4 + 1)²]
Distance = √[(-4)² + (-3)²]
Distance = √(16 + 9)
Distance = √25
Distance = 5 units.
Read more on distance here: brainly.com/question/12470464
#SPJ1
Which of these best explains the next step to simplify this expression?

Answers
Answer:
Make the -4 exponent in the denominator positive.
i need help with "A group of friends were working on a student film. They spent all their budget on equipment and props. They spent $712 on equipment, and 11% of the total budget on props. What was the total budget for their student film?"
Answers
The total budget for their student film $800
How to finding the total money your group spent on props?11% of total budget = (11/100) * total budget
= 0.11 * total budget
Let P be the amount the group spent on props.
Now we can write the equation:
P = 0.11 * total budget
We also know that this group spent $712 on equipment. Let E be the amount they spent on equipment.
So it looks like this:
E=$712
Your total budget is the total cost of your equipment and props. This can be written as:
Total Budget = E + P
Plugging in the E and P values, we get:
Total Budget = $712 + 0.11 * Total Budget
Simplifying this equation, we get:
0.89 * total budget = $712
Dividing both sides by 0.89 gives:
Total budget = $800
So her student film had a total budget of $800.
To know more about dividing visit:-
https://brainly.com/question/15381501
#SPJ1
Which statement about the location of √7 on the number line is true?
A= It is located at the number 7 on the number line.
B= It is located at the number 3.5 on the number line.
C= It is located between the numbers 2 and 3 on the number line.
D=It is located between the numbers 4 and 9 on the number line
Answers
Explanation
The square root of a number is “what number times itself”
2 x 2 = 4
3 x 3 = 9
7 is between 4 and 9 so C is the correct answer
A manufacturer must test that his bolts are 4.00 cm long when they come off the assembly line. He must recalibrate his machines if the bolts are too long or too short. After sampling 121 randomly selected bolts off the assembly line, he calculates the sample mean to be 4.21 cm. He knows that the population standard deviation is 0.83 cm. Assuming a level of significance of 0.02, is there sufficient evidence to show that the manufacturer needs to recalibrate the machines? Step 2 of 3: Compute the value of the test statistic. Round your answer to two decimal places.
Answers
The sample mean of 4.21 cm is significantly different from the specified target mean of 4.00 cm.
Step 1: State the hypotheses.
- Null Hypothesis (H₀): The mean length of the bolts is 4.00 cm (μ = 4.00).
- Alternative Hypothesis (H₁): The mean length of the bolts is not equal to 4.00 cm (μ ≠ 4.00).
Step 2: Compute the value of the test statistic.
To compute the test statistic, we will use the z-test since the population standard deviation (σ) is known, and the sample size (n) is large (n = 121).
The formula for the z-test statistic is:
z = (X- μ) / (σ / √n)
Where:
X is the sample mean (4.21 cm),
μ is the population mean (4.00 cm),
σ is the population standard deviation (0.83 cm), and
n is the sample size (121).
Plugging in the values, we get:
z = (4.21 - 4.00) / (0.83 / √121)
z = 0.21 / (0.83 / 11)
z = 0.21 / 0.0753
z ≈ 2.79 (rounded to two decimal places)
Step 3: Determine the critical value and make a decision.
With a level of significance of 0.02, we perform a two-tailed test. Since we want to determine if the mean length of the bolts is different from 4.00 cm, we will reject the null hypothesis if the test statistic falls in either tail beyond the critical values.
For a significance level of 0.02, the critical value is approximately ±2.58 (obtained from the z-table).
Since the calculated test statistic (2.79) is greater than the critical value (2.58), we reject the null hypothesis.
Conclusion:
Based on the computed test statistic, there is sufficient evidence to show that the manufacturer needs to recalibrate the machines. The sample mean of 4.21 cm is significantly different from the specified target mean of 4.00 cm, indicating that the machine's output is not meeting the desired length. The manufacturer should take action to recalibrate the machines to ensure the bolts meet the required length of 4.00 cm.
for more such question on mean visit
https://brainly.com/question/1136789
#SPJ8
i need help plss dont lie

Answers
Answer:
A x B = 5/6 x 3/4 = 15/24
Answer = 15/24
Step-by-step explanation:
Answer:
We are asked to find the area of the rectangle that has side lengths of 3/4 yard and 5/6 yard.
We know that area of rectangle is width times length.
To find the area of the given rectangle we will multiply both side length as:
Area of regatangle = width x Lenghth
Area of regtangle = 3/4 x 5/6 yard to the 2 power
Area of regtangle = 1/4 x 5/2 yard to the 2 power
Area of regtangle = 1 x 5/ 4x2 yard to the 2 power
Area of regtangle = 5/8 yard to the 2 power
Therefore, the area of the given rectangle would be 5/8 square yards.
Mario constructs a scale model of a building with a rectangular base. His model is 4.2 inches in length and 2 inches in width. The scale of the model is 1 inch = 15 feet.
What is the actual area, in square feet, of the base of the building?
Answers
The actual base of the building is 1890 square feet.
What is a rectangle?A rectangle is a two-dimensional figure with length and width.
The area of a rectangle is Length x width.
We have,
1 inch = 15 feet
Rectangular base:
Length = 4.2 in
Width = 2 in
So,
4.2 in = 4.2 x 15 feet = 63 feet
2 in = 2 x 15 = 30 feet
Now,
The actual base of the building.
= 63 x 30
= 1890 square feet
Thus,
1890 square feet.
Learn more about rectangles here:
https://brainly.com/question/15019502
#SPJ9
Which expressions are equivalent to 64^1Check all that apply

Answers
The right answers are:
4^38^22^6Hope it helps.
please see the attached picture for full solution
Good luck on your assignment

Which expression represents the phrase below?
3 fewer than a number, p
A. 3-P B. p=3
3p
C. 3:p
D. p-3
Answers
Answer:
d) p - 3
3 fewer than menas you need to subtract 3
Step-by-step explanation:
The expression represents the phrase “3 fewer than a number, p” is (p-3).
Given
Expression; the phrase: “3 fewer than a number, p”
What is the phrase?
A small group of words standing together as a conceptual unit, typically forming a component of a clause:
The given number is p which is smaller than a number which is 3.
Expression; the phrase: “3 fewer than a number, p”
Then,
The expression represents the phrase is;
Hence, the expression represents the phrase “3 fewer than a number, p” is (p-3).
Write an algebraic equation for the word sentence. Eight less than a number is 19
Answers
Answer:
8-27=
Step-by-step explanation:
Answer:
x - 8 = 19
Step-by-step explanation:
in this case x is 27. because 27 - 8 = 19
Write a polynomial that represents the area of the shaded region

Answers
The polynomial that represents the area of the shaded region is given as follows:
x² - 3x + 36.
How to obtain the area of a rectangle?The area of a rectangle is given by the multiplication of the width and the length of the triangle, as follows:
A = lw.
For the entire region, the area is given as follows:
A = (x + 1)(x + 1)
A = x² + 2x + 1.
The area of the white region is given as follows:
Aw = 5(x - 7)
Aw = 5x - 35.
Then the area of the shaded region is given as follows:
As = A - Aw
A = x² + 2x + 1 - (5x - 35)
A = x² + 2x + 1 - 5x + 35
A = x² - 3x + 36.
More can be learned about area at https://brainly.com/question/15673093
#SPJ1
A moving company charges a flat rate of $150, and an additional $5 for each box. if a taxi service would charge no flat rate and $15 for each box, how many boxes would you need for it to be cheaper to use the moving company? (do not label your answer)
Answers
Answer:
16 boxes
Step-by-step explanation:
16x5=80+150=230>15x16=240
What is the present value of R13 000 p.a. invested at the beginning of each year for 8years at 10%p.a. compound interest? (NB Use the compound interest tables provided or work to three decimal places only.)
Answers
Given statement solution is :- The present value of R13,000 per year invested for 8 years at 10% compound interest is approximately R69,776.60.
To calculate the present value of an investment with compound interest, we can use the formula for the present value of an annuity:
PV = A *\((1 - (1 + r)^(-n)) / r\)
Where:
PV = Present value
A = Annual payment or cash flow
r = Interest rate per period
n = Number of periods
In this case, the annual payment (A) is R13,000, the interest rate (r) is 10% per year, and the investment is made for 8 years (n).
Using the formula and substituting the given values, we can calculate the present value:
PV = \(13000 * (1 - (1 + 0.10)^(-8)) / 0.10\)
Calculating this expression:
PV = \(13000 * (1 - 1.10^(-8)) / 0.10\)
= 13000 * (1 - 0.46318) / 0.10
= 13000 * 0.53682 / 0.10
= 6977.66 / 0.10
= 69776.6
Therefore, the present value of R13,000 per year invested for 8 years at 10% compound interest is approximately R69,776.60.
For such more questions on present value
https://brainly.com/question/30390056
#SPJ8
1.) A newspaper reporter wrote an article about a recent football game 8,749 people attended the game, but the reporter rounded the number to the nearest hundred in the article. Which number did the reporter use?
Answers
Answer:
8750
Step-by-step explanation:
what is the perimeter of the square ABCD?
Answers
Answer:
P= 4a would be the answer