TBH I don't know what grade it is but who ever answers this is gonna get brainlest. The question is if Jonny had a coupon for a 74% off and he got something for $259 how much will he have to pay.
Answers
Related Questions
Random sampling error is defined as the extent to which a sample statistic differs from a population parameter by
Answers
Random sampling error is defined as the extent to which a sample statistic differs from a population parameter by chance.
What is random sampling?Random sampling is the type of sampling method that gives all the members of a population an equal chance of being chosen.
A random sampling error is defined as the extent to which a sample statistic differs from a population parameter by chance.
Learn more about sampling here:
https://brainly.com/question/24466382
#SPJ1
What value of x makes 3/4x + 5 = 11 true?
Answers
Answer:
x=8
Step-by-step explanation:
3/4x + 5 = 11
3/4x = 6
x = 8
Step-by explanation
3/4x + 5 = 11
3/4x=6
x=8
difer from the true proportion by more than 2% ? A previous study indicates that the proportion of lefthanded sclontists is 9%. Round up to the nearest whicie number. Duestion 13 A. 1.218 B. 1,109 C. 14 D.767
Answers
The total number of samples will be 1109 .
Given ,
Margin of error 0.02
Here,
According to the formula,
\(Z_{\alpha /2} \sqrt{pq/n}\)
Here,
p = proportions of scientist that are left handed
p = 0.09
n = number of sample to be taken
Substitute the values,
\(Z_{0.01} \sqrt{0.09 * 0.91/n} = 0.02\\ 2.33 \sqrt{0.09 * 0.91/n} = 0.02\\\\\\\)
n ≈1109
Thus the number of samples to be taken will be approximately 1109 .
Know more about proportions,
https://brainly.com/question/31548894
#SPJ4
An item has a listed price of $30. If the sales tax rate is 6%, how much is the sales tax (in dollars)?
$
Answers
Answer: $ 1.80 tax, Total price + tax $31.80
Step-by-step explanation:
30 x .06 = 1.8 tax
30 x 1.06 = 31.80
What is the constant of proportionality in the equation, Y = 2.5X
Answers
Answer: 2.5 is the constant
Step-by-step explanation: a constant is the number before the variable so if 2.5 comes before "x" than 2.5 is the constant
Which of the following ordered pairs make this equation true? Y = 2x - 7 A. (1,9) B. (-3, 4) C. (4,1) D. (-1, 5)
Answers
Answer:
(4,1)
Step-by-step explanation:
Using substitution you can determine that 2*4-7 is in fact equal to 1
Compute the orthogonal projection of v [-3 7] onto the line through [7 -4] and the origin projl(v)=[]
Answers
The orthogonal projection of v onto the line through [7, -4] and the origin is [-259/65, 148/65].
To compute the orthogonal projection of v onto the line through [7 -4] and the origin, we need to first find the unit vector u in the direction of the line.
The direction vector of the line is given by [7, -4], so a unit vector in this direction is:
u = [7, -4]/sqrt(7^2 + (-4)^2) = [7/√65, -4/√65]
Next, we need to find the projection of v onto u. This is given by the dot product of v and u, multiplied by u:
proj_u(v) = (v dot u) * u
where dot denotes the dot product.
So, we have:
v = [-3, 7]
u = [7/√65, -4/√65]
v dot u = (-3)(7/√65) + 7(-4/√65) = -37/√65
proj_u(v) = (-37/√65) * [7/√65, -4/√65]
Simplifying, we get:
proj_u(v) = [-259/65, 148/65]
Therefore, the orthogonal projection of v onto the line through [7, -4] and the origin is [-259/65, 148/65].
To learn more about orthogonal visit:
https://brainly.com/question/31046862
#SPJ11
A street light is at the top of a 15 ft tall pole. A woman 6 ft tall walks away from the pole with a speed of 6 ft/sec along a straight path. How fast is the length of her shadow increasing when she is 50 ft from the base of the pole
Answers
Answer:
4 ft/sec
Step-by-step explanation:
Hope it helps
Which unit would you most likely use to represent the time it takes to drive to your friend's house, which is five miles from your house?
A. Days
B. Seconds
C. Minutes
D. Hours
Answers
Answer:
C. minutes
Step-by-step explanation:
Answer: C minutes.
Step-by-step explanation:
Solve the system of linear equations using the Gauss-Jordan elimination method.
2x1 − x2 − x3 = 1
3x1 + 2x2 + x3 = 12
x1 + 2x2 + 2x3 = 8
(x1, x2, x3) =
Answers
The solution gauss jordan elimination method is : (x1, x2, x3) = 2, 3 , 0
Given system of linear equations:
2x1 − x2 − x3 = 1
3x1 + 2x2 + x3 = 12
x1 + 2x2 + 2x3 = 8
Now,
Form the augmented matrix for the system,
\(\left[\begin{array}{cccc}2&-1&-1&1\\3&2&1&12\\1&2&2&8\end{array}\right]\)
Reduce the augmented matrix in the row echelon form,
Thus the matrix is:
\(\left[\begin{array}{cccc}1&0&0&2\\0&1&0&3\\0&0&1&0\end{array}\right]\)
Now the given system of linear equation changed to,
x1 = 2
x2 = 3
x3 = 0
Then the solution of system of linear equation is (2 , 3 , 0) .
Know more about gauss jordan elimination method,
https://brainly.com/question/30763804
#SPJ4
For many years, organized crime ran a numbers game that is now run legally by many state governments. The player selects a three-digit number from 000 to 999. There are 1000 such numbers. A bet of $2 is placed on a number, say number 115. If the number is selected, the player wins $1000. If any other number is selected, the player wins nothing.
Find the expected value for this game and describe what this means.
Answers
The given situation means that on average, a player can expect to lose $0.998 for every $2 bet placed in this game.
The expected value for this game can be calculated by multiplying the probability of winning ($1000) by the probability of selecting the winning number and subtracting the probability of losing ($2) multiplied by the probability of not selecting the winning number. Thus, the expected value is:
E(X) = (1/1000) * $1000 - (999/1000) * $2
= -$0.998
This means that on average, a player can expect to lose $0.998 for every $2 bet placed in this game. In other words, playing this game is not a good financial decision as the expected value is negative.
Learn more about Probability
https://brainly.com/question/24756209
#SPJ4
Please help meeh thank u thank u
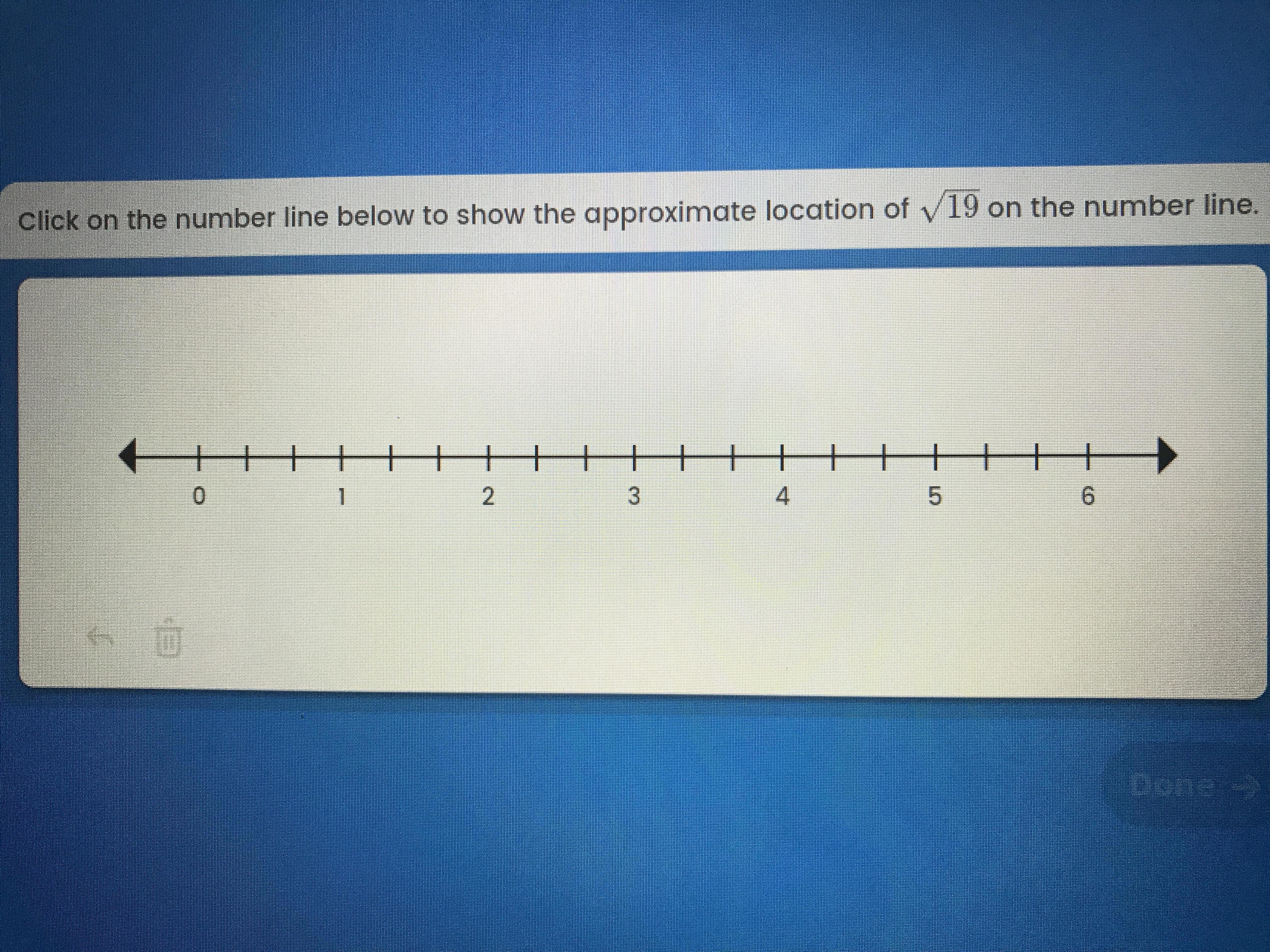
Answers
Answer:
The location would be between 4 and 5.
Step-by-step explanation:
The square root of 19 would be around 4.3. So you would put a mark between the two lines that are in between the 4 and 5.
100 nickels to dollars
5 dollars
Answers
Answer:
5$
Step-by-step explanation:
Answer:
5
Step-by-step explanation:
According to the US Forest Service, the most common tree type found in timberlands in the state of Florida is the longleaf pine tree. There are approximately 17.4 million acres of forested land in Florida. In a sample population, 35% of trees were of this type. Part A What percent expression can be used to estimate the number of acres of longleaf pine trees in Florida? Enter the correct answer in the boxes. Express the percent as a decimal.
Answers
Approximately 6.09 million acres of longleaf pine trees can be estimated to be found in the forested land of Florida, based on the given percentage.
To estimate the number of acres of longleaf pine trees in Florida, we can use the percent expression that represents the proportion of longleaf pine trees in the sample population.
Given:
Total forested land in Florida = 17.4 million acres
Percentage of longleaf pine trees in the sample population = 35%
To find the estimated number of acres of longleaf pine trees, we can multiply the total forested land by the percentage as a decimal:
Estimated acres of longleaf pine trees = 17.4 million acres * 0.35
Calculating the expression:
Estimated acres of longleaf pine trees = 6.09 million acres
Therefore, the percent expression that can be used to estimate the number of acres of longleaf pine trees in Florida is 0.35, or 35% as a decimal.
In summary, approximately 6.09 million acres of longleaf pine trees can be estimated to be found in the forested land of Florida, based on the given percentage.
Know more about longleaf pine trees here;
https://brainly.com/question/6054380
#SPJ11
define the function v : r 2 + - r by v(x1; x2) = min (u1(x1;
x2); u2(x1; x2))
Answers
The function v(x1, x2) returns the minimum value between u1(x1, x2) and u2(x1, x2), allowing us to determine the more cautious or conservative option among the two functions.
The function v(x1, x2) is defined as the minimum value between two other functions u1(x1, x2) and u2(x1, x2). It takes two input variables, x1 and x2, and returns the smaller of the two values obtained by evaluating u1 and u2 at those input points.In other words, v(x1, x2) selects the minimum value among the outputs of u1(x1, x2) and u2(x1, x2). This function allows us to determine the lower bound or the "worst-case scenario" between the two functions at any given point (x1, x2).
The function v can be useful in various contexts, such as optimization problems, decision-making scenarios, or when comparing different outcomes. By considering the minimum of u1 and u2, we can identify the more conservative or cautious option between the two functions. It ensures that v(x1, x2) is always less than or equal to both u1(x1, x2) and u2(x1, x2), reflecting the more pessimistic result among the two.
Therefore, The function v(x1, x2) returns the minimum value between u1(x1, x2) and u2(x1, x2), allowing us to determine the more cautious or conservative option among the two functions.
To learn more about minimum value click here
brainly.com/question/29210194
#SPJ11
A farmer goes to the market to sell a box of eggs. A clumsy horse steps on the box of eggs and breaks a lot of them. The horse’s rider offers to pay for all of the eggs in the box and asks the farmer how many eggs there were. The farmer does not remember the exact number, but when she took them out of the box two at a time, there was 1 egg left. The same thing happened when she took them out three, four, five and six eggs at a time, but when she took them out 7 at a time, there were no eggs left
Answers
The smallest number of eggs that could have been in the box is 1134
The problem is to find the smallest number of eggs that could have been in the box, given the remainder when taking them out by different numbers. Here are the moves toward tackling it:
Allow n to be the quantity of eggs in the container. Then we have the accompanying arrangement of congruences:
n ≡ 1 (mod 2)
n ≡ 1 (mod 3)
n ≡ 1 (mod 4)
n ≡ 1 (mod 5)
n ≡ 1 (mod 6)
n ≡ 0 (mod 7)
For this problem, we have k = 6 k = 6, a i = {1,1,1,1,1,0} a_i = {1,1,1,1,1,0}, M i = {1260,840,630,504,420,720} M_i = {1260,840,630,504,420,720}, and y i = {−1,−2,−3,-4,-5,-6} y_i = {-1,-2,-3,-4,-5,-6}.
Plugging these values into the formula and simplifying modulo 5040, we get:
n = (−1260 + −1680 + −1890 + −2016 + −2100 + 0) mod 5040
n = (−8946) mod 5040
n = (−3906) mod 5040
n = 1134 mod 5040
Therefore, the smallest number of eggs that could have been in the box is 1134
to know more about congruences click here:
https://brainly.com/question/30818154
#SPJ4
HELPPP!!!???PLEASE HELPPP!!

Answers
Answer:
\(\frac{5}{14}\) \(or\) \(0.357142857143\)
Step-by-step explanation:
__________________________________________________________
\(\frac{2}{7}\div\frac{4}{5}\) = \(\frac{5}{14}\) \(because\),
\(\frac{2}{7}\) = \(0.285714285714\)
\(\frac{4}{5}\) = \(0.8\)
\(0.285714285714\) \(\div\) \(0.8\) = \(0.357142857143\)
\(\frac{5}{14}\) = \(0.357142857143\)
__________________________________________________________
Hope this helps! <3
__________________________________________________________
Answer:
it is A
Step-by-step explanation:
Multiply them then divide them by 2
What is the value of x?
Enter your answer in the box.
x =

Answers
Step-by-step explanation:
x+55+80=180(sum of triangle)
or,x+135=180
or,x=180-135
or,x=45
Therefore,x=45°
The value of X or angle C is 45 degrees.
Given:
Two angles of triangle ABC are 80 degrees and 55 degrees.
It is required to find the value third angle X of the triangle.
The sum of three angles of a triangle is 180°.
So, the third angle, X, can be calculated as,
\(80+55+x=180\\135+x=180\\x=45^{\circ}\)
Thus, we can conclude that the value of X is 45°.
To know more about corresponding angles, refer to the link:
https://brainly.com/question/20766807
: Suppose a dog house manufacturer sells two types of dog houses. Let x represent the demand for the deluxe dog house, in thousands, and y represent the demand for the regular dog house, in thousands. If the price-demand functions for the two dog houses respectively are P1 = 8.6 – 0.4x – 0.ly P2 = 8.6 – 0.13 – 0.7y a) What is the equation of the revenue function ? R(x,y)= b) What is the revenue when the demand for deluxe dog houses is 3 and regular dog houses is 9? thousand dollars
Answers
a. The equation of the revenue function is R(x,y) = 8.6x - 0.4x² - 0.1xy + 8.6y - 0.13xy - 0.7y²
b. The revenue when the demand for deluxe dog houses is 3 and regular dog houses is 9, is $122,290
a) The revenue function can be obtained by multiplying the price and demand for each type of dog house and then adding them up. Therefore, the revenue function is:
R(x,y) = (8.6 - 0.4x - 0.1y)x + (8.6 - 0.13x - 0.7y)y
Simplifying and collecting like terms, we get:
R(x,y) = 8.6x - 0.4x² - 0.1xy + 8.6y - 0.13xy - 0.7y²
b) To find the revenue when the demand for deluxe dog houses is 3 and regular dog houses is 9, we substitute x = 3 and y = 9 into the revenue function:
R(3,9) = 8.6(3) - 0.4(3)² - 0.1(3)(9) + 8.6(9) - 0.13(3)(9) - 0.7(9)²
Simplifying and calculating, we get:
R(3,9) = $122.29 thousand
Therefore, the revenue is approximately $122,290 when the demand for deluxe dog houses is 3 and regular dog houses is 9, in thousands of dollars.
Learn more about revenue function at https://brainly.com/question/29148322
#SPJ11
please help 17 points
Answers
Answer:
17
Step-by-step explanation:
Answer:
The answer is N/A.
Step-by-step explanation:
You must put up a problem to solve.
Question 3 of 10
Which equation represents the slope-intercept form of the line below

Answers
Answer:
\(y = \frac{1}{2}x + 8\)
Step-by-step explanation:
The general slope-intercept equation is: y = mx + b.
In this equation, "m" is equal to the slope and "b" is equal to the y-intercept.
Since the slope is "1/2", then this is the "m" value.
Since the line passes the y-axis at "8" when x = 0, then "b" must be "8".
Therefore, the equation of the function in slope-intercept form is \(y = \frac{1}{2}x + 8\).
A plane (at point P) sees a bridge (at point B) at an angle of depression on 28 degrees. The horizontal distance between the plane and the bridge is 3000 feet. Explain which trigonometric equation can be used to solve for the height of the plane (segment PA). What is the height of the plane? You must show all work and calculations to receive full credit. Round your answer to the nearest foot.

Answers
The height of the plane PA is given as; 1591 ft
How to use trigonometric ratios?
There are different trigonometric ratios such as;
cos θ = adjacent/hypotenuse
sin θ = opposite/hypotenuse
tan θ = opposite/adjacent
Now, looking at the given image showing plane and bridge indicated by lines, we can use one of the trigonometric ratios to get the height of the plane PA as;
PA/3000 = tan B
now angle B = 28 degrees from alternate angles postulate. Thus;
PA/3000 = tan 28
PA = 3000 * tan 28
PA = 3000 * 0.5317
PA = 1591 ft
Read more about trigonometric ratios at; https://brainly.com/question/13276558
#SPJ1
\(tan 28^o = \frac{PA}{3000}\)
tan 28° · 3000= PA
(use a scientific calculator)
1595.128= PA
height = 1595
Solve the equation.
,3
y =1,000
Answers
Answer:
I have stated the answer about hope it helps

Use the given information to select the factors of f(x)
f(4)=0
f(-1)=0
f(3/2)=0
Make sure to select ALL correct answers.
(x+1)
(x-1)
(3x+2)
(x+4)
(2x-3)
(2x+3)
(3x-2)
(x-4)
(right answers only!)
Answers
4 is factor of f(x)=x-4, -1 is factor of f(x)=(x+1) and 3/2 is factor of f(x)=2x-3
What is meant by Factor?Factor: A factor is a number that divides another number, leaving no remainder. In other words, if multiplying two whole numbers gives us a product, then the numbers we are multiplying are factors of the product because they are divisible by the product. There are two methods of finding factors: multiplication and division
substitute all x values in the given options to find the factors of f(x)
f(4)=0
substitute in all options
f(4)=4+1 =5
f(4)=4-1=3
f(4)=3(4)+2=14
f(4)=4+4=8
f(4)=2(4)-3=5
f(4)=2(4)+3=11
f(4)=3(4)-2=10
f(4)=4-4=0
4 is one factor of f(x)=x-4
now substitute -1 in all the options
f(-1)=-1+1=0
f(-1)=-1-1=2
f(-1)=3(-1)+2=-1
f(-1)=-11+4=-7
f(-1)=2(-1)-3=-5
f(-1)=2(-1)+3=1
f(-1)=3(-1)-2=-5
f(-1)=(-1)-4=-5
-1 is one factor of f(x)=x+1
now similarly substitute 3/2 in all the options which gives
3/2 is one factor of f(x)=2x-3
to learn more about factors visit:
brainly.com/question/14452738
#SPJ1
Acellus Find the distance between the two points. (-3,-4) (2,2) ✓ [?]
![Acellus Find the distance between the two points. (-3,-4) (2,2) [?]](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/1iPBtACfFDmNu5VkHvjKwUykbMdj2OXa.jpeg)
Answers
Answer:
√61
Step-by-step explanation:
→ Find the distance between y coordinates
2 --4 = 6
→ Find distance between x coordinates
2--3 = 5
→ Find distance
√6² + 5² = √36 + 25 = √61
Determine the intercepts of the line.
y- 6 = 4(x + 5)
y-intercept:
x-intercept:
Answers
Answer:
x intercept: -13/2
y intercept: 26
Step-by-step explanation:
x intercept of the line is the point where y = 0
Substitute y = 0 into the expression;
0 - 6 = 4(X+5)
-6 = 4x + 20
4x = -6-20
4x = -26
x = -26/4
x = -13/2
y intercept is the point where x = 0
y - 6 = 4(0+5)
y-6 = 20
y = 20+6
y = 26
Someone pls help me I will make you as brain

Answers
Answer:
It's -3 :)
Step-by-step explanation:
g(x) = 1 and f(x) = -3 and to find k you need to do f(x)/g(x). Therefore, -3/1 = -3. Hope this helped!
Explanation: I got it right
please help. first one in gets brainliest along with 5* and thanks.

Answers
Answer:
18-x
18/2=6
18-5=13
18/2=6
18-5=13 I’m not sure
Use the linear interpolation method to establish the value of n
which corresponds to A/G = 5.4000 and i = 8% per year with annual
compounding.
Answers
Using linear interpolation, the value of n that corresponds to A/G = 5.4000 and i = 8% per year with annual compounding is approximately 5.40 years.
Linear interpolation is a method used to estimate values between two known data points. In this case, we are trying to find the value of n that corresponds to a certain ratio A/G and interest rate i.
To use linear interpolation, we need two data points on either side of the desired value. Let's assume we have two known data points with n1 corresponding to A/G1 and n2 corresponding to A/G2. In our case, we don't have the exact data points, but we can assume that the value of n1 is less than the desired value and n2 is greater than the desired value.
Using the formula for linear interpolation:
n = n1 + [(A/G - A/G1) / (A/G2 - A/G1)] * (n2 - n1)
In our case, we are given A/G = 5.4000 and i = 8% per year with annual compounding. We need to find the value of n corresponding to this ratio.
Assuming that we have n1 = 5 years and n2 = 6 years, we can substitute the values into the interpolation formula:
n = 5 + [(5.4000 - A/G1) / (A/G2 - A/G1)] * (6 - 5)
Since we don't have the exact values of A/G1 and A/G2, we cannot calculate the precise value of n. However, the result will be approximately 5.40 years.
Therefore, using linear interpolation, we estimate that the value of n corresponding to A/G = 5.4000 and i = 8% per year with annual compounding is approximately 5.40 years.
Learn more about interest rates here:
brainly.com/question/29990363
#SPJ11
A right pyramid with a square base has a base edge length of 24 feet and a slant height of 20 feet. What is the height of the pyramid? 4 feet 8 feet 12 feet 16 feet.
Answers
The height of the pyramid is 16 feet.
What is Pythagoras Theorem?
Pythagoras' theorem is a fundamental principle in geometry that states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
We can use the Pythagorean theorem to find the height of the pyramid.
The slant height of the pyramid is the hypotenuse of a right triangle whose legs are the height of the pyramid and half the length of the base of the pyramid. Since the base is a square, half the length of the base is 12 feet.
Using the Pythagorean theorem:
height² + 12² = 20²
height² = 20² - 12²
height² = 256
height = 16 feet
Therefore, the height of the pyramid is 16 feet.
To know more about Pythagoras theorem visit:
https://brainly.com/question/343682
#SPJ4