Solve by factoring:
x² – 5x - 14=0
O A. x = 7,X = -2
B. x = -7, x = 2
O C. x = -1, x = 14
O D. x=-14, x = 1
Answer ASAP!
Answers
Answer:
A
Step-by-step explanation:
Given
x² - 5x - 14 = 0
Consider the factors of the constant term (- 14) which sum to give the coefficient of the x- term (- 5)
The factors are - 7 and + 2, since
- 7 × 2 = - 14 and - 7 + 2 = - 5, thus
(x - 7)(x + 2) = 0
Equate each factor to zero and solve for x
x - 7 = 0 ⇒ x = 7
x + 2 = 0 ⇒ x = - 2
Answer:
A. x=-7, x=2
Step-by-step explanation:
Related Questions
4.
Which point on the number line
represents 0.3?

Answers
Answer:
Point C
Step-by-step explanation:
because the number is 0.3 you need to start at zero on the number line and go forward 3 marks. That will lead you to your answer of 0.3
Answer: it’s C because it’s the one that’s closer to 3 if u need help just ask
Step-by-step explanation:
Hope this helps
-3
Given m||n, find the value of x. (5x-23)° (7x-1)°

Answers
Answer:
X=17
Step-by-step explanation:
5x-23+7x-1=180
12x=180+24
12x=204
x=17
(a) Find the values of z, zER, for which the matrix
x3 x
9 1
has inverse (marks-2 per part)
x=
x=
x=
(b) Consider the vectors - (3,0) and 7- (5,5).
(i.) Find the size of the acute angle between i and ü. Angle-
(ii). If -(k, 3) is orthogonal to , what is the value of ke k [2 marks]
(c) Let J be the linear transformation from R2 R2 which is a reflection in the horizontal axis followed by a scaling by the factor 2.
(i) If the matrix of J is W y 1₁ what are y and z
y= [2 marks]
z= [2 marks] U N || 62 -H 9 has no inverse. [6 marks-2 per part] [2 marks]
(d) Consider the parallelepiped P in R³ whose adjacent sides are (0,3,0), (3, 0, 0) and (-1,1, k), where k € Z. If the volume of P is 180, find the two possible values of k. [4 marks-2 each]
k=
k=
(e) Given that the vectors = (1,-1,1,-1, 1) and =(-1, k, 1, k, 8) are orthogonal, find the magnitude of . Give your answer in surd form. [3 marks]
v=
Answers
(a) To find the values of z for which the matrix does not have an inverse, we can set up the determinant of the matrix and solve for z when the determinant is equal to zero.
The given matrix is:
|x3 x|
|9 1|
The determinant of a 2x2 matrix can be found using the formula ad - bc. Applying this formula to the given matrix, we have:
Det = (x3)(1) - (9)(x) = x3 - 9x
For the matrix to have an inverse, the determinant must be non-zero. Therefore, we solve the equation x3 - 9x = 0:
x(x2 - 9) = 0
This equation has two solutions: x = 0 and x2 - 9 = 0. Solving x2 - 9 = 0, we find x = ±3.
So, the values of x for which the matrix has no inverse are x = 0 and x = ±3.
(b) (i) To find the size of the acute angle between the vectors (3,0) and (5,5), we can use the dot product formula:
u · v = |u| |v| cos θ
where u and v are the given vectors, |u| and |v| are their magnitudes, and θ is the angle between them.
Calculating the dot product:
(3,0) · (5,5) = 3(5) + 0(5) = 15
The magnitudes of the vectors are:
|u| = sqrt(3^2 + 0^2) = 3
|v| = sqrt(5^2 + 5^2) = 5 sqrt(2)
Substituting these values into the dot product formula:
15 = 3(5 sqrt(2)) cos θ
Simplifying:
cos θ = 15 / (3(5 sqrt(2))) = 1 / (sqrt(2))
To find the acute angle θ, we take the inverse cosine of 1 / (sqrt(2)):
θ = arccos(1 / (sqrt(2)))
(ii) If the vector (-k, 3) is orthogonal to (5,5), it means their dot product is zero:
(-k, 3) · (5,5) = (-k)(5) + 3(5) = -5k + 15 = 0
Solving for k:
-5k = -15
k = 3
So, the value of k is 3.
(c) Let J be the linear transformation from R2 to R2 that reflects points in the horizontal axis and then scales them by a factor of 2. The matrix of J can be found by multiplying the reflection matrix and the scaling matrix.
The reflection matrix in the horizontal axis is:
|1 0|
|0 -1|
The scaling matrix by a factor of 2 is:
|2 0|
|0 2|
Multiplying these two matrices:
J = |1 0| * |2 0| = |2 0|
|0 -1| |0 2| |0 -2|
So, the matrix of J is:
|2 0|
|0 -2|
Therefore, y = 2 and z = -2.
(d) The volume of a parallelepiped can be found by taking the dot product of two adjacent sides and then taking the absolute value of the result.
The adjacent sides of the parallelepiped P are (0,3,0)
To learn more about scaling matrix click here : brainly.com/question/16662440
#SPJ11
**30P** BEST ANSWER GET BRAINLIEST

Answers
The areas of the composite figures are, respectively:
Case A: A = 104 m²
Case B: A = 174 m²
Case C: A = 321.461 cm²
Case D: A = 20 m²
How to determine the area of a composite figure
In this problem we need to determine four case of composite figures, the area of each composite figure is the result of adding or subtracting the areas of different figures. The area formulas to be used below are introduced below:
Rectangle
A = w · l
Triangle
A = 0.5 · w · l
Quarter of a circle
A = 0.25π · r²
Where:
w - Widthl - Lengthr - RadiusA - AreaFinally we determine the area of the composite figures:
Case A
A = (10 m) · (8 m) + 0.5 · (8 m) · (6 m)
A = 80 m² + 24 m²
A = 104 m²
Case B
A = (12 m)² + 0.5 · (12 m) · √[(13 m)² - (12 m)²]
A = 174 m²
Case C
A = (20 cm)² - 0.25π · (10 cm)²
A = 321.461 cm²
Case D
A = (4 m) · (6 m) - 0.5 · (4 m) · (2 m)
A = 24 m² - 4 m²
A = 20 m²
To learn more on areas of composite figures: https://brainly.com/question/23718948
#SPJ1
5. Solve these equations.
a. N + 2.3 = -4.7
b .2N + 6.8 = -10.2
C. N=4= -2.7
Answers
Answer:
a. N + 2.3=-4.7
N= -4.7-2.3
N=. -7
b. 2N+6.8=-10.2
2N= -10.2-6.8
2N= -17
N= -17/2
c. N +4 = -2.7
N= -2.7-4
N = -6.7
What is the formula for the indicated variables? A-lw wh + lh, for w
Answers
Hello there. To solve this question, we have to remember some properties about solving equations for a variable.
Given the following equation:
\(A=lw+wh+lh\)We want to solve it for w.
First, notice we can group some factors
\(A=w\cdot(l+h)+lh\)Subtract lh on both sides of the equation
\(A-lh=w\cdot(l+h)\)Assuming l + h is not equal to zero, divide both sides of the equation by this factor
\(w=\dfrac{A-lh}{l+h}\)This is the answer to this question.
please help!!
Type the correct answer in each box. Use numerals instead of words.

Answers
b = 6
c = 5
Hope this helps
Dario increased his savings from $350 to $413. What is Dario's percent increase in
savings?
Answers
Answer:
18%
Step-by-step explanation:
63/350 × 100 = 18
hope this helps
To sew a pair of jeans, 630 feet of thread is needed. Unfortunately the fabric store only sells thread in yards. How many yards of fabric would you need to buy from the store to make a pair of jeans?
Answers
Answer:
210
Step-by-step explanation
One yard is equal to 3 feet so the simplest way is to divide the number of feet by 3 and that will give you your answer.
Answer:
210
Step-by-step explanation:
a sample of 50 lenses used in eyeglasses yields a sample mean thickness of 3.05 mm and a sample standard devia- tion of .34 mm. the desired true average thickness of such lenses is 3.20 mm. does the data strongly suggest that the true average thickness of such lenses is some- thing other than what is desired? test using a 5 .05.
Answers
A type of statistical hypothesis known as a "null hypothesis" makes the case that a given set of observations does not have any statistical significance.
Using sample data, hypothesis testing is used to determine a hypothesis's credibility. It is represented as H0, and it is sometimes referred to as the "null."
Given; The average thickness and standard deviation of a sample of 50 eyeglass lens samples are 3.05 millimeters and.34 millimeters, respectively.
Such lenses should have a true average thickness of 3.20 mm. Utilizing a =.05
False hypothesis H0: Alternative hypothesis: mu1= mu2 HA: 'mu1' and 'ne'
Sample Size 50 Sample Mean 3.05 Sample Standard Deviation 0.34 Intermediate Calculations Standard Error of the Mean 0.0481 Degrees of Freedom 49 t Test Statistic -3.1196 Two-Tail Test Lower Critical Value -2.0096 Upper Critical Value 2.0096 p-Value 0.0030 Reject the null hypothesis
To learn more about Null hypothesis here
https://brainly.com/question/28920252
#SPJ4
what two positive real numbers whose product is 11 have the smallest possible sum?
Answers
The two positive real numbers with a product of 11 that have the smallest possible sum are √11 and √11. In decimal form, these numbers are approximately 3.3166 and 3.3166.
To determine two positive real numbers whose product is 11 and have the smallest possible sum, we can use the concept of the arithmetic mean-geometric mean inequality.
Let the two numbers be x and y.
We have the following conditions:
1. xy = 11 (product of the two numbers is 11)
2. x > 0, y > 0 (both numbers are positive)
According to the AM-GM inequality, the arithmetic mean of two numbers is always greater than or equal to the geometric mean of those numbers.
Using this inequality, we have:
(x + y)/2 ≥ √(xy)
Substituting the value of xy as 11, we get:
(x + y)/2 ≥ √11
To minimize the sum (x + y), we need to make it as close as possible to the right side of the inequality.
This occurs when equality holds, that is, when:
x = y = √11
So, the answer is approximately (3.3166, 3.3166).
To know more about arithmetic mean-geometric mean refer here:
https://brainly.com/question/28145873#
#SPJ11
statistics the art and science of learning from data 4th edition
Answers
"Statistics: The Art and Science of Learning from Data" (4th edition) is a valuable resource for understanding and applying statistical principles, providing insights into data analysis and decision-making processes.
Statistics is the art and science of learning from data. It involves collecting, organizing, analyzing, interpreting, and presenting data to gain insights and make informed decisions. In the 4th edition of the book "Statistics: The Art and Science of Learning from Data," you can expect to find a comprehensive exploration of these topics.
This edition may cover important concepts such as descriptive statistics, which involve summarizing and displaying data using measures like mean, median, and standard deviation. It may also delve into inferential statistics, which involve making inferences and drawing conclusions about a population based on a sample.
Additionally, the book may discuss various statistical techniques such as hypothesis testing, regression analysis, and analysis of variance (ANOVA). It may also provide real-world examples and case studies to illustrate the application of statistical methods.
When using information from the book, it is important to properly cite and reference it to avoid plagiarism. Be sure to consult the specific edition and follow the guidelines provided by your instructor or institution.
In summary, "Statistics: The Art and Science of Learning from Data" (4th edition) is a valuable resource for understanding and applying statistical principles, providing insights into data analysis and decision-making processes.
Know more about Statistics here,
https://brainly.com/question/31538429
#SPJ11
Pls help me I’m struggling with this math I’ll mark brainliest
Which of the following statements about XY and XZ is true?

Answers
5. What is the length of the unknown leg?

Answers
Answer: a = 8
Step-by-step explanation:
a² + b² = c²
a² + 6² = 10²
a² + 36 = 100
a² = 100 - 36
a² = 64
a = √64
a = 8
a dealership is allowing residents to test drive the following vehicles: 2 sports cars, 3 vans, and 3 trucks. if you select a random car to test drive, what are the odds it is not a sports car? write your answer as 1:2 which is reduced as much as possible.
Answers
Step-by-step explanation:
To find probability ratio wise
Sports Cars: Total Cars
3 + 3 + 2 = 8 total cars
2 sports cars
Ratio is 2:8
Reduces to 1:4
given three points that lie on the parabola, find the equation that represents this parabola in standard form
Answers
The equation that represents this parabola in standard form is y = x²
Find the equation that represents this parabola in standard formFrom the question, we have the following parameters that can be used in our computation:
(0,0) , (1,1) and (-1, 1)
A parabola in standard form is represented as
y = ax² + bx + c
Using the points, we have
(0)²a + (0)b + c = 0
c = 0
Next, we have
(1)²a + (1)b + 0 = 1
a + b = 1
(-1)²a + (-1)b + 0 = 1
a - b = 1
Add the equations
2a = 2
a = 1
So, we have
1 + b = 1
b = 0
This means that the equation is y = x²
Read more about parabola at
https://brainly.com/question/4061870
#SPJ1
Question
Given three points that lie on the parabola, find the equation that represents this parabola in standard form
(0,0) , (1,1) and (-1, 1)
if 35% of a number is 150, find 15% if the number
Answers
Answer:
64 2/7
Step-by-step explanation:
0.35x = 150
x = 3000/7
0.15(3000/7) = 450/7 = 64 2/7
Joey bought cupcakes for his sister's birthday party. 90% of the 50 cupcakes had sprinkles on top. How many cupcakes had sprinkles?
Answers
Answer:
45 cupcakes.
Step-by-step explanation:
90% of 50 = 45.
Hope this helps :)
what equation represents this sentence? 28 is the quotient of a number and 4. responses 4=n28 4 equals n over 28 28=n4 28 equals n over 4 28=4n 28 equals 4 over n 4=28n 4 equals 28 over n
Answers
The equation that represents the sentence "28 is the quotient of a number and 4" is 28 = n/4.
In the given sentence, "28 is the quotient of a number and 4," we can break down the sentence into mathematical terms. The term "quotient" refers to the result of division, and "a number" can be represented by the variable "n." The divisor is 4.
1) Define the variable.
Let's assign the variable "n" to represent "a number."
2) Write the equation.
Since the sentence states that "28 is the quotient of a number and 4," we can write this as an equation: 28 = n/4.
The equation 28 = n/4 represents the fact that the number 28 is the result of dividing "a number" (n) by 4. The left side of the equation represents 28, and the right side represents "a number" divided by 4.
To know more about equation refer here:
https://brainly.com/question/29657983
#SPJ11
Will the following lengths make a triangle? yes/no? (Will give brainy)
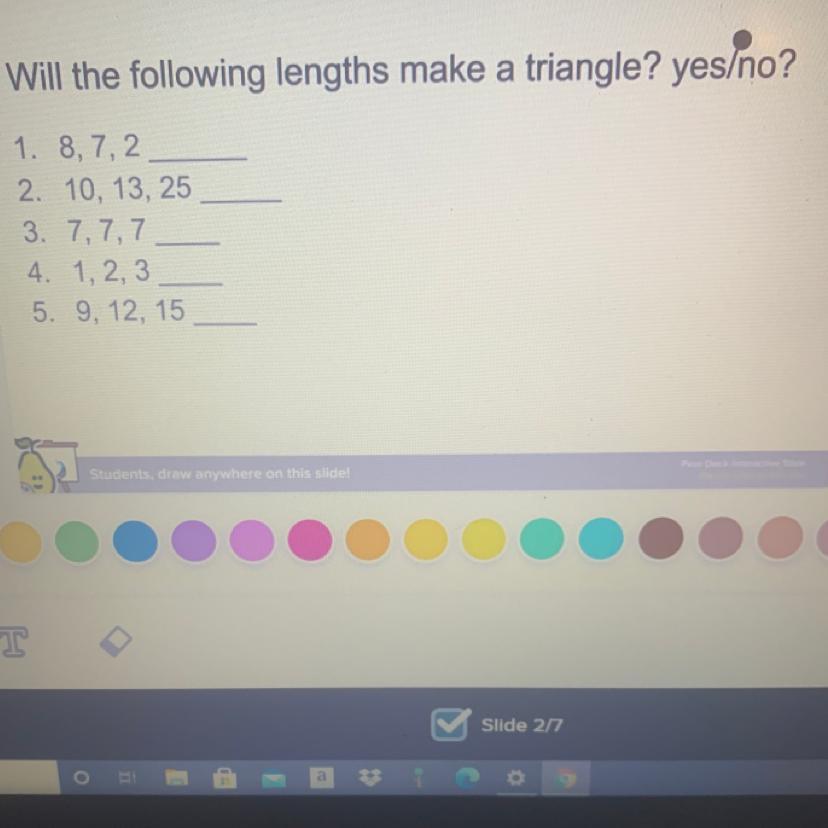
Answers
Answer:
1. Yes
2. No
3. Yes
4. No
5. Yes
Step-by-step explanation:
1. Yes
2. No
3. Yes
4. No
5. Yes
In order to be sure whether given lengths form a triangle or not, we should know the following property.
If sum of any two lengths is larger than the third length then it will form a triangle otherwise not.
Construct a confidence interval for p1 - p2 at the given level of confidence. x1 = 356, n1 = 543, x2 = 413, n2 = 589,99% confidence The researches are __% confident the difference between the two population proportions, p1 - p2, is between and
Answers
The researchers are 99% confident that the difference between the two population proportions, p1 - p2, is between -0.0438 and 0.1364.
To construct a confidence interval for the difference between two population proportions, we can use the formula:
CI = (p1 - p2) ± Z * √[(p1(1 - p1)/n1) + (p2(1 - p2)/n2)]
where p1 and p2 are the sample proportions, n1 and n2 are the respective sample sizes, and Z is the critical value corresponding to the desired level of confidence.
In this case, x1 = 356, n1 = 543, x2 = 413, n2 = 589, and the confidence level is 99%. First, we calculate the sample proportions: p1 = x1/n1 = 356/543 ≈ 0.6552 and p2 = x2/n2 = 413/589 ≈ 0.7012.
Next, we determine the critical value Z for a 99% confidence level, which corresponds to a two-tailed test. From the standard normal distribution table or a calculator, Z ≈ 2.576.
Substituting the values into the formula, we calculate the confidence interval:
CI = (0.6552 - 0.7012) ± 2.576 * √[(0.6552(1 - 0.6552)/543) + (0.7012(1 - 0.7012)/589)]
≈ -0.0438 ± 2.576 * 0.0248
Simplifying, we get the confidence interval as -0.0438 ± 0.0638.
The researchers are therefore 99% certain that the difference between the two population proportions, p1 - p2, is between -0.0438 and 0.1364.
Learn more about critical value here:
https://brainly.com/question/31405519
#SPJ11
what is the sum of 4+(−3)+(−2) ?
Answers
Answer: -1
Step-by-step explanation: 4 + (-3) = 1 1 + (-2) = -1
How would you suggest that managers avoid the quick-fix mentality that makes management by best-seller so tempting?
Answers
You can suggest that managers should avoid the quick-fix mentality that makes management by best-seller so tempting by implementing the following strategies:
1. Understand the Unique Nature of Your Business: Managers should take the time to understand the unique nature of their business. They should realize that what worked for another company may not work for their own company.
2. Avoid Short-Term Solutions: Managers should avoid short-term solutions that provide quick results. They should focus on long-term solutions that will benefit the organization in the long run.
3. Develop a Comprehensive Strategy: Managers should develop a comprehensive strategy that includes long-term goals and objectives. They should also have a plan in place to achieve those goals.
4. Invest in Employee Development: Managers should invest in employee development by providing training and development opportunities. This will help to build a strong workforce that is capable of handling complex challenges.
5. Encourage Collaboration: Managers should encourage collaboration among team members. This will help to create a culture of teamwork and cooperation.
6. Monitor Progress: Managers should monitor progress and adjust strategies as necessary. This will help to ensure that the organization is on track to achieving its goals.
By following these strategies, managers can avoid the quick-fix mentality that makes management by best-seller so tempting. They can focus on long-term solutions that will benefit the organization in the long run.
Hope this helped you...
< Back to Content
4
Use the graph that shows the solution to f(x) = g(x).
f(x) = - *x² + 3x + 1
g(x) = x - 1
f(x)
ox)
What is the solution to f (x) = g(x)?
4
-3-2
x = 0
x = 2
f(x)
3
2
g(x)
2

Answers
Answer:
x = 4
Step-by-step explanation:
Both A and B are similar
We have no problems with f(x) as it is similar on both plots
A graph of g(x) however shows that it is the second plot that is correct
Thus,
To get the solution , we need the point at which both graphs crosses each other
we can see this at the point x = 4 and thus that is our solution
The double-reciprocal transformation of the Michaelis-Menten equation, also called the Lineweaver- Burk plot, is given by
1/V_0 = K_m /(V_max[S]) + 1/V_max
To determine Km from a double-reciprocal plot, you would:
A) multiply the reciprocal of the x-axis intercept by -1.
B) multiply the reciprocal of the y-axis intercept by -1.
C) take the reciprocal of the x-axis intercept.
D) take the reciprocal of the y-axis intercept.
E) take the x-axis intercept, where V_0 = 1/2 V_max.
Answers
To determine Km from a double-reciprocal plot, you would choose option (A) which is to multiply the reciprocal of the x-axis intercept by -1.
In the double-reciprocal plot equation, the x-axis intercept is -1/Km, and the y-axis intercept is 1/Vmax. Therefore, if you take the reciprocal of the x-axis intercept, you get -Km, and multiplying it by -1 gives you Km.
This method is preferred because it is more accurate than estimating Km based on the position of the curve on the plot or by taking the x-axis intercept where V0 = 1/2 Vmax, which can be influenced by experimental error.
To learn more about reciprocal here:
https://brainly.com/question/30519664
#SPJ4
Can someone help me ASAP. I will give brainliest if possible. Due today.
Part A: What type of figure is shown above
Part B: Find the surface area of the figure
Part C: Show your work to support your answer in part B

Answers
a. The type of figure shown is a triangular prism.
b. The surface area of the figure is 118.5 sq. in.
What is a triangular prism?A triangular prism is a 3 dimensional shape which has 2 triangular surfaces and a rectangular base. It has 5 surfaces in total.
Considering the given figure in the question, we have;
a. The type of figure shown is a triangular prism.
b. The surface area of the prism can be deduced as follows;
area of one of its triangular surfaces = 1/2*base*height
= 1/2*5*1.7
= 4.25 sq. in.
area of its base = length x width
= 10x 5
= 50 sq. in.
area of one of its slant sides = length x width
= 10 x 3
= 30 sq. in.
Therefore, the surface area of the figure = (2*4.25) + 50 + (2*30)
= 8.5 + 50 + 60
= 118.5 sq. in.
Learn more about triangular prism at https://brainly.com/question/30739454
#SPJ1
Need help with this

Answers
Answer:
search it up
Step-by-step explanation:
in three flips of an unfair coin the probability of getting three heads is the same as the probability of getting exactly two tails. what is the ratio of the probability of flipping a tail to the probability of flipping a head?
Answers
The ratio of the probability of flipping a tail to the probability of flipping a head when the probability of getting three heads is the same as the probability of getting exactly two tails in three flips is 1/3.
How to find the ratio of probability of flipping a tail to probability of flipping a head?Let p be the probability of flipping a head and q be the probability of flipping a tail.
The probability of getting three heads in three flips is \((p)^3.\)
The probability of getting exactly two tails in three flips is 3pq².
Given that these probabilities are equal, we have:
\((p)^3\)= 3pq²
Simplifying this equation gives:
p = 3q
Dividing both sides by q gives:
p/q = 3
Therefore, the ratio of the probability of flipping a tail to the probability of flipping a head is 1/3.
Learn more about Probability
brainly.com/question/30034780
#SPJ11
Find the width of a rectangular strip of land with length 25 m and area 12 square meters?

Answers
Answer:
5/4
Step-by-step explanation:
The area of a rectangle is given by
A = l*w
1/2 = 2/5 * w
Multiply each side by 5/2
5/2 * 1/2 = 2/5 *w * 5/2
5/4 = w
Formula we use,
→ A = l × w
Then the width of the rectangle,
→ A = I × w
→ 1/2 = 2/5 × w
Now multiply each side by 5/2,
→ 1/2 × 5/2 = 2/5 × w × 5/2
→ w = 5/4
Hence, the width is 5/4.
Someone help big ideas 8.6 number 20

Answers
Answers:
smaller = 45larger = 135============================================================
Explanation:
Let x and y be the two angles such that x < y. Also, we'll make both values to be positive numbers.
Since one angle is 1/3 of the other angle, this means
smaller angle = (1/3)*(larger angle)
x = (1/3)y
3x = y
y = 3x
The two angles add to 180 degrees since they are a linear pair. A linear pair of angles always forms a straight line.
x+y = 180
x+3x = 180
4x = 180
x = 180/4
x = 45
Use that x value to find y.
y = 3x
y = 3*45
y = 135
The two angles are x = 45 and y = 135
---------
Check:
x+y = 45+135 = 180
so the answer is confirmed.