Ryan exponential function of the form f(t)= a×b^t to represent the following situation. assume t is in years. an account has an initial investment of $91,000, increasing by 3.76% per year. F(T)=_________
Answers
Answer
\(f(t) = 91000 *1.0376^t\)
Explanation
Given
\(a = 91000\) -- initial
\(r = 3.76\%\)
Required
The exponential equation
The exponential equation is:
\(y =ab^t\)
Where
\(b=1 +r\)
We used minus, because the rate increases..
So, we have:
\(b=1 +3.76\%\)
\(b=1 +0.0376\)
\(b=1.0376\)
So:
\(y =ab^t\)
\(y = 91000 *1.0376^t\)
Hence:
\(f(t) = 91000 *1.0376^t\)
Related Questions
Please help with writing linear functions

Answers
The function would be \(f(x)=\frac{1}{2}x + 1 \ and\ f(x)=\frac{1}{2}x - \frac{1}{2}\)
What is linear function?
A linear function is one with one or two variables that does not have exponents. It is a function that has a straight line graph.
A linear function has the form f(x) = mx + b, where m is the slope and b is the y-intercept. To find the equation of the linear function given the values of x and f(x), we can use two points and use the slope-intercept form of the equation.
A way to find the equation of the linear function is to use the point-slope form: f(x) = m(x-x1) + f(x1), where (x1,f(x1)) is a point on the line.
If we use the points (-4,-2) and (0,0) we can find the slope:
m = (0 - (-2)) / (0 - (-4)) = 2/4 = 1/2
then using point-slope form:
f(x) = 1/2(x - (-4)) + (-2)
f(x) = 1/2x + 1
so the equation of the linear function is f(x) = 1/2x + 1
Alternatively, we can use the two points (-2,-1) and (0,0) we can find the slope:
m = (0 - (-1)) / (0 - (-2)) = 1/2
then using point-slope form:
f(x) = 1/2(x - (-2)) + (-1)
f(x) = 1/2x - 1/2
so the equation of the linear function is f(x) = 1/2x - 1/2.
Therefore, equation f(x) = 1/2x+1 and f(x) = 1/2x - 1/2 represents the given values of x and f(x)
To know more about linear functions visit,
https://brainly.com/question/2248255
#SPJ1
I need help with 19 math problems. I will give 50 brainly points if you help me. The problems are due tomorrow. I am desparate
Answers
A basketball team plays half of its games during the day and half at night. Ten scores from day games and ten scores from night
games were randomly selected by the team's statistician. The following statistical information was calculated from the final game
scores.
Day Night
Mean 58 72
Median 46 63
Mode 50. 70
Range 21 33
Based on these samples, what generalization can be made?
A. The basketball team scored the same number of points in day games as night games.
OB. The basketball team scored more points in night games than in day games.
OC. The basketball team scored more points in day games than in night games.
OD. Not enough information is provided to draw any of these conclusions,
Answers
Option B
Because the average points scored in the night is more than that of the day
Solve the equations:
5_r-2)^2\(9x^2=36\)
\(5(r-2)^2=35\\\)
\(-4(k-8)^2=16\)
Answers
1. The solution of 5(r-2)² = 35 is r = ±√7 + 2.
2. The solution of -4(k-8)² = 16 is k = (16 ± √(-16)) / 2.
1. 5(r-2)² = 35
First, divide both side by 5 we get
5(r-2)²/5 = 35 /5
(r-2)² = 7
Take square root on both side
r-2 = ±√7
r = ±√7 + 2
2. -4(k-8)² = 16
Divide both side by -4 we get
(k-8)² = -4
k² + 64 - 16k + 4 = 0
k² - 16k + 68 = 0
On solving the quadratic equation we get
k = (16 ± √(-16)) / 2
Learn more about equation here:
https://brainly.com/question/29657983
#SPJ1
What is the mean of the data set below?12, 11, 14, 14, 14, 12, 7
Answers
So 7+11+12+12+14+14+14=84
There is 7 numbers in the data set...
So 84/7=12
12 is your mean
Hope this helps! Have a nice day :)
A machine at a soft-drink bottling factory is calibrated to dispense 12 ounces of cola into cans. A simple random sample of 35 cans is pulled from the line after being filled and the contents are measured. The mean content of the 35 cans is 11.92 ounces with a standard deviation of 0.085 ounce.
Estimate the true mean contents of the cans being filled by this machine with 95% confidence.
Answers
Answer:
The true mean contents of the cans being filled by this machine with 95% confidence is between 11.891 ounces and 11.949 ounces.
Step-by-step explanation:
We have the standard deviation for the sample, so we use the t-distribution to solve this question.
The first step to solve this problem is finding how many degrees of freedom, we have. This is the sample size subtracted by 1. So
df = 35 - 1 = 34
95% confidence interval
Now, we have to find a value of T, which is found looking at the t table, with 34 degrees of freedom(y-axis) and a confidence level of \(1 - \frac{1 - 0.95}{2} = 0.975\). So we have T = 2.032
The margin of error is:
\(M = T\frac{s}{\sqrt{n}} = 2.032\frac{0.085}{\sqrt{35}} = 0.029\)
In which s is the standard deviation of the sample and n is the size of the sample.
The lower end of the interval is the sample mean subtracted by M. So it is 11.92 - 0.029 = 11.891 ounces.
The upper end of the interval is the sample mean added to M. So it is 11.92 + 0.029 = 11.949 ounces.
The true mean contents of the cans being filled by this machine with 95% confidence is between 11.891 ounces and 11.949 ounces.
Simplify (w3)4•(w5)2
Answers
Answer:
\(w^{22}\)
Step-by-step explanation:
\((w^3)^4\cdot(w^5)^2=w^{3*4}\cdot w^{5*2}=w^{12}\cdot w^{10}=w^{12+10}=w^{22}\)
Determine the equation of the line below using the given slope and point.
Slope = m = 4 , Point (-3,-11)

Answers
\((\stackrel{x_1}{-3}~,~\stackrel{y_1}{-11})\hspace{10em} \stackrel{slope}{m} ~=~ 4 \\\\\\ \begin{array}{|c|ll} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{(-11)}=\stackrel{m}{ 4}(x-\stackrel{x_1}{(-3)}) \implies y +11 = 4 ( x +3) \\\\\\ y+11=4x+12\implies {\Large \begin{array}{llll} y=4x+1 \end{array}}\)
The equation is:
⇨ y + 11 = 4(x + 3)Work/explanation:
Recall that the point slope formula is \(\rm{y-y_1=m(x-x_1)}\),
where m is the slope and (x₁, y₁) is a point on the line.
Plug in the data:
\(\rm{y-(-11)=4(x-(-3)}\)
Simplify.
\(\rm{y+11=4(x+3)}\)
Hence, the point slope equation is y + 11 = 4(x + 3).Simplified to slope intercept:
\(\rm{y+11=4x+12}\)
\(\rm{y=4x+1}\) <- this is the simplified slope intercept equation
A square patio had the same number of feet added to its length and width. The
equation A(x) = x² + 12x + 36 represents the area of the new patio in square feet,
where a was the length and width of the original patio, in feet. How many feet were
added to the length and width of the patio?
Answers
The required feet added to both the length and width of the patio is 6 feet.
Given that,
A square patio had the same number of feet added to its length and width. The equation A(x) = x² + 12x + 36 represents the area of the new patio in square feet, where 'x' was the length and width of the original patio, in feet.
Here,
According to the question,
A(x) = x² + 12x + 36
A(x) = x² + 6x + 6x + 36
A(x) = (x + 6)(x + 6)
Length = width = x + 6
Thus, the required feet added to both the length and width of the patio is 6 feet.
Learn more about the area here:
https://brainly.com/question/27934373
#SPJ1
3.52 A coin is tossed twice. Let Z denote the number of heads on the first toss and W the total number of heads on the 2 tosses. If the coin is unbalanced and a head has a 40% chance of occurring, find (a) the joint probability distribution of W and Z; (b) the marginal distribution of W; (c) the marginal distribution of Z
Answers
Answer:
a) The joint probability distribution
P(0,0) = 0.36, P(1,0) = 0.24, P(2,0) = 0, P(0,1) = 0, P(1,1) = 0.24, P(2,1)= 0.16
b) P( W = 0 ) = 0.36, P(W = 1 ) = 0.48, P(W = 2 ) = 0.16
c) P ( z = 0 ) = 0.6
P ( z = 1 ) = 0.4
Step-by-step explanation:
Number of head on first toss = Z
Total Number of heads on 2 tosses = W
% of head occurring = 40%
% of tail occurring = 60%
P ( head ) = 2/5 , P( tail ) = 3/5
a) Determine the joint probability distribution of W and Z
P( W =0 |Z = 0 ) = 0.6 P( W = 0 | Z = 1 ) = 0
P( W = 1 | Z = 0 ) = 0.4 P( W = 1 | Z = 1 ) = 0.6
P( W = 1 | Z = 0 ) = 0 P( W = 2 | Z = 1 ) = 0.4
The joint probability distribution
P(0,0) = 0.36, P(1,0) = 0.24, P(2,0) = 0, P(0,1) = 0, P(1,1) = 0.24, P(2,1)= 0.16
B) Marginal distribution of W
P( W = 0 ) = 0.36, P(W = 1 ) = 0.48, P(W = 2 ) = 0.16
C) Marginal distribution of Z ( pmf of Z )
P ( z = 0 ) = 0.6
P ( z = 1 ) = 0.4
Part(a): The required joint probability of W and Z is ,
\(P(0,0)=0.36,P(1,0)=0.24,P(2,0)=0,P(0,1)=0,P(1,1)=0.24,\\\\P(2,1)=0.16\)
Part(b): The pmf (marginal distribution) of W is,
\(P(w=0)=0.36,P(w=1)=0.48,P(w=2)=0.16\)
Part(c): The pmf (marginal distribution) of Z is,
\(P(z=0)=0.6,P(z=1)=0.4\)
Part(a):
The joint distribution is,
\(P(w=0\z=0)=0.6,P(w=1|z=0)=0.4,P(w=2|z=0)=0\)
Also,
\(P(w=0\z=1)=0,P(w=1|z=1)=0.6,P(w=2|z=1)=0.4\)
Therefore,
\(P(0,0)=0.36,P(1,0)=0.24,P(2,0)=0,P(0,1)=0,P(1,1)=0.24,\\\\P(2,1)=0.16\)
Learn More: https://brainly.com/question/13127182
Find an equation for the line below.

Answers
Answer:
f(x) = -5/3x + 5/3
Step-by-step explanation:
if x = 4 ; f(x) = -5
if x = -2 ; f(x) = 5
f(x) = ax + b
-5 = 4a + b (1)
and
5 = -2a + b (2)
(1) + (2)
2a + 2b = 0
a = -b
(1) -5 = 4a - a
-5 = 3a
a = -5/3
Answer :
f(x) = -5/3x + 5/3
There are 1760 yards in one mile about how many miles will a runner have to run
Answers
Answer:
3
I used to be an olimpic runner and I ran the 400 all the time and I did cross country
tyler says that Figure B is a scaled copy of Figure A because all of the peaks are half as tall.
Answers
Answer:
No
Step-by-step explanation:
For the smaller figure to be a scaled copy, the figure would have to be half as wide as well,
The librarian purchased 22 copies of a best-selling book for $385.66. How much did The librarian purchased 22 copies of a best-selling book for $385.66.
How much did each copy of the book cost?each copy of the book cost?
Answers
Answer:
$17.53
Step-by-step explanation:
385.66 / 22 = 17.53
Domain????!!!!!!!!!!

Answers
Answer:
Interval Notation:
( −∞,−13]
Set-Builder Notation:
{ x| x≤−13}
Step-by-step explanation:
a) Reflection, then translationb) Rotation, then translationc) Reflection, then rotationd) Rotation, then reflection

Answers
We have the following:
Therefore we can conclude that from step 1 to step 2, it is a rotation because it moves on its own axis and from step 2 to 3, it is a reflection

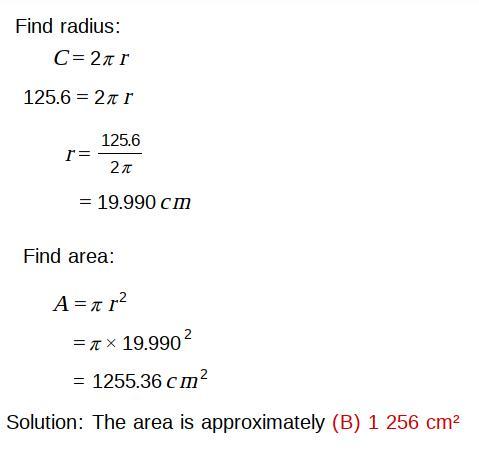
6. Meryll made a pancake with a circumference of 125.6 cm. What is its area? (A) 884 cm² (B) 1 256 cm² (C) 1 468 cm²
Answers
Answer:
(B) 1256 cm²---------------
Let the radius be r.
Use circumference formula and find the value of r:
C = 2πr125.6 = 2*3.14rr = 125.6/6.28r = 20Find the area using the circle area formula:
A = πr²A = 3.14*20²A = 1256The matching choice is (B).
Answer:
Step-by-step explanation:
At a factory that produces pistons for cars, Machine 1 produced 174 satisfactory pistons and 116 unsatisfactory pistons today. Machine 2 produced 180 satisfactory pistons and 20 unsatisfactory pistons today. Suppose that one piston from Machine 1 and one piston from Machine 2 are chosen at random from today's batch. What is the probability that the piston chosen from Machine 1 is satisfactory and the piston chosen from Machine 2 is unsatisfactory
Answers
Answer:
\(0.7\) is the probability that the piston chosen from Machine 1 is satisfactory and the piston chosen from Machine 2 is unsatisfactory
Step-by-step explanation:
The two events are independent of each other.
Hence the probability of choosing satisfactory piston from Machine 1 and unsatisfactory piston from Machine 2 is equal probability of choosing satisfactory piston from Machine 1 + probability of choosing unsatisfactory piston from Machine 2
Substituting the given values we get
Probability of choosing satisfactory piston from Machine 1 and unsatisfactory piston from Machine 2 =\(\frac{174}{174 +116} + \frac{20}{180 +20}\)
=\(\frac{174}{174 +116} + \frac{20}{180 +20}\)
=\(= \frac{174}{290} + \frac{20}{200}\\= 0.6 + 0.1\\= 0.7\)
100 POINTS HELPPPP What entry on a cash flow statement would indicate that a business had a cash inflow from the sale of equipment?
A. Negative CapEx
C. Negative repayment of debt
B. Inflow from sale of PPE
D. Positive issuance of equity
Answers
Answer:
The cash flow statement paints a picture as to how a company’s operations are running, where its money comes from, and how money is being spent. So i think D is the answer
Dewan’s bank account balance is -$16.75. He deposits checks totaling $23.59. What is his new balance? -$1.08
Answers
Answer:
$6.84
Step-by-step explanation:
This is quite a simple question, simply add the new deposited amount into the original balance to get your answer.
Original balance: -$16.75Deposit: $23.59New balance: -$16.75 + $23.59 = $6.84Write an equation in slope intercept form for the line with slope 4/3 and y -intercept -4
Answers
Answer:
y=4/3x-4
Step-by-step explanation:
For this problem, we will apply a slope-intercept form of the equation which is:
y=mx+b
where m is slope and b is the y-intercept
We are given that:
m=4/3
b=-4
Substitute these values into slope-intercept form of equation as:
y=4/3x-4
Question content area top
Part 1
You are certain to get a queen when selecting 49 cards from a shuffled deck. Express the indicated degree of likelihood as a probability value between 0 and 1 inclusive.
Answers
Answer:
Step-by-step explanation:
A standard deck of cards has 52 cards, including 4 queens. If you are certain to get a queen when selecting 49 cards from a shuffled deck, it means that one of those 49 cards must be a queen, and the remaining 48 cards can be any of the remaining 48 cards in the deck.
So, the probability of selecting a queen from a shuffled deck of cards is:
probability = number of favorable outcomes / total number of possible outcomes
Since we are certain to get a queen, the number of favorable outcomes is 4 (the number of queens in the deck), and the total number of possible outcomes is 49 (the number of cards selected from the deck).
Therefore, the probability of selecting a queen is:
probability = 4/49
This fraction cannot be simplified any further. Since the probability value is between 0 and 1 inclusive, we can express the indicated degree of likelihood as a probability value of approximately 0.0816.
In a recent survey of 36 people, 18 said that their favorite color of car was blue.
What percent of the people surveyed liked blue cars? Explain your answer with every step you took to get to it.
Answers
Answer: The percentage of people surveyed who liked blue cars is 50%.
Step-by-step explanation:
Total number of people partaking in survey= 36
number of people who like blue cars= 18
therefore, fraction of people who liked blue cars= \(\frac{18}{36}\)
hence, percentage of people who liked blue cars= (18/36)*100 %
= 50%
To learn more about percentages visit the link below:
https://brainly.com/question/30399396
Answer:
Percentage of people who like blue-coloured cars is 50%
Number of people who were surveyed=36
Number of people who like blue-colored cars=18
Therefore, Percentage of people who like blue cars= (Number of people who like blue cars/ Number of people who were surveyed)*100
=(18/36)*100
=50%
Read more about percentage
https://brainly.in/question/21062337#:~:text=Answer%3A,has%20no%20unit%20of%20measurement.
Find the Missing numbers:
250/20=25/?
Answers
250/20 divided by 10 is 25/2, so 2 would be the missing number.
what kind of line is this
can i have answer

Answers
Answer:
1. intersecting
2. paralle
3. perpendicular
4. intersecting
The box plots shown represent the average mileage of two different types of cars. Use the box plots to compare the data sets. Drag each value to show if it is greater for SUVs, greater for sedans, or if there is not enough information to tell.
Answers
Answer:
Parallel box and whisker plots are regular box and whisker plots, but drawn "one-above-the other" on the piece of paper. To enable to do this easily, draw an x-axis which is big enough for the largest value in the data, and small enough for the smallest value in the data (in the entire collection of data). Plot each box-and-whisker diagram below each other.
Step-by-step explanation:
pls mark
Answer:
Parallel box and whisker plots are regular box and whisker plots, but drawn "one-above-the other" on the piece of paper.
Step-by-step explanation:
Factorise 2y^2 + y + 6xy + 9x - 3
Answers
Answer: (2y+3)(3x+y-1)
Step-by-step explanation:
First regroup the terms.
6xy+9x+2y^2+y-3
Second factor 3x out of 6xy+9x
3x(2y)+9x+2y^2+y-3
3x(2y)+3x(3)+2y^2+y-3
And you get
3x(2y+3)+2y^2+y-3
Third factor by grouping
6 and 5 hundredths written in decimal
Answers
Answer:6.05
Step-by-step explanation:
6 is the ones place. 0 is the tenths place. 5 is the hundredths place.
please help! ASAP!
Find the perimeter of the figure below.

Answers
Answer:
30 ft
Step-by-step explanation:
The Pythagorean theorem can be used to find the length of the unknown side. It tells you the sum of the squares of the legs is equal to the square of the hypotenuse.
12² +a² = 13²
a² = 169 -144 = 25
a = √25 = 5
The sum of the side lengths is ...
(12 +5 +13) ft = 30 ft
The perimeter is 30 feet.
Ravi says,” if 10 years are added to my age, then my age becomes 25.”if Ravi’s present age is x years,
then find the linear equation satisfying the given condition.
Answers
Answer:
The linear equation satisfying the given condition is,
\(x=t+15\)
where x is Ravi's age and t is the number of years
(after 10 years (t=10) his age becomes 25)
Step-by-step explanation:
Let x be Ravi's age
Now, his current age is unknown, but if you add 10 to it, then it becomes 25, so
x + 10 =25
so, his current age is x = 25 =10
x = 15
Now, we find the linear equation satisfying the given condition,
Let t be the number of years that have passed since the present time
so, if 0 years have passed, his age will be 15 years
after 1 year, his age will be 16 years
after 10 years, his age will be 25 years and so on
so
\(x = t + 15\)
this satisfies the given condition, since if we add ten years i.e t = 10,
then we get 25
The equation is:
⇨ x + 10 = 25Work/explanation:
Here's the linear equation for Ravi's age.
Ravi's age is x.
If you add 10 to x you get x + 10.
That gives 25.
So the linear equation is x + 10 = 25.