Ron is seeking a loan wi th a simple in teres t ra te o f 7 % per year . he wan ts to borrow $4,500.

The amount of chlorine in the pool at the start of any given week, we need to know the amount of chlorine in the pool at the start of the previous week.

Let Cn be the amount of chlorine in the pool at the start of the nth week.

We can start by finding the amount of chlorine in the pool at the start of the second week. Since 40% of the chlorine evaporates, we have:

C2 = 0.6(C1 + 16)

Now, we can use this formula to find the amount of chlorine in the pool at the start of the third week:

C3 = 0.6(C2 + 16)

We can continue this process to find a recursive rule for Cn:

Cn = 0.6(Cn-1 + 16)

where C1 = 34.

This formula tells us that to find the amount of chlorine in the pool at the start of any given week, we need to know the amount of chlorine in the pool at the start of the previous week. We can use this formula repeatedly to find the amount of chlorine in the pool at the start of any week.

for such more question on  recursive rule

https://brainly.com/question/24871792

#SPJ11

Answer:

True

Step-by-step explanation:

Answer:

false

Step-by-step explanation:

the new image would increase in size not reduce

Answer:

Step-by-step explanation:

make a graph of what we need more of an explanation

Answer:

A. The points are collinear and coplanar

The probability of getting four of a kind in a hand of 7 cards is approximately 0.0001813.

To calculate the probability of getting four of a kind in a hand of 7 cards, we can break it down into two steps:

Step 1: Calculate the probability of getting four cards of the same value.

The first card can be any value, so the probability is 1. The second card must match the value of the first card, so the probability is 3/51 (there are 3 remaining cards of the same value out of the remaining 51 cards). The third and fourth cards must also match the same value, so the probabilities are 2/50 and 1/49, respectively.

Step 2: Calculate the probability of getting three different cards for the remaining three cards.

After getting four cards of the same value, there are 48 cards remaining. The first of the remaining three cards can be any value other than the four of a kind, so the probability is 48/48. The second card must be a different value than the first card, so the probability is 36/47. The third card must be different from the first two, so the probability is 24/46.

Multiplying the probabilities from Step 1 and Step 2 together, we get:

(1) × (3/51) × (2/50) × (1/49) × (48/48) × (36/47) × (24/46) = 0.0001813

To know more about  probability refer to-

https://brainly.com/question/31828911

#SPJ11

According to the information we can infer that the probability that the hand will contain four of a kind in the given card game is approximately 0.0015.

To calculate the probability of obtaining a four of a kind hand, we need to consider the number of ways to get a four of a kind hand divided by the total number of possible hands.

First, let's calculate the number of ways to get a four of a kind hand. We have 13 different card values (Ace, 2, 3, ..., 10, Jack, Queen, King), and for each value, we need to choose 4 cards out of the 4 available in the deck. So, there are 13 ways to choose the four cards of the same value.

Next, we need to calculate the number of ways to choose the remaining 3 cards with different values. We have 12 remaining card values (excluding the one used for the four of a kind), and for each value, we need to choose 1 card out of the 4 available. Therefore, there are 12 * 4 * 4 = 192 ways to choose the remaining 3 cards.

Now, let's calculate the total number of possible hands. In this card game, each player starts with a hand of 2 cards and then draws 5 more, so the total number of possible hands is given by the combination of 7 cards taken from a deck of 52 cards, which is denoted as C(52, 7) = 133,784,560.

Finally, we can calculate the probability by dividing the number of ways to get a four of a kind hand by the total number of possible hands:

So, we can conclude that the probability of obtaining a four of a kind hand in the given card game is approximately 0.0015, rounded to four decimal places.

Learn more about card games in: https://brainly.com/question/32185466
#SPJ4

The sphere has only one dimension

It is its radius

Then the rule of its volume is

The rule of its surface area is

Let the radius of the sphere is 21 cm

r = 21 cm

Substitute it in the rule of the volume to find it

Use pi = 22/7

Substitute r by 21 in the rule of the area to find it

The volume of the sphere which has a radius of 21 cm is 38808 cubic cm

The surface area of the sphere with same radius is 5544 square cm

The `working` variable is a control variable. We include this variable in the regression to control for the possibility that the relationship between wages and master's degree is due to the fact that people who work tend to earn higher wages than people who do not work.

To check in Stata what is the estimated effect on wages if a person engages in part-time one-year master degree studies, while working at the same time, you can use the following code:

reg wage master_degree working

The `reg` command is used to run a regression. In this case, we are regressing wages on the variable `master_degree`, which indicates whether or not the person has a master's degree, and the variable `working`, which indicates whether or not the person is working.

The `master_degree` variable is our independent variable, and the `wage` variable is our dependent variable. We are interested in the coefficient of the `master_degree` variable, which will tell us the estimated effect of having a master's degree on wages.

If the coefficient is positive, then we can conclude that having a master's degree is associated with higher wages. If the coefficient is negative, then we can conclude that having a master's degree is associated with lower wages.

If we find that the coefficient of the `master_degree` variable is still positive after controlling for the `working` variable, then we can be more confident that the relationship between wages and master's degree is causal.

to learn more about variable click here:

brainly.com/question/31118619

#SPJ11

Answer:

a) (8×10^-3)×(2×10^-4)= 16×10^-7= 1.6×10-6

b) (6×10^2)÷(3×10^-5)= 2×10^(2+5)= 2×10^7

Answer:

Step-by-step explanation:

Area of the rectangular garden is given by the expression,

Area = 6(2x + 5y)

By distributive property,

6(2x + 5y) = 12x + 30y

Therefore, equivalent expression for the area will be,

Area = (12x + 30y)

For x = 3 and y = 4,

Area = 12×(3) + 30×(4)

        = 36 + 120

        = 156

Area of the rectangular garden = 156 square feet.

To calculate the confidence interval for the average proportion of defective widgets, we use the sample data of 24 defective widgets out of a sample size of 425. With a 95% confidence level, we estimate that the average proportion of defective widgets is between 0.0271 and 0.0859.

To estimate the interval, we can use the formula for a confidence interval for a proportion:

Interval Estimate = Sample Proportion ± Margin of Error

where:

- Sample Proportion is the proportion of defective widgets in the sample (defective widgets / total sample size).

- Margin of Error accounts for the variability and is calculated as the critical value multiplied by the standard error.

Step 1: Calculate the Sample Proportion:

Sample Proportion = Defective Widgets / Total Sample Size

Sample Proportion = 24 / 425 ≈ 0.0565

Step 2: Calculate the Margin of Error:

To determine the margin of error, we need the critical value associated with the chosen confidence level. For a 95% confidence level, the critical value is approximately 1.96 (assuming a large sample size).

Margin of Error = Critical Value * Standard Error

Standard Error = sqrt((Sample Proportion * (1 - Sample Proportion)) / Sample Size)

Standard Error = sqrt((0.0565 * (1 - 0.0565)) / 425) ≈ 0.015

Margin of Error = 1.96 * 0.015 ≈ 0.0294

Step 3: Calculate the Confidence Interval:

Confidence Interval = Sample Proportion ± Margin of Error

Confidence Interval = 0.0565 ± 0.0294

Confidence Interval ≈ (0.0271, 0.0859)

Therefore, with a 95% confidence level, we estimate that the average proportion of defective widgets is between 0.0271 and 0.0859.

To know more about confidence interval, click here: brainly.com/question/32546207

#SPJ11

Answer:

Step-by-step explanation:

We can write an equation to represent this problem.

(15 + w)(20 + w) = 1/2 (15)(20)

Foil out the left side of the equation.

300 + 35w + w^2 = 150

Subtract 150 from both sides.

w^2 + 35w + 150    (I rearranged it to be in the form that is most efficient)

Now solve for variable w using the quadratic equation (look up the formula if necessary)

[-35 +_ (square root of the value of 1225-600)] / 2

[-35 +_ (SqRt of 625)] /2

(-35 +_ 25) /2

=  -30, -5

I just realized that it can't increase by a negative number, so I'm not really sure of the answer. Sorry, my bad. However, I feel that this may be of help to other people anyway, so I think I'll leave it up here. Apologies again.

Feel free to read through my reasoning behind my answer, and see if it gives you any ideas or inspiration for solving this problem.

Answer:

equation: y=-2x+2

a=-3

b=-8

Step-by-step explanation:

we need to find the equation of the line containing the point (3,-4) and has the slope (m) of -2

slope-intercept form is given as y=mx+b, where m is the slope and b is the y intercept

we can plug what we know into the equation:

y=-2x+b

we need to find b though

since the point will pass through the point (3,-4) we can subsitute it into the equation to find b

-4=-2(3)+b

-4=-6+b

2=b

the y intercept is 2

so the equation is y=-2x+2

the line also contains the points (a,8) and (5,b)

first, let's find a

a is the x value of the point

we know the value of y (8)

substitute y as 8 and solve for x

8=-2x+2

subtract

6=-2x

-3=x=a

so a is -3

now find b

b is the y value of the point

we know the x value (5)

substitute x as 5 and solve for y

y=-2(5)+2

y=-10+2

y=-8=b

so b is -8

hope this helps!

Answer:

60 x 0.90 = 54

54 questions correct.

Answer:

54

Step-by-step explanation:

90%= 0.9

0.9(60)=54

She answered 54 correctly.

Answer:

2 acres

Step-by-step explanation:

For 2 1/4 hours he mows 1/2 of an acre

I know that 1/4 of an hour is 15 minutes so I added 2 hours and 14 minutes 4 times to get 9 hours.

Now I know 2 1/4 of an hour times 4 equals 9 hours

1/2 an acre times 4 equals 2

He mows 2 acres

Answer:

g. 8\(\sqrt{2}\)

Step-by-step explanation:

since it's a 45 45 90 triangle, both the legs are the same (8) and the hypotenuse(y) would be 8 rad 2 :)

Answer:

\( 134-67 \times 45+89 = - 2792\)

Answer:

\(134 - 67 \times 45 + 89 =-2792 \)

Answer:

This gives us a square with an area of 225 square units.

Step-by-step explanation:

To find the dimensions of the rectangle with the largest area for a given perimeter of 60, we need to use the formula for the perimeter of a rectangle, which is P = 2l + 2w, where P is the perimeter, l is the length, and w is the width.

In this case, we know that P = 60, so we can write:

60 = 2l + 2w

Simplifying this equation, we get:

30 = l + w

To find the largest area of the rectangle, we need to maximize the product of the length and the width, which is the formula for the area of a rectangle, A = lw.

We can solve for one variable in terms of the other using the equation above. For example, we can write:

w = 30 - l

Substituting this expression for w into the formula for the area, we get:

A = l(30 - l)

Expanding and simplifying this expression, we get:

A = 30l - l^2

This is a quadratic equation in l, which has a maximum value when l is halfway between the roots. We can find the roots using the quadratic formula:

l = (-b ± sqrt(b^2 - 4ac)) / 2a

In this case, a = -1, b = 30, and c = 0, so we get:

l = (-30 ± sqrt(30^2 - 4(-1)(0))) / 2(-1)

Simplifying, we get:

l = (-30 ± sqrt(900)) / -2

l = (-30 ± 30) / -2

So the roots are l = 0 and l = 30. We want the smaller value first, so we take l = 0 and find w = 30. This would give us a rectangle with zero area, so it is not a valid solution.

The other root is l = 30, which gives us w = 0. Again, this is not a valid solution because we need both dimensions to be positive.

Therefore, the dimensions of the rectangle with the largest area for a perimeter of 60 are:

l = 15 and w = 15

This gives us a square with an area of 225 square units.

learn more about "Square area":-https://brainly.com/question/24487155

#SPJ11

The volume of oil exiting the pipe is approximately 100 cu in/hr, 2,400 cu in/day, and 1.39 cu ft/day when converting cu in/day to cubic feet per day.



To calculate the volume of oil exiting the pipe every hour, you would need to know the flow rate of the oil in cubic inches per hour. Let's assume the flow rate is 100 cubic inches per hour.To find the volume of oil exiting the pipe every day, you would multiply the flow rate by the number of hours in a day. There are 24 hours in a day, so the volume of oil exiting the pipe every day would be 100 cubic inches per hour multiplied by 24 hours, which equals 2,400 cubic inches per day.

To convert the volume from cubic inches per day to cubic feet per day, you would need to divide the volume in cubic inches by the number of cubic inches in a cubic foot. There are 1,728 cubic inches in a cubic foot. So, dividing 2,400 cubic inches per day by 1,728 cubic inches per cubic foot, we get approximately 1.39 cubic feet per day.

Therefore, the volume of oil exiting the pipe is approximately 100 cubic inches per hour, 2,400 cubic inches per day, and 1.39 cubic feet per day.

To learn more about volume click here

brainly.com/question/22907480

#SPJ11

The graph of exponential function, is described below.

What is an exponential function?

The mathematical formula f(x) = e^x stands for the exponential function. The term, unless specifically stated otherwise, generally refers to the positive-valued function of a real variable, though it can be extended to the complex numbers or generalized to other mathematical objects like matrices or Lie algebras.

The formula for an exponential growth function is y = ab^x, where a > 0 and b > 1. As can be seen in the example of f(x) = 2x below, the graph will ascend. The numbers get smaller as x gets closer to negative infinity, as you can see.

If b > 1 , then the graph's slope is positive and exponential growth is depicted. The value of y approaches infinity as x rises. The value of y approaches zero as x falls.

To know more about exponential function, click on the link

https://brainly.com/question/2456547

#SPJ4

Answer:

1

Step-by-step explanation:

The use of

Parenthesis(1+2)=3

Exponents- none

Multipy- 2 times the 3 is 6

Divide 6/6 is 1

Add- none

Subtract- none

Answer:

9

Step-by-step explanation:

you should have  search it up :)

HAVE A GREAT DAY

Answer:

-9

Step-by-step explanation:

C + D

-----------

C - D

= 8/11 + 10/11

- - - - - - - - - -

8/11 - 10/11

=18/11

- - - - -

- 2/11

=18/11 x 11/-2

= - 9

Answer:

The answer is -9

Step-by-step explanation:

8/11 + 10/11

8/11 - 10/11

18/11

-2/11

= -9

Answer:

26.3 feet

Step-by-step explanation:

We have to first find the length of the side of each tile.

The area of each tile is 19.2 \(ft^2\).

The area of a square is:

A = \(L^2\)

where L = length of side

Therefore:

\(19.2 = L^2\\\\L = \sqrt{19.2} = 4.38 feet\)

6 tiles will be lined end to end to construct the walkway, hence, the length of the walkway is:

6 * 4.38 ≅ 26.3 feet

The particular solution is:  \(y_{p(x)}\) = (-1/32) cos(4x) + (1/32) sin(4x)

and the general solution to the nonhomogeneous differential equation is:

\(y(x) = y_{c(x)} + y_{p(x)} = c_1 cos(4x) + c_2 sin(4x) - (1/32) cos(4x) + (1/32) sin(4x)\)

where c₁ and c₂  are constants determined by initial conditions.

What is the homogeneous differential equation?

A homogeneous differential equation is a differential equation in which all the terms can be expressed as a function of the dependent variable and its derivatives. In other words, a homogeneous differential equation can be written in the form:

F(x, y, y', y'', ..., yⁿ) = 0

To find a particular solution to the nonhomogeneous differential equation:

yⁿ + 16y = cos(4x) + sin(4x)

we can use the method of undetermined coefficients.

First, we find the complementary solution to the homogeneous differential equation:

yⁿ + 16y = 0

The characteristic equation is:

rⁿ + 16 = 0

which has roots:

r = ±4i

The complementary solution is:

\(y_{c(x)} = c_1 cos(4x) + c_2 sin(4x)\)

where c₁ and c₂ are constants determined by initial conditions.

Next, we find a particular solution \(y_{p(x)}\) to the nonhomogeneous differential equation using the following steps:

Find the general form of the nonhomogeneous term:

cos(4x) + sin(4x) = A cos(4x) + B sin(4x)

where A and B are constants to be determined.

Find the derivatives of the general form of \(y_{p(x)}\):

\(y_{p(x)}\)= A cos(4x) + B sin(4x)

\(y'_{p(x)}\)= -4A sin(4x) + 4B cos(4x)

\(y''_{p(x)}\) = -16A cos(4x) - 16B sin(4x)

Substitute the general form of  \(y_{p(x)}\) and its derivatives into the nonhomogeneous differential equation:

(-16A cos(4x) - 16B sin(4x)) + 16(A cos(4x) + B sin(4x)) = cos(4x) + sin(4x)

Simplifying, we get:

(16B - 16A) sin(4x) + (16A + 16B) cos(4x) = cos(4x) + sin(4x)

Since this equation must hold for all values of x, we equate the coefficients of sin(4x) and cos(4x) separately:

16B - 16A = 1

16A + 16B = 1

Solving for A and B, we get:

A = -1/32

B = 1/32

Therefore, the particular solution is:  \(y_{p(x)}\) = (-1/32) cos(4x) + (1/32) sin(4x)

and the general solution to the nonhomogeneous differential equation is:

\(y(x) = y_{c(x)} + y_{p(x)} = c_1 cos(4x) + c_2 sin(4x) - (1/32) cos(4x) + (1/32) sin(4x)\)

where c₁ and c₂  are constants determined by initial conditions.

To learn more about the homogeneous differential equation visit:

https://brainly.com/question/30331454

#SPJ4

Compete question:

Find a particular solution to the non-homogeneous differential equation yⁿ + 16y = cos(4x) + sin(4x)

Answer:

all 3 options are true : A, B, C

Step-by-step explanation:

warning : it has come to my attention that some testing systems have an incorrect answer stored as right answer for this problem.

they say that A and C are correct.

but I am going to show you that if A and C are correct, then also B must be correct.

therefore, my given answer above is the actual correct answer (no matter what the test systems say).

originally the information about the alignment of the point F in relation to point E was missing.

therefore, I considered both options :

1. F is on the same vertical line as E.

2. F is not on the same vertical line as E.

because of optical reasons (and the - incomplete - expected correct answers of A and C confirm that) I used the 1. assumption for the provided answer :

the vertical line of EF is like a mirror between the left and the right half of the picture.

A is mirrored across the vertical line resulting in B. and vice versa.

the same for C and D.

this leads to the effect that all 3 given congruence relationships are true.

if we consider assumption 2, none of the 3 answer options could be true.

but if the assumptions are true, then all 3 options have to be true.

now, for the "why" :

remember what congruence means :

both shapes, after turning and rotating, can be laid on top of each other, and nothing "sticks out", they are covering each other perfectly.

for that to be possible, both shapes must have the same basic structure (like number of sides and vertices), both shapes must have the same side lengths and also equally sized angles.

so, when EF is a mirror, then each side is an exact copy of the other, just left/right being turned.

therefore, yes absolutely, CAD is congruent with CBD. and ACB is congruent to ADB.

but do you notice something ?

both mentioned triangles on the left side contain the side AC, and both triangles in the right side contain the side BD.

now, if the triangles are congruent, that means that each of the 3 sides must have an equally long corresponding side in the other triangle.

therefore, AC must be equal to BD.

and that means that AC is congruent to BD.

because lines have no other congruent criteria - only the lengths must be identical.

Answer:

(x+7)(x+2)

Step-by-step explanation:

If we multiply it out we get:

x * x = x^2

7 * x = 7x

2 * x = 2x

7 * 2 = 14

Add it all, we get: x^2 +9x+14

Therefore these are the factors

These are the true sentences that can be constructed using the symbols:

Increasing function:

If for all x in the open interval containing x₁ and x₂ such that x₁ < x < x₂, then f(x₁) < f(x).

Decreasing function:

If for all x in the open interval containing x₁ and x₂ such that x₁ < x < x₂, then f(x₁) > f(x).

Constant function:

If for all x in the open interval containing x₁ and x₂, then f(x₁) = f(x).

These symbols can be used in constructing true sentences:

Increasing function:

x₁ < x₂ and f(x₁) < f(x₂)

x₁ < x₂ and f(x₁) ≤ f(x₂)

x₁ < x₂ and f(x) < f(x₂) for all x in the open interval containing x₁ and x₂

Decreasing function:

x₁ < x₂ and f(x₁) > f(x₂)

x₁ < x₂ and f(x₁) ≥ f(x₂)

x₁ < x₂ and f(x) > f(x₂) for all x in the open interval containing x₁ and x₂

Constant function:

f(x₁) = f(x₂)

f(x) = f(x₁) for all x in the open interval containing x₁ and x₂

Find out more on true sentences here: https://brainly.com/question/29090535

#SPJ1

Answer:

uh I don't even know what this is

Answer: x = 500

Step-by-step explanation:

Use the Pythagorean Theorem

a²+b²=c²

300² + 400² = x²

90000 + 160000 = x²

250000 = x²

√250000 = √x²

500 = x

hope i explained it :)

   - - - - - - -- - - - - - - - - - - - - - - - - - - --- -- - - - - - - - - - - - - - - -

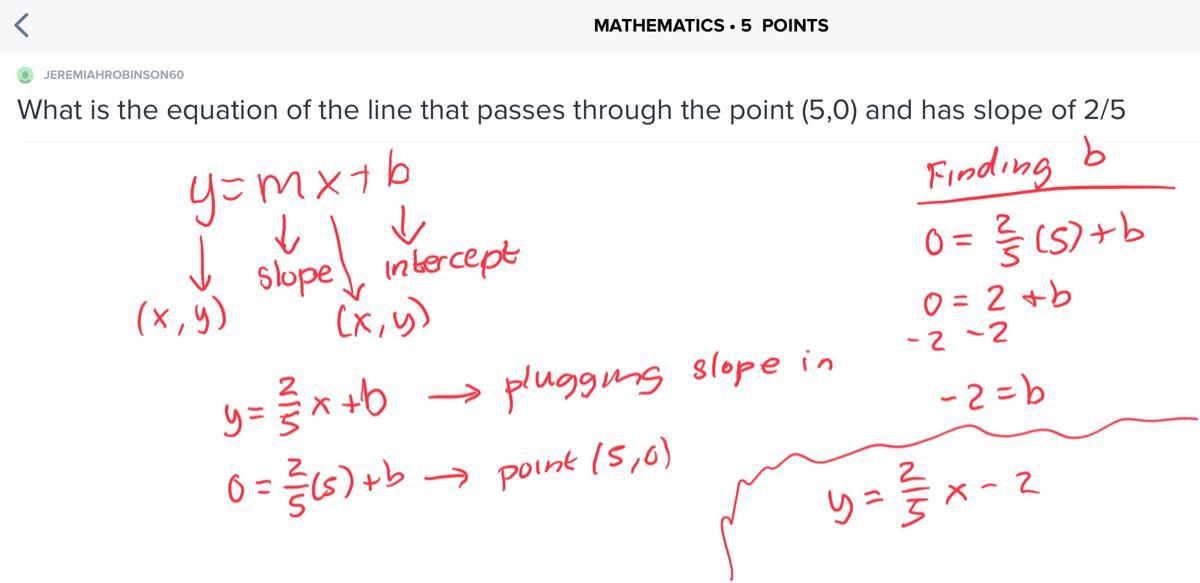
\(\blue\textsf{\textbf{Question:-}}}\)

What is the equation of the line passing through (5,0) with a slope of 2/5?

\(\blue\textsf{\textbf{Answer and Solution:-}}}\)

First let's write the equation in point-slope form:-

y-y1=m(x-x1)

Substitute the values:-

y-0=2/5(x-5)

Now convert to slope intercept:-

y-0=2/5x-2

Which simplifies to:-

y=2/5x-2

       - - - - - - - - - - - - - - - - - - - - -- -- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

1/3, 14/28, 4/7, 0.75 is least to greatest