Answers
Equation: 48=4x-12
Answer: x=15

Related Questions
find the equation of the tangent plane to f(x, y) = x2 − 2xy 3y2 having slope 2 in the positive x direction and slope 2 in the positive y direction.
Answers
The equation of the tangent plane to f(x, y) = x^2 - 2xy + 3y^2, with slopes 2 in the positive x direction and 2 in the positive y direction, is 2x + 4y - 8 = 0.
To locate the equation of the tangent airplane to the floor described with the aid of the feature f(x, y) = \(x^2 - 2xy + 3y^2\), we need to decide the gradient vector and consider it at a given point.
The gradient vector will grant the ordinary vector to the tangent plane, and by way of the use of the slope information, we can discover the equation of the plane.
Calculate the partial derivatives of the feature with recognize to x and y:
f_x = 2x - 2y
f_y = -2x + 6y
Set up a device of equations the use of the given slope information:
f_x = 2
f_y = 2
Solve the machine of equations to discover the factor where the slopes are satisfied:
2x - 2y = 2 --> x - y = 1 --> x = y + 1
-2x + 6y = 2 --> -x + 3y = 1 --> -x = 1 - 3y --> x = 3y - 1
Setting the two expressions for x equal to every other:
y + 1 = 3y - 1
2 = 2y
y = 1
Substitute y = 1 into both expression for x:
x = 1 + 1
x = 2
Therefore, the factor the place the slopes are comfy is (2, 1).
Evaluate the gradient vector at the factor (2, 1):
grad(f) = (f_x, f_y) = (2x - 2y, -2x + 6y)
= (2(2) - 2(1), -2(2) + 6(1))
= (2, 4)
The ordinary vector to the tangent airplane is the gradient vector (2, 4).
Using the point-normal structure of the equation for a plane, the equation of the tangent airplane is:
2(x - 2) + 4(y - 1) + d = 0
To decide the price of d, alternative the coordinates of the factor (2, 1):
2(2 - 2) + 4(1 - 1) + d = 0
0 + 0 + d = 0
d = 0
The equation of the tangent airplane is:
2(x - 2) + 4(y - 1) = 0
Simplifying the equation, we have:
2x - 4 + 4y - 4 = 0
2x + 4y - 8 = 8
For similar question on tangent plane:
https://brainly.com/question/31433124
#SPJ11
Solve 2x - 3 = 1
Solve for x
Answers
Answer:
x=2
Step-by-step explanation:
Add 3
2x=4
divide by 2
x =2
To solve this you need to get the x by itself. To do this we must move the -3 to the other side of the equation. To do this we add 3 to both sides. Now we have
2x = 4
The next step is to divide both sides by 2 so that the x is alone. 4/2 is 2 so
x=2
11/10= x+2/5 Please Explain
Answers
Answer:
x=7/10
Step-by-step explanation:
2/5=4/10
11/10=x+4/10
11/10-4/10=x
7/10=x
Answer:
x=7/10 or 0.7
Step-by-step explanation:
I turned the fractions into decimals
so
1.1=x+0.4
subtract 0.4 from 1.1 to get 0.7
Turn it into a fraction which is 7/10
a searchlight is shaped like a paraboloid of revolution. of the light source is located 2 feet from the base along the axis of symmetry and the depth of the searchlight is 4 feet, what would the width of the opening be?
Answers
The width of the opening is 2x, which equals 2(4√2) = 8√2 feet. To solve this problem, we'll use the properties of a paraboloid of revolution.
Given that the light source is located 2 feet from the base along the axis of symmetry and the depth of the searchlight is 4 feet, we have the vertex of the parabola at (0, 2). We also know the focus of the parabola is at the light source, which is at (0, 0).
Since the parabola opens downward, its equation is of the form (x - h)² = -4p(y - k), where (h, k) is the vertex and p is the distance between the vertex and the focus.
In our case, h = 0, k = 2, and p = 2. So the equation becomes x² = -4(2)(y - 2) or x² = -8(y - 2).
At the opening, the paraboloid is 4 feet deep, so y = -2. Substituting this value into the equation, we get:
x² = -8(-2 - 2) => x² = 32
Now, we find the width by solving for x:
x = ±√32 => x = ±4√2
Learn more about depth here:
https://brainly.com/question/13804949
#SPJ11
The width of the opening of the searchlight is 2√32 feet.
To find the width of the opening of the searchlight, we'll first determine the equation of the parabola and then use that to find the width.
Identify the vertex and focus:
The vertex is at the base of the searchlight (0, 0) and the focus is 2 feet from the base along the axis of symmetry,
so it's located at (0, 2).
Determine the equation of the parabola:
Since the parabola opens upward, its equation will be in the form \((x-h)^2 = 4p(y-k),\)
where (h, k) is the vertex and p is the distance from the vertex to the focus.
In this case, h = 0, k = 0, and p = 2,
so the equation is \(x^2 = 4(2)(y) or x^2 = 8y.\)
Find the width at the depth:
Since the depth of the searchlight is 4 feet, we'll find the width at y = 4. Substitute y = 4 in the equation:
\(x^2 = 8(4)\), which gives \(x^2 = 32\).
To find the width, we need the distance between the two points where x is positive and negative, so x = ±√32.
Calculate the width of the opening:
The width of the opening is the difference between the positive and negative values of x, which is 2√32.
For similar question on width.
https://brainly.com/question/19819849
#SPJ11
Need help solving this to help study for my final exam

Answers
Explanation
To answer this given question, we will make use of the Empirical rule of normal distribution
The Empirical Rule states that 99.7% of data observed following a normal distribution lies within 3 standard deviations of the mean. Under this rule, 68% of the data falls within one standard deviation, 95% percent within two standard deviations, and 99.7% within three standard deviations from the mean.
The image below helps gives a clearer understanding
So in our case, since the mean is 189 mg/dL and the standard deviation is 23
Then we will have the following values at
\(undefined\)
Carlisle Transport had $4,520 cash at the beginning of the period. During the period, the firm collected $1,654 in receivables, paid $1,961 to supplier, had credit sales of $6,916, and incurred cash expenses of $500. What was the cash balance at the end of the period?
Answers
To calculate the cash balance at the end of the period, we need to consider the cash inflows and outflows.
Starting cash balance: $4,520
Cash inflows: $1,654 (receivables collected)
Cash outflows: $1,961 (payments to suppliers) + $500 (cash expenses)
Total cash inflows: $1,654
Total cash outflows: $1,961 + $500 = $2,461
To calculate the cash balance at the end of the period, we subtract the total cash outflows from the starting cash balance and add the total cash inflows:
Cash balance at the end of the period = Starting cash balance + Total cash inflows - Total cash outflows
Cash balance at the end of the period = $4,520 + $1,654 - $2,461
Cash balance at the end of the period = $4,520 - $807
Cash balance at the end of the period = $3,713
Therefore, the cash balance at the end of the period is $3,713.
To learn more about cash : brainly.com/question/31754110
#SPJ11
Select all of the following that are true about the probability function for a t distribution? a. The t-statistic is the number of standard deviations away from the mean b. The area under thc cunve is 1 c. The t distribution ad standard normal curve look more alike when the random sample size is smaller (<5). d. It is symmetrical e. To calculate thc t-statistic vou need the population standard deviation
Answers
The option b "The area under the curve is 1." and the option d "It is symmetrical" are true about the probability function for a t distribution.
When the sample size is small and the population standard deviation is unknown, the t-distribution is used in statistics.
Option b confirms that the total area under the t-distribution curve is 1, indicating that the sum of probabilities for all possible outcomes is also equal to 1.
Option d confirms the symmetry of the t-distribution, implying that the curve is balanced on both sides of the mean. The mean, median, and mode of a symmetrical distribution are all equal.
To summarize, the t-distribution is a useful tool in statistics when working with small sample sizes because it accounts for the uncertainty in the population standard deviation.
To learn more about t distribution link is here
brainly.com/question/13574945
#SPJ4
Suppose if you have a lot of training data from an arbitrary distribution, would you expect the lda classifier to give similar boundaries to the bayes classifier?
Answers
No, the LDA classifier and the Bayes classifier would not necessarily give similar boundaries when trained on a large amount of training data from an arbitrary distribution.
The LDA (Linear Discriminant Analysis) classifier assumes that the input features are normally distributed and that the class covariances are equal. It finds linear boundaries that maximize the separation between classes based on these assumptions. On the other hand, the Bayes classifier makes decisions based on the class conditional probabilities and prior probabilities of the classes. It can handle arbitrary distributions and does not make assumptions about the class covariances. Therefore, even with a large amount of training data, if the distribution of the data does not conform to the assumptions of LDA (e.g., non-normal distributions or unequal class covariances), the LDA classifier may not give similar boundaries to the Bayes classifier.
The LDA and Bayes classifiers may not give similar boundaries when trained on data from an arbitrary distribution, as the LDA classifier relies on specific assumptions about the data distribution that may not hold true in practice.
To know more about arbitrary visit:
https://brainly.com/question/2500029
#SPJ11
Write the equation of the line in SLOPE-INTERCEPT form:
-5
-5
0
5
Slope:
Y-intercept:
Slope Intercept:

Answers
You plan to cut a board into 3 pieces to repair part of a railing. You are going to cut the two ends of the board off into two equal pieces that are 1.1 feet long, if the remaining piece needs to be 2.30 times longer than the each of the first two cuts what length board should you buy? Round to the nearest tenth.
Answers
Answer:
The board should be 4.7 feet long.
Step-by-step explanation:
To find the remaining piece, you just multiply 1.1 by 2.3 which is 2.53. That's the length of the remaining piece.
Then you add all the pieces together.
1.1+1.1+2.53 =
4.73
Round and add label.
4.7 feet
help meeeeeeeeeeeeeeeeeeeeeeeeeee pleaseeeeeeeeeeeeeeeeeeeeee!!!!!

Answers
5% of $1100 = 55
Year given = 7
So, 55 × 7 = 385
She would get after 7 years all total = (1100 + 385) = $1485
What is the value of b in this diagram?
A. 12
B. 10.2
C. 9
D. 8

Answers
Answer:
It is NOT c
Step-by-step explanation:
I clicked c and it was wrong lol.
Answer:
its D
Hope this helps ⊂◉‿◉つ
In the following diagram, points C, A, and B are collinear. Use complete sentences to describe the sum of CA (straight line above) and AB (straight line above)

Answers
Marco is investigating some of the business models of SureSpin, one of Faster Fidget's top competitors. He has learned that they model their cost of production for one type of spinner with the function C(x) = 13,450 + 1.28x, where x is the number of spinners produced. Interpret the model to complete the statement. Type the correct answer in each box. Use numerals instead of words. Based on the model, the fixed cost of production is $
Answers
Answer:
$13,450
Step-by-step explanation:
The fixed costs of production, are the costs incurred that are independent from the production volume, that is, regardless of how many spinners the company produces, those costs will remain the same. If 'x' is the number of spinners produced, interpreting the cost function, we can see that it costs $1.28 to produce each spinner and that there is a cost that does not rely on production of $13,450. Therefore, the fixed cost of production is $13,450.
PLSSS I NEED HELP
Which of the following is the graph ofY= -3/5x+2

Answers
Explanation - Since it’s a negative slope the line goes \ that way .
Answer:
It would be the last graph (c). It would look like the image below.
Explanation:
Since 3 is negative the answer would have a graph with a line going like this \.

Ik I said one more buh I assure u this is the last :p

Answers

Help asap please 40 points for help

Answers
Answer:
a. connect the point (0 , 3) with A
b. connect the origin (0 , 0) with B
c. For A: y = 0.5x +3
For B: y = 0.5x
Step-by-step explanation:
y = ax + b is the general rule for any straight line
a being the slope and b being the y intercept, a is given to be 0.5
y = 0.5x + b, substitute the coordinates of point A
4 = 0.5 *2 +b hence b = 4 - 0.5 *2 = 4 - 1 = 3
so y = 0.5 x + 3 is the equation of the line passing through A
since the second line that passes through B is parallel to the first, hence it has the same slope of 0.5
same procedure, substitute coordinates of B
2 = 0.5 * 4 + b hence b = 2 - 0.5 *4 = 2 - 2= 0
so y = 0.5 x is the equation of the line passing through B
The minimum cost for an Uber ride is $9. A fee of $1.50 will be added for each additional mile. Write a piece wise function to model the situation
Answers
Answer: 9 + 1.50m
Step-by-step explanation:
From the question, we are informed that the minimum cost for an Uber ride is $9 and that a fee of $1.50 will be added for each additional mile.
A piece wise function to model the situation will be:
= 9 + 1.50m
where m = every additional mile
For example, if a person takes 4 additional miles, this will be calculated as:
= 9 + 1.50m
= 9 + 1.50(4)
= 9 + 6
= $15
PLEASE READ! HELPFUL INFORMATION
Answers
Answer:
u kind of forgot the attachment bestie
Simplify , using the law of exponents

Answers
Answer:
option c (third one down)
Step-by-step explanation:
well its not a or d cause -10 times -3 is a positive number and then using law of exponents add the values of exponent and u get b as the only one that makes sense:)
Rashaad leans a 22-foot ladder against a wall so that it forms an angle of 65° with the ground. How high up the wall does the ladder reach?
Answers
20 feet
Explanation:

A quantity with an initial value of 8200 grows continuously at a rate of 0.55% per decade. what is the value of the quantity after 97 years, to the nearest hundredth?
Answers
The value of the quantity after 97 years, considering a growth rate of 0.55% per decade, to the nearest hundredth, is 8,649.28.
We have,
The continuous growth formula:
\(A = P e^{rt}\)
Where:
A is the final value of the quantity
P is the initial value of the quantity = 8200
r is the growth rate (as a decimal) = 0.55% = 0.55/100 = 0.0055
t is the time in years = 97 years
e is the mathematical constant approximately equal to 2.71828
Since a decade is a period of 10 years, divide the given time of 97 years by 10.
Number of decades = 97 years / 10 = 9.7 decades
Substitute.
\(A = P e^{rt}\\A = 8200 \times e^{0.0055 * 9.7}\)
\(A = 8200 \times e^{0.05335}\)
A = 8200 * 1.05479
A = 8649.278
A = 8649.28
Therefore,
The value of the quantity after 97 years, considering a growth rate of 0.55% per decade, to the nearest hundredth, is 8,649.28.
Learn more about equations here:
https://brainly.com/question/17194269
#SPJ12
Simplify (4.3)(4.3 ²)⁴
Answers
Answer:
\(4.3^{9}\)
Step-by-step explanation:
\((4.3^{1})\)\((4.3^{2})\)\((4.3^{2})\)\((4.3)^{2}\)\((4.3^{2})\) If I expand this out a little, it might be easier to see. If I do not have an exponent, it is understood that there is only one of those factors so the exponent is 1.
\((4.3^{2})^{4}\) means that we are taking\(4.3^{2}\) four times.
The Product of Power Principle tells us that when we are multiplying by same base we add the exponents.
\(4.3^{9}\) or 506953 Rounded to the nearest whole number
Ue Greatet Common Factor (GCF) and the Ditributive Property to write 3550 a a product
Answers
By using GCF and distributive Property, it is obtained that
3550 = 5 \(\times\) 710 as a product
What is Greatest Common Factor and distributive property?
Greatest Common factor (GCF) of two numbers a and b is the greatest number that divides both a and b.
Distributive property is the property over both addition and multiplication.
If a, b and c are three numbers, then distributive property is given by
\(a \times (b + c) = a \times b + a \times c\)
3550 = 3000 + 550
Now,
\(3000 = 2\times 2 \times 2\times 3\times 5 \times 5 \times 5\\550 = 2 \times 5 \times 5 \times 11\)
GCF of 3000 and 550 = 5
3550 = 5 \(\times\) 600 + 5 \(\times\) 110
3550 = 5 \(\times\) (600 + 110) [Distributive Property]
3550 = 5 \(\times\) 710
To learn more about GCF and distributive Property, refer to the link-
https://brainly.com/question/17879515
#SPJ4
Fatima is taking a test that is 45 minutes long.
She finishes the test at 10:50 a.m.
What time did she start the test?
Answers
Answer:10:05
Step-by-step explanation:
subtract 45 from 50!
Answer:
She started the test at 10:05 am
Step-by-step explanation:
Take the end time and subtract the time of the test
10:50-45 = 10:05
She started the test at 10:05 am
pls help me solve this question.... with working
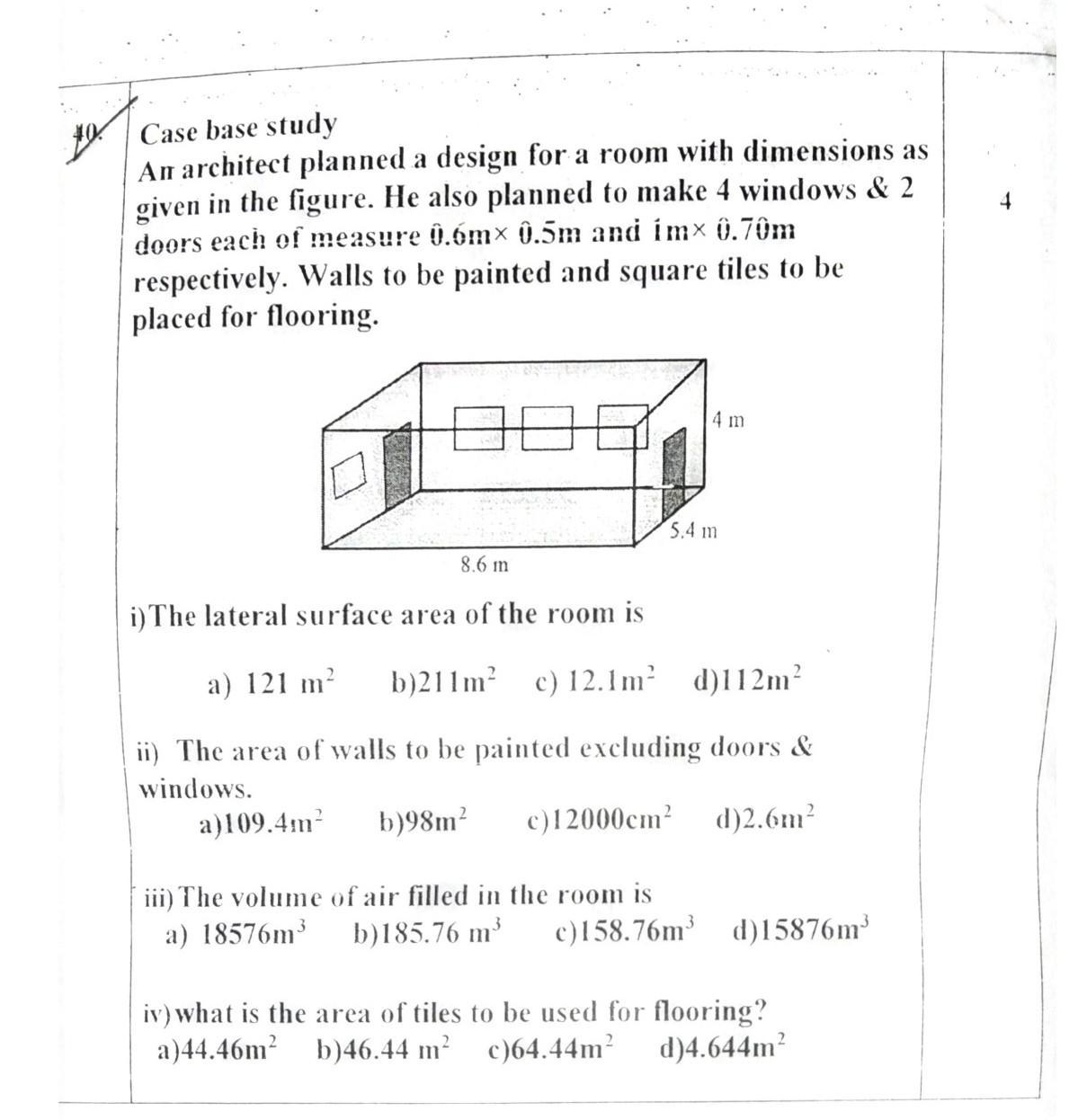
Answers
According to the information, the lateral area of the room is 112m²; the area to be painted is 109.4m²; the volume is 185.76m³ and the floor area is 46.44m².
How to calculate the lateral area of the room?To calculate the area of the lateral surface of the room we must find the area of all the sides and then add it as shown below:
5.4*4=21.6m²8.6*4=34.4m²21.6m² + 34.4m² = 56m²56m² * 2 = 112m²How do you find the surface to be painted (excluding doors and windows)?To find the area of the surface to be painted, excluding windows and doors, we must find the area of the doors and windows and subtract it from the total lateral area as shown below:
0.6*0.5=0.3m²0.3m² * 4 = 1.2m²0.6*0.70=0.42m²0.42m² * 2 = 0.84m²1.2m² + 0.84m² = 2.04m²112m² - 2.04m² = 109.96m²How to find the volume of the room?To find the volume of the room, we must multiply the height, by the length, by the width as shown below:
8.6*5.4*4=185.76m³How to find the surface area of the floor?To find the surface area of the floor we must multiply the length of the width by the length of the length as shown below:
8.6*5.4=46.44m²Learn more about area at: https://brainly.com/question/27683633
#SPJ1
what is 12 with the exponent of 2 multiplied by 6 with the exponent of 2?
Answers
Answer:
\( {12}^{2} \times {6}^{2} = {(12 \times 6)}^{2} = {72}^{2} \)
in 2010, haiti was hit by a devastating earthquake, which registered at 7.0
Answers
Answer:Richter scale measures intensity of earthquake as power of 10. The intensity of the Chilean earthquake( year 1960) was approx 316.23 times greater than the earthquake in Haiti (year 2010)How to measure the greatness of earthquakes from Richter scale's intensities?Suppose that the first measured earthquake was of intensity (on Richter scale)Suppose the second measured earthquake was of intensity(on Richter scale).It is a fact that each unit of the the Richter scale measurement increases as the power of 10.So if b = a + 1, then the second earthquake was 10 times greater than the first earthquake.If we have b = a + x, then we have:Second earthquake was times greater than the first earthquake.As it was given that the earthquake in Haiti was of 7.0 intensity on Richter scale, and that the Chilean earthquake was about 9.5 intensity on Richter scaleThe difference is 9.5 - 7 = 2.5
Step-by-step explanation:
If you sell $1000 worth of popcorn, then your Boy Scout dues are free. Ethan sold $1000 worth of popcorn. Conclusion: Ethan's Boy Scout dues are free.
This conclusion was draw using the law of contrapositive
This conclusion was draw using the law of detachment
This conclusion was draw using the law of syllogism
An invalid conclusion was made
Answers
two cities are 51 miles away from each other. how far would the cities be on a map that has a scale of 3 inches:17 miles?
Answers
The distance that would be between the cities on a map that has a scale of 3 inches:17 miles is 9 inches.
What is an expression?An expression is a way of writing a statement with more than two variables or numbers with operations such as addition, subtraction, multiplication, and division.
Example: 2 + 3x + 4y = 7 is an expression.
We have,
The two cities are 51 miles away from each other.
3 inches:17 miles
Now,
17 miles = 3 inches
Multiply 3 on both sides.
17 x 3 miles = 3 x 3 inches
51 miles = 9 inches
Thus,
There would be 9 inches between the cities on the map.
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ1
