Answers
Related Questions
Find the value of x.
A.5
B. 40
C. 45
D. 20

Answers
(B) x = 40
Step-by-step explanation:
4x + 20 = 180
4x = 160
x = 40
Answer:
B. 40
Step-by-step explanation:
This is a straight angle; this means that 20 and 4x are supplementary angles.
SUPPLEMENTARY ANGLES: TWO ANGLES WITH A SUM OF 180°.
\(20 + 4x = 180.\)
First, subtract 20 from 180 and from 20.
\(20 - 20 = 0\) (cancels itself out).
\(180 - 20 = 60.\)
Now we are left with 4x = 160.
Lastly, divide 160 from 4.
\(160/4 = 40.\)
Therefore, the value of x is 40.
Here's an attachment to make this process easier to understand.

integral of 1/sqrt(x^2 - a^2) dx
Answers
To solve the integral of 1/sqrt(x^2 - a^2) dx, we can use the substitution method. Let u = x^2 - a^2, then du/dx = 2x, and dx = du/2x.
Substituting into the integral, we get:
∫ 1/sqrt(x^2 - a^2) dx = ∫ 1/sqrt(u) * du/2x
= (1/2) ∫ 1/sqrt(u) du
= (1/2) * 2sqrt(u) + C
= sqrt(x^2 - a^2) + C
Therefore, the answer to the integral of 1/sqrt(x^2 - a^2) dx is sqrt(x^2 - a^2) + C, where C is the constant of integration.
In summary, the integral of 1/sqrt(x^2 - a^2) dx can be solved using the substitution method, where u = x^2 - a^2. The final answer is sqrt(x^2 - a^2) + C, where C is the constant of integration.
To know more about substitution method visit :-
https://brainly.com/question/12688945
#SPJ11
The integral of \(\frac{1}{\sqrt{x^2 - a^2}} dx\) is \(\ln\left|\frac{\sqrt{x^2 - a^2}}{a} + \frac{x}{a}\right| + C\), where C is the constant of integration
What is intergration?
Integration is a fundamental concept in calculus that involves finding the antiderivative or integral of a function. It is the reverse process of differentiation, which is concerned with finding the derivative of a function.
To find the integral of \(1/\sqrt(x^2 - a^2) dx\), we can use a trigonometric substitution. Let's substitute \(x = a sec(\theta)\), where \(sec(\theta)\) is the reciprocal of the cosine function.
By making this substitution, we can express dx in terms of \(d(\theta)\) as follows:
\(dx = a sec(\theta) tan(\theta) d(\theta)\)
Now, let's substitute these values into the integral:
\(\int \frac{1}{\sqrt{x^2 - a^2}} dx\\\\= \int \frac{1}{\sqrt{(a \sec(theta))^2 - a^2}} (a \sec(\theta) \tan(\theta)) d(\theta)\\\\= \int \frac{1}{\sqrt{a^2(\sec^2(theta) - 1)}} (a \sec(\theta) \tan(\theta)) d(\theta)\\\\= \int \frac{1}{\sqrt{a^2(\tan^2(theta))}} (a \sec(\theta) \tan(\theta)) d(\theta)\\\\= \int \frac{1}{a \tan(theta)} (a \sec(\theta) \tan(\theta)) d(\theta)\)
Simplifying the expression, we have:
\(= \int \sec(\theta) d(\theta)\)
The integral of \(sec(\theta)\) can be evaluated as the natural logarithm of the absolute value of \(sec(\theta)\) plus the tangent\((\theta)\):
\(= \ln|\sec(\theta) + \tan(\theta)| + C\)
Finally, substituting back \(x = a sec(\theta)\), we get:
\(= \ln|\sec(\theta) + \tan(\theta)| + C\\\\= \ln\left|\frac{\sqrt{x^2 - a^2}}{a} + \frac{x}{a}\right| + C\)
Therefore, the integral of \(\frac{1}{\sqrt{x^2 - a^2}} dx\) is \(\ln\left|\frac{\sqrt{x^2 - a^2}}{a} + \frac{x}{a}\right| + C\), where C is the constant of integration
To learn more about integration visit:
https://brainly.com/question/27419605
#SPJ4
The dimensions of a rectangular prism are shown in the table.
Length: 10 in
Width: 12 in
Height: 16 in
What is the surface area of the rectangular prism?
Answers
Explanation: The formula for the surface area of a rectangular prism is 2(WL+HL+HW) where W=width, L=length, and H=height. Since all the dimensions are given, you simply need to substitute each value into the formula. Thus, the formula becomes: 2(12*10+16*10+16*12)
=2(120+160+192)
=2*472
=944 (solve using the order of operations)
Hope this helps シ

Sydney used 14 gallons and 4 cups of paint to repaint his house. how many cups of paint did Sydney use?
Answers
Answer:
228 cups were used
Step-by-step explanation:
16 cups = 1 gallon
16 (1 gallon) x 14 (as the question provides) = 224
Add the last 4 cups
= 228
228 cups were used
Please help
Simplify -1/2(b+2)+3b
(A) 5/2b-1
(B) 7/2b+1
(C) 5/2b+2
Answers
The expression is simplified to 5/2b - 1. Option A
What are algebraic expressions?Algebraic expressions are expressions that comprise of variables, terms, coefficients, constants and factors.
They may also contain arithmetic operations such as addition, subtraction, multiplication, division, bracket, etc.
Given the expression;
-1/2(b+2)+3b
expand the bracket
-b - 2/2 + 3b
Find the lowest common multiple
-b - 2 + 6b /2
Add the like terms
5b - 2/2
5b/2 - 2/2
5/2b - 1
Thus, the expression is simplified to 5/2b - 1. Option A
Learn more about algebraic expressions here:
https://brainly.com/question/4344214
#SPJ1
what’s the median of this set? 67,68,69,69,69,71,71,72,73,75
a)69
b) 69.5
c) 70
d) 70.5
e) 71
Answers
Answer:
c) 70
Step-by-step explanation:
the median is the middle number in the set. since there are 2 in the middle (69 and 71) and them together and then divide by 2 to find your median. there are 2 in the middle because there is an even amount of numbers in the set.
The median of the given data set is 70. Therefore, option C is the correct answer.
What is median of data?The median is the value in the middle of a data set, meaning that 50% of data points have a value smaller or equal to the median and 50% of data points have a value higher or equal to the median. For a small data set, you first count the number of data points (n) and arrange the data points in increasing order.
The given data set is 67,68,69,69,69,71,71,72,73,75.
There are 10 observation in the given data set.
Here 5th and 6th terms are in middle.
So, average of 69 and 71 is 69+71/2
= 70
Therefore, option C is the correct answer.
Learn more about the median here:
https://brainly.com/question/28060453.
#SPJ2
9 identical candies cost 11 dollars with some pennies, and 13 of the same candies cost 15 dollars with pennies. How much does one candy cost?
Answers
One candy costs approximately 1.22 dollars.
To find how much does one candy cost
Let's assume the cost of one candy is x dollars.
The information provided indicates that nine candies cost eleven dollars, which is stated as nine times eleven.
Similar to how 13 sweets cost 15 dollars, 13x = 15 can be used to illustrate this.
To find the cost of one candy, we need to solve for x in either of the equations. Let's solve the first equation:
9x = 11
Dividing both sides of the equation by 9:
x = 11/9
Therefore, one candy costs approximately 1.22 dollars (rounded to two decimal places).
Learn more about equation here : brainly.com/question/2972832
#SPJ1
diced turnip in a particular restaurant has a yield percentage of 88%. if a cook begins with 38 ounces of turnips, how many ounces of diced turnips should she expect to yield after processing?
Answers
The cook should expect to yield 33.44 ounces of diced turnips after processing if a cook begins with 38 ounces of turnips with yield percentage of 88%.
When the cook begins with 38 ounces of turnips, she should expect a yield of 88% for diced turnips. This means that after processing, 88% of the original weight of turnips will remain as diced turnips.
To calculate the expected yield of diced turnips, we can use the formula
Expected yield = Original weight of turnips x Yield percentage / 100
Plugging in the values given, we get:
Expected yield = 38 ounces x 88 / 100
Expected yield = 33.44 ounces
Therefore, the cook should expect to yield 33.44 ounces of diced turnips after processing. This calculation assumes that the yield percentage is accurate and that the processing of turnips is consistent. By using the expected yield, the cook can estimate the amount of diced turnips that can be prepared for a dish, ensuring that there is enough supply for customers while minimizing waste.
To know more about yield percentage here
https://brainly.com/question/14040107
#SPJ4
In this problem, use properties of definite integrals. 5 If [x² dx = -625 and "x³ dx = 625 what does 4 4 Enter an exact number. Need Help? Read It Watch It ********* 5 [²x³ -5 dx equal?
Answers
The answer is -1250. This can be found using the properties of definite integrals, specifically the property that the integral of a function is equal to the negative of the integral of the function's negative.
The first step is to find the integral of x² dx. This is equal to -625, as given in the problem. The next step is to find the integral of x³ dx. This is equal to 625, as also given in the problem. Now, we can use the property of definite integrals to find the integral of ²x³ - 5 dx. This is equal to -625 - (-5) = -1250.
The reason for this is that the integral of a function is equal to the negative of the integral of the function's negative. In this case, the function is ²x³ - 5. The negative of this function is -²x³ + 5. The integral of ²x³ - 5 is equal to -625. The integral of -²x³ + 5 is equal to 5. Therefore, the integral of ²x³ - 5 dx is equal to -625 - (-5) = -1250.
To learn more about definite integrals click here : brainly.com/question/32963975
#SPJ11
Which pairs of events describe independent events? Select all that apply.
O Rolling a six-sided die and having it land on a 4, and then choosing a blue marble from a jar of marbles.
O Rolling a six-sided die and having it land on a 3,
and then rolling a six-sided die and having it land on a 2.
O Choosing a blue marble from a jar of marbles, not replacing it, and then drawing a red marble.
O Entering a raffle 3 times and winning one prize, and then winning another prize in the same raffle.
O Drawing a 3 from a deck of cards, not replacing it, and then drawing a 4 from the same deck of cards.
Answers
When one event's occurrence or non-occurrence doesn't affect the occurrence or non-occurrence of another event, then such events are called independent events. The correct options are A and B.
Which pair of events are called independent events?When one event's occurrence or non-occurrence doesn't affect the occurrence or non-occurrence of another event, then such events are called independent events.
Symbolically, we have:
Two events A and B are said to be independent iff we have:
\(P(A \cap B) = P(A)P(B)\)
Comparing it with chain rule will give
\(P(A|B) = P(A)\\P(B|A) = P(B)\)
Thus, showing that whether one occurred or not, the other one doesn't care about it (independence).
Two events are known to be independent even if the result of one event does not affect the result of the second event.
Rolling a dice in an independent event and choosing a blue marble is another independent event. The two events are independent and the result of one does not affect the other.
Thus, A is correct.
Rolling a dice in an independent event, and rolling it again won't affect the result of the second roll. Therefore, rolling a dice twice or for n number of times is an independent event.
Thus, B is correct.
Hence, the correct options are A and B.
Learn more about Independent Events:
brainly.com/question/1210781
#SPJ1
Can anyone give me the answers and like a better explanation than my teacher

Answers
The Area of shaded regions is shown below.
1. Area of shaded region
= Area of rectangle - Area of Triangle
= 9 x 7 - 1/2 x 7 x 6
= 63 - 21
= 42
2. Area of shaded region
= Area of rectangle - Area of semicircle
= 12 - 6.28
= 5.72
3. Area of shaded region
= 6 x 7 + 2x 5
= 42 + 10
= 52
4. Area of shaded region
= 43 x 30 - 2 (3.14 x 10 x 10)
= 1290 - 628
= 662
5. Area of shaded region
= 12 x 8 + 3.14 x 8 x 8 /2
= 96 + 100.48
= 196.48
6. Area of shaded region
= 8 x 10 + 2 x 4 x 2
= 80 + 16
= 96
7. Area of shaded region
= 1/2 x 24 x 24 - 18 x 6
= 288 - 108
= 180
Learn more about Area here:
https://brainly.com/question/1631786
#SPJ1
another one! please help ..

Answers
Answer:
n has to be negative
Step-by-step explanation:
To solve this equation, 15 has to be subtracted from 2 which is negative
Since n is positive then the overall result will be negative
The owner of a hotel is installing a patio around three sides of her pool as shown. 2p2 + 4p - 6 patio p2 - 3p + 2 3p 5 pool Which statements are true about the expression representing the area of the patio? The expression representing the area of the patio has a constant term. The expression representing the area of the patio is a trinomial. The expression representing the area of the patio has four terms. The expression representing the area of the patio is a polynomial. The expression representing the area of the patio is cubic.

Answers
Answer:
-The expression representing the area of the patio has four terms.
-The expression representing the area of the patio is cubic.
-The expression representing the area of the patio has a constant term.
wrong answer:
The expression representing the area of the patio is a trinomial.
Answer:
Step-by-step explanation:

Use the pH formula, pH = -log[H+] to answer the following: a) Determine the pH level of a substance which has a hydronium ion concentration of 5.3 x 10-¹¹ mol/L. [2 marks A3] b) The pH of a substance can range between 3 and 4.7 Determine the range of the hydronium ion concentration in the substance. [4 marks A3] c) What is the average rate of change of the hydronium ion level with respect to the pH level when the pH of the substance changes from 3 to 4.7? The units for your answer should be lons level/ PH level [3 marks D1]
Answers
c) the average rate of change of the hydronium ion level with respect to the pH level when the pH changes from 3 to 4.7 is approximately -1.1735 x 10^(-3) mol/L per pH level.
a) To determine the pH level of a substance with a hydronium ion concentration of 5.3 x 10^(-11) mol/L, we can use the pH formula:
pH = -log[H+]
In this case, [H+] = 5.3 x 10^(-11) mol/L. Plugging this value into the formula:
pH = -log(5.3 x \(10^{(-11)}\))
Calculating the logarithm:
pH ≈ -(-10.28)
pH ≈ 10.28
Therefore, the pH level of the substance is approximately 10.28.
b) The pH range of a substance is given as 3 to 4.7. We can determine the range of the hydronium ion concentration by using the inverse of the pH formula:
[H+] = \(10^{(-pH)}\)
For the lower pH value (pH = 3):
[H+] = 10^(-3) = 1 x\(10^{(-3)}\) mol/L
For the upper pH value (pH = 4.7):
[H+] = \(10^{(-4.7) }\)
≈ 1.995 x 10^(-5) mol/L
Therefore, the range of the hydronium ion concentration in the substance is approximately 1 x \(10^{(-3)}\) mol/L to 1.995 x \(10^{(-5)}\) mol/L.
c) The average rate of change of the hydronium ion level with respect to the pH level can be calculated by finding the difference in hydronium ion concentration divided by the difference in pH level.
Δ[H+] = [H+]₂ - [H+]₁
ΔpH = pH₂ - pH₁
Using the values from part b:
Δ[H+] = (1.995 x \(10^{(-5)}\) - 1 x \(10^{(-3)}\)) mol/L
ΔpH = 4.7 - 3
Calculating the average rate of change:
Average rate of change = Δ[H+] / ΔpH
Substituting the values:
Average rate of change ≈ (-1.996 x \(10^{(-3)}\)) mol/L / (1.7)
To know more about inverse visit:
brainly.com/question/30339780
#SPJ11
the accompanying dataset provides data on monthly unemployment rates for a certain region over four years. compare​ 3- and​ 12-month moving average forecasts using the mad criterion. which of the two models yields better​ results? explain.
Answers
To compare the 3-month and 12-month moving average forecasts using the mean absolute deviation (MAD) criterion, we need to calculate the MAD for each model and then compare them. The MAD is a measure of the average magnitude of the forecast errors, and a lower MAD indicates a better forecast.
To calculate the MAD for the 3-month moving average model, we need to first calculate the forecasted values for each month by taking the average of the unemployment rates for the previous 3 months. For example, the forecasted value for April 2018 would be the average of the unemployment rates for January, February, and March 2018. We then calculate the absolute deviation between the forecasted value and the actual value for each month, and take the average of those deviations to get the MAD for the 3-month moving average model.
We can repeat this process for the 12-month moving average model, but instead of taking the average of the previous 3 months, we take the average of the previous 12 months.
Once we have calculated the MAD for both models, we can compare them to determine which model yields better results. Generally, a lower MAD indicates a better forecast. However, it is important to note that the MAD criterion only considers the magnitude of the forecast errors and does not take into account the direction of the errors (i.e., overestimation versus underestimation).
Learn more about MAD,
https://brainly.com/question/29111637
#SPJ4
Full Question ;
The accompanying dataset provides data on monthly unemployment rates for a certain region over four years. Compare 3- and 12-month moving average forecasts using the MAD criterion. Which of the two models yields better results? Explain. Click the icon to view the unemployment rate data. Find the MAD for the 3-month moving average forecast. MAD = (Type an integer or decimal rounded to three decimal places as needed.) A1 fx Year D E F G H I 1 2 3 1 с Rate(%) 7.8 8.3 8.5 8.9 9.4 9.6 9.4 9.5 9.7 9.9 9.8 10.1 9.9 9.7 9.8 9.91 9.7 9.4 9.6 9.4 9.3 9.5 9.9 9.5 9.2 9.1 8.9 A B Year Month 2013 Jan 2013 Feb 2013 Mar 2013 Apr 2013 May 2013 Jun 2013 Jul 2013 Aug 2013 Sep 2013 Oct 2013 Nov 2013 Dec 2014 Jan 2014 Feb 2014 Mar 2014 Apr 2014 May 2014 Jun 2014 Jul 2014 Aug 2014 Sep 2014 Oct 2014 Nov 2014 Dec 2015 Jan 2015 Feb 2015 Mar 2015 Apr 2015 May 2015 Jun 2015 Jul 2015 Aug 2015 Sep 2015 Oct 5 7 3 ) 1 2 3 1 5 7 9.1 ) 9. 1 2 3 1 5 7 ) 9.1 8.9 8.9 8.9 8.9 8.7 8.4 8.3 8.3 8.4 8.1 8.1 8.4 8.2 8.3 7.7 7.9 7.9 7.8 1 2 2015 Dec 2016 Jan 2016 Feb 2016 Mar 2016 Apr 2016 May 2016 Jun 2016 Jul 2016 Aug 2016 Sep 2016 Oct 2016 Nov 2016 Dec 3 1 5 3 2 2
what are the multipils of 17
Answers
Answer:
17, 34, 51, 68, 85, 102, 119, 136, 153, 170
hope this helps
Answer:
The multiples of 17 are: 17, 34, 51, 68, 85, 102, 119, 136, 153, 170, 187, 204, 221, 238, 255, 272, 289, 306, 323, 340, 357, 374, 391, 408, 425, 442, 459, 476, 493, 510, 527, 544, 561, 578, 595, 612, 629, 646, 663, 680, 697, 714, 731, 748, 765, 782, 799, 816, 833, 850, 867, 884, 901, 918, 935, 952, 969, 986.
Step-by-step explanation:
Easiest way to solve this is to probably grab a piece of paper and just add 17 until you find whatever you’re looking for!
Suppose John takes the shuttle van directly from Berkeley to the airport, and Jane drives from Berkeley and takes the parking shuttle. They leave at the same time, and their travel times are independent. The chance that John gets to the airport before Jane is
Answers
There is a 50-50 chance that John gets to the airport before Jane. Therefore, the chance that John gets to the airport before Jane is 1/2.
Suppose John takes the shuttle van directly from Berkeley to the airport, and Jane drives from Berkeley and takes the parking shuttle. They leave at the same time, and their travel times are independent. The chance that John gets to the airport before Jane is 1/2.Solution:
Since the travel times of John and Jane are independent and given that they leave at the same time, the chance that John gets to the airport before Jane is equal to the probability that Jane arrives at the airport after John.
There are two possible outcomes, either John arrives first or Jane arrives first. Both of these outcomes are equally likely.
Since the probability of two mutually exclusive events happening is equal to the sum of their probabilities, and we know that the probability of both John and Jane arriving at the same time is 0, the probability that John gets to the airport before Jane is:1/2
Learn more about probability:
https://brainly.com/question/29204361
#SPJ11
The difference between two numbers is40. Four times the smaller is equal to5 more than the larger. What are the numbers?
Answers
Those numbers are x=55 and y= 15.
---------
1) Gathering the data
x-y=40
4y=x+5
2) Rewriting the system, and then solving it
x-y=40 ⇒x=40+y
4y=x+5
Applying substitution in the 2nd equation
4y=(40+y)+5
4y =40+y+5 Subtracting y from both sides
4y-y= 40+y-y +5
3y=40+5
3y= 45 Dividing by 3
y=15
Replacing in the first equation
x-15=40 Adding 15 to both sides
x-15+15=40+15
x=55
3) Those numbers are x=55 and y= 15.
Jaidyn’s family drinks a total of 10 gallons of milk every 5 weeks . How many gallons of milk does the family drink per week? How many weeks does it take the family to consume one gallon of milk?
Answers
Answer:
2 gallons of milk per week
0.35 weeks(3.5 days) to consume 1 gallon
Step-by-step explanation:
Jaidyn's family drinks 10 gallons of milk every 5 weeks, which is a ratio of
10:5
To find how many gallons of milk they drink per week, you'd have to divide 10/5
10 ÷ 5 = 2
Considering that 1 week is 7 days, it would take them 0.35 weeks to consume 1 gallon of milk, which is the same as 3 and a half days.
b) If the joint probability distribution of three discrete random variables X, Y, and Z is given by, f(x, y, z)=. (x+y)z 63 for x = 1,2; y=1,2,3; z = 1,2 find P(X=2, Y + Z ≤3).
Answers
The probability P(X=2, Y+Z ≤ 3) is 13. Random variables are variables in probability theory that represent the outcomes of a random experiment or event.
To find the probability P(X=2, Y+Z ≤ 3), we need to sum up the joint probabilities of all possible combinations of X=2, Y, and Z that satisfy the condition Y+Z ≤ 3.
Step 1: List all the possible combinations of X=2, Y, and Z that satisfy Y+Z ≤ 3:
X=2, Y=1, Z=1
X=2, Y=1, Z=2
X=2, Y=2, Z=1
Step 2: Calculate the joint probability for each combination:
For X=2, Y=1, Z=1:
f(2, 1, 1) = (2+1) * 1 = 3
For X=2, Y=1, Z=2:
f(2, 1, 2) = (2+1) * 2 = 6
For X=2, Y=2, Z=1:
f(2, 2, 1) = (2+2) * 1 = 4
Step 3: Sum up the joint probabilities:
P(X=2, Y+Z ≤ 3) = f(2, 1, 1) + f(2, 1, 2) + f(2, 2, 1) = 3 + 6 + 4 = 13
They assign numerical values to the possible outcomes of an experiment, allowing us to analyze and quantify the probabilities associated with different outcomes.
Learn more about random variables here:
https://brainly.com/question/32245509
#SPJ11
according to government data, 22% of american children under the age of six live in households with incomes less than the official poverty level. a study of learning in early childhood chooses an srs of 300 children. find the probability that more than 20% of the sample are from poverty households. be sure to check that you can use the normal approximation.
Answers
The probability that more than 20% of the sample are from poverty households is approximately 0.8365.
What is the probability?
Probability is simply how likely something is to happen. Whenever we're unsure about the outcome of an event, we can talk about the probabilities of certain outcomes—how likely they are. The analysis of events governed by probability is called statistics.
We can use the normal approximation to the binomial distribution to solve this problem, given that the sample size is relatively large (n=300) and the probability of success (p=0.22) is not too close to 0 or 1.
Let X be the number of children in the sample who live in poverty households. Then X follows a binomial distribution with parameters n=300 and p=0.22.
The mean of X is given by μ = np = 300 x 0.22 = 66, and the standard deviation is σ = sqrt(np(1-p)) = sqrt(300 x 0.22 x 0.78) ≈ 6.23.
We want to find the probability that more than 20% of the sample are from poverty households, which is equivalent to finding P(X > 0.2n) = P(X > 60).
To use the normal approximation, we can standardize X as follows:
Z = (X - μ) / σ
Then, we have:
P(X > 60) = P(Z > (60 - 66) / 6.23) ≈ P(Z > -0.96)
Using a standard normal table or calculator, we can find that the probability of Z being greater than -0.96 is approximately 0.8365.
Therefore, the probability that more than 20% of the sample are from poverty households is approximately 0.8365.
To learn more about the probability visit:
https://brainly.com/question/13604758
#SPJ1
HELP I WILL GIVE BRAINLIEST!

Answers
Answer:
I think it's 41°
Step-by-step explanation:
Because the angle represents Tan, by using the inverse of tan and the formula of Opp/Adj, the answer will be 41.08 Which is 41°
I hope This helps! and make me brainliest as you said, PLEASE..
What is the value of b?

Answers
1. Turn this standard notation 25,000,000 into scientific notation.
Answers
Answer:
25,000,000 = 2.5 × 10^7
The ^ is raised to the power.
Hope this helps :)
Step-by-step explanation:
2.5 × 10^7 = 25,000,000
Write an equation with integer coefficients that has a zero at 3 with a multiplicity of 2,a zero at - 5/4.
Answers
We're asked to develop a polynomial
Integer coefficient that has zero at 3 is (x-3)
Multiplicity of 2 gives (x - 3) (x - 3)
And a zero at -5/4 gives (x + 5/4)
Put together, we have: (x - 3) (x - 3)(x + 5/4) = (x - 3)^2 (x + 5/4) = 0
Can anyone solve this correctly?

Answers
Answer:
J. x = 1
Step-by-step explanation:
Write an equation based on the image of the scales.
3x + 6 = 9
Subtract 6.
3x = 3
Divide by 3.
x = 1
If you haven't learned to solve equations yet, the same thought process can be used with the scale image. The scales are balanced. To keep them balanced, remove 6 of the pieces marked "1" from BOTH sides of the scale. Then the scale will have three x's on one side and three "1"'s on the other side. So each x has to be one "1".
URGENT!! HOMEWORK DUE TONIGHT!!
A dilation of a linear function will have a slope that is steeper or less steep than the slope of a parent function.
True or False
Answers
Step-by-step explanation: Stretches and compression change the slope of a linear function.
If the line becomes steeper, the function has been stretched vertically or compressed horizontally.
If the line becomes flatter, the function has been stretched horizontally or compressed vertically.
Horizontal Stretch/Compression by a factor of b
f(x)→ f(1• f(x→f(1 bx) • b > 1 stretches away from the y-axis.
• 0 < |b| < 1 compresses toward the y-axis.
• y-intercepts remain the same.
Vertical Stretch/Compression by a factor of a
• f(x) → a f(x) • a > 1 stretches away from the x-axis.
• 0 < |a| < 1 compresses toward the x-axis.
• x-intercepts remain the same.Stretches and compression's change the slope of a linear function.
If the line becomes steeper, the function has been stretched vertically or compressed horizontally.
If the line becomes flatter, the function has been stretched horizontally or compressed vertically.
Horizontal Stretch/Compression by a factor of b
• f(x)→f(1 bx) • b > 1 stretches away from the y-axis.
• 0 < |b| < 1 compresses toward the y-axis.
• y-intercepts remain the same.
Vertical Stretch/Compression by a factor of a
• f(x) → a f(x) • a > 1 stretches away from the x-axis.
• 0 < |a| < 1 compresses toward the x-axis.
• x-intercepts remain the same.Stretches and compression change the slope of a linear function.
If the line becomes steeper, the function has been stretched vertically or compressed horizontally.
If the line becomes flatter, the function has been stretched horizontally or compressed vertically.
Horizontal Stretch/Compression by a factor of b
• f(x)→f(1 bx) • b > 1 stretches away from the y-axis.
• 0 < |b| < 1 compresses toward the y-axis.
• y-intercepts remain the same.
Vertical Stretch/Compression by a factor of a
• f(x) → a f(x) • a > 1 stretches away from the x-axis.
• 0 < |a| < 1 compresses toward the x-axis.
• x-intercepts remain the same.
Reason
When we use dilation scale factors, we can shrink or expand a figure. We know each angle is congruent, or the same. We know each segment is proportional, the slope of each segment is maintained, and the perimeter of the preimage and image have the same scale factor.
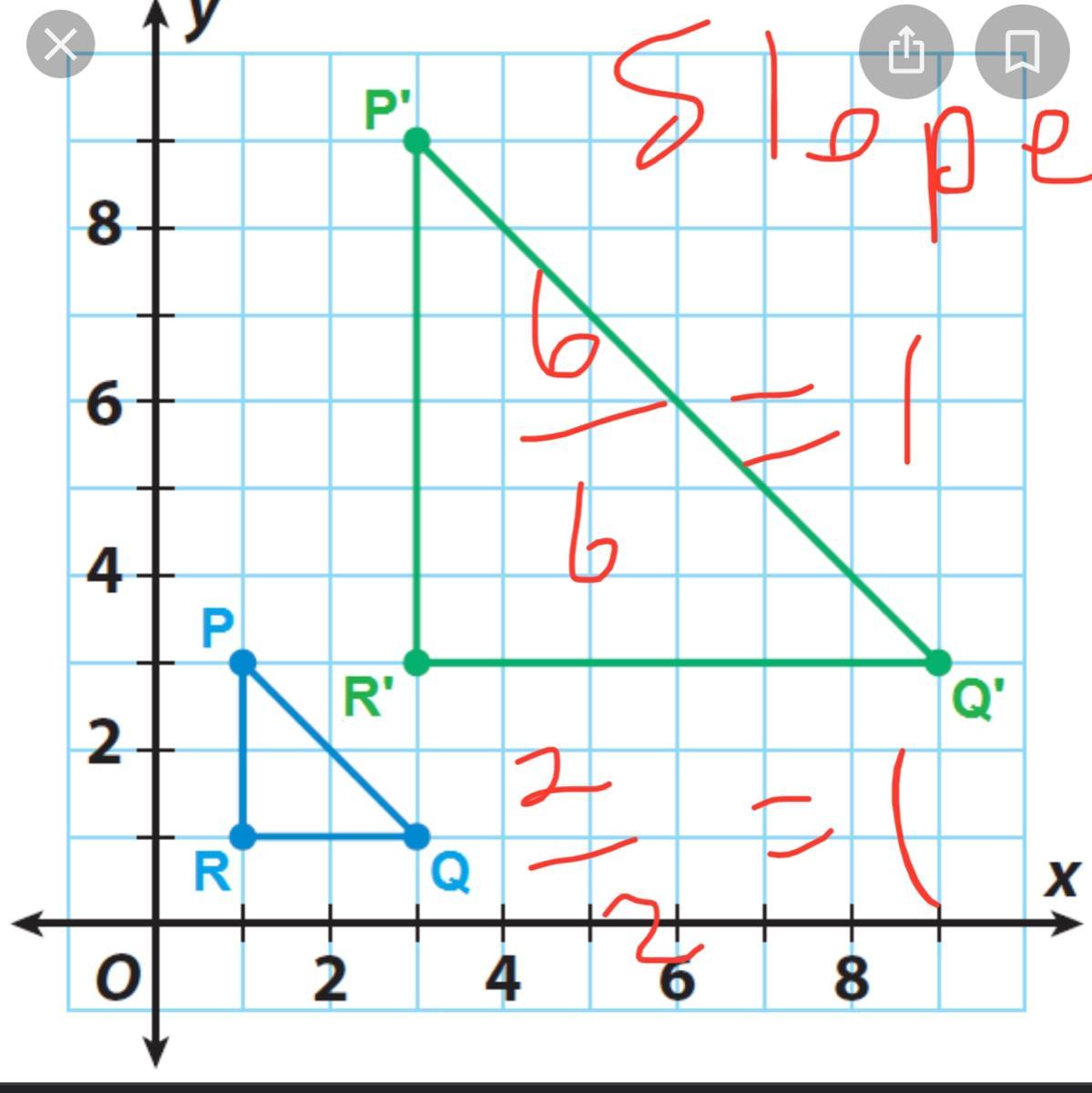
Slope does not change. See example attached.
Caroline has 12 cherries and 13 strawberries. What percentage are the cherries?
Answers
Answer:
12/25=48%
Step-by-step explanation:
total=denominator=12+13=25
numerator=no of cherries=12
12/25=x/100
1200=25x
x=48%
A percentage is a number or ratio that represents a fraction of 100.
Percentage formula = (Required number / Total number) x 100
The percentage of cherries is 48%.
What is a percentage?A percentage is a number or ratio that represents a fraction of 100.
Example:
50% = 50 /100 = 1/2
25% = 25/100 = 1/4
We have,
Number of cherries = 12
Number of strawberries = 13
The total number of fruits:
= 12 + 13
= 25
Percentage formula = (Required number / Total number) x 100
The percentage of cherries:
= (Number of Cherries / Total number of fruits) x 100
= (12 / 25) x 100
[ 25 x 4 = 100 ]
= 12 x 4
= 48%
Thus,
The percentage of cherries is 48%.
Learn more about percentages here:
https://brainly.com/question/14772302
#SPJ2
What is the current flowing through an element if the charge flow is given by q(t) = (t + 2) mc?
Answers
The current flowing through the element if the charge flow is given by q(t) = (t + 2) mc is dc/dt(t + 2)
The expression for the charge flow is given by q(t) = (t + 2) mc, then the current flowing through an element can be calculated as follows:To determine the current flowing through an element if the charge flow is given by q(t) = (t + 2) mc, we need to differentiate the expression with respect to time (t).dq(t)/dt = d/dt (t + 2) mc = mdc/dt(t + 2) dc/dt = current flowing through the element
The current flowing through the element if the charge flow is given by q(t) = (t + 2) mc is dc/dt(t + 2)
Learn more about Current
brainly.com/question/13076734
#SPJ4
Which inequality represents all possible solutions of -12d2 -6?
A d< - 1/2
B d> - 1/2
C d< 1/2
D d> 1/2
Answers
The inequality the represents the expression -12d < - 6 is d < 1/2.
What is inequality?Mathematical expressions with inequalities are those in which the two sides are not equal. Unlike to equations, we compare two values in inequality. Less than (or less than or equal to), larger than (or greater than or equal to), or not equal to signs are used in place of the equal sign. Equations are not always balanced on both sides using a "equal to" sign in mathematics. Occasionally it might be about a "not equal to" connection, meaning that something is superior to or inferior to another.
The given inequality is -12d < - 6.
The inequality can be written as:
d < -6/ -12
d < 1/2
Hence, the inequality the represents the expression -12d < - 6 is d < 1/2.
Learn more about inequality here:
https://brainly.com/question/30231190
#SPJ1
