Please please please help John wants to buy a new video game it cost $39.00. He has $14.00 in his piggy
bank. He works for farmer Jones down the street for $5.00 an hour. How many
hours will he need to work before he can buy his new video game? Make and equation
Answers
Answer:
5 hours
Step-by-step explanation:
39 - 14 = 25
5 x 5 = 25
14 + 25 = 39
Answer:
5 hours
Step-by-step explanation:
John needs $39.00, he has $14 now, and He earns $5 a week working. x= how many hours.
Start by setting the equation,
$39.00= $5x + $14.00
Then solve by
1) subtracting 14 from both sides;
39(-14)= 5x+14(-14), by subtracting 14 on both sides you cancelled out the 14;
39 -14 = 5x becomes $25= 5x (39 -14 =25)
2) divide both sides by 5 to get x alone
25 (/5)=5x (/5) which becomes 25/5=x
3) 25/5 is 5
John must work 5 hours to have enough money to buy his video game.
Related Questions
Does anybody know the answer to those two questions

Answers
Answer:
Question Number 2 is 20
Question number 3 is 18x-32
Step-by-step explanation:
A Sequence of Transformations
Example 6: Translate ABCDEF (x, y)=(x+1, y - 6), and then dilate, with G as the center
of dilation, by a scale factor of 3. Graph the final image.
How will you and your

Answers
Answer:
steps below
Step-by-step explanation:
(3,3)(6,3)(5,0)(6,-1)(3,-1)(4,0) ==> (x+1,y-6)
==> (4,-3)(7,-3)(6,-6)(7,-7)(4,-7)(5,-6) ==> dilation by factor 3 and center G (9,-9)
==> (-6,9)(3,9)(0,0)(3,-3)(-6,3)(-3,0)
check: NO=9 AB=3 NO/AB = 3

Find 2x^3-x^3+4x+7 divided by x+3
Answers
Answer:
(x^3+4x+7)/(x+3)
Step-by-step explanation:
you just combine the like terms on the top and put it in a fraction. let me know if that helped.
An aquarium 4 ft long, 2 ft wide, and 4 ft deep is full of water. (recall that the weight density of water is 62. 5 lb/ft3. ) (a) find the hydrostatic pressure (in lb/ft2) on the bottom of the aquarium
Answers
The hydrostatic pressure on the bottom of the aquarium is 8044 lb/ft2.
The hydrostatic pressure on the bottom of the aquarium can be found using the formula:
P = ρgh
where P is the pressure, ρ is the density of the fluid (water), g is the acceleration due to gravity, and h is the depth of the fluid.
Here, ρ = 62.5 lb/ft3, g = 32.2 ft/s2 (acceleration due to gravity), and h = 4 ft (depth of water).
Thus, the hydrostatic pressure on the bottom of the aquarium is:
P = (62.5 lb/ft3) x (32.2 ft/s2) x (4 ft)
= 8044 lb/ft2
Therefore, the hydrostatic pressure on the bottom of the aquarium is 8044 lb/ft2.
Learn more about hydrostatic pressure here:
https://brainly.com/question/14810152
#SPJ11
Researchers investigated whether there is a difference between two headache medications, R and S. Researchers measured the mean times required to obtain relief from a headache for patients taking one of the medications. From a random sample of 75 people with chronic headaches, 38 were randomly assigned to medication R and the remaining 37 were assigned to medication S. The time, in minutes, until each person experienced relief from a headache was recorded. The sample mean times were calculated for each medication. Have the conditions been met for inference with a confidence interval for the difference in population means? A Yes, all conditions have been met. B No, because the data were not collected using a random sample. © No, because cause and effect cannot be inferred since there is a random sample No, because the sample sizes are not large enough to assume the distribution of the difference in sample means is approximately normal. (E) No, because the sample sizes are not the same.
Answers
The correct answer is option D. No, because the sample sizes are not large enough to assume the distribution of the difference in sample means is approximately normal.Researchers investigated whether there is a difference between two headache medications, R and S.
Researchers measured the mean times required to obtain relief from a headache for patients taking one of the medications. From a random sample of 75 people with chronic headaches, 38 were randomly assigned to medication R and the remaining 37 were assigned to medication S. The time, in minutes, until each person experienced relief from a headache was recorded. The sample mean times were calculated for each medication.To construct a confidence interval, we require that some conditions are satisfied. The assumptions that should be met for inference with a confidence interval for the difference in population means are as follows:The data is independent;The data in each group should be roughly normally distributed;Both groups have the same variance, and each group should have a large enough sample size so that the central limit theorem (CLT) holds. The CLT holds for the difference in the sample means since both samples are taken from a population that is normally distributed. We can also assume that the sample size is large enough to use the t-distribution since each sample has more than 30 observations. As a result, all of the conditions have been met. As a result, we may employ a t-distribution to make inferences about the population difference between the two headache medications.
for more questions on headache
https://brainly.com/question/14974427
#SPJ11
f(x) = 3x^2 + 4x - 6
g(x) = 6x^3 – 5x^2 - 2
Find (f - g)(x).
Answers
Answer:
(f-g)(x) = -6x^3 + 8x^2 + 4x - 4
Step-by-step explanation:
subtract g(x) from f(x)
(f-g)(x) = (3x^2 + 4x - 6) - (6x^3 - 5x^2 -2)
distribute the negative
(f-g)(x) = 3x^2 + 4x - 6 - 6x^3 + 5x^2 +2
like terms and in order of exponent
(f-g)(x) = -6x^3 + 8x^2 + 4x - 4
If an airplane moves at a constant speed of 120 miles per hour for 3 hours. How far does it go?
Answers
Answer:
360 miles
Step-by-step explanation:
For every one hour traveled, 120 miles are covered. If there are three hours traveled, then we would have 120+120+120 (aka 120*3). At the end of the 3 hours, we will have traveled 360 miles. Thank you for choosing Southwest, enjoy your day.
How many years will it take $2,000 to grow to $3,300 if it is invested at 4.75% compounded continuously?
Answers
Answer:
10.54263764
Step-by-step explanation:
\(3300=2000e^{.0475t}\\\\1.65=e^{.0475t}\\\ln(1.65)=.0475t\\.500775288=.0475t\\t=10.54263764\)
Answer:
It will take about 10.5 years for the investment to reach $3,300.
Step-by-step explanation:
Continuous compound is given by:
\(\displaystyle A=Pe^{rt}\)
Where P is the principal, e is Euler's number, r is the rate, and t is the time (in this case in years).
Since our principal is $2,000 at a rate of 4.75% or 0.0475, our equation is:
\(\displaystyle A=2000e^{0.0475t}\)
We want to find the number of years it will take for our investment to reach $3,300. So, substitute 3300 for A and solve for t:
\(3300=2000e^{0.0475t}\)
Divide both sides by 2000:
\(\displaystyle e^{0.0475t}=\frac{33}{20}\)
We can take the natural log of both sides:
\(\displaystyle 0.0475t=\ln\left(\frac{33}{20}\right)\)
Therefore:
\(\displaystyle t=\frac{1}{0.0475}\ln\left(\frac{33}{20}\right)\approx 10.54\text{ years}\)
It will take about 10.5 years for the investment to reach $3,300.
helppp pleaseeeeeeee

Answers
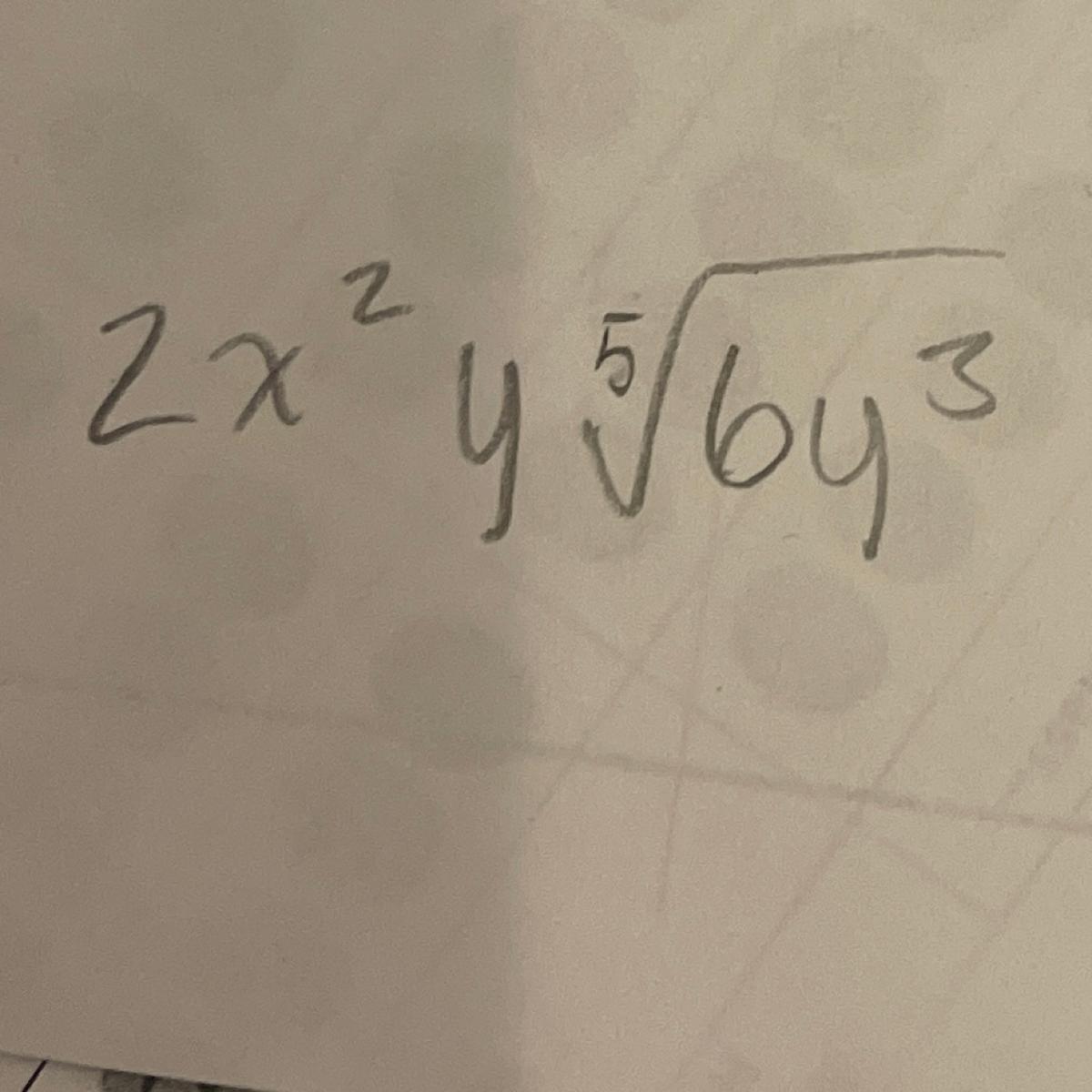
0.001429 in scientific notation
Answers
Answer:
1.429 × 10^-3
Step-by-step explanation:
since it's below 1, you're moving the decimal to the right, which makes the 3 negative.
Noel is a computer repairman. To fix a computer, he charges a customer $40 per hour, plus a fixed fee of $15 for the service call, as represented by the function f(x) = 40x + 15. In the equation, what is represented by the variable “x”?
A. the amount Noel charged per hour
B. the number of hours Noel worked
C. the fixed fee for the service call
D. the total cost of the repair job
Answers
Answer:
B
Step-by-step explanation:
40x(amount noel worked) because he is charging 40$ at a rate of 40 p/h so it is B.
Kimberly goes bowling. It costs $5 to rent the
shoes and $3.75 for every game she plays. Which
function represents the amount of money, y.
Kimberly will pay for bowling x games?
A. y = 5x + 3.75
B. y = 3.75x + 5
C. y = 5x - 3.75
D. y = 3.75 - 5
Answers
Answer:
B is the correct function
Step-by-step explanation:
3)
The probability that Max does not bring his calculator to Maths lesson is 0.2.
Complete the tree diagram for two successive lessons.
Answers
Step-by-step explanation:
that's it b is for brings
b compliment is for doesn't bring

A store is marking down the price of a television 25% from the original price of $250 which expression can be used to find the markdown price
Answers
Answer:
62.5%
Step-by-step explanation:
Thw price markdown will be 62.5%
Answer a I think
Step-by-step explanation:
Which of the following quadratic regression equations best fits the data shown below?

Answers
Answer:
Step-by-step explanation:
In order to do this, you have to know how to use your calculator's regression equation function.
First enter in the data. Hit "stat" then 1:Edit and enter all the x values into L1. After each value, hit enter. When you're done with the x list, arrow over to L2 and enter in all the y-values. If there are already values there you need to clear, arrow up to highlight L1, hit "clear", then "enter" and the values will disappear. Do that for both lists if you need to.
After the data is listed in L1 and L2, hit "stat" again and arrow over to "Calc". Hit 5:QuadReg. If you have a TI 83, your equation will be there for you. If you have a TI 84, you'll need to arrow down to "calculate" to get the equation. Regardless, the equation is
\(y=3.48x^2+1.22x+3.44\), the last choice in your options.
Answer: D
Becasye I got it from him
Mayra buys a sweater for $20. The next month, the store increases the price of the sweater to $24. What is the percent increase in the price of the sweater?
A. 20% increase, because 24 over 20 equals X over 100.
B. 17% increase, because 4 over 24 equals X over 100.
C. 17% increase, because 20 over 24 equals X over 100.
D. 20% increase, because 4 over 20 equals X over 100.
Answers
Answer:
I think it is D.
Step-by-step explanation: So I am not 100% Sure on this, but if D is wrong, it is 100% A.
Answer:
D
Step-by-step explanation:
Because 20% increased is like 4 over 20, 4 times 20 is 100 so x is over 100
the length of an arc of a circle is 1/5 of its circumference. if the area of the circle is346.5cm²,find the angle subtended by the arc at the centre of the angle
Answers
Answer:
72°
Step-by-step explanation:
From the question,
Area of the circle = πr²
A = πr²................. Equation 1
Where r = radius of the circle.
⇒ r = √(A/π)............. Equation 2
Given: A = 346.5 cm², π = 3.14
r = √(346.5/3.14)
r = √(110.35)
r = 10.5 cm.
Therefore,
circumference of the circle = 2πr = 2×3.14×10.5
circumference = 65.94 m
If the length of the arc(s) is 1/5 of its circumference.
Therefore, length of arc (s) = 13.188
⇒ length of arc/circumference = 13.188/65.94 = 1/5
s/2πr = θ/360
Where θ = angle substends at the center of the circle
1/5 = θ/360
θ = 360/5
θ = 72°
The graph of a function is shown below. What is its range?
O (1, 2, 4)
O (1, 2, 3, 5)
O All real numbers.
O (1, 2, 3, 4)

Answers
(1,2,4)
Step-by-step explanation:Range describes the y-values of a graph.
Range
Range is the y-values that a graph covers. Remember that the y-values are found on the vertical axis. If the graph is not continuous, then the values between the points are not included in the range. Similar to the range, the domain of a graph is the x-values that a graph covers. If there is a coordinate point with a y-value, then that y-value should be included in the range.
Finding Range
In order to find the range, we need to find all the unique y-values of the graph. Additionally, the range is given in numerical order. This means starting from the least value and going up to the greatest. The lowest y-value is 1, then 2, and finally 4. Even though there are two points where y = 2, we are only looking for unique values. This means that the range is (1,2,4).
(3). Harvard Bridge, which connects MIT with its fraternities across the Charles River, has a length of 364.4 Smoots plus one ear. The units of one Smoot is based on the length of Oliver Reed Smoot, Jr., class of 1962, who was carried or dragged length by length across the bridge so that other pledge members of the Lambda Chi Alpha fraternity could mark off (with paint) 1-Smoot lengths along the bridge. The marks have been repainted biannually by fraternity pledges since the initial measurement, usually during times of traffic congestion so that the police could not easily interfere. (Presumably, the police were originally upset because a Smoot is not an SI base units, but these days they seem to have accepted the units.) The figure shows three parallel paths, measured in Smoots (S), Willies (W), and Zeldas (Z). What is the length of 64.0 Smoots in (a) Willies and (b) Zeldas?
Answers
The length of 64.0 Smoots in Zeldas is 16.0 Willies and 5.33 Zeldas. The bridge, which links MIT with its fraternities over the Charles River, is the Harvard Bridge. It measures 364.4 Smoots plus one ear in length.
The Smoot is a unit of length based on the height of Oliver Reed Smoot Jr., the Lambda Chi Alpha fraternity's class of 1962. Because he was carried or dragged length by length over the bridge, the additional ear indicates the length of his head.
Length of Harvard Bridge = 364.4 Smoots + 1 ear.
Therefore, 1 Smoot = 364.4/1.0
= 364.4 Smoots
Length of 64.0 Smoots in (a) Willies
To find the length of 64.0 Smoots in Willies, we use the conversion ratios:
1 Willie = 4.0 Smoots
Hence, the length of 64.0 Smoots in Willies is:
64.0 Smoots × (1 Willie/4.0 Smoots)
= 16.0 Willies.
Length of 64.0 Smoots in (b) Zeldas
To find the length of 64.0 Smoots in Zeldas, we use the conversion ratios:1 Zelda = 3.0 Willies,1 Willie = 4.0 Smoots
Hence, the length of 64.0 Smoots in Zeldas is:64.0 Smoots × (1 Willie/4.0 Smoots) × (1 Zelda/3.0 Willies) = 5.33 Zeldas.
To know more about Harvard visit-
brainly.com/question/3745154
#SPJ11
Find a polynomial p(x) which has real roots at −2,1, and 7 and
has the following end behavior:
limx→[infinity]p(x) = −[infinity],
limx→-[infinity]p(x) = −[infinity]
Answers
A polynomial function is a mathematical expression with more than two algebraic terms, especially the sum of many products of variables that are raised to powers.
A polynomial function can be written in the formf(x)=anxn+an-1xn-1+...+a1x+a0,where n is a nonnegative integer and an, an−1, an−2, …, a2, a1, and a0 are constants that are added together to obtain the polynomial.
The end behavior of a polynomial is defined as the behavior of the graph of p(x) for x that are very large in magnitude in the positive or negative direction.
If the leading coefficient of a polynomial function is positive and the degree of the function is even, then the end behavior is the same as that of y=x2. If the leading coefficient of a polynomial function is negative and the degree of the function is even,
then the end behavior is the same as that of y=−x2.To obtain a polynomial function that has the roots of −2, 1, and 7 and end behavior as limx→[infinity]p(x) = −[infinity] and limx→−[infinity]p(x) = −[infinity], we can consider the following steps:First, we must determine the degree of the polynomial.
Since it has three roots, the degree of the polynomial must be 3.If we want the function to have negative infinity end behavior on both sides, the leading coefficient of the polynomial must be negative.To obtain a polynomial that passes through the three roots, we can use the factored form of the polynomial.f(x)=(x+2)(x−1)(x−7)
If we multiply out the three factors in the factored form, we obtain a cubic polynomial in standard form.f(x)=x3−6x2−11x+42
Therefore, the polynomial function that has real roots at −2, 1, and 7 and has the end behavior as limx→[infinity]p(x) = −[infinity] and limx→−[infinity]p(x) = −[infinity] is f(x)=x3−6x2−11x+42.
To know more about real roots, click here
https://brainly.com/question/21664715
#SPJ11
Write fraction in Ascending Order :

Answers
Answer:
In ascending order: 1/2, 3/5, 6/11, 8/9
What is the НСF of
4725
5850
Answers
Answer:
433345344 Answer
Step-by-step explanation:
Please help I do not know the answer

Answers
Answer:
answer is y = 3/4x - 5
Step-by-step explanation:
picture below.
The 3/4 comes from the line. You would use rise over run to find the slope, which in this case is 3/4. and the -5 is basically the y-intercept

the support for a basketball hoop forms a right triangle as shown. what is the length, x, of the horizontal portion of the support?
Answers
According to Pythagorean Theorem, The length of the horizontal portion of the support, x, is 3.87 feet.
The length of the horizontal portion of the support, x, can be determined by using the Pythagorean Theorem. The Pythagorean Theorem states that a2 + b2 = c2, where a and b are the sides of a right triangle and c is the hypotenuse.
In this case, a = 7 feet, b = x, and c = 8 feet. We can use the Pythagorean Theorem to solve for x: 72 + x2 = 82, or 49 + x2 = 64. This can be solved for x to get x2 = 15 and x = √15 ≈ 3.87 feet.
Therefore, the length of the horizontal portion of the support, x, is 3.87 feet.
For more such questions on Pythagorean Theorem.
https://brainly.com/question/29769496#
#SPJ11
PLEASE HELP ASAP
WILL GIVE BRAINLEST ONLY IF CORRECT
SOLVE FOR THE INDICATED ANGLE

Answers
Answer:
57\(degrees\)
Step-by-step explanation:
For the first triangle the third angle is not given
So we take that angle as x
First = x+35+45=180
x+80=180
x=180-80
x=100
As we got 100*
For the second triangle
We take the variable as y
Second triangle=y+23+100=180
y+123=180
y=180 -123=57
Hence the answer is 57degrees
Please help, any help would be appreciated.

Answers
Answer:
160,170 and 240,250
Step-by-step explanation:
sorry if wrong
Solve the equation for y.
1+y=-4/5-2
Answers
Answer:
y = -19/5
Step-by-step explanation:
Answer:
To solve, you should try to isolate the y-term.
1+y=-4/5-2
Subtract 1 from both sides.
y=-4/5-2-1
Combine the like terms on the right sides (subtract).
y=-2 4/5 - 1
y= -3 4/5
Hope this helps! :)
Select the correct answer. Let f(x) and g(x) be polynomials as shown below. Which of the following is true about f(x) and g(x)? f(x) and g(x) are closed under multiplication because when multiplied, the result will be a polynomial. f(x) and g(x) are closed under multiplication because when multiplied, the result will not be a polynomial. f(x) and g(x) are not closed under multiplication because when multiplied, the result will be a polynomial. f(x) and g(x) are not closed under multiplication because when multiplied, the result will not be a polynomial.
Answers
f(x) and g(x) are not closed under subtraction because when subtracted, the result will be a polynomial, the correct option is B.
What is Polynomial?A polynomial is a mathematical equation that solely uses the operations addition, subtraction, multiplication, and non-negative integer exponentiation of variables. Variables are sometimes known as indeterminate in mathematics. Majorly used polynomials are binomial and trinomial.
Given f(x) and g(x) two polynomial functions in the standard form of the polynomial,
According to Closure Property, when something is closed, the output will be the same as the input.
The polynomials f(x) and g(x) can be seen in the image.
On subtracting the two polynomials, the output will be a polynomial and so it is closed under subtraction.
Therefore, The reason why f(x) and g(x) are not closed under subtraction is that the outcome of subtraction will be a polynomial, making option B the best choice.
Learn more about Polynomials here:
https://brainly.com/question/11536910
#SPJ1
Complete question:

Which of the following is true?
Sine is negative in Quadrant I.
Tangent is positive in Quadrant III.
Cosine is positive in Quadrant III.
Sine is negative in Quadrant II.
Answers
B) Tangent is positive in Quadrant III is correct!
10. Find the exact value of each expression. c. sin(2sin-4 ()
Answers
To find the exact value of the expression sin(2sin^(-1)(x)), where x is a real number between -1 and 1, we can use trigonometric identities and properties.
Let's denote the angle sin^(-1)(x) as θ. This means that sin(θ) = x. Using the double angle formula for sine, we have: sin(2θ) = 2sin(θ)cos(θ).Substituting θ with sin^(-1)(x), we get: sin(2sin^(-1)(x)) = 2sin(sin^(-1)(x))cos(sin^(-1)(x)).
Now, we can use the properties of inverse trigonometric functions to simplify the expression further. Since sin^(-1)(x) represents an angle, we know that sin(sin^(-1)(x)) = x. Therefore, the expression becomes: sin(2sin^(-1)(x)) = 2x*cos(sin^(-1)(x)).
The remaining term, cos(sin^(-1)(x)), can be evaluated using the Pythagorean identity: cos^2(θ) + sin^2(θ) = 1. Since sin(θ) = x, we have:cos^2(sin^(-1)(x)) + x^2 = 1. Solving for cos(sin^(-1)(x)), we get:cos(sin^(-1)(x)) = √(1 - x^2). Substituting this result back into the expression, we have: sin(2sin^(-1)(x)) = 2x * √(1 - x^2). Therefore, the exact value of sin(2sin^(-1)(x)) is 2x * √(1 - x^2), where x is a real number between -1 and 1.
To learn more about Pythagorean identity click here:
brainly.com/question/10285501
#SPJ11