PLEASE PLEASE HELP ME OUT!! CURRENTLY REALLY STRUGGLING AND TRYING TO PASS THIS SCHOOL YEAR, WILL GIVE BRAINLIEST!

Answers
Answer:
3. X = (-4, -5), Y = (0, -3), Z = (-1, -7)
4. Refer back to my explanation.
5. a. Refer back to my explanation.
5. b. 28 units^2; please solve for the other rectangle.
5. c. No, because translation means moving the figure, not changing the shape or size.
Step-by-step explanation:
Warning in advance: the picture is very blurry when looking at the numbers on the graph so I have tried my best to decipher them.
3.
First, write down the ordered pairs for X, Y, and Z.
X = (-1, 4)
Y = (3, 6)
Z = (2, 2)
Note that horizontal movement (moving left or right) affects only the x coordinate. If you are moving to the left, you will subtract from the x coordinate. If you are moving to the right, you will add to the x coordinate.
Vertical movement (moving up or down) affects only the y coordinate. If you are moving up, you will add to the y coordinate. If you are moving down, you will subtract from the y coordinate.
Apply this concept to the ordered pairs:
Moving left = subtraction from x coordinate
Moving down = subtraction from y coordinate
X2 = (-1 - 3, 4 - 9) = (-4, -5)
Y2 = (3 - 3, 6 - 9) = (0, -3)
Z2 = (2 - 3, 2 - 9) = (-1, -7)
Your new image should have X at (-4, -5), Y at (0, -3), and Z at (-1, -7).
4.
After reading the concepts and seeing my explanation and demonstration, please try number 4 on your own. If you have any questions, feel free to ask me for help.
5. a.
The same goes for this problem since it is the same concept.
5. b.
To find the area of a rectangle, use the equation length × width, also known as l × w. In order to figure out what the length and width are equal to, count the units on the graph. JK is 7 units wide. Since this is a rectangle, ML will be the same width, 7 units. KL is 4 units long so JM will also be 4 units long. Now that we know the number of units, we can plug the numbers into the formula:
4 units × 7 units = 28 units^2
The question asks to solve for the area of each rectangle so please try the same for the rectangle you create from problem 5. a.
5. c.
The area of a figure will not change after translation because translation does not affect the figure's size or shape. If the figure is only moved, then no part of its area is changed so no, the area of a figure will not change.
Related Questions
A campfire has a flame 24 inches tall after burning for 4 hours. After 6 hours, it is 20 inches tall. Write a linear equation to model the relationship between height h of the fire and time t. Predict how tall the fire will be after burning 8 hours.
(I dont have many points as this is a new account but i will mark brainiest if work is shown and correct answer)
Answers
The linear equation that model the above is h = 32 -2t and the height of fire after 8 hours is 16 inches
What is a linear equation?A linear equation is an equation in which the highest power of the variable is always 1. It is also known as a one-degree equation. The standard form of a linear equation in one variable is of the form Ax + B = 0. Here, x is a variable, A is a coefficient and B is constant.
Using the equation for a straight line and writing similar
h = mt + c
h = height, t = hours m and c are constants
at h = 24, t = 4
Equation becomes
24 = 4m + c--------------1
at h = 20, t = 6
20 = 6m + c---------------2
Subtract equation 2 from 1 so that the eqution reduces to
4 = -2m
m = 4/-2
m = -2
substitute m in equation 1
24 = 4( -2) + c
24 = -8 + c
c = 32
substituting m and c in he linear equation above we have
h = 32 - 2t
After 8 hours, the height h = 32 -2(8) which is 16 inches
In conclusion the linear equation is h = 32 - 2t and the height of fire after 8 hours is 16 inches
Learn more about linear equation: https://brainly.com/question/4074386
#SPJ1
Lena won a charity raffle. Her prize will be randomly selected from the 9 prizes shown below. The prizes include 7 rings, 1 camera, and 1 headset.
(a) Find the odd against Lena winning a headset.
(b) Find the odds in favor of Lena winning a headset

Answers
Answer:
(b) Find the odds in favor of Lena winning a headset
Step-by-step explanation:
what is the difference between (4,4) and (1,-3)
Answers
Answer:
7/3
Step-by-step explanation:
If I solved it correctly, you use y2-y1 / x2-x1. From here you get -3-4/1-4=-7/-3 which when you divide equals 7/3. LMK IF its wrong and ill figure it out champ!
What equation is equivalent to 8.8 x 10^9 divided by 2.2 x 10^-3?
Answers
The quantities and y are proportional.
2
y
5.8
5.8
7.5
7.5
11.2
11.2
Find the constant of proportionality (r) in the equation y = rr.

Answers
Answer:
r = 1
Step-by-step explanation:
We know that x and y are proportional, with the the equation y = rx representing the proportional relationship. Where r = constant of proportionality
Thus the constant of proportionality would be:
r = y/x
Using any of the given pair of values from the table, say (5.8, 5.8),
r = 5.8/5.8
r = 1
what is the slope of the line

Answers
Answer:
3
Step-by-step explanation:
3/1
Find the values of a - f.

Answers
Answer:
a=42 , b=21 , c=38 , d=55 , e= 46 , f=101

SOMONE PLEASE HELPy4.3-211A-4-3-2 -1112334-1+-2+-3+1-4What is the slope of the line?
Answers
find the absolute valve of 1 1/2 - 2/31
Answers
Answer: To find the absolute value of the expression 1 1/2 - 2/31, we first need to convert the mixed number 1 1/2 into an improper fraction.
1 1/2 can be written as (2 * 1 + 1) / 2, which is equal to 3/2.
Now we can subtract 2/31 from 3/2:
3/2 - 2/31 = (3 * 31 - 2 * 2) / (2 * 31) = (93 - 4) / 62 = 89/62.
The absolute value of a fraction is the positive value without considering its sign. So, the absolute value of 89/62 is 89/62.
Therefore, the absolute value of 1 1/2 - 2/31 is 89/62.
Jenny won a charity raffle. Her prize will be randomly selected from the 9 prizes shown below. The prizes include 4 rings, 3 cameras, and 2 headsets.
Prizes
(a) Find the odds against Jenny winning a headset.
(b) Find the odds in favor of Jenny winning a headset.
Answers
The odds against Jenny winning a headset are 88.89% or 8 out of 9, and the odds in favor of Jenny winning a headset are 11.11% or 1 out of 9.
Since Jenny won a charity raffle, and her prize will be randomly selected from the 9 prizes shown below, and the prizes include 7 rings, 1 camera, and 1 headset, to find the odds against Jenny winning a headset, and find the odds in favor of Jenny winning a headset, the following calculations must be performed:
· 1 headset out of 9 total prizes
· 1/9 = headset
· 1/9 x 100 = 11.11%
· 100 - 11.11 = 88.89%
Therefore, the odds against Jenny winning a headset are 88.89% or 8 out of 9, and the odds in favor of Jenny winning a headset are 11.11% or 1 out of 9.
QUESTION 25
Solve for a. Enter a number answer only.
25
a
24

Answers
Answer: 7
Step-by-step explanation:
We can use the Pythagorean theorem (a^2+b^2=c^2) so 25^2=625, and 24^2=576
576+b^2=625 b^2=49 b - (a)=7
Please Please help! I am so beyond stuck. I have figured out what is wrong with the first step but I cant figure out what is going on with the second one!
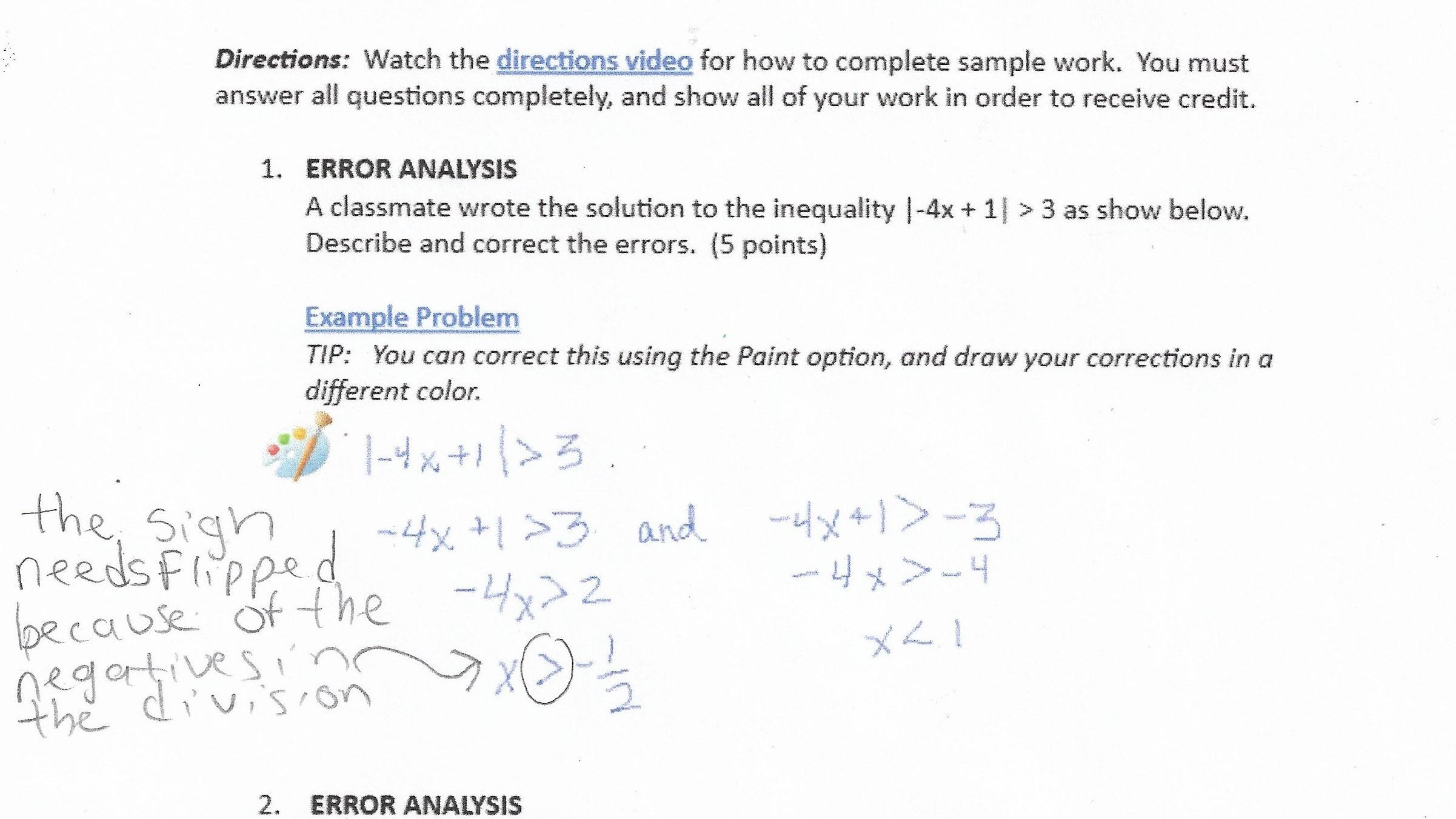
Answers
Answer:
\(x<-\frac{1}{2}\text{ or } x>1\)
Step-by-step explanation:
So we have the equation:
\(|-4x+1|>3\)
First, note that since the sign is greater than, this is an or inequality (not an "and" inequality). This is the student's first mistake.
So, let's solve this inequality.
Case 1:
\(-4x+1>3\)
Subtract 1 from both sides:
\(-4x>2\)
Divide both sides by -4:
\(x<-\frac{1}{2}\)
The student did not flip the sign when doing this step, hence the incorrect answer.
You correctly spotted the student's mistake. Nicely done!
Case 2:
\(-4x+1<-3\)
This is what you're missing: for this second instance, we must flip the sign to less than right away. This is because we are essentially multiplying the 3 by a negative. So, we must flip the sign in order to be correct.
Now solve. Subtract 1 from both sides:
\(-4x<-4\)
Divide both sides by -4. Since we're dividing by a negative, flip the sign:
\(x>1\)
So, our solutions are:
\(x<-\frac{1}{2}, x>1\)
As mentioned previously, this is an or inequality. Therefore, our final answer is:
\(x<-\frac{1}{2}\text{ or } x>1\)
Edit: Improved Answer
Algebra 2 Unit 1 Assessment
Which of the following contains multiple variables?
4a + 5b + 1
4a + 5a + 1
4a - 1
4 - 1
Answers
Answer:
I would have to say A
Step-by-step explanation:
B has 2 of the same variables while A has to different variables and C&D have no variables there for the answer is A
How can areas of composite 2-D figures be found using simple 2-D shapes?
Answers
Answer:
hmm length x breadth... I'd simple formula
If the Earth's real diameter is 12,756km and the Earth's model diameter is 25mm, what is Jupiter's model diameter if the real diameter is 142,984km?
Answers
Jupiter's model diameter will be 280 mm, if the real diameter is 142,984km?.
What is Proportion?Proportion can be referred to as a part, share, or number considered in comparative relation to a whole. Proportion is defined as when two ratios are equivalent, they are in proportion. It is an equation or statement used to depict that two ratios or fractions are equal.
real diameter : model diameter
12,756 : 25
142,984 : x
where x is the model diameter of jupiter
x = (25 x 142,984) / 12,756
x = 280 mm
Therefore, the model diameter of Jupiter is 280mm
Learn more about proportion here: https://brainly.com/question/19994681
#SPJ1
a) (10 pts) Re-express the given differential equation as a first order differential equation by utilizing matrix
and vector notation and in accordance with ()
= () form.
b) (10 pts) Is the system obtained in (a) stable, neutrally stable of unstable? Determine this using matrix.
c) (10 pts) Compute the eigenvalues and eigenvectors of matrix.
d) (10 pts) Using the results computed in (c) find and matrices and show that =
−
relationship
(i.e., the diagonalization relationship) is a valid relationship.

Answers
a) To re-express the given differential equation as a first-order differential equation using matrix and vector notation, we can rewrite it in the form:
\(x' = Ax\)
where x is a vector and A is a square matrix.
b) To determine the stability of the system obtained in part (a), we need to analyze the eigenvalues of matrix A.
If all eigenvalues have negative real parts, the system is stable.
If at least one eigenvalue has a zero real part, the system is neutrally stable.
If at least one eigenvalue has a positive real part, the system is unstable.
c) To compute the eigenvalues and eigenvectors of matrix A, we solve the characteristic equation
\(det(A - \lambda I) = 0\),
where λ is the eigenvalue and I is the identity matrix.
By solving this equation, we obtain the eigenvalues.
Substituting each eigenvalue into the equation
\((A - \lambda I)v = 0\),
where v is the eigenvector, we can solve for the eigenvectors.
d) Once we have computed the eigenvalues and eigenvectors of matrix A, we can construct the diagonalization relationship as follows:
\(A = PDP^{(-1)}\)
where P is a matrix whose columns are the eigenvectors of A, and D is a diagonal matrix whose diagonal elements are the eigenvalues of A.
To show that this relationship is valid, we can compute \(PDP^{(-1)}\) and verify that it equals A.
For such more questions on differential equation
https://brainly.com/question/18760518
#SPJ8
Due at 11:59, help pls :)

Answers
-5: yes
9: no
7: yes
A population of values has a normal distribution with �=189.7 and �=96.7. You intend to draw a random sample of size �=62.
Find the probability that a single randomly selected value is between 189.7 and 213.
P(189.7 < X < 213) =
Find the probability that a sample of size �=62 is randomly selected with a mean between 189.7 and 213.
P(189.7 < M < 213) =
Enter your answers as numbers accurate to 4 decimal places. Answers obtained using exact z-scores or z-scores rounded to 3 decimal places are accepted.
Answers
The probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213 is approximately 0.9702.
To find the probability that a single randomly selected value is between 189.7 and 213, we can use the standard normal distribution.
Step 1: Calculate the z-scores for the given values using the formula:
z = (x - μ) / σ
For 189.7:
z1 = (189.7 - 189.7) / 96.7 = 0
For 213:
z2 = (213 - 189.7) / 96.7 ≈ 0.2417
Step 2: Utilize a standard typical conveyance table or number cruncher to find the probabilities comparing to the z-scores.
P(189.7 < X < 213) = P(0 < Z < 0.2417) ≈ 0.0939
Therefore, the probability that a single randomly selected value is between 189.7 and 213 is approximately 0.0939.
To find the probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213, we use the central limit theorem. Under specific circumstances, the testing dispersion of the example mean methodologies a typical conveyance
Step 1: Calculate the standard error of the mean (σ_m) using the formula:
σ_m = σ / sqrt(n)
σ_m = 96.7 / sqrt(62) ≈ 12.2878
Step 2: Convert the given qualities to z-scores utilizing the equation:
z = (x - μ) / σ_m
For 189.7:
z1 = (189.7 - 189.7) / 12.2878 = 0
For 213:
z2 = (213 - 189.7) / 12.2878 ≈ 1.8967
Step 3: Utilize a standard typical conveyance table or mini-computer to find the probabilities relating to the z-scores.
P(189.7 < M < 213) = P(0 < Z < 1.8967) ≈ 0.9702
Therefore, the probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213 is approximately 0.9702.
For more such questions on probability, click on:
https://brainly.com/question/7965468
#SPJ8
Someone help me please

Answers
Answer:
formulas below
Step-by-step explanation:
•Area (A) of a regular polygon
A=(1/2)aP
where a is the length of the apothem and P is the perimeter.
•Apothem=S÷2tan(180/n)
a=apothem
S=one side length of the polygon
n=number of sides of the polygon
•Perimeter=(number of sides of the polygon × length of one side)
Note that the answer for the perimeter is in cm and the answer for the area of the polygon is in units
BOOKS Eduardo is writing a historical novel. He wrote 16 pages today, bringing his total number of pages written to more than 50. How many pages p did Eduardo write before today? Complete the inequality that represents this situation. Then solve the inequality.
Answers
Answer:
p > 34
He wrote more than 34 pages before today.
Step-by-step explanation:
Eduardo wrote 16 more pages, increasing his total number of pages above 50.
p = pages he wrote before today
p + 16 > 50
p > 50 - 16
p > 34
He wrote more than 34 pages before today.
you mix 1/2 quart of blue paint for every 1/3 quart of red paint to make 5 quart of purple paint. How much blue paint and how much red paint do you use?
Answers
The amount of blue paint and red paint that you use will be 3 quarts and 2 quarts, respectively.
What is Algebra?Algebra is the study of abstract symbols, while logic is the manipulation of all those ideas.
The acronym PEMDAS stands for Parenthesis, Exponent, Multiplication, Division, Addition, and Subtraction. This approach is used to answer the problem correctly and completely.
You mix 1/2 quart of blue paint for every 1/3 quart of red paint to make 5 quarts of purple paint.
Let 'x' be the amount of blue paint and 'y' be the amount of red paint. Then the equations are given as,
x / y = (1/2) / (1/3)
x / y = 3/2 ...1
x + y = 5 ...2
From equations 1 and 2, then we have
(3/2) y + y = 5
(5/2) y = 5
y = 2 quart
Then the value of 'x' is given as
x + 2 = 5
x = 3 quart
The amount of blue paint and red paint that you use will be 3 quarts and 2 quarts, respectively.
More about the Algebra link is given below.
https://brainly.com/question/953809
#SPJ1
Given f(x) = 2x – 3, find the x value such that f(x) = 8.
Answers
Answer: 5.5
Step-by-step explanation:
Plugging it into the formula, we get
2x-3 = 8
Add 3 to both sides:
2x = 11
Divide by 2 on both sides:
x = 5.5
What is the volume of a cylinder with a height of 13.6 cm and a base with a radius of 6.6 cm, to the nearest tenth of a cubic centimeter? !!!asap
Answers
Answer:
1,861.1 cubic centimeters
Step-by-step explanation:
Volume of a cylinder is
\(v = \pi {r}^{2}h\)
so you would plug it in and solve
\(v = \pi ({6.6})^{2}13.6\)
-8/9 + (-2)/57
find the absolute value of the following rational number
Answers
The absolute value of the Rational number -474/513 is 474/513.
To find the sum of the rational numbers -8/9 and -2/57, you need to have a common denominator. The least common multiple (LCM) of 9 and 57 is 513. So, you can rewrite the fractions with a common denominator:
-8/9 = (-8/9) * (57/57) = -456/513
-2/57 = (-2/57) * (9/9) = -18/513
Now, you can add the fractions:
-456/513 + (-18/513) = (-456 - 18)/513 = -474/513
To find the absolute value of the rational number -474/513, you simply ignore the negative sign and take the value as positive:
| -474/513 | = 474/513
Therefore, the absolute value of the rational number -474/513 is 474/513.
For more questions on Rational .
https://brainly.com/question/30339525
#SPJ8
Help!!!! Please
Asap

Answers
Answer:
events at A and B are independent because P(A)(B)≠P(A)
what is 2+3x1000
plzzzzzzz help need it so bad
Answers
Answer:
answer: 3002
Hope that helps!!
Answer:
3x^1000+2
Step-by-step explanation:
If there are three boys for every four girls in the chorus what the ratio to represents the relationship between the number of girls in the chorus and the number of students in the chorus.
Answers
Answer:
4/7
Step-by-step explanation:
I think this is the awnser
What is the angle of elevation to a building 1300m away that is 250m high?
•79.1°
•78.9°
•11.1°
•10.9°
Answers
Answer:
∅ = 10.9º
Step-by-step explanation:
tan = opp/adj = 250/1300
∅ = arctan250/1300
use calculator
∅ = 10.9º
Which expression is equivalent to StartRoot 8 x Superscript 7 Baseline y Superscript 8 Baseline EndRoot? Assume x greater-than-or-equal-to 0.
x y squared StartRoot 8 x cubed EndRoot
2 x cubed y cubed StartRoot x y squared EndRoot
2 x cubed y Superscript 4 Baseline StartRoot 2 x EndRoot
4 x cubed y Superscript 4 Baseline StartRoot x EndRoot
Answers
The expression that is equivalent to StartRoot \(8 x^7 y^8\) EndRoot is (\(2 x^3 y^4\) StartRoot 2 x EndRoot)^2.
To understand why this is the case, let's break down each expression and simplify them step by step:
StartRoot \(8 x^7 y^8\) EndRoot:
We can rewrite 8 as \(2^3\), and since the square root can be split over multiplication, we have StartRoot \((2^3) x^7 y^8\) EndRoot. Applying the exponent rule for square roots, we get StartRoot \(2^3\) EndRoot StartRoot \(x^7\) EndRoot StartRoot \(y^8\) EndRoot.
Simplifying further, we have 2 StartRoot \(2 x^3 y^4\) EndRoot StartRoot \(2^2\) EndRoot StartRoot \(x^2\) EndRoot StartRoot \(y^4\) EndRoot. Finally, we obtain 2 \(x^3 y^4\) StartRoot 2 x EndRoot, which is the expression in question.
(\(2 x y^2\) StartRoot 8 x^3 EndRoot)^2:
Expanding the expression inside the parentheses, we have \(2 x y^2\)StartRoot \((2^3) x^3\) EndRoot. Applying the exponent rule for square roots, we get \(2 x y^2\) StartRoot \(2^3\) EndRoot StartRoot \(x^3\) EndRoot.
Simplifying further, we have \(2 x y^2\) StartRoot 2 x EndRoot. Squaring the entire expression, we obtain (\(2 x y^2\) StartRoot 2 x EndRoot)^2.
Therefore, the expression (\(2 x^3 y^4\) StartRoot 2 x EndRoot)^2 is equivalent to StartRoot \(8 x^7 y^8\) EndRoot.
For more such questions on expression
https://brainly.com/question/1859113
#SPJ8
A commuter airline makes lattes in the galley and sells them to passengers. A regular latte contains a shot of espresso, 1 cup of 2% milk, steamed, and 0.5 cup of whipped cream. The low-fat latte contains a shot of espresso, 1.25 cups of skim milk, frothed, and no whipped cream. The plane begins its journey with 100 shots of espresso, 60 cups of skim milk, 60 cups of 2% milk, and 30 cups of whipped cream. The airline makes a profit of $1.58 on each regular latte and $1.65 on each low-fat latte. Assuming that all lattes that are made can be sold, what would be the ideal mix of regular and low-fat lattes to maximize the profit for the airline?
Answers
Answer:
To maximize profit, the amount of each type of coffees made are;
The number of regular latte made = 52 cups
The number of low-fat latte made = 48 cups
Step-by-step explanation:
The given parameters are;
The number of espresso in a regular latte = 1 shot
The number of cups of 2% milk in regular latte = 1 cup
The amount of whipped cream in a regular latte = 0.5 cup
The number of cups of skim milk in the low-fat latte = 1.25 cup
The number of espresso in a low-fat latte = 1 shot
The amount of whipped cream in a low-fat latte = 0 cup
The number of cups of espresso in the journey = 100 shots
The number of cups of skim milk in the journey = 60 cups
The number of cups of 2% milk in the journey = 60 cups
The amount of profit the airline makes on each regular latte = $1.58
The amount of profit the airline makes on each low-fat latte = $1.65
Let 'x' represent the number regular lattes made and let 'y' represent the number low-fat lattes made, we have;
x ≤ 30/0.5 = 60
x ≤ 60
y ≤ 60
y ≤ 60/1.25 = 48
∴ y ≤ 48
x + y ≤ 100
Therefore, the given that more profit is made from the sale of low-fat latte than for regular latte, the maximum number of low-fat latte should be made
Therefore, the number of low-fat latte made, y = 48
Therefore, the number of regular latte made, x ≤ 100 - 48 = 52
For maximum profit, the maximum number of low-fat latte, 'x', should be made
Therefore, x = 52
The ideal mix of regular and low-fat lattes to maximize profit is 52 cups of regular latte and 48 cups of low-fat latte