please help!!! Your boss hands you a memo with a summary of the monthly data. The number of imports is shown as f(x), and the number of exports is shown as g(x). Use the data in the table below, representing both functions, to explain to your boss the solution to the system of equations and what that solution represents.
Month f(x) = No. of imports g(x) = No. of exports
January (1). 2 3
February (2) 4 4
March (3) 6 5
April (4) 8 6
Answers
Step-by-step explanation:
To find the solution to the system of equations represented by the data in the table, we need to first write out the equations. Let's call the number of imports in a given month "x" and the number of exports "y". Then we have:
For January: 2x + 3y = total trade
For February: 4x + 4y = total trade
For March: 6x + 5y = total trade
For April: 8x + 6y = total trade
Related Questions
a library has 67,982 books. the children's section represents 1/3 of the total books. of the remaining books, 75% are fiction. how many books are in the non-fiction section?
Answers
Answer:
11331 non fiction books
Step-by-step explanation:
Given :
Total books = 67982
Childrens section = 1/3 of total
Number of children's books :
(1/3 * 67982) = 22660 books (approximately)
Books left :
67982 - 22660 = 45,322 books
Fiction books:
75% of remaining
0.75 * 45322
33991.5
= 33991 books
If the only section left is non - fiction, then
Non - fiction books = (45322 - 33991) = 11331
A ladder of length (2x+6) feet is positioned x feet from a wall. If the ladder reaches a height of (2x+4) feet along the wall. Find the longest leg.
A. 10ft
B. 24ft
C. 26ft
D. 13cm
Answers
Using the Pythagoras theorem, the longest leg has the length of 24 feet.
Given that,
A ladder of length (2x+6) feet is positioned x feet from a wall.
Height of the ladder = (2x + 6) feet
Distance of ladder from the wall = x feet
Height of the wall that the ladder is placed = (2x + 4) feet
These three lengths form s right triangle where (2x + 6) feet is the hypotenuse.
Longest leg is (2x + 4) feet
Using the Pythagoras theorem,
(2x + 6)² = (2x + 4)² + x²
4x² + 24x + 36 = 4x² + 16x + 16 + x²
4x² + 24x + 36 = 5x² + 16x + 16
x² - 8x - 20 = 0
(x - 10) (x + 2) = 0
x = 10 or x = -2
x = 2 is not possible.
So x = 10
Longest leg = 2x + 4 = 20 + 4 = 24 feet
Hence the length of the longest leg is 24 feet.
Learn more about Pythagoras theorem here :
https://brainly.com/question/343682
#SPJ1
How many square millimeters are in 6 millimeters?
Answers
Answer:
0.0093 square inches
Step-by-step explanation:
Please help asap !!! I will give points !!!

Answers
The value of p when q is 2 is 1
What is inverse variation?Inverse variation is the relationships between variables that are represented in the form of y = k/x, where x and y are two variables and k is the constant value.
This means that has x increases y will decrease and vice versa.
If p is inversely proportional to q , then p = kq
when p = 2, q = 4
2 = k4
k = 2/4
k = 1/2
When q = 2
p = 1/2 × 2
p = 1
Therefore the value of p is 1
learn more about inverse variation from
https://brainly.com/question/13998680
#SPJ1
How tall is the tree?
5ft
35°
-20ft-
[?]ft
Round to the nearest foot.
Answers
Answer:
19
Step-by-step explanation:
tan35=x/20
20tan35=x
x=14.0041507641942
x+5=19.0041507641942
The nearest foot is 19
help please and thank you

Answers
Answer:
1) 9x
2) 3b
3) 3m
4) 5a
5) 7x
6) 3a + 5b
7) 9x - 4
8) 2b + m
9) 13a + b + 6c
10) 9b + 1
11) 7p
12) 2a + b -10
Step-by-step explanation:
A designer has designed different tops, pants, and jackets to create outfits. How many different outfits can the models wear if she has designed the following pieces?
seven tops, two pants, eight jackets
There are a total of different outfits
Answers
The most appropriate choice for combination will be given by-
Models can wear 112 different types of outfit.
What is combination?
Combination determines the number of ways of selecting some number of particular items from a group of given item, here the order of selection do not matter.
If there are n objects and from there, r objects are chosen, number of ways will be given by
\(n \choose r\) = \(\frac{n!}{(n - r)! \times r!}\)
Here,
Number of tops = 7
Number of pants = 2
Number of jackets = 8
From 7 tops the designer can choose 1 top in \(7 \choose 1\) ways
= \(\frac{7!}{(7-1)!\times 1 !}\)
= \(\frac{7!}{6!}\)
= \(7\)
From 2 pants the designer can choose 1 pant in \(2 \choose 1\) ways
= \(\frac{2!}{(2-1)!\times 1 !}\)
= \(\frac{2!}{1!}\)
= \(2\)
From 8 jackets the designer can choose 1 jacket in \(8 \choose 1\) ways
= \(\frac{8!}{(8-1)!\times 1 !}\)
= \(\frac{8!}{7!}\)
= \(8\)
Total number of different outfits models can wear = \(7 \times 2 \times 8\)
= 112
To learn more about combination, refer to the link:
https://brainly.com/question/11732255
#SPJ9
Five houses in the neighborhood sold this month for the following prices:
$328,000, $297,500, $415,000, $389,000, $345,000.
The house with the highest selling price sold for how much more than the median selling price?
Answers
Answer:
70,000
Step-by-step explanation:
Median= 345,000
Higest selling house= 415,000
415,000-345,000= 70,000
i need help!!!! does anyone know this..!!???

Answers
The period of oscillation is 3 seconds
What is period of oscillation?A Oscillation is the periodic change of a measure around a central value or between two or more states, usually in time.
The time taken for an oscillating particle to complete one cycle of oscillation is known as the Period of oscillating particle. It is measured in seconds
Oscillation can also be vibration or revolution or cycle.
Therefore, using the graph to determine the period. Then the wave particle made a complete oscillation at 3 second.
This means that the period of the particle is 3 seconds.
learn more about period of oscillation from
https://brainly.com/question/22499336
#SPJ1
Find the next two terms in the sequence.
6a-3, 7a-4, 8a-5, 9a-6,
O 10a - 7, 11a - 8
O 10a - 5, 11a - 4
O 8a - 7, 7a-8

Answers
5x-3(-5y+6x) +4y i need help please
Answers
To simplify the expression \(\displaystyle\sf 5x-3(-5y+6x)+4y\), we can follow the order of operations, which involves simplifying the expressions within parentheses, applying the distributive property, and combining like terms.
Using \(\displaystyle\sf \) tags for formatting, the expression becomes:
\(\displaystyle\sf 5x-3(-5y+6x)+4y\)
First, we simplify the expression within the parentheses:
\(\displaystyle\sf 5x-3(-5y+6x)+4y\)
\(\displaystyle\sf 5x+15y-18x+4y\)
Next, we can combine the like terms:
\(\displaystyle\sf 5x-18x+15y+4y\)
\(\displaystyle\sf -13x+19y\)
Therefore, the simplified expression is \(\displaystyle\sf -13x+19y\).
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\underline{\textcolor{red}{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
please choose a method to apply to each problem and solve for all of the variables.leave answer in radical form

Answers
Answer
Inverse trig will be used to solve for this unknown angle.
Unknown angle = 32.6°
Explanation
In a right-angle triangle, the side opposite the right angle is the Hypotenuse, the side opposite the given angle that is non-right angle is the Opposite and the remaining side is the Adjacent.
For this question,
Hypotenuse = 38
Opposite = ?
Adjacent = 32
So, to get the angle marked, we will use inverse-trig to say CAH means
Cos x = (32/38)
x = Cos⁻¹ (32/38)
x = 32.6°
Hope this Helps!!!
Please answer correctly with complete solution

Answers
1.)
a. The probability that a randomly selected value will be greater than 1,550 is 0.0026 or about 0.26%.
b. The probability that a randomly selected value will be less than 1,430 is 0.00005 or about 0.005%.
c. The probability that a randomly selected value will be between 1,380 and 1,620 is approximately 1 or 100%.
d. The lowest value of the upper 35% of the random variable is approximately 1,507.93.
2.)
a. The area below 80 is approximately 0.6293 or 62.93%.
b. The area below 95 is approximately 0.9082.
c. The area below 68 is approximately 32.12%.
d. The area below 99 is approximately 5.48%.
What is probability?
In mathematics, probability is a measure of the likelihood or chance of an event occurring. It is a number between 0 and 1, where 0 means that the event is impossible and 1 means that the event is certain.
1)
a. We need to find the probability that a randomly selected value will be greater than 1,550. We can use the standard normal distribution to calculate this probability. First, we need to standardize the variable X using the formula:
Z = (X - μ) / σ
where X is the variable, μ is the mean, and σ is the standard deviation.
So,
Z = (1550 - 1500) / 18 = 2.78
Using a standard normal distribution table or calculator, we find that the probability of a Z-score being greater than 2.78 is approximately 0.0026.
Therefore, the probability that a randomly selected value will be greater than 1,550 is 0.0026 or about 0.26%.
b. Similarly, we can find the probability that a randomly selected value will be less than 1,430 by standardizing the variable and using the standard normal distribution table or calculator.
Z = (1430 - 1500) / 18 = -3.89
Using the standard normal distribution table or calculator, we find that the probability of a Z-score being less than -3.89 is approximately 0.00005.
Therefore, the probability that a randomly selected value will be less than 1,430 is 0.00005 or about 0.005%.
c. To find the probability that a randomly selected value will be between 1,380 and 1,620, we first need to standardize the variables.
Z1 = (1380 - 1500) / 18 = -6.67
Z2 = (1620 - 1500) / 18 = 6.67
Using the standard normal distribution table or calculator, we find the probability of a Z-score being less than -6.67 is very close to 0 and the probability of a Z-score being greater than 6.67 is also very close to 0. Therefore, the probability of a Z-score being between -6.67 and 6.67 is approximately 1.
Therefore, the probability that a randomly selected value will be between 1,380 and 1,620 is approximately 1 or 100%.
d. We need to find the lowest value of the upper 35% of the random variable. To do this, we need to find the Z-score that corresponds to the 65th percentile (since the upper 35% is the complement of the lower 65%).
Using the standard normal distribution table or calculator, we find that the Z-score corresponding to the 65th percentile is approximately 0.385.
So,
0.385 = (X - 1500) / 18
Solving for X, we get:
X = 1500 + (0.385 x 18) = 1507.93
Therefore, the lowest value of the upper 35% of the random variable is approximately 1,507.93.
2.)
a. The standard value for X = 80 can be calculated as follows:
Z = (X - μ) / σ
Z = (80 - 75) / 15 = 0.33
The area below 80 can be found using the standard normal distribution table or calculator.
P(Z < 0.33) = 0.6293
Therefore, the area below 80 is approximately 0.6293 or 62.93%.
b. For X = 95, we can calculate the standard value and find the area below 95 using the same method as in part (a).
Z = (95 - 75) / 15 = 1.33
Using a standard normal distribution table or calculator, we can find the area below 95 to be approximately 0.9082. Therefore, the answer is:
b. The area below 95 is approximately 0.9082.
c. For X = 68, we can calculate the standard value and find the area above 68 as follows:
Z = (68 - 75) / 15 = -0.47
Using a standard normal distribution table or calculator, we can find the area above -0.47, which is equivalent to the area below 0.47, as 0.3212 or approximately 32.12%.
d. For X = 99, we can calculate the standard value and find the area above 99 as follows:
Z = (99 - 75) / 15 = 1.6
Using a standard normal distribution table or calculator, we can find the area above 1.6 as 0.0548 or approximately 5.48%.
Hence,
1.)
a. The probability that a randomly selected value will be greater than 1,550 is 0.0026 or about 0.26%.
b. The probability that a randomly selected value will be less than 1,430 is 0.00005 or about 0.005%.
c. The probability that a randomly selected value will be between 1,380 and 1,620 is approximately 1 or 100%.
d. The lowest value of the upper 35% of the random variable is approximately 1,507.93.
2.)
a. The area below 80 is approximately 0.6293 or 62.93%.
b. The area below 95 is approximately 0.9082.
c. The area below 68 is approximately 32.12%.
d. The area below 99 is approximately 5.48%.
To learn more about the probability, visit:
https://brainly.com/question/24756209
#SPJ1
Marts is solving the equation S=2nrh+2nr2 for h. Which should be the result?
Answers
Step-by-step explanation:
Hope you understand this

A window has an actual height of 4.5 feet. If a blueprint has a scale of 3 inches = 2 feet, find the height of the window in the blueprint. Select one: 9 inches O 7.5 inches O 9.5 inches O 6.75 inches
Answers
2 feet: 3 inches
1 feet: 3/2 inches
4*5 feet: 3x4*5/2
=6.75 Inchea
Answer for brainlest

Answers
Answer:
∠1 = ∠8
Step-by-step explanation:
Because they are ALTERNATE EXTERIOR ANGLE
Answer:
∠1 = ∠8
Step-by-step explanation:
Because alternate exterior angles are equal.
Also there is no angle given so you cant work them out.
simply put:
∠1 = ∠8
∠1 = ∠4
∠3 = ∠2
∠1 = ∠5
∠2 = ∠6
∠2 = ∠7
∠5 = ∠8
∠4 = ∠8
∠7 = ∠6
∠7 = ∠3
Let me know if I missed out on anymore! It was long that's why.
Hope this kind of explained it :)
Prove that if an integer n is the sum of two squares (n = a2+ b2 for a, b Z) then n = 4q or n= 4q+1 or n= 4q + 2 for some qЄ Z. Deduce that 1234567 cannot be written as the sum of two squares.
Answers
The number 1234567 cannot be written as a sum of two squares.
Let q = x^2+y^2,
Let a and b be two even numbers, such that
a= 2x and b = 2y
then
n = (2x)^2 + (2y)^2 = 4 (x^2+y^2)
implies n = 4q
Let a and b be two odd numbers, such that
a = 2x+1 and b = 2y+1
then
n = (2x+1)^2 + (2y+1)^2 = 4x^2+4y^2+1 = 4q+2
Let a be an even number and b be an odd number, such that
a= 2x and b = 2y+1
then
n = (2x)^2 + (2y+1)^2 = 4q+1
But, the given number 1234567 = (4×308641)+3 which is of the form 4q+3, hence it cannot be written as the sum of two squares.
To know more about the even numbers
https://brainly.com/question/2289438
#SPJ4
HELPPPPPP PLEASEEEEEEEEEEEEEEEEEEEEE
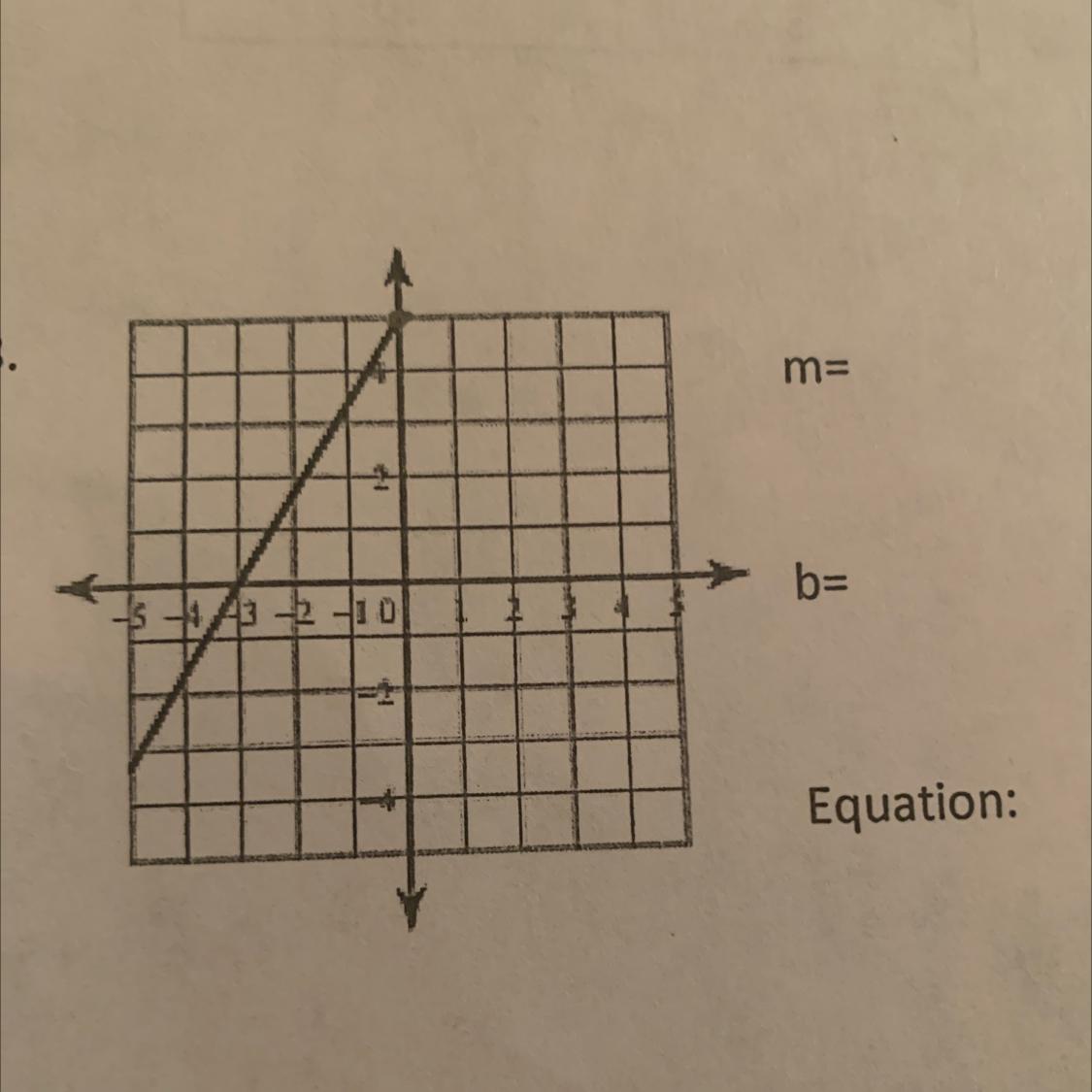
Answers
b = 5
equation = y = 2x + 5
The rule of 72 is used to determine how long it will take an investment to . For an investment of $5,000 earning 7% annually, this will take about years.
Answers
Answer:
10.3 years
Step-by-step explanation:
The Rule of 72 is a rule used to determine the period of time it would take an investment to double at a given fixed annual rate of return. The rule of 72 helps investors to have an estimate of how long an initial investment would double. The rule of 72 is given by the formula:
t = 72 / r
where r is the annual rate of return and t is the approximate time in years required for the investment to double.
t = 72 / r
Given that r = 7%
t = 72 / r = 72 / 7 = 10.3 years
Therefore it would take 10.3 years for an investment of $5,000 earning 7% annually to double to about $10,000
What is the M.A.D. (mean absolute deviation) of the following data set?
8 9 9 7 8 6 9 8
Answers
The mean absolute deviation is 0.75
How to determine the mean absolute deviationTo calculate the mean absolute deviation (M.A.D.), you need to find the average of the absolute differences between each data point and the mean of the data set
From the information given, we have that the data set is;
8 9 9 7 8 6 9 8
Let's calculate the mean, we get;
Mean = (8 + 9 + 9 + 7 + 8 + 6 + 9 + 8) / 8
Mean = 64 / 8
Divide the values
Mean = 8
Let's determine the absolute difference, we get;
Absolute differences=
|8 - 8| = 0
|9 - 8| = 1
|9 - 8| = 1
|7 - 8| = 1
|8 - 8| = 0
|6 - 8| = 2
|9 - 8| = 1
|8 - 8| = 0
Find the mean of the absolute differences:
Average of absolute differences = (0 + 1 + 1 + 1 + 0 + 2 + 1 + 0) / 8
Absolute difference = 6 / 8 = 0.75
Learn more about mean absolute deviation at: https://brainly.com/question/447169
#SPJ1
FIND THE PRODUCT
(x - y)(8x + y)

Answers
Answer:
Step-by-step explanation:
8x^2 + xy - 8xy - y^2
8x^2 - 7xy - y^2
rainer has 12 apples he gave 8 apples to his friend.How many apples does he have now.
Answers
Answer: he has 4 apples now
Step-by-step explanation:
He has 12 apples to begin with and he gives away 8 to his friend
So, 12 - 8
This gives you 4
Answer:
4
Step-by-step explanation:
12 - 8 = 4
To double check: 8 + 4 = 12
Have an amazing day!
PLEASE RATE!!
Jax bought a package of plastic utensils for a picnic. There are a total of 40 utensils,
and 10 of them are knives. What percent of the plastic utensils are knives?
4) Pick the model that represents the problem.
0%
0%
?
Submit
10
10
What percent of the plastic utensils are knives?
100%
40
100%
40
Answers
Answer:
25% were knives
Step-by-step explanation:
The perimeter of the TCL 3-Series Roku Smart TV is 112 inches. The width of the TV is 16 inches greater than its height. Find the width and the height of the TV.
Answers
Answer: Height = 20 inches, Width = 36 inches
Step-by-step explanation:
Let the height be \(h\) and the width be \(h+16\).
Using the formula for the perimeter of a triangle,
\(2(h+h+16)=112\\\\2(2h+16)=112\\\\2h+16=56\\\\2h=40\\\\h=20 \implies h+16=36\)
y= -x - 8x - 16 how many solutions
Answers
Answer:
1 solution
Key:
Assume x is the the range x > 0 ≥ 1, in other words, assume x is equal to 1.
Step-by-step explanation:
\(y = -x - 8x - 16\\= -x - 8x\\= 9x\\= 9x - 16\)
A boy walks 5km due north and then 4km due east. Find the bearing of his current position from the starting point, how far is the boy now from the starting point
Answers
what is the slope of the line

Answers
Answer:
The slope is 1/2.
Step-by-step explanation:
To find the slope of a line, use the equation (y2-y1 / x2-x1)
Let's say (4,3) is x2 and y2. That means (0,-2) is x1 and y1.
(3 - 0) / (4 - -2)
(3 - 0) / (4 + 2)
3/6, which simplifys to 1/2
Computer systems analysts
Database administrators
Computer software engineers,
systems software
Gaming and sports book writers
and runners
Environmental science and
protection technicians, including
health
Manicurists and pedicurists
Physical therapists
Physician assistants
504
650
119 154
350 449
18 24
36
78
47
100
220
29.0
28.6
28.2
28.0
28.0
27,6
27.1
146
34
99
5
10
22
47
18
Bachelor's degree
Bachelor's degree
Bachelor's degree
Short-term on-thi
Associate degree
Postsecondary vocational award
Master's degree
Master's degree
173
66
83
27.0
Based on the above table, what is the fastest growing listed occupation for those without a college degree?
a. Gaming and sports book writers and runners
b. Dental assistants
c. Marriage and family therapists
d. Manicurists and pedicurists
Answers
Based on the above table , the fastest growing listed occupation for those without a college degree is Gaming and sports book writers and runners.
Therefore option A is correct.
What is a college degree?The college degree is described as a qualification awarded to students after completing the requirements for a specific course of study of which the two most common types of degrees are the Bachelor of Arts and Bachelor of Science.
With reference to the table, the occupations that do not require a college degree are:
Gaming and sports book writers and runnersEnvironmental science and protection technicians, including healthManicurists and pedicuristsWe were able to conclude that Based on the above table , the fastest growing listed occupation for those without a college degree is Gaming and sports book writers and runners with a rate of 24.6%.
Learn more about college degrees at: https://brainly.com/question/30008674
#SPJ1
Solve the following systems of inequalities and select the correct graph: 2x − y < 4 x + y < −1 In each graph, the area for f(x) is shaded and labeled A, the area for g(x) is shaded and labeled B, and the area where they have shading in common is labeled AB.
Answers
The solution to the system of inequalities 2x − y < 4 and x + y < −1 is the intersection of the shaded regions below the lines 2x - y = 4 and x + y = -1, labeled AB on the graph. Regions A and B represent the solutions to each individual inequality.
To solve the system of inequalities 2x − y < 4 and x + y < −1, we can use the following steps
Solve each inequality for y in terms of x
2x - 4 < y
-x - 1 < y
Plot the boundary lines for each inequality as dotted lines. To do this, we can use the equality signs instead of the inequality signs.
2x - 4 = y
-x - 1 = y
Shade the region that satisfies each inequality. To do this, we can test a point that is not on the boundary line in each inequality. For example, we can test (0,0) in the first inequality
2(0) - 0 < 4
0 < 4
Since (0,0) satisfies the first inequality, we shade the region below the line 2x - y = 4. Similarly, we can test (0,-2) in the second inequality
(0) + (-2) < -1
-2 < -1
Since (0,-2) satisfies the second inequality, we shade the region below the line x + y = -1.
The solution to the system of inequalities is the intersection of the shaded regions, which is labeled AB in the graph. The shaded region labeled AB is the region that satisfies both inequalities, which is the region below both lines.
To know more about system of inequalities:
https://brainly.com/question/19526736
#SPJ1

Which value of x makes 7+5(×-3)=22
Answers
Answer:
x=6
Step-by-step explanation:
7+5(×-3)=22
expand the brackets:
7 + 5x - 15 = 22
simplify:
5x - 8 = 22
add 8 to both sides:
5x = 30
divide both sides by 5
x = 6
hope this helps
brainliest plz?
x
Answer:
7+5 (x-3)=22
7+5x-15=22
5x+ -8=22
5x=30
x=6
Step-by-step explanation:
PEMDAS
First distribute in the parentheses
Then add like terms together
once you have -8, add it on both sides
then divide 5 from both sides to leave the unknown variable by itself
Finally you have the answer
I hope this helps! :)