Answers
Step-by-step explanation:
by complete angle
x+38+52+220=360
x+310=360
x=360-310
x=50
Related Questions
Peter is going to carpet a room that measured 9 x 13 feet. The carpet costs $18.75 a square foot. How much will peter spend
Answers
Answer:
$2193.75
Step-by-step explanation:
9 times 13
times 18.75
Given: f(x) = 2x-1 and g(x) = 2x-3
Find:
(fog)(x)
HELP PLEASEE!!
Answers
Answer:
(fog)(x) = 4x - 7
Step-by-step explanation:
f(x) = 2x-1 and g(x) = 2x-3;
(fog)(x) = f(g(x)
= f(2x - 3) = 2(2x - 3) - 1
= 4x - 6 - 1
= 4x - 7
find the equation of the line parallel to H passes through (-4,2)

Answers
Answer:
y = \(\frac{1}{3}\) x + \(\frac{10}{3}\)
Step-by-step explanation:
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
Calculate the slope m of H using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (0, - 3) and (x₂, y₂ ) = (3, - 2) ← 2 points on the line
m = \(\frac{-2+3}{3-0}\) = \(\frac{1}{3}\)
Parallel lines have equal slopes, thus
y = \(\frac{1}{3}\) x + c ← is the partial equation
To find c substitute (- 4, 2) into the partial equation
2 = - \(\frac{4}{3}\) + c ⇒ c = 2 + \(\frac{4}{3}\) = \(\frac{10}{3}\)
y = \(\frac{1}{3}\) x + \(\frac{10}{3}\) ← equation of parallel line
Assume that in a given year the mean mathematics sat score was 572, and the standard deviation was 127. a sample of 72 scores is chosen. What is the probability that the sample mean score is less than 567?
Answers
According to the Central Limit Theorem, for a sufficiently large sample size, the distribution of sample means will be approximately normal, regardless of the shape of the population distribution.
To calculate the probability, we need to convert the sample mean score to a z-score and then find the corresponding area under the standard normal distribution curve.
Calculate the standard error of the mean (SE):
SE = σ / sqrt(n)
SE = 127 / sqrt(72)
SE ≈ 14.96
Calculate the z-score:
z = (X - μ) / SE
z = (567 - 572) / 14.96
z ≈ -0.334
Find the probability corresponding to the z-score:
We can find the area to the left of the z-score -0.334. Let's denote this probability as P(z < -0.334).
The probability that the sample mean score is less than 567 is approximately equal to P(z < -0.334).
Learn more about Central Limit Theorem here : brainly.com/question/898534
#SPJ11
Starting with a1, write an explicit formula for the sequence below. 2,5, 10, 17, 26, an=_________
Answers
The explicit formula for the given sequence is an = 2 + n².
To find the explicit formula for the given sequence, we need to identify the pattern or rule that generates the terms. By observing the sequence, we can see that each term is obtained by adding consecutive odd numbers to the previous term.
Let's break it down step by step:
The first term, a1, is given as 2.
To obtain the second term, a2, we add 3 (the first odd number) to a1: 2 + 3 = 5.
To obtain the third term, a3, we add 5 (the second odd number) to a2: 5 + 5 = 10.
To obtain the fourth term, a4, we add 7 (the third odd number) to a3: 10 + 7 = 17.
To obtain the fifth term, a5, we add 9 (the fourth odd number) to a4: 17 + 9 = 26.
From the observations above, we can see that each term can be obtained by adding the sum of the first n odd numbers (1, 3, 5, 7, ...) to the first term, a1.
The sum of the first n odd numbers is given by the formula: n^2.
Therefore, the explicit formula for the given sequence is:
an = a1 + n²
Substituting the given value of a1 = 2 into the formula, we have:
an = 2 + n²
So, the explicit formula for the given sequence is an = 2 + n².
To know more about sequence check the below link:
https://brainly.com/question/6561461
#SPJ4
An ice-skating rink hosts different events on different days of the week they keep track of ages of the people who attended the event in summarize the data on the box plots below which box plot shows data that are skewed to the left
Answers
Answer: Aerobic class
Step-by-step explanation:
I’m not sure but I believe skewed to the left means having a longer tail so that’s the one the has the longer tail to the left.
The data on the box plots showing data that are skewed to the left will have the longer part to the left of the median.
What is a box plot?A box plot is a graphical plot of data in are form of a box.
The box plot shows a five number summary of data in th plot:
The minimum,The first quartile (Q1),The median,The third quartile (Q3),and The maximumA left skewed box plot have more data to the left of the median.
Therefore, the data on the box plots showing data that are skewed to the left will have the longer part to the left of the median.
Learn more about box plot at: https://brainly.com/question/14277132
#SPJ2
Help me in the second question please
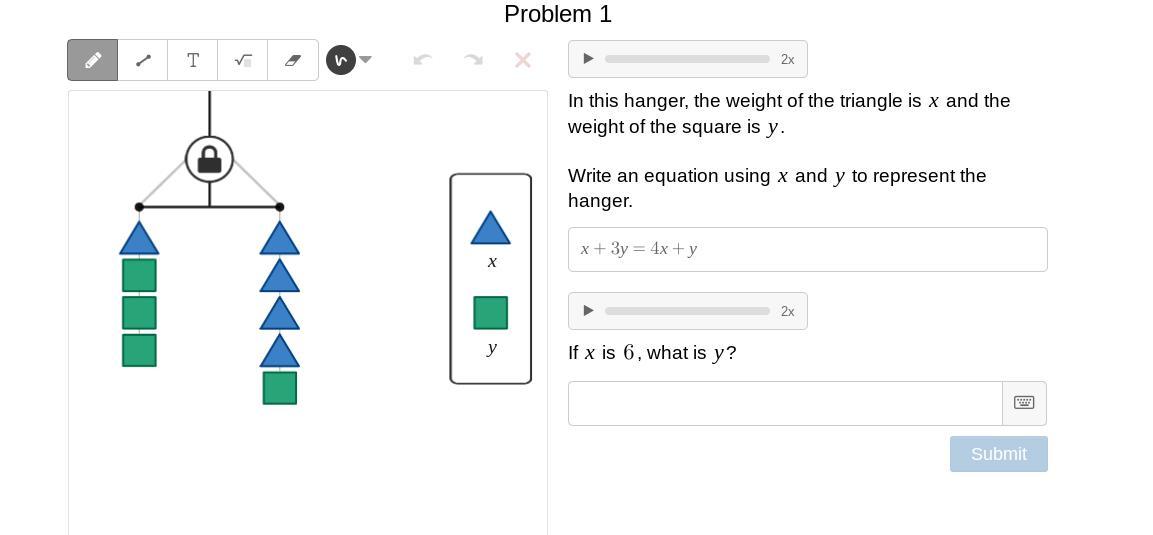
Answers
The equation representing the hanger is 3x - 2y = 0 and when x = 6, y = 9
What is an equation?An equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign =.
Given that, the weight of the square is y
And, the weight of the triangle is x
Now, the equation according to the diagram is;
x + 3y = 4x + y
4x - x + 3y - y = 0
3x - 2y = 0
Now, when, x = 6
So, y =
3x - 2y = 0
3x = 2y
3(6) = 2y
18 = 2y
y = 9
Hence, the equation representing the hanger is 3x - 2y = 0 and when x = 6, y = 9
Learn more about equations, click;
https://brainly.com/question/29657988
#SPJ1
4x^2 + 12xy + 9y^2
Can someone answer this and show the work?
Answers
ANSWER:
=(2x+3y)^2
WORKINGS:
4x^2+12xy+9y^2
2 =(2x)^2
2 +2(2x)(3y)+(3y)^2
2 =(2x+3y)^2
Chris bought 12.4 gallons of gasoline. There are approximately 3.8 liters in 1 gallon. Which measurement is closetsto the number of liters of gasoline Chris bought? A 11.44 L B 2.74 L C 47.12 L D 14.20 L2
Answers
Answer:
C 47.12 L
Step-by-step explanation:
1 gallon = 3.8 liters
12.4 gallons = x
Cross Multiply
= 12.4 × 3.8
47.12 liters
Option C is correct
What is 86 Inches in Feet?
Answers
86 inches are equivalent to 7.17 feet
To convert inches to feet, you can divide the number of inches by 12 (since there are 12 inches in a foot):
86 inches ÷ 12 inches/foot = 7.17 feet
The units of measurement used to measure length or distance are inches and feet. One inch is equivalent to one-twelfth of a foot, while one foot is equal to twelve inches. As a result, you may easily convert feet to inches by multiplying the number of feet by 12. 36 inches, for instance, is equivalent to 3 feet (3 feet x 12 inches/foot).
Divide the number of inches by 12 to convert the measurement to feet. As an illustration, 24 inches (12 inches per foot) equals 2 feet.
Inches and feet are often used to measure an object's height, breadth, and length in the United States.
For more such questions on measurement
https://brainly.com/question/777464
#SPJ4
let y=4tanx. (a) find the differential dy= 4(sec^2x) dx. (b) evaluate dy and δy when x=π/4 and dx=−0.3 dy= and δy=
Answers
When x = π/4 and dx = -0.3, dy = -2.4 and δy = 2.4. δx is an infinitesimal change in x
To find the differential of y, we differentiate y = 4tanx with respect to x. Using the derivative rules, we have dy = 4(sec^2x)dx.
To evaluate dy and δy when x = π/4 and dx = -0.3, we substitute these values into the expression for dy.
When x = π/4, sec^2(π/4) = 2, so dy = 4(2)dx = 8dx.
Given that dx = -0.3, we can calculate dy as follows: dy = 8(-0.3) = -2.4.
To evaluate δy, we use the fact that δx is an infinitesimal change in x. Therefore, δx = 0.3.
Using δy = dy = 4(sec^2x)δx, we substitute x = π/4 and δx = 0.3: δy = 4(2)(0.3) = 2.4.
Learn more about differential here:
https://brainly.com/question/31383100
#SPJ11
estimate [infinity] n = 1 (2n 1)−9 correct to five decimal places.
Answers
Using the formula for an infinite geometric series, the sum of the given series can be estimated as S ≈ 6.00000.
To estimate the sum of the series n = 1 to infinity of (2n+1) - 9, we can use the formula for an infinite geometric series:
S = a / (1 - r)
where S is the sum of the series, a is the first term, and r is the common ratio. In this case, a = (2(1)+1) - 9 = -6 and r = 2.
Thus, we can estimate the sum as:
S ≈ -6 / (1 - 2) = 6
To express this answer correct to five decimal places, we would write:
S ≈ 6.00000
Learn more about geometric series here: brainly.com/question/4617980
#SPJ11
let m be the number of units to make and b be the number of units to buy. if it costs $2 to make a unit and $3 to buy a unit and 4000 units are needed, the objective function is min 4000 (m b) max 8000m 12000b min 2m 3b max 2m 3b
Answers
The objective function is "min 2m + 3b" which represents the cost of making m units and buying b units. To find the optimal solution, we need to minimize this cost. To begin, we are given that the total number of units needed is 4000. This implies that m + b = 4000.
Now, let's solve for m and b separately.
1. Solving for m:
We want to minimize the cost of making m units, which costs $2 per unit. Therefore, the cost of making m units is 2m dollars.
2. Solving for b:
We want to minimize the cost of buying b units, which costs $3 per unit. Therefore, the cost of buying b units is 3b dollars.
To summarize:
- The cost of making m units is 2m dollars.
- The cost of buying b units is 3b dollars.
- The total number of units needed is 4000, so m + b = 4000.
The objective function "min 2m + 3b" represents the total cost. We want to minimize this cost.
Let's learn more about function:
https://brainly.com/question/11624077
#SPJ11
Find the vertex of this function and decide whether it is a maximum point or a minimum point. Also, lable what point it's specifically at.

Answers
Okay, here we have this:
Considering the provided graph we are going to identify the requested vertex and decide whether it is a maximum point or a minimum , so we obtain the following:
Let us remember that the lowest or highest point is the vertex of the parabola. In this case we can see that the highest point is (-1, 9). So the vertex is (-1, 9), and since it is the highest, then it will be a maximum.
In the image you can see the vertex drawn in blue.

T/F the proportion of the variation in the dependent variable y that is explained by the estimated regression equation is measured by the .
Answers
The proportion of the variation in the dependent variable y that is explained by the estimated regression equation is measured by the coefficient of determination.
What is coefficient of determination?
The effectiveness of a statistical model in forecasting a result is shown by the coefficient of determination (R²). The dependent variable in the model is a representation of the result.
R² can have a value of 0 or 1, with 1 being the maximum achievable. Simply said, a model's R² will be closer to 1 the more accurate its predictions are.
R² is a more precise measure of goodness of fit. The amount of volatility in the dependent variable that the model can account for.
You can typically tell whether your linear regression data's R² is high or low by graphing it. The graphs below, for instance, display two sets of simulated data:
Dots represent the observations.
The line of best fit, or predictions of the model, is displayed as a black line.
Purple lines represent the deviations (the residuals) between the data and their expected values.
To learn more regression equation, click on below link:
https://brainly.com/question/14184702
#SPJ4
How long is pi?
pi
pi
pi
pi
pi
pi
Answers
Answer:
here is the pi numbers these are the first 100 digits
Step-by-step explanation:
3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679 ...
Partial derivatives Find the first partial derivatives of the function.g(x,y,z) = 6x²yz³ - 7x/y
Answers
Consequently, the following are the first partial derivatives of g(x,y,z) = 6x2yz3 - 7x/y:
G/x equals (12xyz3) - (7/y).
G/Y equals (6x2z3) - (7x/y2)
∂g/∂z = (18x²y²z²)
To find the first partial derivatives of the function g(x,y,z) = 6x²yz³ - 7x/y, we need to take the partial derivative of the function with respect to each of its variables, one at a time.
Let's start with x:
The partial derivative of g with respect to x is found by treating y and z as constants and differentiating 6x²yz³ and 7x with respect to x.
So,
∂g/∂x = (12xyz³) - (7/y)
Next, let's take the partial derivative with respect to y:
The partial derivative of g with respect to y is found by treating x and z as constants and differentiating 6x²yz³ and 7x/y with respect to y.
So,
∂g/∂y = (6x²z³) - (7x/y²)
Finally, let's take the partial derivative with respect to z:
The partial derivative of g with respect to z is found by treating x and y as constants and differentiating 6x²yz³ with respect to z.
So,
∂g/∂z = (18x²y²z²)
To learn more about : derivatives
https://brainly.com/question/30396691
#SPJ11
6th GRADE MATH:
Noah bought 2.5 kilograms of colored tile chips for a mosaic art project. What is the mass of the chips in grams?
Answers
Kilo means a thousand so you multiply the 2.5 by 1,000
Answer:
2500 grams
Step-by-step explanation:
to go from kilogram to grams, u should multiply the kilograms by 1000 to get the grams
Please Help! I'm very stuck on this last question that I'm on, dose anyone mind if they could help me out please?

Answers
1. None of these
---We cannot classify angles which are not on parallel lines intersected by a transversal.
2. Alternate interior angles
---These angles are on the inside of the parallel lines (interior) and on opposite (alternate) sides of the transversal.
3. Corresponding Angles
---These angles are in the same relative position. This makes them corresponding angles.
4. Alternate Exterior Angles
---These angles are on opposite (alternate) sides of the transversal and outside (external) of the parallel lines.
5. Corresponding Angles
---These angles are in the same relative position, which makes them corresponding.
Hope this helps!
Need help on this question. What is the value of 1500 ÷ ( 6^2 +4^3) • 37?

Answers
GIVEN:
We are given the expression shown below;
\(1500\div(6^2+4^3)\cdot37\)Required;
To find the value of the expression;
Step-by-step solution;
We begin by simplifying the parenthesis as follows;
\(\begin{gathered} (6^2+4^3) \\ \\ =36+64 \\ \\ =100 \end{gathered}\)Now we re-write the expression;
\(\begin{gathered} 1500\div(100)\cdot37 \\ \\ =\frac{1500}{100}\times37 \\ \\ =15\times37 \\ \\ =555 \end{gathered}\)ANSWER:
The value of the expression is 555
Which statement is not true of histograms?
The number of intervals tells you the size of the data set.
An outlier will show as a gap in the data.
Each interval must be the same size.
The data should be sorted before the graph is made.
Answers
The statement "The number of intervals tells you the size of the data set" is not true of histograms. Option A
Characteristics of histogramsThe number of intervals, or bins, in a histogram is determined by the data and by the preferences of the person creating the graph. A larger number of intervals will result in a graph with more detail, but may also make it harder to see trends or patterns in the data.
The size of the data set is not determined by the number of intervals in a histogram, but by the number of data points that are being represented.
The other statements are true of histograms. An outlier can show as a gap in the data if it falls outside the range of the intervals being used.
Learn more about histograms at:https://brainly.com/question/28164315
#SPJ1
This recipe makes 15 cupcakes.
Deepak only wants to make 12 cupcakes.
How much of each ingredient does he need?
Recipe: Makes 15 cupcakes
12 ounces butter
10 ounces sugar
15 ounces flour
5 eggs
Answers
8 ounces sugar
12 ounces flour
4 eggs
Simplify to create an equivalent expression.
(3n+6)⋅3+(2n+2)⋅4
Please help as soon as possible. Thanks! =D
Answers
Answer:
i don"t know ask your mom
Answer:
\((9n + 18) + (8n + 8) \\ = (9n + 8n) + (18 + 8) \\ = 17n + 26\)
If the selling price of an article is Rs19000 and 13 percent Vat is levied on it. How much should a customer pay for it?
Answers
The price that the customer pays after the VAT is Rs. 21,470.
In the question, we are given that the selling price of an article is Rs. 19000, and 13% VAT is levied on it.
We are asked to find the price that the customer pay.
The total price that the customer pays is the sum of the selling price and the VAT that he/she needs to pay.
The selling price is given to be Rs. 19000.
The VAT is 13%, that is, 13% of Rs. 19000 = Rs. 0.13*19000 = Rs. 2470.
Thus, the total price that the customer pays = The selling price + The VAT,
or, the total price that the customer pays = Rs. 19000 + Rs. 2470 = Rs. 21470.
Thus, the price that the customer pays after the VAT is Rs. 21,470.
Learn more about VAT at
https://brainly.com/question/17220117
#SPJ9
14 POINTS AND BRAINLIEST HELP IM TAKING A TIMED TEST!! SHOW ALL WORK

Answers
Answer:
-1.5Step-by-step explanation:
Solving in steps
(x-2)/7 = (2x+1)/44(x-2) = 7(2x +1)4x - 8 = 14x + 714x - 4x = -8 - 710x = - 15x = -15/10x = -1.5The ratio of men to women working for a company is 7:5. If there are 192 employees total, how many women work for the company?
Answers
7:5 mean that to every 7 men there is 5 woman.
There are 54 women working for the company.
What is Ratio?The ratio is defined as a relationship between two quantities, it is expressed as one divided by the other.
The ratio of men to women working for the company is 7:5, which means that for every 7 men, there are 5 women.
We can express this as a fraction by writing 7/7 as 1 and 5/7 as 5/7, so the ratio becomes 1:5/7.
This fraction can be rewritten as 7/7:35/7, and since the two fractions have the same denominator, we can say that the ratio is equal to 7:35.
Since there are 192 total employees, and the ratio of men to women is 7:35, we can set up the following proportion:
7/35 = x/192
We can solve this proportion by cross-multiplying:
7/35 × 192 = x
This simplifies to:
x = 54
Therefore, the company hired 54 female employees.
To learn more about the Ratio click here:
brainly.com/question/1504221
#SPJ2
what is the explicit rule for the nth term of the geometric sequence? 5, 20, 80, 320, 1,280, …
Answers
To determine the explicit rule for the nth term of the given geometric sequence 5, 20, 80, 320, 1,280, ..., use the formula:`an = a1 * r^(n - 1)`, where an represents the nth term of the sequence.`a1`represents the first term of the sequence.`r` represents the common ratio between terms of the sequence.
The given sequence is 5, 20, 80, 320, 1,280, ...Let's now apply the formula to find the explicit rule for the nth term of the given sequence.`an = a1 * r^(n - 1)`Where a1 = 5 (first term of the sequence)`r` can be found by dividing any term by the preceding term, as `r = (second term) / (first term)`In this case,`r = (20) / (5) = 4`
Substituting the values in the formula:`an = a1 * r^(n - 1)``an = 5 * 4^(n - 1)` Therefore, the explicit rule for the nth term of the given geometric sequence is `an = 5 * 4^(n - 1)`.You can use this formula to find the value of any term in the sequence, given its position (n) in the sequence.For example, to find the 150th term of the sequence, substitute n = 150 in the formula: `a150 = 5 * 4^(150 - 1)`. This simplifies to `a150 = 1.442 x 10^90`.
Learn more about geometric sequence
https://brainly.com/question/27852674
#SPJ11
O Personal math Iainer23 4 596 7 810VieFind the sum. You may find using a number line to be helpful. Express your answer as a simplified mixednumber, if necessary.Ste3+1/2VidТехThe result isPrit
Answers
We will find the sum of 3+ 1 1/2 with help of a
What is the proper coefficient for water when the following equation is completed and balanced for the reaction in basic solution?C2O4^2- (aq) + MnO4^- (aq) --> CO3^2- (aq) + MnO2 (s)
Answers
The proper coefficient for water when the equation is completed and balanced for the reaction in basic solution is 2.
A number added to a chemical equation's formula to balance it is known as coefficient.
The coefficients of a situation let us know the number of moles of every reactant that are involved, as well as the number of moles of every item that get created.
The term for this number is the coefficient. The coefficient addresses the quantity of particles of that compound or molecule required in the response.
The proper coefficient for water when the equation is completed and balanced for the chemical process in basic solution is 2.
Learn more about coefficient, here:
https://brainly.com/question/13018938
#SPJ1
Which list is in order from least to greatest
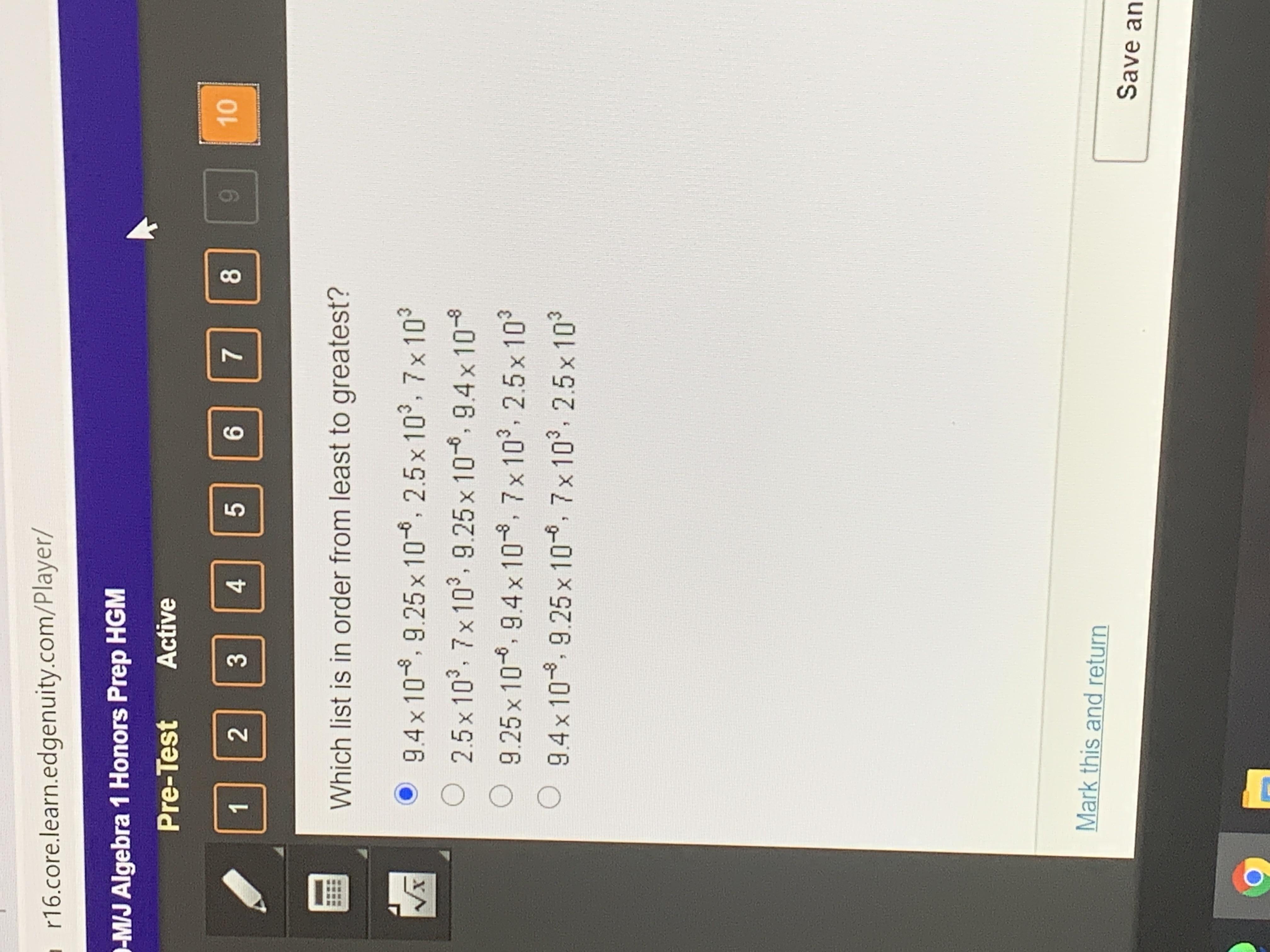
Answers
The correct answer to this question is A. 9.4 x \(10^{-8}\), 9.25 x \(10^{-6}\), 2.5 x \(10^{3}\), 7 x
Explanation:
These numbers are written in scientific notation. In this system, the exponent shows the number of spaces the period has been moved to make the number shorter. Additionally, a positive exponent shows the number has a certain number of digits to the right, while a negative exponent shows the number has digits to the left. In this way, the numbers presented can be converted as:
9.4 x \(10^{-8}\) = 0.000000094 (Exponent indicates eight spaces to the left)
9.25 x \(10^{-6}\) = 0.00000925 (Exponent indicates six spaces to the left)
2.5 x \(10^{3}\) = 2500 (Exponent indicates three spaces to the right)
7 x \(10^{3}\) = 7000 (Exponent indicates three spaces to the right)
