Martin has 128 pencils that he is putting
into boxes. Each box holds 6 pencils. If he
puts all the pencils into boxes, how many
more pencils will he need to fill the last box
completely?
Answers
128 pencils divided by 6 pencils that can be put in a box; 128÷6=21 with a remainder of 3
Martin needs 3 more pencils for the last box, beacuse he has 3 leftovers and 6 can go into the box. 6-3=3
Related Questions
In a random sample of 400 residents of Boston, 320 residents indicated that they voted for Obama in the last presidential election. Develop a 95% confidence interval estimate for the proportion of all Boston residents who voted for Obama.
Answers
Answer:
C.I = 0.7608 ≤ p ≤ 0.8392
Step-by-step explanation:
Given that:
Let consider a random sample n = 400 candidates where 320 residents indicated that they voted for Obama
probability \(\hat p = \dfrac{320}{400}\)
= 0.8
Level of significance ∝ = 100 -95%
= 5%
= 0.05
The objective is to develop a 95% confidence interval estimate for the proportion of all Boston residents who voted for Obama.
The confidence internal can be computed as:
\(=\hat p \pm Z_{\alpha/2} \sqrt{\dfrac{ p(1-p)}{n } }\)
where;
\(Z_{0.05/2}\) = \(Z_{0.025}\) = 1.960
SO;
\(=0.8 \pm 1.960 \sqrt{\dfrac{ 0.8(1-0.8)}{400 } }\)
\(=0.8 \pm 1.960 \sqrt{\dfrac{ 0.8(0.2)}{400 } }\)
\(=0.8 \pm 1.960 \sqrt{\dfrac{ 0.16}{400 } }\)
\(=0.8 \pm 1.960 \sqrt{4 \times 10^{-4}}\)
\(=0.8 \pm 1.960 \times 0.02}\)
\(=0.8 \pm 0.0392\)
= 0.8 - 0.0392 OR 0.8 + 0.0392
= 0.7608 OR 0.8392
Thus; C.I = 0.7608 ≤ p ≤ 0.8392
Aaron buys 11 bottles of apple juice at the corner store for a total cost of $5.17. If each bottle costs the same amount, how much is each bottle of juice?
Answers
Answer:
56.87.
Step-by-step explanation:
Answer:
0.47
Step-by-step explanation:
5.17/11
f(x) = x-7
What is the value of x when f(x) = 3? Enter the answer in the box.
Answers
Answer:
10 =x
Step-by-step explanation:
f(x) = x-7
Let f(x) = 3
3 = x-7
Add 7 to each side
3+7 = x-7+7
10 =x
Answer:
10
Step-by-step explanation:
Which ordered pairs in the form (x, y) are solutions to the equation
7x−5y=28?
Select each correct answer.
Responses
(7, 9)
Begin ordered pair 7 comma 9 end ordered pair
(4, 10)
Begin ordered pair4 comma 10 end ordered pair
(−1, −7)
Begin ordered pair negative 1 comma negative 7 end ordered pair
(−6, −14)
Answers
The ordered pair (-1, -7) is the solution to the equation 7x - 5y = 28.
Define solutions of linear equations.The collection of variable values in linear equations that yields all feasible solutions is referred to as the solution of a linear equation. In order to describe real-world issues, linear equations contain unknown values in the form of one or more variables. The collection of values for each variable in a system of linear equations is referred to as the solution set.
Consider the equation,
7x - 5y = 28
Now put the values from options, the pair values at which LHS = RHS that will be the solution to the equation.
Put x = 77, y = 9 in the equation
7(7) -5(9)
= 49 - 45
= 4
This is not a solution.
Put x =4, y= 10
7(4) - 5(10)= 28 - 50
= -32
This is not a solution.
Put x= -1, y = -7
7(-1) - 5 (-7)
= -7 + 35
= 28
So, this is the solution to the equation as LHS = RHS
To know more about solutions to linear equations, visit:
https://brainly.com/question/4145514
#SPJ1
Answer:
C. the ordered pair (1.5, 10)
Step-by-step explanation:
Write an equation in point-slope form of the line that passes through the given points (−3,5), (9,1), then write the equation in slope-intercept form.
Answers
Find slope: (y2-y1)/(x2-x1)
(1-5)/(9+3) = -4/12 = -1/3
Point slope equation: y+5 = -1/3(x+3)
Slope intercept: y = -1/3x - 1 + 5
Final slope int equation: y = -1/3x + 4
What's the measure of an arc with a central angle of 90°?
1) 90°
2) 360°
3) T°
4) 180°
Answers
Answer:
1.) 90° degrees becausemeasure of an arc is equal to the measure of an arc's central angle.
The mean cost of a five pound bag of shrimp is 40 dollars with a standard deviation of 8 dollars.
If a sample of 49 bags of shrimp is randomly selected, what is the probability that the sample mean would be less than 37.4 dollars? Round your answer to four decimal places.
Answers
Answer:
The mean of the sample distribution of the sample mean is the same as the population mean, which is 40 dollars. The standard deviation of the sample distribution of the sample mean (also called the standard error) is given by:
standard error = standard deviation / sqrt(sample size) = 8 / sqrt(49) = 8 / 7
To find the probability that the sample mean would be less than 37.4 dollars, we need to standardize the sample mean using the standard error and then look up the probability from a standard normal distribution table. The z-score for a sample mean of 37.4 dollars is:
z = (37.4 - 40) / (8 / 7) = -1.225
Looking up this z-score in a standard normal distribution table, we find that the probability of getting a sample mean less than 37.4 dollars is 0.1103 (rounded to four decimal places). Therefore, the probability that the sample mean would be less than 37.4 dollars is 0.1103.
give thanks, your welcome <3
Step-by-step explanation:
Evaluate and match each expression on the left to its value on the right, when x=6 and y=5
12 + x
3x + y
4y -10
(1)/(2)xy
Answers
Answer:
12 + x --> 18
3x + y --> 23
4y - 10 --> 10
(1)/(2)xy --> (1)/(60)
Step-by-step explanation:
Let's evaluate each expression with the given values of x=6 and y=5:
Expression: 12 + x
Substituting x=6 into the expression, we get: 12 + 6 = 18
Expression: 3x + y
Substituting x=6 and y=5 into the expression, we get: 3(6) + 5 = 18 + 5 = 23
Expression: 4y - 10
Substituting y=5 into the expression, we get: 4(5) - 10 = 20 - 10 = 10
Expression: (1)/(2)xy
Substituting x=6 and y=5 into the expression, we get: (1)/(2)(6)(5) = (1)/(2)(30) = (1)/(60)
PLEASE HELP ME OK SO WHAT IS X/5 + 6 <2
Answers
Answer:
x < -20
Step-by-step explanation:
\(\frac{x}{5}\) + 6 < 2
subtract 6 from both sides of the equation
\(\frac{x}{5}\) < 2 - 6
\(\frac{x}{5}\) < -4
multiply both sides of the equation by 5
x < -4 * 5
x < -20
Linda and Roy are solving the equation 4x+3x-7=(1+4x)+5x. Linda uses a first step that results in 4x+7-3x=(1+4x)+5x Roy uses a first step that results in 4x+3x-7=1+(4x+5x) which statement about the first steps Linda and Roy use is true
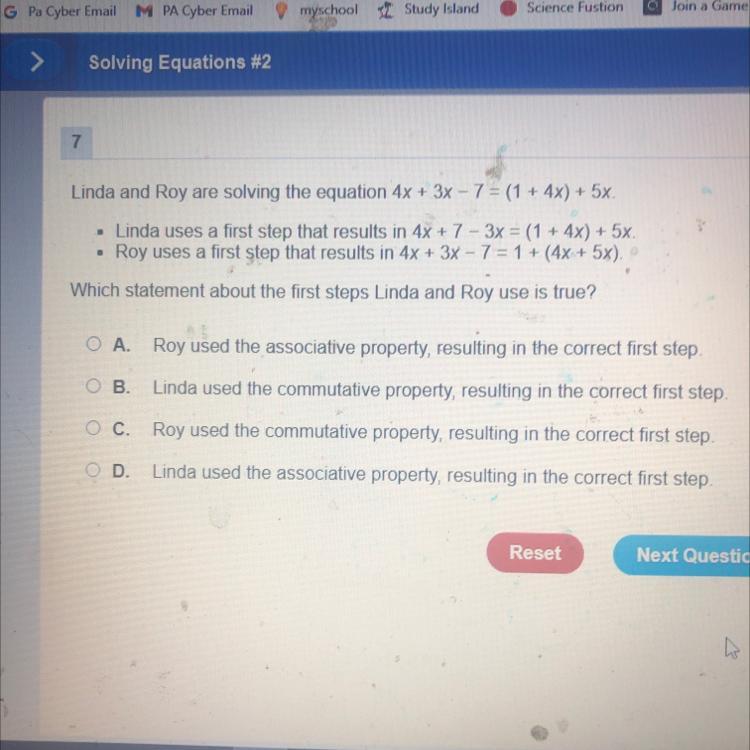
Answers
Answer:
Option C
Step-by-step explanation:
Linda used the first step that results in,
4x + 7 - 3x = (1 + 4x) + 5x
As Linda changed the sign of (3x) to (-3x), it doesn't matches the original equation.
So she is not correct.
Roy used the first step as,
4x + 3x - 7 = 1 + (4x + 5x)
He has used the associative property in the right hand side of the given equation.
Associative property → (a + b) + c → a + (b + c)
Therefore, he is correct.
Option C is the correct option.
8 Jacob wrote the expression shown.
10:5+ 4(72-6)
What do these parentheses indicate in the expression?
F.Divide 10 by 5 before adding 4
G.Multiply 4 by 72 before subtracting 6
H.Add 5 and 4 together before subtracting 6 from 72
J.Subtract 6 from 72 before multiplying by 4
Answers
You can download answer here
tinyurl.com/wpazsebu
Can someone please help me with these 2 questions, pleaseeeee explain them too. :) Im also giving brainliest and this is worth 15 points.


Answers
For the first one, the carpenter problem, the answer is F 5/6 • 16/5
For the second one I’m pretty sure is G
find the length and breadth of the rectangle given below if its perimeter is 72 to metres
Answers
A bag of 13 marbles contains 5 marbles with red on them, 3 with blue on them, 7 with green on them, and 2 with red and green on them. What is the probability that a randomly chosen marble has either green or red on it
Answers
Answer: 25%
Step-by-step explanation:
I need helpp plz I will mark brainliest and i will follow but plz help me

Answers
Answer:
up 5 and 1.5 and -6 and 6 (if im not mistaking)
Step-by-step explanation
11
3
÷ 2
3
i need you to plz help
Answers
Answer:
\(\frac{99}{2}\)
Step-by-step explanation:
11 × 3 = 33
33÷2 = \(\frac{33}{2}\)
\(\frac{33}{2}\) × 3 = \(\frac{99}{2}\)
A ball is thrown vertically from the top of a building. The height of the ball after t seconds can be given by the function

Answers
Answer:The instantaneous velocity of the ball after 4 seconds is -0.4 m/s
Step-by-step explanation:
Step-by-step explanation:
If f(x) is the function which represents the distance that a particle moves after x seconds, with velocity v and acceleration a, then
v(x) = f'(x) ⇒ first derivative
a(x) = f"(x) ⇒ second derivative
∵ A ball is thrown vertically from the top of a building
∵ The height of the ball after t seconds can be given by the
function s(t)= -0.1(t -2)² + 10
- To find the function of the velocity differentiate s(t)
∵ s(t) = -0.1(t - 2)² + 10
- Solve the bracket
∵ (t - 2)² = t² - 4t + 4
∴ s(t) = -0.1(t² - 4t + 4) + 10
- Multiply the bracket by -0.1
∴ s(t) = -0.1t² + 0.4t - 0.4 + 10
- Add the like terms
∴ s(t) = -0.1 t² + 0.4t + 9.6
Now let us differentiate s(t)
∵ s'(t) = -0.1(2)t + 0.4(1)
∴ s'(t) = -0.2t + 0.4
- s'(t) is the function of velocity after time t seconds
∵ s'(t) = v(t)
∴ v(t) = -0.2t + 0.4
We need to find the instantaneous velocity of the ball after 4 seconds
Substitute t by 4
∴ v(4) = -0.2(4) + 0.4
∴ v(4) = -0.8 + 0.4
∴ v(4) = -0.4
∴ The v is -0.4 m/s ⇒ -ve means the velocity is downward
The instantaneous velocity of the ball after 4 seconds is -0.4 m/s
Find the coordinates of the point on a circle with radius 4 at an angle of 2pi/3
{Please help!!}
Answers
Answer:
The coordinates of the point on a circle with radius 4 at an angle of \(\frac{2\pi}{3}\) radians are x = -2 and y = 3.464.
Step-by-step explanation:
This problem ask us to determine the rectangular coordinates from polar coordinates. The polar coordinates of the point in rectangular form is expressed by the following expression:
\((x,y) = (r\cdot \cos \theta, r\cdot \sin \theta)\)
Where \(r\) and \(\theta\) are the radius of the circle and the angle of inclination of the point with respect to horizontal, measured in radians. If \(r = 4\) and \(\theta = \frac{2\pi}{3}\,rad\), the coordinates of the point are:
\((x,y) = \left(4\cdot \cos \frac{2\pi}{3},4\cdot \sin \frac{2\pi}{3} \right)\)
\((x,y) = (-2, 3.464)\)
The coordinates of the point on a circle with radius 4 at an angle of \(\frac{2\pi}{3}\) radians are x = -2 and y = 3.464.
4:5=___:35 fill in the missing value
Answers
Answer: 28:35
Step-by-step explanation: The missing number is 28, here's how to solve it:
So, if we know both components of the first ratio AND the second component of the 2nd ratio, we need to divide the 2nd components from each ratio. So, 35 / 5 = 7. Now, we need to multiply 7 by 4. We get 28. So, the 2nd ratio is 28:35. I hope this helps!
(Look at the attachment for a better idea)

Ms. Rios wants to store her son’s old clothes in a box. She believes the clothes will fit in a box that has a volume of 12 cubic feet³. What could be the dimensions of Ms. Rios' box?
Is it
6 ft x 4 ft x 2 ft
4 ft x 4 ft x 4 ft
4 ft x 2 ft x 2 ft
3 ft x 2 ft x 2 ft
Please Help
Answers
Answer:
the fourth one
Step-by-step explanation:
3x2x2 = 12
Answer:Last one,
Step-by-step explanation:
it equals 12, making it the only one that works.
help me please
Indicate the method you would use to prove the two triangles. If no method applies, enter "none".
AAS
SSS
NONE
SAS
ASA

Answers
Based on the information given, we know that the two triangles have two pairs of congruent angles. This is sufficient to prove that the two triangles are congruent using the AAS (Angle-Angle-Side) postulate. Therefore, the method I would use to prove the two triangles congruent is **AAS**.
2
1
-1
-2
Determine the period.
2 4
6 8 10 12 14
Acellus

Answers
According to the information we can infer that the period of the graph is 8.
How to determine the period of the graph?To determine the period of the graph we have to consider that the period of a grah is the distance between rigdes. So, in this case we have to count what is the difference between each rigde.
In this case, the distance between rigdes is 8 units because the first is located in the line 1 an the second is located in the line 9. So we can conclude that the period of the graph is 8.
Learn more about period in: https://brainly.com/question/23532583
#SPJ1
Simone wrote that 2+5.8=6.
Use the drop-down menus to explain why 6 is incorrect.
2+5.8 cannot be 6 because 2+5.8 is
Six is a correct sum to the expression +5.8, not 2+5.8.
Answers
0.2+5.8=6
Answer: The correct equation should be 0.2+5.8=6
Step-by-step explanation: First of all let's look at the equation without the decimal ".8". So, it would be 2+5 which equals 7. Since the sum is greater, adding a decimal will make the sum larger than 6. 2+5.8=7.8, therefore the correct equation being 0.2+5.8=6. Hopes this makes sense and helps!
what is the distance formula between (7,11) and (-1,5)
Answers
Let's begin by listing out the information given to us:
\(\begin{gathered} (x_{1,}y_1)=(7,11) \\ (x_{2,}y_2)=(-1,5) \end{gathered}\)The distance formula is given by the Pythagoras theorem shown below:
\(\begin{gathered} d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ d=\sqrt[]{(-1-7)^2+(5-11)^2}=\sqrt[]{(-6)^2+(-6)^2} \\ d=\sqrt[]{36+36}=\sqrt[]{72} \\ d=6\sqrt[]{2} \end{gathered}\)OR
The distance formula is the difference between two points on the same coordinate, all squared
d
You will create an estimate in order to assist your boss in gaining the job!
The equation that is given by the customer for the pool is (units are in feet): x2 + y2 = 81
- Your performance needs to include the following calculations:
- Compute the radius and diameter of the pool.
- Compute the volume of water in the pool using the above calculations and a height of 4 ft.
- Assuming that water costs $0.22 per gallon and that pools cost $185 per foot of radius calculate the total cost of materials for the pool. Keep in mind that there are 7.48 gallons of water per square foot.
- Assuming that a pool will take 2.5 hrs. per foot of radius, compute the total labor hours for the pool.
- If you are to pay someone $19/hr, compute the total cost of labor.
- Find a total cost for the project.
- All of the above is done showing all work.
- A final paragraph that describes your steps and findings.
Answers
The solution has been explained below.
We will use the following equation, x² + y² = 81, where x and y are the pool's dimensions in feet, to determine the necessary estimations for the pool project.
1. Calculate the pool's diameter and radius:
We can infer that the radius of the pool is equal to the square root of 81 because the equation depicts a circle. As a result, r = 81 = 9 ft.
The pool's diameter is simply equal to twice its radius, or d = 2r = 18 feet.
2. Calculate the amount of water in the pool:
Since the pool is 4 feet high, we can determine its volume by multiplying the area of its circle-shaped base by the height.
The formula A = πr² can be used to calculate the area of the pool's base,
Therefore, V = Ah = πr²h = 1017.87 cubic feet represents the volume of water in the pool.
3. Determine the pool's total material cost:
There are 7.48 gallons in a cubic foot and a gallon of water costs $0.22.
As a result, Cw = V × 7.48 × 0.22 = 1017.87 × 7.48 × 0.22 = $1,619.72 is the entire cost of water for the pool.
If the radius of the pool is $185 per foot, then the cost of the materials is Cm = r × 185 = 9 × 185 = $1,665.
4. Calculate the pool's total labour hours:
A pool's construction is estimated to take 2.5 hours for every foot of its radius.
So, L = r × 2.5 = 9 × 2.5 = 22.5 hours of labour would be needed to complete this pool.
5. Calculate the total cost of labour for the project:
If you pay someone $19 per hour, Cl = L × 19 = 22.5 × 19 = $427.5.
6. Find the project's overall cost:
The project's overall cost is the product of its labour and material costs:
Cost as a whole equals Cm + Cw + Cl, which is 1,665 + 1,619.72 + 427.5, or $3,712.22.
In conclusion, the pool has an 18-foot diameter and a 9-foot radius according to the supplied equation. There are roughly 1017.87 cubic feet of water in the pool. Water and building supplies are included in the pool's projected material cost of $3,284.72. 22.5 hours of labour in total are needed, with a labour cost estimate of $427.5. Consequently, $3,712.22 is the expected final cost of the pool renovation.
Learn more about functions click;
https://brainly.com/question/31062578
#SPJ1
5) Which choice shows the numbers correctly ordered? A) VA * 4.15 < V21 B) 1

Answers
Answer:
I believe the correct answer is C
Step-by-step explanation:
if you convert 1/5 into a decimal it would be 0.2
if you convert 1/21 to a decimal it would be 0.4 then you make it a square root it would be 0.6
4.15 is already a decimal so you don't have to do anything to it.
if you turn 21 into a square root the would be 4.5
so if you line all the numbers up from smalllest to lagerst it would look like this
1/5,1/21,21,4.15
so c is the corrcet answer
HELP ME PLS (Solve 6!)

Answers
Answer:
720
Step-by-step explanation:
This means 6x5x4x3x2x1
Determine the equation of the circle with radius 9 and center ( − 1 , − 8 ).
Answers
The equation of the circle with radius 9 and center ( -1,-8 ) is( x + 1 )² + ( y + 8 )² = 81.
What is the equation of the circle?The standard form equation of a circle with center (h, k) and radius r is:
( x - h )² + ( y - k )² = r²
Given that the circle has a center of (-1, -8) and the radius is 9.
Hence; from the standard form of the equation of the circle:
Center ( h , k ) = ( -1, -8 )
h = -1
k = -8
And radius r = 9
Plug these values into the above formula and simplify.
( x - h )² + ( y - k )² = r²
( x - ( -1 ) )² + ( y - ( -8 ) )² = 9²
Simplify
( x + 1 )² + ( y + 8 )² = 81
Therefore, the equation of the circle is ( x + 1 )² + ( y + 8 )² = 81.
Learn more about equation of circle here: brainly.com/question/29288238
#SPJ1
the temperatures at midday on march 1st in five cities are shown in the bar chart below. What is the difference in temperature between rome and munich?
Answers
The difference in temperature between Rome and Munich is given as follows:
6ºC.
How to obtain the difference in temperatures?The difference in temperature between Rome and Munich is given by the subtraction of Rome's temperature by Munich's temperature.
The bar graph in the context of this problem gives the temperature for each town.
From the bar graph given by the image presented at the end of the answer, the temperatures for Rome and Munich are given as follows:
Rome: 11 ºC.Munich: 5 ºC.Hence the difference in temperature between Rome and Munich is given as follows:
11 - 5 = 6ºC.
Missing InformationThe graph is given by the image presented at the end of the answer.
More can be learned about difference of two amounts at https://brainly.com/question/17301989
#SPJ1

Prove that
(secx+tanx)² =CSCx+1/CSC x-1

Answers
To prove that (secx+tanx)² = (cscx+1)/(cscx-1), we will start with the left-hand side (LHS) of the equation and simplify it step by step until it matches the right-hand side (RHS) of the equation.
LHS: (secx+tanx)²
Using the trigonometric identities secx = 1/cosx and tanx = sinx/cosx, we can rewrite the LHS as:
LHS: (1/cosx + sinx/cosx)²
Now, let's find a common denominator and simplify:
LHS: [(1+sinx)/cosx]²
Expanding the squared term, we get:
LHS: (1+sinx)² / cos²x
Next, we will simplify the denominator:
LHS: (1+sinx)² / (1 - sin²x)
Using the Pythagorean identity sin²x + cos²x = 1, we can replace 1 - sin²x with cos²x:
LHS: (1+sinx)² / cos²x
Now, let's simplify the numerator by expanding it:
LHS: (1+2sinx+sin²x) / cos²x
Next, we will simplify the denominator by using the reciprocal identity cos²x = 1/sin²x:
LHS: (1+2sinx+sin²x) / (1/sin²x)
Now, let's simplify further by multiplying the numerator and denominator by sin²x:
LHS: sin²x(1+2sinx+sin²x) / 1
Expanding the numerator, we get:
LHS: (sin²x + 2sin³x + sin⁴x) / 1
Now, let's simplify the numerator by factoring out sin²x:
LHS: sin²x(1 + 2sinx + sin²x) / 1
Using the fact that sin²x = 1 - cos²x, we can rewrite the numerator:
LHS: sin²x(1 + 2sinx + (1-cos²x)) / 1
Simplifying further, we get:
LHS: sin²x(2sinx + 2 - cos²x) / 1
Using the fact that cos²x = 1 - sin²x, we can rewrite the numerator again:
LHS: sin²x(2sinx + 2 - (1-sin²x)) / 1
Simplifying the numerator, we have:
LHS: sin²x(2sinx + 1 + sin²x) / 1
Now, let's simplify the numerator by expanding it:
LHS: (2sin³x + sin²x + sin²x) / 1
LHS: 2sin³x + 2sin²x / 1
Finally, combining like terms, we get:
LHS: 2sin²x(sin x + 1) / 1
Now, let's simplify the RHS of the equation and see if it matches the LHS:
RHS: (cscx+1) / (cscx-1)
Using the reciprocal identity cscx = 1/sinx, we can rewrite the RHS:
RHS: (1/sinx + 1) / (1/sinx - 1)
Multiplying the numerator and denominator by sinx to simplify, we get:
RHS: (1 + sinx) / (1 - sinx)
Now, we can see that the LHS and RHS are equal:
LHS: 2sin²x(sin x + 1) / 1
RHS: (1 + sinx) / (1 - sinx)
Therefore, we have proven that (secx+tanx)² = (cscx+1)/(cscx-1).
For more such questions on prove, click on:
https://brainly.com/question/30831725
#SPJ8