Let g(x)=-1/2x+ 6 and h (x) =g(x)-3 which statement descibes the graph of h(x)?
Answers
The statement describing the graph of h(x) is "The graph of h(x) is a vertical translation of the graph of g(x) down 3 units".
In the question, are given two graphs g(x) = - (1/2)x + 6, and h(x) = g(x) - 3.
We are asked for the statement that best describes h(x).
Now, h(x) = g(x) - 3, can be seen as the translation of g(x).
For any graph m(x), n(x) is its translation if n(x) = m(x + p) + q.
The translation is vertical is q ≠ 0, and horizontal when p ≠ 0. Also,
if p > 0, then the horizontal translation is p units right,if p < 0, then the horizonal translation is |p| units left,if q > 0, then the vertical translation is q units up, andif q < 0, then the vertical translation is |q| units down.For, h(x) = g(x) - 3, when we compare it with n(x) = m(x + p) + q, we have p = 0, and q = -3.
Since, q ≠ 0, we have vertical translation, and since q < 0, the translation is |q| units down.
Thus, The statement describing the graph of h(x) is "The graph of h(x) is a vertical translation of the graph of g(x) down 3 units".
Learn more about translations at
https://brainly.com/question/11821088
#SPJ9
The provided question is incomplete. The complete question is:
"Let g(x) = - (1/2)x + 6 and h(x) = g(x) - 3. Which statement describes the graph of h (x)?
The graph of h(x) is a vertical translation of the graph of g(x) up 3 units.The graph of h(x) is a horizontal translation of the graph of g(x) right 3 units.The graph of h(x) is a horizontal translation of the graph of g(x) left 3 units.The graph of h(x) is a vertical translation of the graph of g(x) down 3 units."Related Questions
The mean number of words per minute (WPM) read by sixth graders is 8989 with a standard deviation of 1616 WPM. If 6666 sixth graders are randomly selected, what is the probability that the sample mean would be greater than 92.2592.25 WPM
Answers
The probability of obtaining a sample mean greater than 92.25 WPM is extremely small, approaching zero.
What is the probability of obtaining a sample mean greater than 92.25 WPM, given a population mean of 8989 WPM and a standard deviation of 1616 WPM?We can use the Central Limit Theorem to solve this problem, since we have a large sample size (n=6666) and a known population mean (μ=8989) and standard deviation (σ=1616).
The Central Limit Theorem states that the distribution of the sample means will be approximately normal with a mean of μ and a standard deviation of σ/√n.
So, for this problem:
The sample size is n=6666The population mean is μ=8989The population standard deviation is σ=1616We want to find the probability that the sample mean would be greater than 92.25 WPM, which we can convert to a z-score using the formula z = (x - μ) / (σ / √n), where x is the sample mean.
z = (92.25 - 8989) / (1616 / √6666) = -116.45
Now, we can use a standard normal distribution table or a calculator to find the probability that a standard normal random variable is greater than -116.45. Since this probability is extremely small (essentially zero), we can conclude that the probability of getting a sample mean greater than 92.25 WPM is also essentially zero.
Therefore, the answer to the problem is that the probability of getting a sample mean greater than 92.25 WPM is essentially zero, or very close to 0.
Learn more about Central Limit Theorem
brainly.com/question/18403552
#SPJ11
describe the graph of the solution

Answers
First, we want to note two things:
We have a solid circle at -10, so -10 IS part of the solution.We have shading to the right of -10, meaning we also need to include numbers to the right of -10, or numbers greater than -10.
We can describe this with an inequality: x ≥ -10
Be sure you use ≥ and not >, since -10 is included.
We can describe this with interval notation: [ -10, infty )
Be sure you use [ and not ( on -10, since -10 is included.
You can also use set-builder notation: { x | x ≥ -10 }
Find the area of a triangle with a =174, b =138, and c =188. round your answer to the nearest tenth, if necessary.
Answers
Answer:11486.34
Step-by-step explanation:
p is semiperimeter
p=(a+b+c)/2
p=(174+138+188)/2
For Heron equation,
S=\(\sqrt{p*(p-a)*(p-b)*p-c}\)
so, it is 11487.34
Answer:
11,486.3 square units
Step-by-step explanation:
You want the area of the triangle with side lengths a=174, b=138, c=188.
Area
The area of a triangle can be found from the lengths of the three sides using Heron's formula:
A = √(s(s -a)(s -b)(s -c)) . . . . . . . where s = (a+b+c)/2, the "semiperimeter"
CalculationThe calculation is shown in the second attachment.
s = (174 +138 +188)/2 = 500/2 = 250
A = √(250(250 -174)(250 -138)(250 -188)) = √(250(76)(112)(62))
A = √131936000 ≈ 11486.3
The area of the triangle is about 11486.3 square units.
__
Additional comment
An effectively equivalent way to find the area is to use the Law of Cosines to find an angle, then use the trig formula for the area.
C = arccos((a²+b²-c²)/(2ab)) = arccos(13976/48024) ≈ 73.080899°
Area = 1/2(ab·sin(C)) = 1/2(24012·0.9567166) ≈ 11486.3
We say this is "effectively equivalent" not only because it gives the same area, but because using the relation between cos(C) and sin(C), you can demonstrate that this formula gives Heron's formula for the area.


say i bought 600 stickers, stickers no one can get, and the price of the grand total of these stickers was 26.97$, and every 10 stickers i sold for 2$ would i make profit back or even more??
Answers
Given the price the stickers can be sold for, and the cost in total of the stickers, you can make profit.
How to find the profit ?The total revenue that can be made on the sale of the 600 stickers that you got, would be:
= ( Number of stickers in total / Number of stockers per pack ) x Price per sticker pack
= 600 / 10 x 2
= 60 x 2
= $ 120
Your profit would be:
= Total revenue from stickers - total cost
= 120 - 26. 97
= $ 93. 03
Find out more on profit at https://brainly.com/question/25112972
#SPJ1
Need help ASAP please
Part 1:
You started saving for a car when you were in middle school. You have done some research and discovered that it will cost you about $9,500 for a used car that would fit your needs. You were encouraged to invest some of your savings to help you get to your financial goal faster. So far you have saved $7,500. If you invest this money in a savings account with a 3.3% interest rate compounded annually, how long will it take to earn enough money to go purchase the car? Use the compound interest formula A = P (1 + i)n, where A is the accumulated amount, P is the principal, i is the interest rate per year, and n is the number of years. Round your final answer to the nearest tenth.
Answers
Answer:
7.3 years.
Step-by-step explanation:
A = P(1 + i)^n
9500 = 7500(1 + 0.033)^n where n = number of years.
1.033^n = 9500/7500
n log 1.033 = log(9500/7500)
n = log(9500/7500)/log 1.033
n = 7.28 = 7.3.
Find the lowest common denominator (multiple). Type the equivalent fractions. Then, add or subtract. Simplify your answer. 1
2
1
3
Answers
Answer:what
Step-by-step explanation:what does this mean
Question 4 Which one is correct about omitted variable bias? Check all that apply. (Two correct answer.) If the omitted variable and included variable are correlated, there is a bias. If the omitted variable is relevant, there is a bias. Random assignment of included variables cuts the relationship between the omitted variable and included variable and bring the bias to zero. If the omitted variable and included variable are correlated AND the omitted variable is relevant, there is a bias.
Answers
The correct statements about omitted variable bias are:
1. If the omitted variable and included variable are correlated, there is a bias.
2. If the omitted variable is relevant, there is a bias.
Omitted variable bias refers to the bias introduced in an econometric model when a relevant variable is left out of the analysis. The bias occurs when the omitted variable is correlated with both the dependent variable and the included variables in the model.
If the omitted variable and included variable are correlated, there is a bias because the included variable may capture some of the effects of the omitted variable. In this case, the estimated coefficient of the included variable will be biased, as it will include the influence of the omitted variable.
Similarly, if the omitted variable is relevant, there is a bias because it has a direct impact on the dependent variable. By excluding the relevant variable, the model fails to account for its influence, leading to biased estimates of the coefficients of the included variables.
Random assignment of included variables does not eliminate omitted variable bias. While random assignment may help control for confounding factors and reduce bias in certain experimental designs, it does not address the issue of omitting a relevant variable from the analysis. Omitted variable bias can still exist even with random assignment of included variables.
Learn more about variable here:
https://brainly.com/question/29583350
#SPJ11
help..................

Answers
Answer:
Line A
Step-by-step explanation:
Suppose two cards are drawn at random without replacement from a deck of 4 cards numbered 1, 2, 3, 4. Let X be the number on the first card and Y be the number of the second card. 1. Find the conditional probability of X given Y = 2. 2. Use this to compute P(X ≤ 2|Y = 2).
Answers
1. The conditional probability of X being 1 given that Y = 2 is 1/2. 2. The probability of X being less than or equal to 2 given that Y = 2 is 1/2.
To find the conditional probability of X given Y = 2, we need to consider the possible outcomes when Y = 2 and determine the probability of X being a specific value.
Let's calculate the conditional probability:
1. Find the conditional probability of X given Y = 2:
When Y = 2, we have two possible outcomes: (1, 2) and (3, 2). Out of these two outcomes, only one has X ≤ 2, which is (1, 2).
Therefore, the conditional probability of X being less than or equal to 2 given that Y = 2 is:
P(X ≤ 2 | Y = 2) = P(X = 1 | Y = 2) = 1/2
2. Use this to compute P(X ≤ 2 | Y = 2):
We have already determined that P(X ≤ 2 | Y = 2) = P(X = 1 | Y = 2) = 1/2.
For more about probability:
https://brainly.com/question/31828911
#SPJ4
8^5 = 2^2m+3
Solve m
Answers
Answer:
\(m=6\)
Step-by-step explanation:
Exponent properties:
We can use exponent property \(a^{b^c}=a^{(b\cdot c)}\) to solve this problem.
Rewrite \(8\) as \(2^3\), then apply exponent property \(a^{b^c}=a^{(b\cdot c)}\) to simplify:
\(2^{3^5}=2^{2m+3},\\2^{15}=2^{2m+3}\)
If \(a^b=a^c\), then \(b=c\), because of log property \(\log a^b=b\log a\). Using this log property, you can take the log of both sides and divide by \(\log a\) to get \(b=c\)
Therefore, we have:
\(15=2m+3\)
Subtract 3 from both sides:
\(12=2m\)
Divide both sides by 6:
\(m=\frac{12}{2}=\boxed{6}\)
Alternative:
Given \(8^5=2^{2m+3}\), to move the exponent down, we'll use log properties.
Start by simplifying:
\(\log 32,768=2^{2m+3}\)
Take the log of both sides, then use log property \(\log a^b=b\log a\) to move the exponent down:
\(\log(32,768)=\log 2^{2m+3},\\\log (32,768)=(2m+3)\log 2\)
Divide both sides by \(\log2\):
\(2m+3=\frac{\log (32,768)}{\log(2)}\)
Subtract 3 from both sides:
\(2m=\frac{\log (32,768)}{\log(2)}-3\)
Divide both sides by 2:
\(m=\frac{\log (32,768)}{2\log(2)}-\frac{3}{2}=\boxed{6}\)
Answer the question to get points

Answers
Answer:
4. E=4x-15(vertically opposite)
F=E(corresponding angles)
E=4x-15
5.3x+6x+O=180(angles on a straight line)
9x+0=180
9x=180-0
9x/9=180/9
x=20
MN¯has an endpoint at M(–2, –2) and an endpoint at N(7, –5).
What is the length of MN to the nearest tenth?
Answers
The length of the segment MN is 9.5 units
How to determine the length of the segment MN?The endpoints of the line segment MN are given as
M = (-2, -2)
N = (7, -5)
The length of the segment MN is calculated as:
MN = √(x2 - x1)^2 + (y2 - y1)^2
Substitute the known values in the above equation
So, we have
MN = √(-2 - 7)^2 + (-2 + 5)^2
Evaluate the sum and the difference
So, we have
MN = √-9^2 + 3^2
Evaluate the exponents
So, we have
MN = √81 + 9
Evaluate the sum
So, we have
MN = √90
Evaluate the exponents
So, we have
MN = 9.5
Hence, the length of the segment MN is 9.5 units
Read more about length at
https://brainly.com/question/7243416
#SPJ1
Write the hypothesis and the conclusion of each conditional.
1. If 3x-7 = 32, then x = 13.
Answers
Answer:
XDdddDddDDDDDDDDDDDDDDDDDDD
Deborah had 3 1/4 yards of ribbon she used 3/8 of a yard for a project how much ribbon does she have left
Answers
PLEASE ITS URGENT!!!
find the constant of proportionality for the table of values
X.
2
3
4
Y.
5
7.5
10
K=
Answers
2 x 2.5 = 5
3 x 2.5 = 7.5
4 x 2.5 = 10
What is the area of this figure? Enter your answer in the box. units2

Answers
Answer:
42 un^2
Step-by-step explanation:
You can split the hexagon into 2 triangles and a rectangle. For this, I will use the triangle that goes from (-5, 2) to (2, 2) to (-1, 4), the triangle that goes from (-5, -2) to (2, -2) to (-2, -4), and the rectangle that goes from (-5, 2) to (-5, -2) to (2, -2) to (2, 2). The top triangle is 7 units by 2 units, so using triangle formula, you get 7*2/2 = 7. The rectangle is 4 by 7, so you get 4*7=28, and the bottom triangle is 7 units by 2 units (identical to top triangle) so you get 7. 28+7+7=42 units^2
How to get 5% of any number
Answers
______________________________________________________
Five percent is considered a half of 10 percent. So, to find 5% of a number, all you need to do is divide 10 percent of the number by 2.
Example: Let's find 5 percent of 230. You need to take 23 and divide it by 2, and you get 11.5.________________________________________________________
Hope this helps! If so, lmk! Thanks and good luck!
John is saving to buy a new car that will cost him $24,000. John started his savings at the beginning of the school year and has been able to accumulate $1000 after the first month. John plans to continue his savings at a rate proportional to the amount he still needs to save. Determine John's savings amount as function of time Hint: A variable y is said to be proportional to a variable x if y=cx for some constant c.
Answers
John's savings amount as a function of time is S(t) = $24,000 / 25. Initially, he needs to save $24,000 for a new car. After the first month, he has saved $1,000. The savings amount is directly proportional to the time elapsed. The constant of proportionality is 1/24. Thus, John's savings amount can be determined based on the remaining amount he needs to save.
John's savings amount can be represented as a function of time and is proportional to the amount he still needs to save. Let's denote the amount John needs to save as N(t) at time t, and his savings amount as S(t) at time t. Initially, John needs to save $24,000, so we have N(0) = $24,000.
We know that John has saved $1,000 after the first month, which means S(1) = $1,000. Since his savings amount is proportional to the amount he still needs to save, we can write the proportionality as:
S(t) = k * N(t)
where k is a constant of proportionality.
We need to find the value of k to determine John's savings amount at any given time.
Using the initial values, we can substitute t = 0 and t = 1 into the equation above:
S(0) = k * N(0) => $1,000 = k * $24,000 => k = 1/24
Now we have the value of k, and we can write John's savings amount as a function of time:
S(t) = (1/24) * N(t)
Since John's savings amount is proportional to the amount he still needs to save, we can express the amount he still needs to save at time t as:
N(t) = $24,000 - S(t)
Substituting the expression for N(t) into the equation for S(t), we get:
S(t) = (1/24) * ($24,000 - S(t))
Simplifying the equation, we have:
24S(t) = $24,000 - S(t)
25S(t) = $24,000
S(t) = $24,000 / 25
Therefore, John's savings amount at any given time t is S(t) = $24,000 / 25.
To know more about proportional savings, refer here:
https://brainly.com/question/29251832#
#SPJ11
What is the base in the expression 4⁵?
A. 4
B. 5
C. 9
D. 20

Answers
Answer:
4
Step-by-Step Explantion:
Base it always the big number not the little one on the top.
Is the average of a sum the sum of the averages?.
Answers
The average of a sum and the sum of the averages are not same.
The average of a sum is mean of sum of data. Total sum divided by total number of sum.
Whereas , The sum of the average is sum of mean of data. Total sum of averages of data.
These two terms are not same.
For example : Assume x₁ , x₂ , x₃ , . . . , xₙ be a data
S₁ , S₂ , . . . , Sₙ be sum of data
A₁ , A₂ , . . . , Aₙ be average of data
where n is total number of sum of data
The Average of sum will be = S₁ + S₂ + . . . + Sₙ / n
The sum of average will be = A₁ + A₂ + . . . + Aₙ
Hence, The average of sum and Sum of average are different.
To know more about Average here
https://brainly.com/question/16612044
#SPJ4
HELP ME HELP HELPPPPPPPP

Answers
Answer:
1/2
Step-by-step explanation:
all of the other fraction simplify to 3/4, 1/2 does not
If you do 12/16 and divide it by 2 you get 6/8 if you try to break it down anymore you get 3/4 and that’s the lowest you can go. Therefore, it’s 1/2.
When a mantis shrimp strikes, its arm-club gets up to a velocity of 23 m/s moving away from its body. Scientists calculate the acceleration at 100,000 m/s/s.
How long does it take the arm-club to get to 23 m/s?
Answers
Answer:
\(t=4.6\times 10^{-4}\ s\)
Step-by-step explanation:
We have,
Initial velocity of arm club is 23 m/s
Acceleration is 100000 m/s²
It is required to find the time taken by it to take the arm-club to get to 23 m/s. It means v = -23 m/s. It is based on the equation of kinematics such that :
\(v=u+at\\\\t=\dfrac{v-u}{a}\\\\t=\dfrac{-23-23}{100000}\\\\t=4.6\times 10^{-4}\ s\)
So, the time taken by it is \(4.6\times 10^{-4}\ s\).
find x, please show the work

Answers
You find this answer by solving the equation
8x-1+27=90 combine similar numbers
8x+26=90 Subtract 26 from both sides
8x=64 Divide both sides by 8
X=8
Substitute variable x for 8 and the angle you’re left with is 63 degrees
Solve the equation for x. √x-6+3=10

Answers
Given circle with diameter AB. Find the angle of C
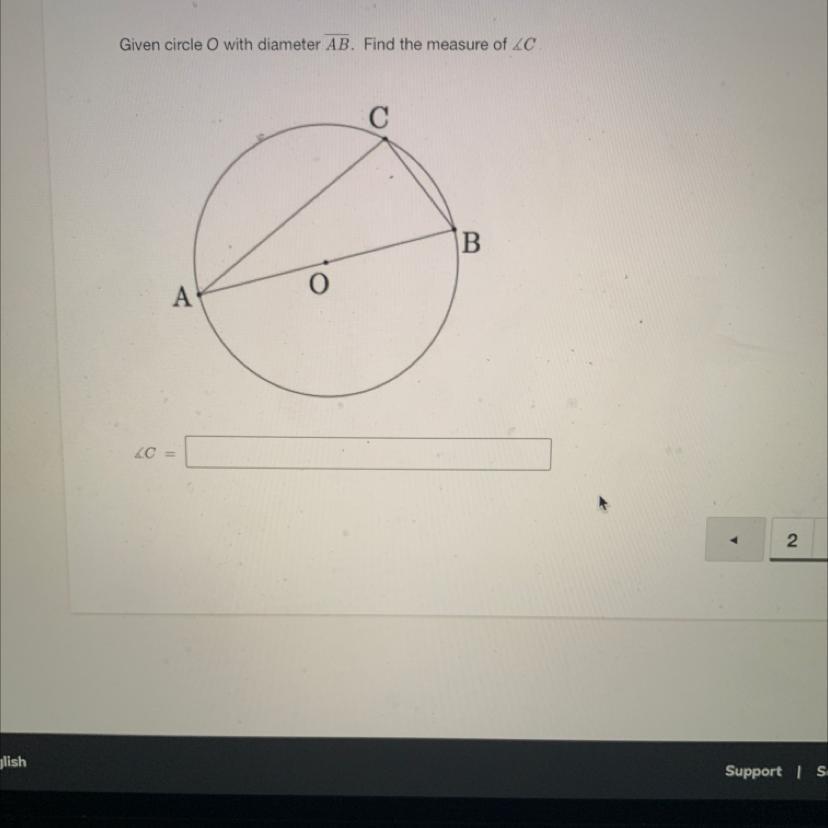
Answers
Answer:
90°
Step-by-step explanation:
Since AB is the diameter of the circle with center O and C is any point on the circle.
So, ACB is a semicircular arc.
Angle inscribed in a semicircle is right angle.
\(\therefore \measuredangle C =90\degree \)
Which of the following allows for someone to draw conclusions about a population from the information collected in a population sample? a. magnitude statistics b. central tendency c. inferential statistics d. effect size
Answers
The correct answer is c. Inferential Statistics.
Inferential statistics is the branch of statistics that allows for drawing conclusions about a population based on the information collected from a sample. When conducting research or surveys, it is often impractical or impossible to collect data from an entire population.
Instead, a representative sample is chosen, and inferential statistics are used to make inferences or predictions about the larger population. By analyzing the characteristics and patterns observed within the sample, inferential statistics enable researchers to make generalizations or draw conclusions about the population as a whole.
This process involves applying various statistical techniques, such as hypothesis testing and confidence intervals, to estimate population parameters and assess the reliability of the conclusions. Magnitude statistics, central tendency, and effect size are important concepts in statistics, but they do not specifically address the ability to draw population-level conclusions from sample data.
Magnitude statistics focuses on the size of the effect or relationship between variables, central tendency measures summarize the central values of a dataset, and effect size quantifies the strength of an effect or relationship.
Learn more about Statistics
brainly.com/question/29093686
#SPJ11
find x for − 1/5(x − 4) = −2 i need help asap please help
Answers
-1/5x= -3
x= 15
Mark Brainly pls
Find the zeros of the function.
Enter the solutions from least to greatest.
h(2) = -5x2 + 180
lesser x=
greater x =

Answers
Answer:
lesser x = -6
greater x = 6
Step-by-step explanation:
-5x² + 180 = 0
5x² = 180
x² = 36
x = ±6
Answer:
x = - 6, x = 6
Step-by-step explanation:
Given
h(x) = - 5x² + 180
To find the zeros let h(x) = 0 , that is
- 5x² + 180 = 0 ( subtract 180 from both sides )
- 5x² = - 180 ( divide both sides by - 5 )
x² = 36 ( take the square root of both sides )
x = ± \(\sqrt{36}\) = ± 6 , then
lesser x = - 6
greater x = + 6
Explain how the uncertainty of a measurement relates to the accuracy and precision of the measuring device. Include the definitions of accuracy and precision in your answer.
Answers
In the context of measurement, accuracy and precision refer to two related but distinct concepts. Accuracy is the degree to which a measurement is close to the true value of what is being measured, while precision is the degree to which repeated measurements of the same quantity are close to each other.
The uncertainty of a measurement refers to the degree of doubt or lack of confidence in the result obtained from a measuring instrument. It is typically represented by an interval around the measured value that indicates the range within which the true value is likely to lie.
The accuracy of a measuring device is related to its ability to provide measurements that are close to the true value. If a measuring device is highly accurate, then its measurements will be close to the true value, and the uncertainty associated with those measurements will be relatively small. On the other hand, if a measuring device is not very accurate, then its measurements may be far from the true value, and the uncertainty associated with those measurements will be relatively large.
The precision of a measuring device is related to its ability to provide measurements that are close to each other when measuring the same quantity repeatedly. A measuring device that is highly precise will give measurements that are very close to each other, and the uncertainty associated with those measurements will be relatively small. Conversely, a measuring device that is not very precise will give measurements that are far apart, and the uncertainty associated with those measurements will be relatively large.
for such more question on Accuracy
https://brainly.com/question/23748575
#SPJ11
The digit 8 in which number represents a value of 8 thousandths?
Choose 1 answer:
A) 4.618
B) 8,000.1
C) 7.822
Answers
Answer:
B) 8,000.18 is in thousands place.
The third digit to the right of decimal point is in the thousandths place. The fourth digit to the right of decimal point is in the ten thousandths place and so on.
Step-by-step explanation:
Hope it is helpful to....