In which of these situations do the quantities combine to make 0?
A. In the morning, the temperature rises 30 degrees. In the evening, it
falls by 30 degrees.
B. Kathy receives $20 as a gift. She gives half of her $20 to a friend
and keeps the rest for herself.
C. A player in a game earns 4 points for getting an answer right. She
then earns 4 points for making it around the board.
D. A hot air balloon rises 40 feet. It then rises another 40 feet.
Answers
Answer:
A
Step-by-step explanation:
A= 0
B= 10
C=8
D= 80
Which means the answer is A
Related Questions
Hey could you guys help me answer this and explain it to me? It’s math

Answers
Answer:
C. 8
Step-by-step explanation:
d = 8
4 + \(\frac{d}{2}\)
Substitute d for 8
4 + 8/2
Simplify
4+4
Simplify
8
Answer:
c = 8
Step-by-step explanation:
if d = 8, that would mean that
4 + 8/2 (because 8 has substituted for d)
then you do 8 ÷ 2 = 4
4 + 4 = 8
Write the first 4 terms of the sequence defined by the given rule. f(n)=n^3-1
Answers
the total surface area of a closed cylinder is 5000cm squared. find the dimensions of the cylinder that maximizes its volume
Answers
The dimensions of the cylinder that maximizes its volume are radius = 16.29 cm and height = 32.57 cm
Total surface area of a cylinderThe total surface area of a cylinder is given by A = 2πr² + 2πrh where
r = radius of cylinder and h = height of cylinder.Since the total surface area of the cylinder A = 5000 cm². So,
A = 2πr² + 2πrh
5000 = 2πr² + 2πrh
Making h subject of the formula, we have
h = 2500/πr - r
Volume of a cylinder
Since the volume of the cylinder V = πr²h where
r = radius of cylinder and h = height of cylinder.Substituting h into V, we have
V = πr²h
V = πr²(2500/πr - r)
V = 2500r - πr³
Maximizing the volume
Since we want to maximize V, we differentiate with respect to r and equate to zero.
So, dV/dr = d(2500r - πr³)/dr
dV/dr = 2500 - 3πr²
Equating dV/dr to zero, we have
dV/dr = 0
2500 - 3πr² = 0
2500 = 3πr²
Dividing both sides by 3π, we have
r² = 2500/3π
Findng the radius of the cylinderTaking square root of both sides, we have
r = √(2500/3π)
r = 50/√(3π) cm
r = 50/√(9.4247) cm
r = 50/3.07
r = 16.29 cm
Value of radius that gives maximum volume
We need to determine if this value of r gives a maximum for V. So, we differentiate dV/dr.
So, d(dV/dr)/dr = d²V/dr²
d²V/dr² = d(2500 - 3πr²)/dr
d²V/dr² = 0 - 6πr
d²V/dr² = - 6πr
Substituting the value of r into the equation, we have
d²V/dr² = - 6πr
d²V/dr² = - 6π(50/√(3π))
d²V/dr² = - 6π(50/√(3π))
Since d²V/dr² = - 6π(50/√(3π)) < 0. V is maximum at r = 50/√(3π) cm
Finding the height of the cylinderSubstituting the value of r into h, we have
h = 2500/πr - r
h = 2500/π(16.29) - 16.29
h = 2500/51.17 - 16.29
h = 48.86 - 16.29
h = 32.57 cm
So, the dimensions of the cylinder that maximizes its volume are radius = 16.29 cm and height = 32.57 cm
Learn more about dimensions of cylinder here:
https://brainly.com/question/14472257
Which one of the figures is the Pre-Image? Please help me no links!

Answers
Answer:
the one in black
Step-by-step explanation:
Find the equation of the line that passes through (-4, 2) and is perpendicular to the line that goes through (-4, 6) and (5, 2).
Answers
Answer:
9x -4y = -44
Step-by-step explanation:
You want the equation of the line through (-4, 2) and perpendicular to the line through (-4, 6) and (5, 2).
Equation of a LineThe equation of a line through (h, k) and perpendicular to the line through (x1, y1) and (x2, y2) can be written as ...
(x2 -x1)(x -h) +(y2 -y1)(y -k) = 0
Using the given points, this becomes ...
(5 -(-4))(x -(-4)) +(2 -6)(y -2) = 0
9(x +4) -4(y -2) = 0
Expanding this gives the general form equation:
9x -4y +44 = 0
Subtracting the constant gives the standard form equation:
9x -4y = -44

5+ (-11) – (-11).
Please help.

Answers
Answer:
5
Step-by-step explanation:
(-11)-(-11) would cancel out, so that leaves 5 as the answer :)
Answer: 126 i think cause 5+ (-11) – (-11) = 126 hope this helped have a nice day :)
Step-by-step explanation:
The points −−5, 11 and r, 9 lie on a line with slope 2. Find the missing coordinate r.

Answers
Solution
\(\begin{gathered} Let\text{ }(x_1,y_1),\text{ }(x_2,y_2) \\ Let\text{ }m=slope \\ m=\frac{y_2-y_1}{x_2-x_1} \end{gathered}\)\(\begin{gathered} If(-5,-11)=\text{ }(x_1,y_1),then\text{ }x_1=-5,\text{ }y_1=-11 \\ (r,9)=\text{ }(x_2,y_2),then\text{ }x_2=r,\text{ }y_1=9 \end{gathered}\)Using the Slope formula written above;
\(\begin{gathered} 2=\frac{9-(-11)}{r-(-5)} \\ 2=\frac{20}{r+5} \\ Cross\text{ }multiply \\ 2(r+5)=20 \\ Expansion\text{ }of\text{ }bracket \\ 2r+10=20 \\ 2r=20-10 \\ 2r=10 \\ Divide\text{ }both\text{ }sides\text{ }by\text{ }2 \\ \frac{2r}{2}=\frac{10}{5} \\ r=5 \end{gathered}\)Therefore, the missing co-ordinate r is 5.
Write the equation for the graph below:
See attached image.

Answers
The equation for the graph above is y = 5sin(2x/3).
What is a sine wave?In Mathematics and Geometry, a sine wave is sometimes referred to as a sinusoidal wave, or sinusoid and it can be defined as a fundamental waveform that is typically used for the representation of periodic oscillations, in which the amplitude of displacement at each interval is directly proportional to the sine of the displacement's phase angle.
Mathematically, a sine wave can be represented or modelled by this mathematical equation:
y = asin(bx)
Where:
a represents the amplitude of a sine wave. b represents the periodicity.Based on the graph of this sine wave, we have:
Amplitude, a = 5.
Periodicity, b = 2π/period = 2π/(3π) = 2/3
Therefore, the required sine wave function is given by;
y = asin(bx)
y = 5sin(2x/3)
Read more on sine function here: https://brainly.com/question/12150120
#SPJ1

Your sample is normally distributed with a mean age of 36. The standard deviation in this sample is 4 years. You would expect:
Answers
Kindly find complete question attached below
Answer:
Kindly check explanation
Step-by-step explanation:
Given a normal distribution with ;
Mean = 36
Standard deviation = 4
According to the empirical rule :
68% of the distribution is within 1 standard deviation of the mean ;
That is ; mean ± 1(standard deviation)
68% of subjects :
36 ± 1(4) :
36 - 4 or 36 + 4
Between 32 and 40
2.)
95% of the distribution is within 2 standard deviations of the mean ;
That is ; mean ± 2(standard deviation)
95% of subjects :
36 ± 2(4) :
36 - 8 or 36 + 8
Between 28 and 44
3.)
99% is about 3 standard deviations of the mean :
That is ; mean ± 3(standard deviation)
99% of subjects :
36 ± 3(4) :
36 - 12 or 36 + 12
Between 24 and 48

Translate into an expression:
two more than three times x
Answers
Answer:
3x + 2
Step-by-step explanation:
Answer:
2+3x
Step-by-step explanation:
What is the difference between the Commutative Property and Associative Property of addition?
Answers
Answer:
The commutative property of addition states that the order of the numbers being added does not affect the result of the addition. For example, "a + b = b + a" is the commutative property of addition.The associative property of addition states that when three or more numbers are being added, the grouping of the numbers being added does not affect the result. For example, "(a + b) + c = a + (b + c)" is the associative property of addition.In simple terms, the commutative property of addition allows for numbers to be added in any order and still get the same result, while the associative property of addition allows for numbers to be grouped in any way and still get the same result.Hope this helps! Enjoy Learning!:D
What is the independent
variable?
What is the dependent
variable?
Answers
Answer:
Independent variable is what you are testing in a experiment.
Dependent variable is what you're measuring in a experiment.
Step-by-step explanation:
An example would be testing how fast plants can grow after two weeks of giving each plant a different amount of water.
The independent variable would be the plants. You are testing to see how fast the plants can grow depending on the amount of water you give each plant in a matter of two weeks.
The dependent variable would be the amount of water you give each plant. You are giving each plant different amounts of water each day in a span of two weeks to see which one will grow faster.
Hope this helps.
how do u solve 4n-6=10n
Answers
Answer:
n = -1
Step-by-step explanation:
4n - 6 = 10n
Combine like terms: (you can see that both 4n and 10n both have a n. This means that they are like terms. You would just add/subtract them as usual)
4n - 10n = 6
-6n = 6
n = -1
Answer:
4n-4n-6=10n-4n
6/-6=6n/6
-1=n
Step-by-step explanation:
if f(x)=2x^2+3x-6 what is the value of f(2)
Answers
Answer:
8
Step-by-step explanation:
put in '2' where 'x' is
2 (2^2) + 3 ( 2) - 6 = 8
Has anyone done the inverse functions mastery text on edmentum it’s super hard stuck on this question and the pictures wont help
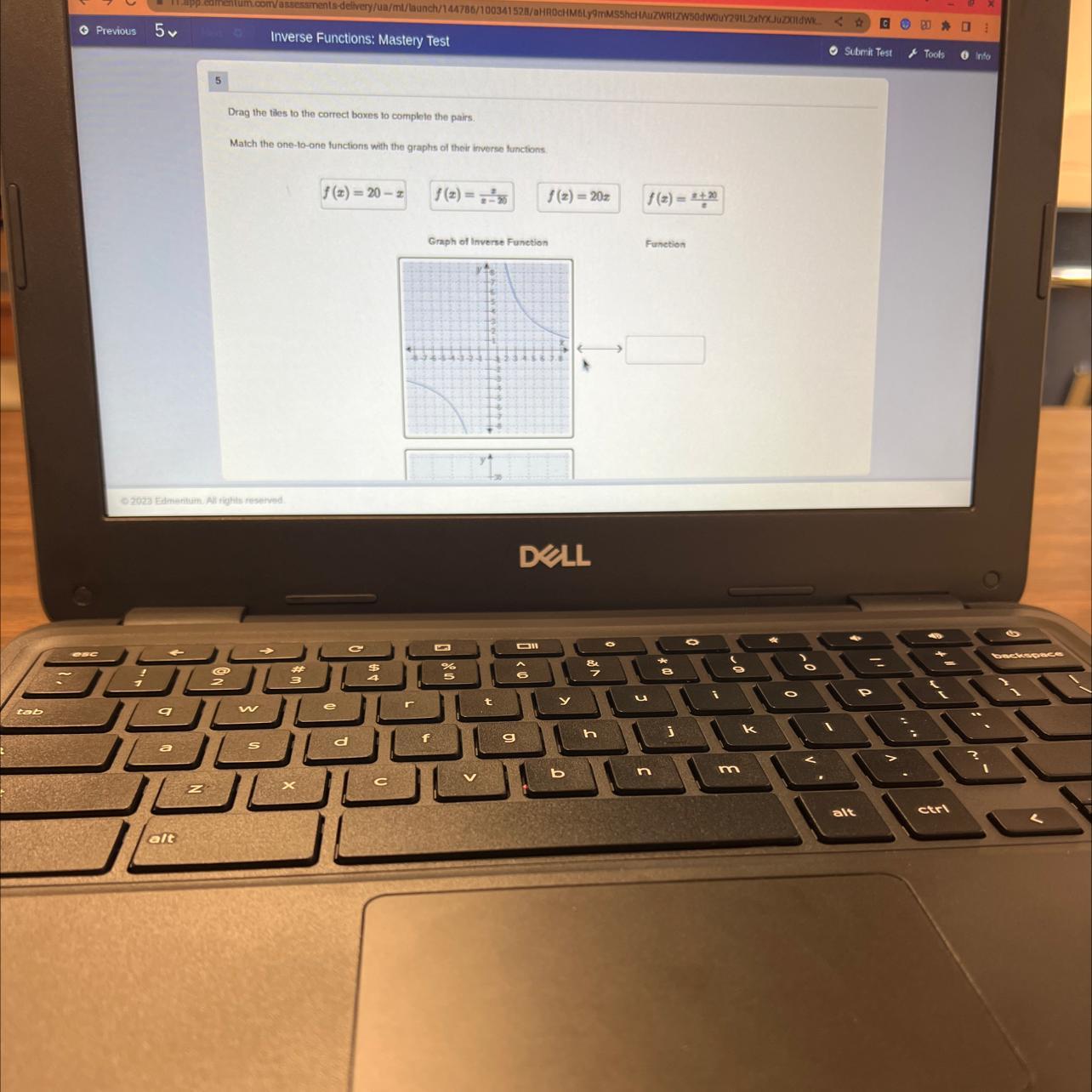
Answers
Type the correct answer in the box.
A railroad construction team wants to calculate an equation to ensure they set a second railroad track parallel to the first. The equation
that describes the first railroad track is y = 3z 9. The second railroad track goes through the point (2,9).
What is the equation, in slope-intercept form, of the second railroad track?
Answers
Then the linear equation for the second railroad track is:
y = 3*x + 3
What is the equation, in slope-intercept form, of the second railroad track?We know that the equation will be parallel to the line:
y = 3x + 9
Remember that two lines are parallel if the lines have the same slope, then the other linear equation is something like:
y = 3x + b
To find the value of b we use the fact that the line passes through (2, 9), this means that:
9 = 3*2 + b
9 - 6 = b
3 = b
Then the linear equation for the second railroad track is:
y = 3*x + 3
If you want to learn more about linear equations:
https://brainly.com/question/1884491
#SPJ1
Answer: Y+3x+3 your welcome
Step-by-step explanation:
dont worry bout allat
Tall hedges are shading a rose garden. At the time the gardener wants the garden to be in sunlight, a four- foot vertical pole casts a five foot shadow. Suppose that we want to cut the hedges back only to a height of 8 feet. How far must the edge of the garden be from the base of the hedge if we do not want any part of the rose garden to be in the shade?
Answers
Answer:
Step-by-step explanation:
A 4-ft pole casts a 5-ft shadow, so an 8-ft hedge casts a 10-ft shadow. Tge hedges must be 10 ft from the garden.
cho hàm số f(x) liên tục trên đoạn [a;b] và có nguyên hàm F(x) thỏa F(a)=10;F(b)=2022 Khi đó \int _a^b\: f(x) dx bằng
Answers
The result follows from the fundamental theorem of calculus.
\(\displaystyle \int_a^b f(x) \, dx = F(b) - F(a) = 2022 - 10 = \boxed{2012}\)
Solve for x. Round to the nearest tenth, if necessary.
1.8
P
х
1400
o

Answers
Answer:
13.6
Step-by-step explanation:
Mr. Williams asks to buy 12 of a pan of brownies that is 23 full.
Answers
Answer:
10 i think thats not really a questuon tho
work out the total surface area of this triangular prism 12cm by 5cm by 10cm by 13cm
Answers
To calculate the total surface area of a triangular prism, we need to find the area of each face and add them up.
The triangular faces have base 5cm and height 12cm, so each one has an area of:
(1/2) x 5cm x 12cm = 30cm²
There are two of these faces, so their combined area is:
2 x 30cm² = 60cm²
The rectangular faces have dimensions 5cm x 10cm and 5cm x 13cm, so their areas are:
5cm x 10cm = 50cm²
5cm x 13cm = 65cm²
Again, there are two of these faces, so their combined area is:
2 x (50cm² + 65cm²) = 230cm²
Finally, we add up the areas of all the faces to get the total surface area:
60cm² + 230cm² = 290cm²
Therefore, the total surface area of this triangular prism is 290cm².
Answer this question

Answers
Answer:
The answer is 18
Step-by-step explanation:
\(\left[\begin{array}{cc}2&3\\6&4\end{array}\right] \left[\begin{array}{ccc}4&2&1\\7&5&3\end{array}\right]\)
\([2*4+3*7][2*2+3*5][2*1+3*3]\\\[6*4+4*7][6*2+4*5][6*1+4*3]\)
\([8+21][4+15][2+9]\\\[24+28][12+20][6+12]\)
\([29][19][11]\\\[52][32][18]\)
\(\left[\begin{array}{ccc}29&19&11\\52&32&18\end{array}\right]\)
18 the only answer choice that appears.
Hope this helps!
Which of the following is the maximum value of the equation y = −x2 − x + 6?
Answers
Step-by-step explanation:
f(x) = y= -x²-x+6
When y is max, f'(x) = 0
f'(x) = -2x-1
Equating to 0,
-2x-1= 0
x= -1/2
Put the value of x in f(x)
y = -(-1/2)² -(-1/2) +6
= -1/4+1/2+6
= 6+1/2
y= 13/2
8. If a=-10, b-4, show that a - b ≠ b - a
Answers
Given : a = -10 and b = -4
To prove : a - b ≠ b - a
Proof : To put the values according to question,
-10 - (-4) = -4 - (-10)-10 + 4 = -4 + 10-6 ≠ 6Hence verified
Find the square root of 1681
Answers
Answer: The square root of 1681 is 41.
Answer:
41
Step-by-step explanation:
It is 41
41 * 41 = 1681
41 ^ 2 = 1681
Medical records indicate that people with more education tend to live longer; the correlation is 0.48. The slope of the linear model that predicts lifespan from years of education suggests that on average people tend to live 0.8 extra years for each additional year of education they have. The slope of the line that would predict years of education from lifespan is
Answers
Answer:
0.288
Step-by-step explanation:
Given that :
Correlation (R) = 0.48
Slope of linear model which predicts Lifespan from years of education (m) = 0.8
To determine the value of slope of the model which predicts years of eductauoon from lifespan:
The square of the regression Coefficient is multiplied by the inverse of the slope of linear model which predicts Lifespan from years of education
Hence,
(R² * 1/m)
0.48² * 1/0.8
0.2304 * 1.25
= 0.288
Solve: log2(x-1)+log2(x+5)=4
Answers
Answer: X=3
Step-by-step explanation:
Solve the compound - 20 ≤ 2x - 20 ≤ 10

Answers
Answer:
0≤ x ≤ 15
Step-by-step explanation:
- 20 ≤ 2x - 20 ≤ 10-20 + 20 ≤ 2x - 20 + 20 ≤ 10 + 20 ⇒ add 200 ≤ 2x ≤ 30 ⇒ divide by 20≤ x ≤ 15 ⇒ answeror x = [0,15]

What does 1/4+2/3 equal
Answers
Answer:
\(\frac{11}{12}\)
Step-by-step explanation:
\(Rule: \frac{x}{y} \pm \frac{a}{b} = \frac{x \pm y}{z}\\\frac{1}{4}+\frac{2}{3} = \frac{3}{12}+\frac{8}{12} (LCM)\\=\frac{3+8}{12}\\=\frac{11}{12}\\\)
Simplify the expression 2y+8(y+1)+5(x+5)
Answers
Answer:
\(7x+8y+33\)
Step-by-step explanation:
\(2y+8(y+1)+5(x+5)\\\\2y+(8)(y)+(8)(1)+(5)(x)+(5)(5)\\\\2y+8y+8+5x+25\\\\7x+8y+33\)