In the above triangle, would you use SINE, COSINE, or TANGENT to solve for the missing side (TYPE IN ALL CAPS) The measure of the missing angle is (ROUND TO THE NEAREST DEGREE). 1 2 3 4 5 6 7 8 9

Answers
Once we know the measure of the unknown angle, we can relate its value with the opposite side which measure 10 units and the hypotenuse of 39 units by means of the cosine and tangent functions, that is,
\(\begin{gathered} \cos \text{ ? =}\frac{\text{ adjacent side to ?}}{\text{hypotenuse}}=\frac{x}{39} \\ \tan \text{ ? =}\frac{\text{ opposite side to ?}}{\text{adjacent side to ?}}=\frac{10}{x} \end{gathered}\)where x denotes the missing side.
Would you use SINE, COSINE or TANGENT to solve for the missing side? Answer: COSINE and TANGENT.
Now, in order to find the missing angle, we must use the sine function because it relates the leg 10 with the hypotenuse of 39 units, that is,
\(\sin \text{ ? =}\frac{\text{ opposite side to ?}}{\text{hypotenuse}}=\frac{10}{39}\)which gives
\(\text{ sin ? =}0.2564\)So, by applying the inverse sine function to both sides, we have
\(\begin{gathered} \text{ ? =sin}^{-1}(0.2564) \\ \text{ ? = 14.857 degre}es \end{gathered}\)Therefore, by rounding to the nearest degree, the measure of the missing angle is 15 degrees
Related Questions
4/5(x+1) write the expression in standard form
Answers
Explanation: Hope this helps
what are two decimals that are equivalent to 2.200
Answers
Answer:
2.200 = 2.2 = 2.20 = 2.2000 = 2.20000...I could go on...
Step-by-step explanation:
With the growth of internet service providers, a researcher decides to examine whether there is a correlation between cost of internet service per month and degree of customer satisfaction (higher scores mean high satisfaction). The researcher only includes programs with comparable types of services. Calculate the Pearson's correlation coefficient for the dataset below and interpret what that means.
Answers
Answer:
The correlation is 0.373 . There is a moderate positive linear association between Dollars and Satisfaction.
Step-by-step explanation:
Given the data:
Dollars Satisfaction
70.99 32
76.01 34
67.24 27
69.33 17
67.59 23
74.06 29
76.04 25
72.63 31
68.01 26
67.98 31
Using technology, the Pearson correlation Coefficient calculator ; we can obtain the Pearson correlation Coefficient is 0.3729
Interpretating this value, a correlation Coefficient value of 0.3729 is positive, this means a positive linear relationship exists between the values. Also 0.3729 is closer to 0 than 1 ; this mean that the strength of their relationship is moderate to weak .
Hence, a correlation Coefficient value of 0.3729 means that a weak positive relationship exists between dollar and satisfaction
In a survey of 2,800 people who owned a certain type of car, 560 said they would buy that type of car again. what percent of the people surveyed were satisfied with the car?
Answers
20%
2800/560
= 0.2%
=20%
What's the area of the composite figure?

Answers
Answer:
\(\huge\boxed{\bf\: 83 \: yd^{2}}\)
Step-by-step explanation:
Consider rectangle ABCD.
Here,
AB = DC = 9 yd (length = l)
AD = BC = 7 yd (width = w)
Area of this rectangle:
= l * b
= 9 * 7
= 63 yd²
\(\rule{150pt}{2pt}\)
Now, take rectangle DEGF.
Here,
DE = FG = 5 yd (length = l)
DF = EG = 4 yd (width = w)
Area of this rectangle:
= l * b
= 5 * 4
= 20 yd²
\(\rule{150pt}{2pt}\)
Total area of the compisite figure:
= Area of rectangle ABCD + Area of rectangle DEGF
= 63 yd² + 20 yd²
= 83 yd²
\(\rule{150pt}{2pt}\)
Refer to the attachment for the labelling of the figure.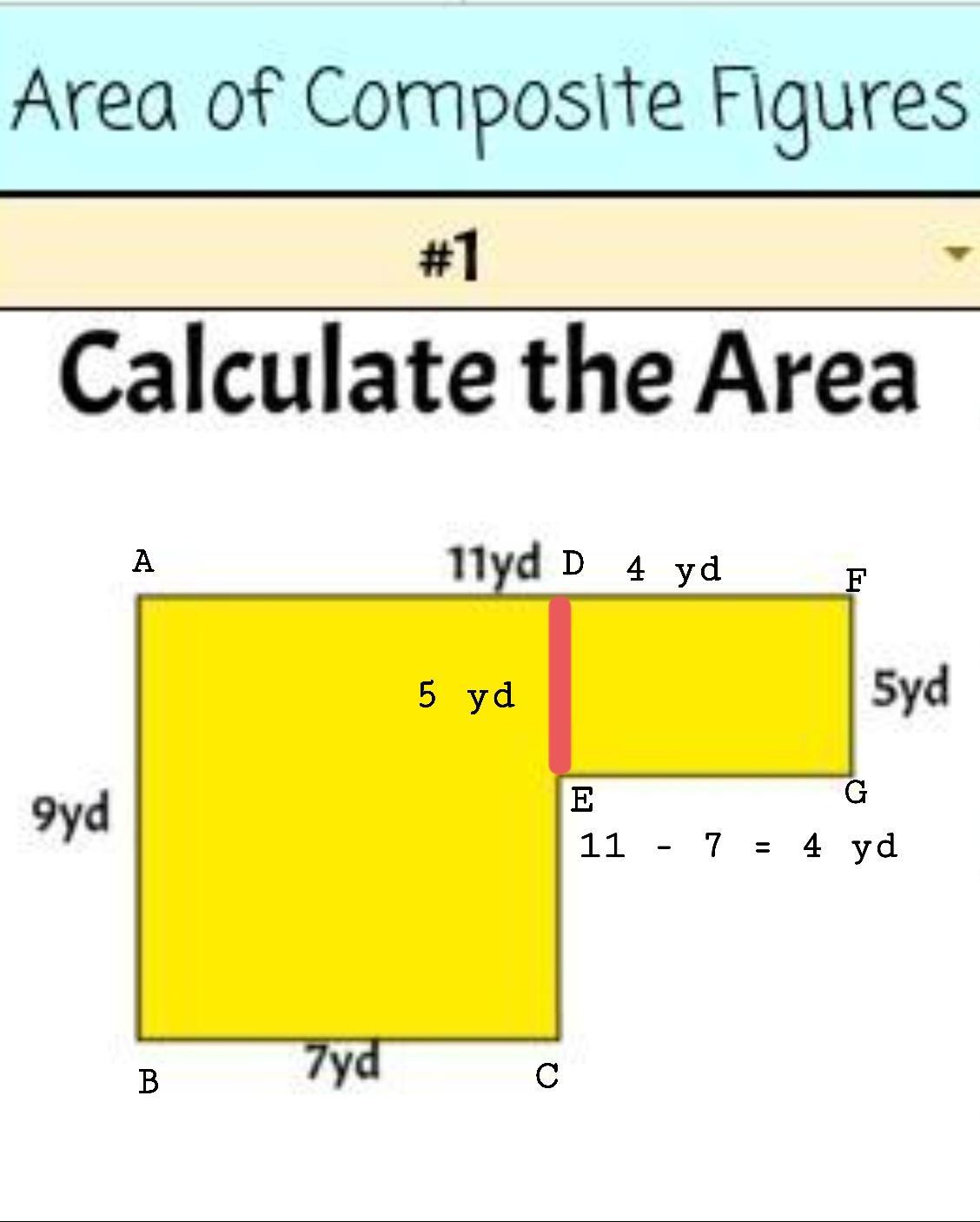
The domain of this emath equation is

Answers
Answer:
x ≥ 5
Step-by-step explanation:
You want the domain of the equation y = √(x -5) -1.
DomainThe domain of the function is the set of x-values for which it is defined. The square root is not defined for negative values, so the domain is ...
x -5 ≥ 0
x ≥ 5 . . . . . . the domain of this function
__
Additional comment
The range is the set of y-values the function may produce. Here, that set of values is y ≥ -1.
<95141404393>
Write the given expression in the form f(x) = a(x - h)² + k. Identify the vertex.
f(x)=3x²-24x-5
(Simplify your answer. Use integers or fractions for any numbers in the expression.)
Answers
The coordinate of the vertex of the given equation is: (4, -53)
What is the vertex of the quadratic expression?The given form of expression in vertex form is given as:
f(x) = a(x - h)² + k
where:
(h, k) is the coordinate of the vertex
The given quadratic equation is expressed as:
f(x) = 3x² - 24x - 5
Using completing the square, we can rewrite the given equation as:
3(x - 4)² - 53
Setting f(x) equal to it gives us:
f(x) = 3(x - 4)² - 53
Using the vertex form f(x) = a(x - h)² + k, we can say that the values of a, h and k are: 3, 4 and -53
Thus, (h, k) = (4, -53)
Read more about Vertex of quadratic expression at: https://brainly.com/question/1480401
#SPJ1
A certain medication is available only in 400 μg tablets. If the patient needs to take 2.4 mg per day, how many tablets must she take? Help me please. SIMPLIFY YOUR ANSWER. Expert only.
Answers
Answer: 6 tablets.
Step-by-step explanation:-Given:
• A certain medication is available only in 400 μg tablets.
So, in milligrams :- 0.4 mg
• It is also given that the patient needs to take 2.4 mg per day.
Solution:
So, in order to know how many tablets she should take, please do as following:-
\( \frac{2.4}{0.4} \)
\( = > \: 6\)
Hence, she must take 6 tablets per day.
✍️ By Benjemin ☺️
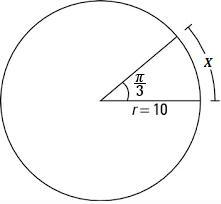
For the following, find the arc length:
(Use \large \pi=3.14 and round your final answer to the hundredths.)
x=_________
Answers
Answer:
x = 10.47
Step-by-step explanation:
circumference = 2 * 10 * π = 20π
centre angle of a circle (in radiant) = 2π
x : 20π = π/3 : 2π
x = (20π * π/3)/ 2π
x = 10 * π/3
x = 10π/3
x = 10.466667
x = 10.47
3x² + 2x + 2 + 4x² + 5
Answers
Answer:
7x^2 + 2x + 7
Hope this helps :)
Answer:
7x²+2x+7
Step-by-step explanation:
Hope this helps. Plz give brainliest.
Seventy-five 6th- grade students chose to watch a movie on the last day of school. This is 25% of the 6th-grade class. How many total students are in the 6th grade?
Answers
25% = 75/x
To solve for x, we can cross-multiply:
0.25x = 75
Then, we can divide both sides by 0.25:
x = 300
Therefore, there are 300 6th-grade students in total.
Given that a function, g, has a domain of -1 ≤ x ≤ 4 and a range of 0 ≤ g(x) ≤ 18 and that g(-1) = 2 and g(2) = 8, select the statement that could be true for g.
Answers
The correct statement regarding the function g(x), considering it's domain and range, is given as follows:
D. g(3) = 18.
What are the definitions of the domain and the range of a function?Before knowing the definition of the domain and the range of a function, the input and the output of a function must be identified. In this function, they are given as follows:
Input: x.Output: g(x).The domain of a function is composed by all the values assumed by the input of the function. Hence, in this problem, g(x) can only be calculated for values of x that are between -1 and 4, inclusive. g(-5) and g(5), for example, cannot be calculated.
The range of a function is composed by all the values assumed by the output of the function. Hence, in this problem, the values assumed by g(x) must be between 0 and 18, inclusive, thus values such as g(x) = -1 or g(x) = 19 are not valid.
Thus the correct statement is given as follows:
g(3) = 18.As:
x = 3 is between -1 and 4.g(x) = 18 is between 0 and 18, inclusive.It does not contradict any of g(-1) = 2 and g(2) = 8, such as option c g(2) = 4 did.Missing informationThe options are given as follows:
A. g(5) = 12.
B. g(1) = -2.
C. g(2) = 4.
D. g(3) = 8.
More can be learned about the domain and the range of a function at https://brainly.com/question/10197594
#SPJ1
Stefan sells Jin a bicycle for $176 and a helmet for $16. The total cost for Jin is 160% of what Stefan spent originally to buy the bike and helmet. How much did Stefan spend originally? How much money did he make by selling the bicycle and helmet to Jin?
Answers
Answer:
Stefan originally paid $120 for the bicycle and helmet.
He made $72 by selling them to Jin.
Step-by-step explanation:
Given that:
Selling price of bicycle = $176
Selling price of helmet = $16
Total price = 176+16 = $192
This is 160% of what Stefan paid originally.
Let,
x be the original amount paid by Stefan.
160% of x = 192
\(\frac{160}{100}x=192\\1.6x=192\)
Dividing both sides by 1.6
\(\frac{1.6x}{1.6}=\frac{192}{1.6}\\x=120\)
Original amount paid by Stefan = $120
Profit = 192 - 120 = $72
Hence
Stefan originally paid $120 for the bicycle and helmet.
He made $72 by selling them to Jin.
what is the area of this parallelogram?
the answers i have are
A=19 and a half ft2
A=42 ft2
A=61 and a half ft2
A=71 and 3 fourths ft2

Answers
Answer:
71.75
Step-by-step explanation:
Base x height (hope this helped)
Which equation shows the distributive property?
a = b + c
a(b + c) = ab + ac
ab – bc = ac
ab(c) = a + b + c
Answers
Answer:
The second equation which is a(b+c) = ab + ac.
Step-by-step explanation:
Remember that when you think of the word distribute, you want to see a variable or a number behind the parentheses. The fourth equation would not work despite there being distributive in which it will give you abc. The second equation is a great example of what a distributive property problem may do.
Maddie has $50. she worked for five days and she now has $270. what integer represents the average increase in value PER DAY
Answers
a = 2, b = 3, c =4 than find the value of ab bc ca
Answers
To find the values of ab, bc, and ca, we simply need to multiply the corresponding variables.
ab = 2 * 3 = 6
bc = 3 * 4 = 12
ca = 4 * 2 = 8
Therefore, ab = 6, bc = 12, and ca = 8.
3 • 4=12
4 • 2=8
ab=6
bc=12
ca=8
The slope of MN¯¯¯¯¯¯¯ is −3.
Which segments are parallel to MN¯¯¯¯¯¯¯?
Select each correct answer.
Responses
WX¯¯¯¯¯¯, where W is at (2, 6) and X is at (4, 0)
segment W X, , where , W, is at , begin ordered pair 2 comma 6 end ordered pair, and , X, is at , begin ordered pair 4 comma 0 end ordered pair
RS¯¯¯¯¯, where R is at (1, 3) and S is at (4, 2)
segment R S, , where , R, is at , begin ordered pair 1 comma 3 end ordered pair, and , S, is at , begin ordered pair 4 comma 2 end ordered pair
TU¯¯¯¯¯, where T is at (8, 1) and U is at (5, 10)
segment T U, , where , T , is at , begin ordered pair 8 comma 1 end ordered pair, and, U, is at , begin ordered pair 5 comma 10 end ordered pair
PQ¯¯¯¯¯, where P is at (5, 6) and Q is at (8, 7)
Answers
TU is parallel to MN.
Given that the slope of a line MN is -3, we need to find a line which is parallel to the MN.
So we know that the parallel lines are having equal slope so the line which is parallel to the MN it must have slope of -3.
Therefore,
Considering the line TU, find the slope = y₂-y₁ / x₂-x₁
= 10-1 / 5-8 = -9/3 = -3
Since, the slope of the line TU is -3 therefore we can say the lines TU and MN are parallel liens.
Hence TU is parallel to MN.
Learn more about slopes, click;
https://brainly.com/question/3605446
#SPJ1
The slope of the line with equation y = ax + b is greater than the slope of the line with equation y = cx + b. Which of the following
statements must be true about the relationship between a and c?
Case
O a
0 a=c
O a>c
O a ≥c+1
Answers
Answer: a > c
Step-by-step explanation:
(please respond if I'm wrong)
The slope of the line with equation y = ax + b is greater than the slope of the line with equation. y = cx + b. a ≥ c + 1, and a 0 a=c don't make a lot of sense, since it says greater than the slope of y = cx + b.
The only answer that makes most sense is a > c, since a is greater than c.
Hope this helps
On Monday, a baker made cookies. He had enough cookies to completely fill 2
equal-sized trays. He sells the cookies for $3 each.
2 3 4 5
12
At the end of the day on Monday, the trays are pictured above. How much mone
did the baker earn selling cookies on Monday?
10
4 78910
12
Answers
Answer:
Step-by-step explanation:
it is my first time doing dis so it is 12.
PLEASE HELP!!!
I need help with this math homework

Answers
9514 1404 393
Answer:
x = 14√2y = 14z = 14√3Step-by-step explanation:
These are two "special triangles." The ratios of side lengths are numbers not difficult to remember. They can save a lot of work in problems like this.
Ratio of side lengths in 45°-45°-90° isosceles right triangle: 1 : 1 : √2.
Ratio of side lengths in 30°-60°-90° right triangle: 1 : √3 : 2.
__
The side marked x has the same length as the side marked 14√2.
x = 14√2
The unmarked hypotenuse of both triangles is √2 times the marked side length, so is (14√2)(√2) = 28.
The side marked y is half the length of the hypotenuse of that triangles so is ...
y = 28/2
y = 14
And the side marked z is √3 times the short side of the 30°-60°-90° triangle, so is ...
z = 14√3
_____
Alternate solution
The mnemonic SOH CAH TOA can help you remember trig relationships. Here, 14√2 is opposite the given 45° angle, and x is adjacent. The trig relation is ...
Tan = Opposite/Adjacent
tan(45°) = 14√2/x
x = 14√2/tan(45°) = 14√2
__
To find y or z, we need to know the length of the hypotenuse in the 45° triangle. The given side is opposite the angle, so we can use the sine relation:
Sin = Opposite/Hypotenuse
hypotenuse = 14√2/sin(45°) = 28
Now, we can find y and z:
sin(30°) = y/hypotenuse
y = (28)sin(30°) = 14
cos(30°) = z/hypotenuse
z = (28)cos(30°) = 14√3
How do you change six-hundred-three-thousandths to a fraction?
Change 0.603 to a fraction. ----------------

Answers
Answer:
Step-by-step explanation:
Step-by-Step Solution
0.603 = 603/1000
as a fraction
To convert the decimal 0.603 to a fraction, just follow these steps:
Step 1: Write down the number as a fraction of one:
0.603 = 0.603/1
Step 2: Multiply both top and bottom by 10 for every number after the decimal point:
As we have 3 numbers after the decimal point, we multiply both numerator and denominator by 1000. So,
0.603/1
= (0.603 × 1000)/(1 × 1000) = 603/1000
.
(This fraction is alread reduced, We can't reduce it any further).
Answer: your answer is 0.603 Over 1000
603\1000
603
1000
Expressed as a single fraction, 3/4x - 2/5x is equal to
Answers
The single fraction is 7x/20
What is a fraction?A fraction can be defined as the part of a whole variable, number or element.
The different types of fractions are;
Simple fractionsMixed fractionsProper fractionsComplex fractionsImproper fractionsWhat are algebraic expressions?Algebraic expressions are mathematical expressions consisting of terms, coefficients, variables, factors and constants.
They are made of operations such as multiplication, addition, subtraction, division, etc
Given the expression;
3/4x - 2/5x
Find the LCM
15x - 8x/20
7x/20
Thus, the value is 7x/20
Learn more about fractions here:
https://brainly.com/question/11562149
#SPJ1
You borrow 15,000 for three years at 10% how much will you owe in interest only
Answers
Answer:
4500
Step-by-step explanation:
15000/10 is 1500, 1500 x 3 = 4500
Convert the measurement as indicated.
16 yd to feet
16 yd = ft
Answers
16yd x 3ft
=48ft
Cody and Tyler were once the same height. Since then, Tyler's height has increased by 20%, while Cody has grown half as much. If Tyler is 4 feet tall now, how many inches tall is Cody now? 30 POINTS!! :) please show work!!
Answers
answer: he is really short lol (JK ignore this answer, look at the answer below)
Answer:
24 inches
Step-by-step explanation:
how many inches tall is Cody now?
since Cody grew half as much he is 2 feet tall now, which is 24 inches(it's asking for the answer in inches so do not put feet).
1 feet = 12 inches.
2 feet = 24 inches.
Ace Carlos
Answer:
43.2 inches
Step-by-step explanation:
if tyler has grown by 20℅ and cody is half as much then cody is 10℅.
if tyler is 4 feet then,
4 feet = 100℅
x. = 20℅
x = 20℅ × 4 feet /100℅
x = 0.8 feet
if cody has grown half as much, 0.8/2 = 0.4feet
if they were equal once to find Cody's height we can, 4feet-0.4feet = 3.6 feet = 43.2 inches
#22Graph the function and tell wether or not it has a point of discontinuity at x = 0. If there is a discontinuity, tell wether it is removable or non removable.

Answers
Answer:
Removable discontinuity at x=0.
Step-by-step explanation:
By looking at the denominator of h(x), there will be a discontinuity.
Since the denominator cannot be zero, x has to be different from 0. Therefore, there is a discontinuity at x=0.
Now, to determine what type of discontinuity, check if there is a common factor in the numerator and denominator of the function. If there is an existent common factor, there is a removable discontinuity or a hole.
\(\begin{gathered} h(x)=\frac{x^3+x}{x} \\ h(x)=\frac{x(x^2+1)}{x}=x^2+1 \end{gathered}\)There is a removable discontinuity, or a hole, at x=0.
Please help me with this proof.

Answers
Answer:
See below
Step-by-step explanation:
For the second step, \(\angle T\cong\angle R\) by Alternate Interior Angles. The rest of the steps appear to be correct.
Tammy writes the equation -1 / 8 = -1/8. Is she correct? Explain.
Answers
The equation -1/8 = -1/8 means that both sides of the equation have the same value, which they do.
The negative sign in front of the fractions means that they are less than zero. The fraction 1/8 means one divided by eight, which is a small quantity.
So, -1/8 means a small negative quantity and -1/8 means the same small negative quantity.
Therefore, Tammy's equation is true and both sides are equal.
What is the equation, in point-slope form, of the line that is parallel to the given line and passes through the point (-3, 1)?

Answers
Answer:
y-1= -1/3(x+3)
Step-by-step explanation:
y-y1=m(x-x1)
y-1=m(x+3)
the slope is rise over run
the slope is -1/3
Answer:
y - 1 = 3/2 (x + 3)
Step-by-step explanation:
To find the equation of a line parallel to the given line and passing through the point (-3, 1), we can use the point-slope form of a linear equation. The point-slope form is given by:
y - y₁ = m(x - x₁)
Where (x₁, y₁) is the given point and m is the slope of the line.
First, let's calculate the slope of the given line using the two points (-2, -4) and (2, 2):
slope = (y₂ - y₁) / (x₂ - x₁)
= (2 - (-4)) / (2 - (-2))
= 6 / 4
= 3/2
Since the line we want to find is parallel to the given line, it will have the same slope. Therefore, the slope (m) of the new line is also 3/2.
Now we can substitute the values into the point-slope form using the point (-3, 1):
y - 1 = (3/2)(x - (-3))
y - 1 = (3/2)(x + 3)
The equation in point-slope form of the line parallel to the given line and passing through the point (-3, 1) is:
y - 1 = 3/2 (x + 3)