In a right triangle,the altitude q from the right angle to the hipotenuse divides..........

Answers
Then we have the next equivalence
\(\frac{4}{q}=\frac{q}{16}\)then we need to isolate the q
\(\begin{gathered} q^2=4\cdot16 \\ q^2=64 \\ q=\sqrt[]{64} \\ q=8 \end{gathered}\)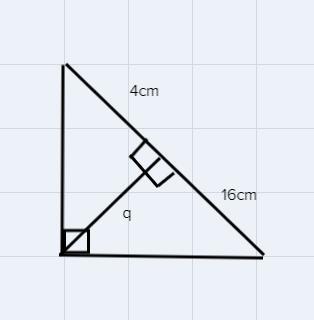
Related Questions
Find a power series for the function, centered at c, and determine the interval of convergence. f(x) = 9 3x + 2 , c = 6
Answers
Answer:
\(\frac{9}{3x + 2} = 1 - \frac{1}{3}(x - \frac{7}{3}) + \frac{1}{9}(x - \frac{7}{3})^2 - \frac{1}{27}(x - \frac{7}{3})^3 ........\)
The interval of convergence is:\((-\frac{2}{3},\frac{16}{3})\)
Step-by-step explanation:
Given
\(f(x)= \frac{9}{3x+ 2}\)
\(c = 6\)
The geometric series centered at c is of the form:
\(\frac{a}{1 - (r - c)} = \sum\limits^{\infty}_{n=0}a(r - c)^n, |r - c| < 1.\)
Where:
\(a \to\) first term
\(r - c \to\) common ratio
We have to write
\(f(x)= \frac{9}{3x+ 2}\)
In the following form:
\(\frac{a}{1 - r}\)
So, we have:
\(f(x)= \frac{9}{3x+ 2}\)
Rewrite as:
\(f(x) = \frac{9}{3x - 18 + 18 +2}\)
\(f(x) = \frac{9}{3x - 18 + 20}\)
Factorize
\(f(x) = \frac{1}{\frac{1}{9}(3x + 2)}\)
Open bracket
\(f(x) = \frac{1}{\frac{1}{3}x + \frac{2}{9}}\)
Rewrite as:
\(f(x) = \frac{1}{1- 1 + \frac{1}{3}x + \frac{2}{9}}\)
Collect like terms
\(f(x) = \frac{1}{1 + \frac{1}{3}x + \frac{2}{9}- 1}\)
Take LCM
\(f(x) = \frac{1}{1 + \frac{1}{3}x + \frac{2-9}{9}}\)
\(f(x) = \frac{1}{1 + \frac{1}{3}x - \frac{7}{9}}\)
So, we have:
\(f(x) = \frac{1}{1 -(- \frac{1}{3}x + \frac{7}{9})}\)
By comparison with: \(\frac{a}{1 - r}\)
\(a = 1\)
\(r = -\frac{1}{3}x + \frac{7}{9}\)
\(r = -\frac{1}{3}(x - \frac{7}{3})\)
At c = 6, we have:
\(r = -\frac{1}{3}(x - \frac{7}{3}+6-6)\)
Take LCM
\(r = -\frac{1}{3}(x + \frac{-7+18}{3}+6-6)\)
r = -\frac{1}{3}(x + \frac{11}{3}+6-6)
So, the power series becomes:
\(\frac{9}{3x + 2} = \sum\limits^{\infty}_{n=0}ar^n\)
Substitute 1 for a
\(\frac{9}{3x + 2} = \sum\limits^{\infty}_{n=0}1*r^n\)
\(\frac{9}{3x + 2} = \sum\limits^{\infty}_{n=0}r^n\)
Substitute the expression for r
\(\frac{9}{3x + 2} = \sum\limits^{\infty}_{n=0}(-\frac{1}{3}(x - \frac{7}{3}))^n\)
Expand
\(\frac{9}{3x + 2} = \sum\limits^{\infty}_{n=0}[(-\frac{1}{3})^n* (x - \frac{7}{3})^n]\)
Further expand:
\(\frac{9}{3x + 2} = 1 - \frac{1}{3}(x - \frac{7}{3}) + \frac{1}{9}(x - \frac{7}{3})^2 - \frac{1}{27}(x - \frac{7}{3})^3 ................\)
The power series converges when:
\(\frac{1}{3}|x - \frac{7}{3}| < 1\)
Multiply both sides by 3
\(|x - \frac{7}{3}| <3\)
Expand the absolute inequality
\(-3 < x - \frac{7}{3} <3\)
Solve for x
\(\frac{7}{3} -3 < x <3+\frac{7}{3}\)
Take LCM
\(\frac{7-9}{3} < x <\frac{9+7}{3}\)
\(-\frac{2}{3} < x <\frac{16}{3}\)
The interval of convergence is:\((-\frac{2}{3},\frac{16}{3})\)
HELP ME PLEASEE ASAPAPAP PLEASE PLEASE PLEASE PLEASE!!!!!!!!!!!

Answers
Answer: AEC = 80
X=30
YW ;)
I have 7 cookies. If I eat 1/4 of them of the cookies. How many cookies do I have left?
Answers
Answer:
6 thirds
Step-by-step explanation:
you're welcome
10. The volume of Cube A is 125 cubic inches. The face of Cube B has an area of 36 square inches.
Which cube has a greater side length?
Answers
Answer:
Cube B
Step-by-step explanation:
a = \(\sqrt[3]{125}\)
a = 5
6in x 6in = 36\(in^{2}\)
5 < 6 so A < B
The side length of Cube A ≈ 5 inches
The side length of Cube B = 6 inches
Cube B has a greater side length than Cube A.
We have,
To compare the side lengths of Cube A and Cube B, we need to find the side length of each cube.
For Cube A:
The volume of Cube A = side length³
125 = side length³
To find the side length of Cube A, we take the cube root of 125:
side length of Cube A = ∛125 ≈ 5 inches
For Cube B:
The area of one face of Cube B = side length²
36 = side length²
To find the side length of Cube B, we take the square root of 36:
side length of Cube B = √36 = 6 inches
Now, we can compare the side lengths of both cubes:
The side length of Cube A ≈ 5 inches
The side length of Cube B = 6 inches
Since 6 inches is greater than 5 inches, Cube B has a greater side length than Cube A.
Thus,
Cube B has a greater side length than Cube A.
Learn more about cubes here:
https://brainly.com/question/11168779
#SPJ7
please help image attached! x=?

Answers
Answer:
90°
Step-by-step explanation:
from the figure and the measurements it is a square, the diagonals are perpendicular and form 4 angles of 90°, so 90° is your answer.
Plz answer last question and im lost!

Answers
Answer:
\(\pi\) radian
Step-by-step explanation:
We know that angle for a full circle is 2\(\pi\)
In the given figure shape is semicircle
hence,
angle for semicircle will be half of angle of full circle
thus, angle for given figure = half of angle for a full circle = 1/2 * 2\(\pi\) = \(\pi\)
Thus, answer is \(\pi\) radian
alternatively, we also know that angle for a straight line is 180 degrees
and 180 degrees is same as \(\pi\) radian.
what are the similarities and differences in linear and exponential in intercepts?
what are the similarities and differences in linear and exponential in domain and range?
what are the similarities and differences in linear and exponential in asymptotes?
what are the similarities and differences in linear and exponential in misc.?
Answers
Answer:
What is a linear function? A linear function is a function whose graph is a straight line. The rate of change of a linear function is constant. The function shown in the graph below, y = x + 2, is an example of a linear function.
Graph of linear function
Graph of linear function
A linear function has a constant rate of change. The rate of change is the slope of the linear function. In the linear function shown above, the rate of change is 1. For every increase of one in the independent variable, x, there is a corresponding increase of one in the dependent variable, y. This gives a slope of 1/1 = 1.
A linear function is typically given in the form y = mx + b, where m is equal to the slope, or constant rate of change.
Examples of linear functions include:
If a person drives at a constant speed, the relationship between the time spent driving (independent variable) and the distance traveled (dependent variable) will remain constant.
Assuming no change in price, the relationship between the number of pounds of bananas a person buys (independent variable) and the total cost of the bananas (dependent variable) will remain constant.
If a person earns an hourly wage at their job, the relationship between the time spent working (independent variable) and the amount earned (dependent variable) will remain constant.
Save Timeline Autoplay Speed Normal
Video Quiz Course
71K views
Exponential Functions
What is an exponential function? An exponential function is a function that involves exponents and whose graph is a smooth curve. The rate of change in an exponential function is not constant. The functions shown in the graph below, y = 0.5x and y = 2x, are examples of exponential functions.
Graphs of exponential functions
Graphs of exponential functions
An exponential function does not have a constant rate of change. The rate of change in an exponential function is the value of the independent variable, x. As the value of x increases or decreases, the rate of change increases or decreases as well. Rather than a constant change, as in the linear function, there is a percent change.
An exponential function is typically given in the form y = (1 + r)x, where r represents the percent change.
Examples of exponential functions include:
Step-by-step explanation:
What tip would a waiter receive if a customer adds a 20% tip to a meal that costs $42.18?
Answers
Answer:
Step-by-step explanation:
the waiter would receive an $8.44 tip
Hope you his helps:)
Two cards are drawn from a deck of 52 cards. The first card is replaced before drawing the second card. Find the probability that the first card is red and the second card is a 7
Answers
The probability that the first card is red and the second card is a 7 is 1/26.
What is the probability?Probability determines the chances that an event would happen. The probability the event occurs is 1 and the probability that the event does not occur is 0.
Probability that the first card is a red and the second is a 7 = (number of red cards / total number of cards) x (number of 7 / total number of card)
Probability that the first card is a red and the second is a 7 = (26 / 52) x (4/52) = 1/26
To learn more about probability, please check: https://brainly.com/question/13234031
#SPJ1
can someone help me with this

Answers
ANSWER: 0,1 my friend have a good day
Which number is rational?
-2.101001001
-.8974512
1.2547569
5.3333333
Answers
Answer:
D
Step-by-step explanation:
Took the test
Si 10 obreros construyen un consultorio médico en 12 dias.¿Con cuántos obreros se hará la misma obra en 15 dias?
Answers
A total of 8 workers are required for a building time of 15 days.
How many workers are needed to complete a building?
In this problem we find a case of inverse relationship, in which the number of workers (n) is inversely proportional to building time (t), in hours. The situation is represented by following formulas:
n ∝ 1 / t
n = k / t
Where k is the proportionality ratio, which can be eliminated by building the following formula:
n₁ · t₁ = n₂ · t₂
If we know that n₁ = 10, t₁ = 12 and t₂ = 15, then the required number of workers is:
n₂ = n₁ · (t₁ / t₂)
n₂ = 10 · (12 / 15)
n₂ = 10 · (4 / 5)
n₂ = 8
To learn more on inverse relationship: https://brainly.com/question/30094619
#SPJ1
Solve for b
10, b, 150degrees, 12degrees

Answers
Hello!
We have all angles of the triangle:
We will use the law of cosines. This relation is valid for all sides of any t
We have:
angle A = 12°
côté a = 10
angle B = 150°
This is therefore the first case of application of the sine law.
So:
\(\sf \dfrac{b}{sin~B} = \dfrac{a}{sin~A}\)
\(\sf b =\dfrac{sin~B~*~a}{sin~A} = \dfrac{sin~150~*~10cm}{sin~12} = \dfrac{arcsin~0.5~*~10cm}{arcsin~0.2079116908} = \dfrac{30~*~10cm}{12} = \dfrac{300cm}{12} = \boxed{\sf25cm}\)
b = 25cm
Adding (4-7i)+(-11+9i)
Answers
your answer is -7+16i, because 4-11=-7 and 7i+9i is 16i
Find the coordinate vector [Bold x ]Subscript Upper B of x relative to the given basis B
Answers
Answer:
coordinate value is [-3,4].
Reltive is mio pie x 78.9 :)
hoped this helped :)
What is 5% as a fraction?
Answers
Answer:
The answer would be 5/100 or 1/20 in it's simplest form
Step-by-step explanation:
So let's say you have only 5% of a pie and the rest of your family gets the rest, if you put that in a form of a fraction, the answer would be 5 over 100 (5/100). But if you simplify, the answer would be 1/20 since 5 goes into 5 once and 20 goes into 100 5 times.
Ignore the messy writing, i was in a hurry.

Which is an equation in slope intercept form of the line that passes through (1, 3) and (2, 4)?
Answers
Answer:
y = x + 2
Step-by-step explanation:
The slope-intercept equation is:
y = mx + b
First, you should use the point-slope formula to find the slope. Plug in the "x" and "y" values given from the points:
Point 1: (1,3) Point 2: (2,4)
y₁ - y₂ = m(x₁ - x₂) <--- Point-slope formula
(3 - 4) = m(1 - 2) <--- Plug in "x" and "y" values from points
-1 = -m <--- Simplify inside parentheses
1 = m <--- Divide both sides by -1
Finally, use one of the points to solve for the y-intercept (b):
Point 1: (1,3) m = 1
y = mx + b <--- Slope-intercept formula
3 = 1(1) + b <--- Plug in values from point and slope (m)
3 = 1 + b <--- Multiply slope and "x" value
2 = b <--- Subtract 1 from both sides
Since the slope = 1, it does not need to be included in the final formula. The final answer is:
y = x + 2
Use the function below to find F(2).

Answers
Answer:
Step-by-step explanation:
\(F(2)=\frac{1}[3} \cdot 4^{2}=\frac{16}{3}\)
Perimeters of Squares (cm) 8 7 6 5 4 3 2. 1 0 1 2 3 4 5 6 7 8 9 Length of Sides of Squares (cm) Look at this graph of data, If the graph represents the length of sides and the perimeter of different squares, what would be another ordered pair? A. (3,7) B. (12,3) C. (3,12) D. (4,12) Item #24084 HU

Answers
We have a linear function that relates length of sides of squares and perimeter of the squares.
We can derive the function knowing that the perimeter is the sum of the length of the sides. If a square has 4 equal sides, the perimeter will be 4 times the length side.
Then, if perimeter is y and side length is x, we will have the relation:
\(y=4x\)Then, we can test for each value of x, what is the value of the perimeter y:
\(\begin{gathered} A)x=3\longrightarrow y=4\cdot3=12\neq7 \\ B)x=12\longrightarrow y=4\cdot12=48\neq3 \\ C)x=3\longrightarrow y=4\cdot3=12 \\ D)x=4\longrightarrow y=4\cdot4=16\neq12 \end{gathered}\)Answer: The only correct ordered pair is (3,12) [Option C]
HELP ASAP! 20 POINTS
Translate the sentence into an equation
the sum of 3 times a number and 8 is 5
(use the variable x for the unknown number
Answers
Answer:
3(x+8)=5
Step-by-step explanation:
Hope it's correct and sorry if it's not
Answer:
8+5 (3 times x)
Step-by-step explanation:
Please mark me as brainliest
5x²-7x+4 FACTORISATION??
Answers
Answer:
The answer is given in attached file.
Step-by-step explanation:

please help me with this :D

Answers
Answer:
b=24
Step-by-step explanation:
the sides are equal to each other so
b+24=2b
24+24=2 times 24
48=48
so b=24
What is the equation of the line parallel to the line y=3/4x and passes through the point (-4,10)

Answers
Answer:
D. y = 3/4x + 13
Step-by-step explanation:
lmk if you want an explanation
Student Council has 1,064 flowers. They want to divide the flowers evenly among 28 centerpieces. How many flowers will be in each centerpiece?
Answers
To find out how many flowers will be in each centerpiece, we can divide the total number of flowers by the number of centerpieces:
Total number of flowers: 1,064
Number of centerpieces: 28
Number of flowers in each centerpiece = Total number of flowers / Number of centerpieces
Number of flowers in each centerpiece = 1,064 / 28 = 38
Therefore, there will be 38 flowers in each centerpiece.
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\textcolor{red}{\underline{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
Answer: 38
Step-by-step explanation:
The question is asking you to divided.
1064 divided by 28 = 38
So there will be 38 flowers in each centerpiece
which of these events are mutually exclusive if you roll a pair of dice?i. a: at least one die is a 3ii. b: at least one die is a 6iii. c: the sum is 11
Answers
When rolling a pair of dice, there are 36 possible outcomes, each representing a unique combination of the numbers on the two dice.
Event A: "at least one die is a 3" is not mutually exclusive with Event B: "at least one die is a 6" or Event C: "the sum is 11"
Event A and Event B are mutually exclusive because when one of the die is a 6, the other die cannot be a 3 and vice versa.
Event A and Event C are not mutually exclusive because there are some outcomes where at least one die is a 3 and the sum is 11. For example (3,8), (4,7) and (6,5) are all outcomes where at least one die is 3 and the sum is 11.
Event B and Event C are not mutually exclusive because there are outcomes where at least one die is a 6 and the sum is 11. For example (5,6) and (6,5) are both outcomes where at least one die is a 6 and the sum is 11.
So, in conclusion, Event A and Event B are mutually exclusive, but Event A and Event C and Event B and Event C are not mutually exclusive.
To know more about combination , click here:
brainly.com/question/29677982
#SPJ4
Consider the following question: How many years has each person played on the soccer team? Which best describes the question? It is a statistical question because the data may vary for each player. It is a statistical question because the data will all be the same. It is not a statistical question because the answers are all whole numbers. It is not a statistical question because there is only one answer per person.
Answers
Answer:
Its the first one
Step-by-step explanation:
It is a statistical question because the data may vary for each player. :)
Please answer as fast as you can.
what is 30 + 5(2x -1) + 20
Answers
Answer:
1 Collect like terms.
5(2x-1)+(30+20)
2 Simplify.
5(2x-1)+50
3 Expand by distributing terms.
10x-5+50
4 Collect like terms.
10x+(-5+50)
5 Simplify.
10x+45
Hope this helps!! <3
Answer:
10x + 55
Hope this helps :)
Let f(x, y) = x² + xy + y². What is the direction of minimum rate of change at point (5,2)? (Enter a vector, using [,] brackets, such that the first coordinate (x-coordinate) is 1, 0, or -1.) Components of vector of minimum change direction are
Answers
Answer:
Step-by-step explanation:
To find the direction of minimum rate of change for the function f(x, y) = x² + xy + y² at the point (5, 2), we need to find the gradient vector (also known as the gradient or the vector of partial derivatives) and then find the direction orthogonal to the gradient vector, as this direction will have the minimum rate of change.
First, let's find the gradient vector by computing the partial derivatives of f(x, y) with respect to x and y:
∂f/∂x = 2x + y
∂f/∂y = x + 2y
Now, evaluate the gradient vector at the point (5, 2):
∇f(5, 2) = (2(5) + 2, 5 + 2(2)) = (12, 9)
The gradient vector is (12, 9). To find the direction orthogonal to the gradient vector, we can swap the x and y components and negate one of them. Since the question asks for a vector with an x-component of 1, 0, or -1, we'll negate the x-component:
Orthogonal vector = (-1, 12)
So, the direction of minimum rate of change at point (5, 2) is given by the vector [-1, 12].
Noel paid $204 for 8 tickets to a soccer game. Each ticket cost the same amount.
What was the cost of each ticket in dollars and cents?
Answers
Answer:
$25.50
Step-by-step explanation:
8 tickets cost $204.
$204 ÷ 8 = $25.50
You randomly draw three cards from a normal deck of 52 cards. What is the probability that the first card is not a spade, thesecond card is a spade, and the third card is also a spade if you do not replace the first or second card? Enter your answeras a decimal rounded to the nearest thousandth.
Answers
Total number of cards in the normal deck is 52
Number of spades in the normal deck = 13
Probability of drawing a spade(first card) = 13/52 = 1/4
Probability of not drawing a spade(first card) = 1 - 1/4 = 3/4
Since the first card is not replaced,
number of cards left = 52 - 1 = 51
number of spades left = 13
Probability of drawing a spade(second card) = 13/51
Since the second card is not replaced,
total number of cards left = 51 - 1 = 50
number of spades left = 13 - 1 = 12
Probability that the third card is a spade = 12/50 = 6/25
Thus, the probability that the first card is not a spade, the second card is a spade, and the third card is also a spade = 3/4 x 13/51 x 6/25 = 0.046