if sally gets scores 73, 85, 93, the 4th score is 20 points less than 5th score which is 2/3 of the average of the first three. what must she score on the 6th and 7th test to average an 85
Answers
There is no solution that allows Sally to average an 85 with the given scores.
Let's start by finding the 5th score:
We know that the 4th score is 20 points less than the 5th score, so let's call the 5th score "x". Therefore, the 4th score is "x - 20".
We also know that the 5th score is 2/3 of the average of the first three scores. The average of the first three scores is (73 + 85 + 93)/3 = 83.67. So, (2/3) * 83.67 = 55.78 is the 5th score (rounded to two decimal places).
Therefore, x = 55.78/(2/3) = 83.67 is the 5th score.
Now, let's calculate the total score Sally needs to average an 85:
She already has 73 + 85 + 93 + 83.67 = 334.67 points. To average an 85 over seven tests, she needs a total of 7 * 85 = 595 points.
To find the scores she needs on the 6th and 7th tests, we can subtract the points she already has from the total points she needs:
595 - 334.67 = 260.33
So, Sally needs a total of 260.33 points on the 6th and 7th tests combined.
Let's call the 6th score "y" and the 7th score "z". We can set up an equation to solve for these two scores:
(y + z)/2 = 260.33
Multiplying both sides by 2:
y + z = 520.67
Since Sally needs to average an 85 over seven tests, the sum of all seven scores must be:
7 * 85 = 595
The sum of the first five scores is:
73 + 85 + 93 + 83.67 + 83.67 = 418.34
So, the sum of the last two scores must be:
595 - 418.34 = 176.66
Now we can set up another equation:
y + z = 176.66
We can solve this equation for one of the variables. Let's solve for "y":
y = 176.66 - z
Now we can substitute this into the first equation:
(176.66 - z + z)/2 = 260.33
176.66/2 = 260.33
88.33 = 260.33
This equation does not make sense.
To learn more about average here:
https://brainly.com/question/24057012
#SPJ4
Related Questions
can u help me on this i will mark u as brilliant student...

Answers
Answer:
hope this help you
have a great day

Answer:
i) The order of matrix is 2 ×3
ii)The order of matrix is 3×3
3)A square matrix in which each diagonal element is unit ,all other element being zero is called a unit matrix. for example ..Unit matrix is given in figure.. 4 )(1 +tan^2A).cos^2A=1 next step sec^2A×cos^2A next step 1 i.e (1+tan^2A=sec^2A) and (sec^2×cos^2A=1)
Please help me with this
Just bottom part

Answers
f(n)=5^n
f(4)=?
soln...
just put the value n=4
f(4)=5^4
=625.......
PLEASE HELP WILL MARK BRANLIEST!!!

Answers
The child can make 120 different bracelets using one of each charm. To solve this problem, we need to use a combinatorial approach.
The number of different bracelets that the child can make depends on the number of charms that can be used for each bracelet & the order in which they are arranged. Since each charm can be used only once, we have to choose five charms out of the total of five available charms, which can be done in 5C5 ways.
We can think of this problem as a permutation problem. There are five distinct charms, and we need to choose five of them to make a bracelet. The order in which we choose the charms matters, as each order gives us a different bracelet. Therefore, we need to use the formula for permutations.
The formula for permutations is given by:
nPr = n! / (n-r)!
where n is the total number of objects, and r is the number of objects we want to choose.
For this problem, n = 5 (since there are five distinct charms), and r = 5 (since we want to choose all five charms).
Plugging these values into the formula, we get:
5P5 = 5! / (5-5)! = 5! / 0! = 5 x 4 x 3 x 2 x 1 / 1 = 120
Therefore, the child can make 120 different bracelets.
Alternatively, we can also think of this problem as a multiplication principle problem. There are five distinct charms, & we need to choose one charm out of five for the first position, one charm out of four for the second position, one charm out of three for the third position, one charm out of two for the fourth position, & one charm out of one for the fifth position.
Using the multiplication principle, we can multiply these numbers together to get the total number of different bracelets:
5 x 4 x 3 x 2 x 1 = 120
Therefore, the child can make 120 different bracelets using one of each charm.
To know more about combinatorial approach-
brainly.com/question/30589727
#SPJ1
Can someone plz help me with this I need to get an 92 choose all that apply

Answers
Step-by-step explanation:
54, 54, 72
\(180 - 2 \times 54 = \\ = 180 - 108 = \\ = 72\)
What is (f + g)(x)?
f(x) = -3x + 6
g(x) = 2x?
Answers
Answer:
(f + g)(x) = -x + 6
Step-by-step explanation:
Step 1: Define
f(x) = -3x + 6
g(x) = 2x
Step 2: Find (f + g)(x)
(f + g)(x) = -3x + 6 + 2x
(f + g)(x) = -x + 6
Answer:
-x +6
Step-by-step explanation:
f(x) = -3x + 6
g(x) = 2x
(f + g)(x)= Add the two functions
= -3x + 6 + 2x
Combine like terms
= -x +6
what is 3 1/2x - 5 1/2x,x=2/5
Answers
Answer:
\(-\dfrac{4}{5}\)
Step-by-step explanation:
We have the following expression
\(3 \;\dfrac{1}{2} x - 5\;\dfrac{1}{2}x\)
and asked to evaluate this expression at \(\[x = {2\over\displaystyle 5\]\)
First convert \(3 \; \dfrac{1}{2}\) and \(5 \; \dfrac{1}{2}\) to mixed fractions
\(3 \; \dfrac{1}{2} = \dfrac{3 \times 2 + 1}{2} = \dfrac{7}{2}\\\)
\(5 \; \dfrac{1}{2} = \dfrac{5 \times 2 + 1}{2} = \dfrac{11}{2}\)
Therefore
\(3 \;\dfrac{1}{2} x - 5\;\dfrac{1}{2}x\\\\= \dfrac{7}{2}x - \dfrac{11}{2}x\\\\= \dfrac{7 - 11}{2} x\\\\= -\dfrac{4}{2}x \\\\= -2x\\\\\)
\(\mathrm{For \;x =\dfrac{2}{5}},\\\\= -2x \\= - 2 \times \dfrac{2}{5}\\\\= -\dfrac{4}{5}\)
4. A pizza shop has 12" pizzas with 6 slices and 16" pizzas with slices. Which pizza has bigger slices?
Answers
Subtract
1/13
31
55
62
110
2
3/5
55
7
10
-
3
22. Simplify the answer.
Answers
When we subtract 3 from 22, the simplified answer is 19. The subtraction operation involves removing or deducting one value from another, resulting in the difference between the two quantities.
To subtract 3 from 22, we can perform the subtraction operation as follows:
22
3
19
We align the numbers vertically and subtract each corresponding place value from right to left. In this case, subtracting 3 from 2 requires borrowing or regrouping. However, since 2 is greater than 3, we can directly subtract 3 from 2 and write the difference, which is 1, in the one's place.
Therefore, the simplified answer is 19.
The subtraction process involves taking away or removing a certain quantity from another. In this case, we subtracted 3 from 22, resulting in a difference of 19. The process of simplifying the answer is simply expressing the result in its most concise and reduced form.
By subtracting 3 from 22, we removed 3 units from the original value of 22, leaving us with 19. This can be visualized as taking away three objects from a group of 22 objects, resulting in a remaining count of 19.
In summary, when we subtract 3 from 22, the simplified answer is 19. The subtraction operation involves removing or deducting one value from another, resulting in the difference between the two quantities.
for such more question on simplified
https://brainly.com/question/11680269
#SPJ8
Find the equation for the plane through Po(-4,5,-1) perpendicular to the following line.
x=-4-t, y=5+3t, z=-5t, -[infinity]o
Using a coefficient of 1 for x, the equation of the plane is
Answers
Given point and line are Po(-4, 5, -1) and x = -4 - t, y = 5 + 3t, z = -5t, -[infinity]o respectively. To find the equation for the plane through Po(-4,5,-1) perpendicular to the line, we will use the following steps First, we will calculate the direction vector for the given line.
We know that the direction ratios of the line are (-1, 3, -5)Therefore, the direction vector of the line is given as V1 = (-1, 3, -5) We know that the given plane is perpendicular to the given line and passes through the given point, therefore the normal vector of the plane is equal to the direction vector of the given line.Let the normal vector of the plane be V2 = (a, b, c) = V1 = (-1, 3, -5) Therefore, a = -1, b = 3, and c = -5.
Now, we will use the equation of the plane in the normal form that is (a, b, c) . (x - x1, y - y1, z - z1) = 0Here, (x1, y1, z1) = (-4, 5, -1)Therefore, the equation of the plane is (-1, 3, -5) . (x + 4, y - 5, z + 1) = 0 Simplifying the above equation, we get the following equation:: The equation of the plane through Po(-4,5,-1) perpendicular to the given line is x + 3y - 5z + 32 = 0.:Given point and line are Po(-4, 5, -1) and x = -4 - t, y = 5 + 3t, z = -5t, -[infinity]o respectively. To find the equation for the plane through Po(-4,5,-1) perpendicular to the line, we will use the following steps.Step 1: First, we will calculate the direction vector for the given line.
To know more about line visit :
https://brainly.com/question/2696693
#SPJ11
If in ∆ABC, the bisectors of ∠B and ∠C intersect each other at O. Prove that ∠BOC = 90° + 1/2∠ A.
ps- pls help istg

Answers
It has been proved that if the bisectors of ∠B and ∠C intersect each other at O, then; ∠BOC = 90°+ ¹/₂∠A
How to prove angles?A △ ABC such that the bisectors of ∠ ABC and ∠ ACB meet at a point O.
To prove :
∠BOC = 90° + ¹/₂∠A
Proof :
In △BOC, we have
∠1 + ∠2 + ∠BOC = 180°
In △ ABC, we have, ∠A + ∠B + ∠C = 180°
∠A + 2(∠1) + 2(∠2) = 180°
¹/₂∠A + ∠1 + ∠2 = 90°
∠1 + ∠2 = 90° - ¹/₂∠A
Therefore, in equation 1,
90° - ¹/₂∠A + ∠BOC = 180°
∠BOC = 90°+ ¹/₂∠A
Read more about Proving Angles at; https://brainly.com/question/13878372
#SPJ1
PLEASE HELP IK THIS ISN'T MATH BUT PLEASE HELP ITS TIMED

Answers
brainliest? if not, that’s okay. :)
PLEASE ANSWER THIS FAST!

Answers
Problem 3
Answer: x = 47Explanation: The angles opposite the congruent sides, are congruent angles. We consider these the base angles. This triangle is isosceles.
==================================================
Problem 4
Answer: x = 32Explanation:
Refer to the diagram below. Note how I've added in the variable y and marked another angle as 53 degrees which is one of the base angles of the left triangle. The base angles of the triangle on the right are y degrees each. Also, note the vertical angles both are y.
For the left triangle, the three angles add up to 180 which means we can solve for y to go from 53+53+y = 180 to y = 74
Then the interior angles of the triangle on the right has the three angles (y,y,x) add to 180 as well. So we have y+y+x = 180 which turns into 74+74+x = 180. That solves to x = 32
==================================================
Problem 5
Answer: x = 58Explanation:
Refer to the diagram below. I've added another base angle x, and the angle 90-x is complementary to this. This is because the two pieces must add to 90.
For the triangle on the bottom, the two base angles 74 and 74, add to the third angle 90-x and we would get 180. So we solve 74+74+(90-x) = 180 to get x = 58

Answer:
3. x = 47
4. x = 32
5. x = 58
Step-by-step explanation:
4)2 ?
Which of the following expressions is equivalent to (x
Answers
Answer:
2 is the equivalent to the expression
A soup can is in the shape of a cylinder with a radius of 1 inch and a height of 3 inches.
How much paper is used for the label of the soup can, which covers the lateral surface area of the soup can? (Round your answer to the nearest hundredth) _ in2

Answers
use composition of functions to determine whether f(x) and g(x) are inverse of each other. show all work for full credit.
f(x)=4/5 x=1
g(x)=5x-5/4
Answers
After computing the composition of f(g(x)) and g(f(x)), it is clear that f(x) and g(x) are not inverse functions of each other.
To determine whether f(x) and g(x) are inverse functions of each other, we need to check whether the composition of the two functions f(g(x)) and g(f(x)) result in the identity function, which is equal to x.
First, let's find f(g(x)):
f(g(x)) = f(5x - 5/4) (substituting g(x) into f(x))
f(g(x)) = 4/5(5x - 5/4) + 1 (substituting the expression for f(x))
f(g(x)) = 4x - 1 + 1
f(g(x)) = 4x
Now let's find g(f(x)):
g(f(x)) = g(4/5x + 1) (substituting f(x) into g(x))
g(f(x)) = 5(4/5x + 1) - 5/4 (substituting the expression for g(x))
g(f(x)) = 4x + 5 - 5/4
g(f(x)) = 4x + 20/4 - 5/4
g(f(x)) = 4x + 15/4
Since f(g(x)) = 4x and g(f(x)) = 4x + 15/4, we can see that the two compositions are not equal to x, which means that f(x) and g(x) are not inverse functions of each other.
Therefore, we can conclude that f(x) and g(x) are not inverse functions of each other.
To learn more about composition please click on below link
https://brainly.com/question/17872426
#SPJ1
WILL MARK BRAINLIEST:
What is the equation of a line that passes through the point (6, -2) and has zero slope.
x = 6x = 6
y = −2y = −2
y = 6y = 6
x = −2
Answers
Answer:
x=6
Step-by-step explanation:
x = 6x = 6
x = 6x = 6
thanks
Please mark it as brainliast
PLS HELP WILL GIVE BRAINLEST
Which figure can be formed from the net?
An unfolded square base pyramid with the sides of the base being eight millimeters and the length from the base to the tip is ten millimeters.
A) A pyramid with a triangle base has two sides measurements of ten millimeters. The length from the base to the tip of a triangle is eight millimeters.
B)A pyramid with a triangle base has two sides measurements of eight millimeters. The length from the base to the tip of a triangle is ten millimeters.
C)A pyramid with a square base has two sides measurements of ten millimeters. The length from the base to the tip of a triangle is eight millimeters.
D)A pyramid with a square base has two sides measurements of eight millimeters. The length from the base to the tip of a triangle is ten millimeters.
Answers
Answer:
D
Step-by-step explanation:
2. The name of the 1500-meter gold medal winner for each of the Olympics
from 1896 to 2000 is placed in a jar. (If a man has won the race more than
once, his name will be included for each race he won.)
A. If one name is drawn from the jar, which country has the best chance
of having a name drawn?
B. Three names are drawn without replacing the name after it is drawn.
What is the probability that the three winners will be from either
New Zealand or Australia?
pLEASE HELP
Answers
The probability shows that when one name is drawn from the jar, the country that has the best chance of having a name drawn is Great Britain.
How to calculate probability?It should be noted that Great Britain has won 6 golds in the 1500 meters Olympics and the next placed country is Kenya. In this case, the country that has the best chance of having a name drawn is Great Britain.
Note that New Zealand has 3 gold medals while Australia has 2 gold medals. Also, the Olympics has taken place 29 times.
The probability that the winners will be from either New Zealand or Australia will be:
= 3/29 + 2/29
= 5/29
Learn more about probability on:
brainly.com/question/24756209
#SPJ1
Answer
A. Great Britain
B. 3/29+2/29=5/29
please give me the brainliest.
True or False? The blue radius is perpendicular to the green chord.
Answers
The statement that the blue radius is perpendicular to the green cord is false
From the complete question,
The blue radius does not bisect the green chord
This means that the blue radius and the green chord do not meet at 90 degrees
Since both lines do not meet at 90 degrees, then they are not perpendicular
Hence, the statement that the blue radius is perpendicular to the green cord is false
Read more about radius and chords at:
https://brainly.com/question/1869643
Use Green's Theorem to evaluate oint_c xy^2 dx + x^5 dy', where 'C' is the rectangle with vertices (0,0), (3,0), (3,5), and (0,5)
Find and classify the critical points of z=(x^2 - 4 x)(y^2 - 5 y) Lo
Answers
To evaluate the line integral using Green's Theorem, we need to find the curl of the vector field and then calculate the double integral over the region enclosed by the curve. Answer : the critical points of the function z = (x^2 - 4x)(y^2 - 5y) are (x, y) = (0, 0) and (x, y) = (0, 4)
Given the vector field F = (xy^2, x^5), we can find its curl as follows:
∇ × F = (∂Q/∂x - ∂P/∂y)
where P is the x-component of F (xy^2) and Q is the y-component of F (x^5).
∂Q/∂x = ∂/∂x (x^5) = 5x^4
∂P/∂y = ∂/∂y (xy^2) = 2xy
Therefore, the curl of F is:
∇ × F = (2xy - 5x^4)
Now, we can apply Green's Theorem:
∮C P dx + Q dy = ∬D (∇ × F) dA
where D is the region enclosed by the curve C.
In this case, C is the rectangle with vertices (0,0), (3,0), (3,5), and (0,5), and D is the region enclosed by this rectangle.
The line integral becomes:
∮C xy^2 dx + x^5 dy = ∬D (2xy - 5x^4) dA
To evaluate the double integral, we integrate with respect to x first and then with respect to y:
∬D (2xy - 5x^4) dA = ∫[0,5] ∫[0,3] (2xy - 5x^4) dx dy
Now, we can calculate the integral using these limits of integration and the given expression.
As for the second part of your question, to find the critical points of the function z = (x^2 - 4x)(y^2 - 5y), we need to find the points where the partial derivatives with respect to x and y are both zero.
Let's calculate these partial derivatives:
∂z/∂x = 2x(y^2 - 5y) - 4(y^2 - 5y)
= 2xy^2 - 10xy - 4y^2 + 20y
∂z/∂y = (x^2 - 4x)(2y - 5) - 5(x^2 - 4x)
= 2xy^2 - 10xy - 4y^2 + 20y
Setting both partial derivatives equal to zero:
2xy^2 - 10xy - 4y^2 + 20y = 0
Simplifying:
2y(xy - 5x - 2y + 10) = 0
This equation gives us two cases:
1) 2y = 0, which implies y = 0.
2) xy - 5x - 2y + 10 = 0
From the second equation, we can solve for x in terms of y:
x = (2y - 10)/(y - 1)
Now, substitute this expression for x back into the first equation:
2y(2y - 10)/(y - 1) - 10(2y - 10)/(y - 1) - 4y^2 + 20y = 0
Simplifying and combining like terms:
4y^3 - 32y^2 + 64y = 0
Factoring out 4y:
4y(y^2 - 8y +
16) = 0
Simplifying:
4y(y - 4)^2 = 0
This equation gives us two cases:
1) 4y = 0, which implies y = 0.
2) (y - 4)^2 = 0, which implies y = 4.
So, the critical points of the function z = (x^2 - 4x)(y^2 - 5y) are (x, y) = (0, 0) and (x, y) = (0, 4).
To classify these critical points, we can use the second partial derivative test or examine the behavior of the function in the vicinity of these points.
Learn more about Green's Theorem : brainly.com/question/27549150
#SPJ11
At noon on March 21st, what is the local sidereal
time?
a.
00:00
b.
06:00
c.
12:00
d.
18:00
e.
21:00
Answers
According to the question The local sidereal time (LST) at noon on March 21st is approximately 12:00 (option c).
To determine the LST, we need to consider the rotation of the Earth and its relation to the stars. LST is based on the apparent motion of the vernal equinox, which completes a full cycle in approximately 24 hours.
Since noon is halfway through the day, we can estimate that the LST at noon would be approximately half of 24 hours, which is 12:00. This assumes that we are considering a location where the local time is synchronized with the standard time zone and there are no factors like daylight saving time or variations in the location's longitude that can affect the LST calculation.
Therefore, the correct answer is option c) 12:00 local sidereal time.
To know more about motion visit -
brainly.com/question/32756130
#SPJ11
The triangles shown below must be congruent.
151-
80⁰
10
O A. True
OB. False
51
80⁰
10
Answers
Answer:
false
Step-by-step explanation:
The sum of three consecutive integers is 105. Find the smallest integer.
Answers
Answer:
34.
Step-by-step explanation:
The three integers would be 34,35,36 because the sum of the three numbers is 105.
tests for tuberculosis. Suppose that for the population of adults that is taking the test; 5% have tuberculosis The test correctly identifies 74.6% of the tlme adults with tuberculosis and correctly identifies those without tuberculosis 76.53% of the time: Suppose that POS stands for the test gives positive result and S means that the adult really has tuberculosis What is the probability of an adult getting NEG result and truly having tuberculosis? A.0.0373 B.0.0127 C.0.2230 D.0,7270
Answers
The probability of an adult getting a NEG result and truly having tuberculosis is 0.01725, which is closest to option (A) 0.0373.
Let's use the following notation:
P(TB) represents the probability that an adult has tuberculosis, which is given as 0.05.
P(POS|TB) represents the probability that the test is positive given that an adult has tuberculosis, which is given as 0.746.
P(NEG|TB) represents the probability that the test is negative given that an adult has tuberculosis, which is 1 - P(POS|TB) = 0.254.
We are asked to find the probability of an adult getting a NEG result and truly having tuberculosis, which can be calculated using Bayes' theorem as follows:
P(TB|NEG) = P(NEG|TB) * P(TB) / P(NEG)
We can calculate P(NEG) using the law of total probability, which considers the two possible cases for an adult: having tuberculosis (TB) or not having tuberculosis (TB').
P(NEG) = P(NEG|TB) * P(TB) + P(NEG|TB') * P(TB')
= 0.254 * 0.05 + 0.7653 * 0.95
= 0.7352
Now we can substitute the values into Bayes' theorem:
P(TB|NEG) = 0.254 * 0.05 / 0.7352
= 0.01725
Therefore, the probability of an adult getting a NEG result and truly having tuberculosis is 0.01725, which is closest to option (A) 0.0373.
Learn more about Bayes' theorem here: brainly.com/question/29598596
#SPJ4
PLZZ HELP FOR BRAINLIEST
What is the y-intercept of the graph?
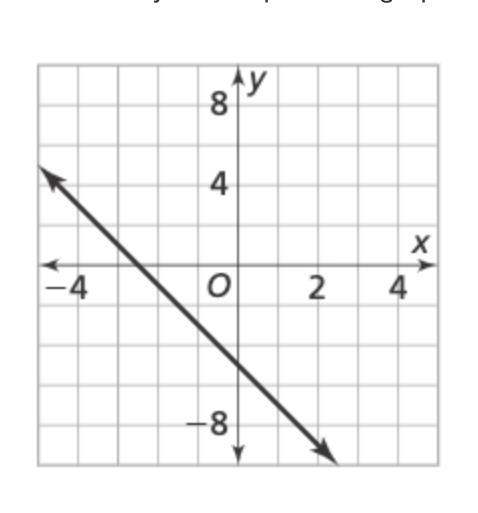
Answers
Answer:
-5
Step-by-step explanation:
The school cafeteria sells two kinds of wraps: vegetarian and chicken. The vegetarian wrap costs $1.00 and the chicken wrap costs $2.70. Today they made $119.60 from the 72 wraps sold. How many of the wraps sold were vegetarian
Answers
Answer:
vegetarians= 28
chickens= 44
Step-by-step explanation:
let the number of vegetarians be x
and chickens be y
So
x+2.7y=119.6----------1
x+y=72--------------2
from 2, x= 72-y
put x= 72-y in 1
72-y+2.7y=119.6
-y+2.7y=119.6-72
1.7y=47.6
divide both sides by 1.7
y=47.6/1.7
y=28
put y=28 in 1
x+28=72
y= 72-28
y=44
In softball, a batting avearage is the number of hits divided by the number of times at bat. Does player 1 have the greater batting avearage? Player 1, 42 hits, at bats 90. Player 2, 38 hits, at bats 80
Answers
Player 1 has a greater batting average than Player 2 since their batting average is calculated as 42/90, which is greater than 38/80.
batting average, we need to calculate the batting averages for both Player 1 and Player 2 based on the given information.
Batting average is calculated by dividing the number of hits by the number of times at bat.
For Player 1, we have 42 hits and 90 at-bats. So, the batting average for Player 1 can be calculated as:
Batting Average = Number of Hits / Number of At-Bats
= 42 / 90
= 0.4667
For Player 2, we have 38 hits and 80 at-bats.
Thus, the batting average for Player 2 is:
Batting Average = Number of Hits / Number of At-Bats
= 38 / 80
= 0.475
Comparing the two batting averages, we can see that Player 2 has a higher batting average of 0.475, whereas Player 1 has a batting average of 0.4667.
Therefore, Player 2 has the greater batting average between the two players.
It's worth noting that batting average is typically represented as a decimal rounded to three decimal places.
In this case, Player 2 has a higher batting average of 0.475, indicating a greater success rate in getting hits relative to at-bats compared to Player 1's batting average of 0.4667.
For similar question on average.
https://brainly.com/question/130657
#SPJ8
Perform the calculation and report your results to the correct number of significant figures. (10.52)(0.6721)
(19.09−15.347)
Answers
The results of the calculations are approximately 7.07 and 3.74, respectively, to the correct number of significant figures.
Performing the calculation:
(10.52)(0.6721) = 7.0671992
Rounding to the correct number of significant figures, we have:
(10.52)(0.6721) ≈ 7.07
Next, let's calculate (19.09 - 15.347):
(19.09 - 15.347) = 3.743
Rounding to the correct number of significant figures, we have:
(19.09 - 15.347) ≈ 3.74
Therefore, the results of the calculations are approximately 7.07 and 3.74, respectively, to the correct number of significant figures.
Learn more about significant figures here
https://brainly.com/question/30169
#SPJ11
Write a cosine function that has an amplitude of 2, a midline of 5 and a period of T.
Answers
phase shift is 0
General equation
y=AcosB(x-C)+Dc is phase shift
y=AcosBx+DMidline is D
y=AcosBx+5A is amplitude
y=2cosBx+5B is period
2π/TSo
Final equation
y=2cos(2πx/T)+5Not mandatory from now
For some special cases 2π/T=omega
So Equation yields
y=2cos(\(\omega x\))+5Answer:
\(f(x)=2 \cos (2x)+5\)
Step-by-step explanation:
The cosine function is periodic, meaning it repeats forever.
Standard form of a cosine function:
f(x) = A cos(B(x + C)) + D
A = amplitude (height from the mid-line to the peak)2π/B = period (horizontal distance between consecutive peaks)C = phase shift (horizontal shift - positive is to the left)D = vertical shiftGiven:
Amplitude = 2 ⇒ A = 2\(\sf Period=\pi \implies \dfrac{2 \pi}{B}=\pi \implies B=2\)mid-line = 5 ⇒ D = 5Inputting the given values into the standard form:
\(\implies f(x)=2 \cos (2x)+5\)

what are the steps to induction nsls
Answers
These steps are often referred to as the principle of mathematical induction or PMI.
The steps for mathematical induction are:
Base Case: Show that the statement holds for some particular value of n, usually n = 1 or n = 0.
Inductive Hypothesis: Assume that the statement holds for some arbitrary value of n = k, where k is a positive integer.
Inductive Step: Using the inductive hypothesis, show that the statement also holds for n = k + 1.
Conclusion: By the principle of mathematical induction, the statement is true for all positive integers n.
These steps are often referred to as the principle of mathematical induction or PMI. They are used to prove statements that involve an infinite set of integers by showing that the statement holds for a base case, assuming that it holds for an arbitrary value, and then showing that it holds for the next integer in the set.
Learn more about mathematical induction here
https://brainly.com/question/29503103
#SPJ11