If gdp is $20 trillion how many years will it take for a gdp to increase to 80$ trillion if growth is 7 percent
Answers
Answer:
19. 8 years
Step-by-step explanation:
Using the exponential growth function :
A = Pe^rt
A = final amount or growth unit
P = initial or present growth unit
Rate, r = growth rate
80 = 20e^0.07t
80/20 = e^0.07t
4 = e^0.07t
Take In of both sides
In(4) = 0.07t
1.3862943 = 0.07t
t = 19.804205
t = 19. 8 years
Related Questions
Please help quick I have other question if you don't know this one

Answers
3.12 If h(t)= [u(t-1)- u(t - 4)] and x(t) = t[u(t)- u(t-2)], obtain graphically the response y(t). For what value of t does y(t) reach its maximum value?
Answers
The response y(t) graphically, we can first plot the individual functions h(t) and x(t) on a graph, and then determine their convolution to obtain y(t). Let's go step by step:
Plotting h(t):
The function h(t) is defined as h(t) = [u(t-1) - u(t-4)].
The unit step function u(t-a) is 0 for t < a and 1 for t ≥ a. Based on this, we can plot h(t) as follows:
For t < 1, h(t) = [0 - 0] = 0
For 1 ≤ t < 4, h(t) = [1 - 0] = 1
For t ≥ 4, h(t) = [1 - 1] = 0
So, h(t) is 0 for t < 1 and t ≥ 4, and it jumps up to 1 between t = 1 and t = 4. Plotting h(t) on a graph will show a step function with a jump from 0 to 1 at t = 1.
Plotting x(t):
The function x(t) is defined as x(t) = t[u(t) - u(t-2)].
For t < 0, both u(t) and u(t-2) are 0, so x(t) = t(0 - 0) = 0.
For 0 ≤ t < 2, u(t) = 1 and u(t-2) = 0, so x(t) = t(1 - 0) = t.
For t ≥ 2, both u(t) and u(t-2) are 1, so x(t) = t(1 - 1) = 0.
So, x(t) is 0 for t < 0 and t ≥ 2, and it increases linearly from 0 to t for 0 ≤ t < 2. Plotting x(t) on a graph will show a line segment starting from the origin and increasing linearly with a slope of 1 until t = 2, after which it remains at 0.
Obtaining y(t):
To obtain y(t), we need to convolve h(t) and x(t). Convolution is an operation that involves integrating the product of two functions over their overlapping ranges.
In this case, the convolution integral can be simplified because h(t) is only non-zero between t = 1 and t = 4, and x(t) is only non-zero between t = 0 and t = 2.
The convolution y(t) = h(t) * x(t) can be written as:
y(t) = ∫[1,4] h(τ) x(t - τ) dτ
For t < 1 or t > 4, y(t) will be 0 because there is no overlap between h(t) and x(t).
For 1 ≤ t < 2, the convolution integral simplifies to:
y(t) = ∫[1,t+1] 1(0) dτ = 0
For 2 ≤ t < 4, the convolution integral simplifies to:
y(t) = ∫[t-2,2] 1(t - τ) dτ = ∫[t-2,2] (t - τ) dτ
Evaluating this integral, we get:
\(y(t) = 2t - t^2 - (t - 2)^2 / 2,\) for 2 ≤ t < 4
For t ≥ 4, y(t) will be 0 again.
Maximum value of y(t):
To find the value of t at which y(t) reaches its maximum value, we need to examine the expression for y(t) within the valid range 2 ≤ t < 4. We can graphically determine the maximum by plotting y(t) within this range and identifying the peak.
Plotting y(t) within the range 2 ≤ t < 4 will give you a curve that reaches a maximum at a certain value of t. By visually inspecting the graph, you can determine the specific value of t at which y(t) reaches its maximum.
Learn more about function here:
https://brainly.com/question/11624077
#SPJ11
Find an angle with a positive measure and an angle with a negative measure that are coterminal with each angle. 175
Answers
Answer:
185 anle with the postive measure and a negative measure
Please answer this really fast.
The linear factors (2x+3) and (3x-1) can be rewritten as a standard form trinomial in the form ax^2+bx+c. Find the product of a,b and c. Your answer is just 1 number.
Answers
Answer:
abc = - 126
Step-by-step explanation:
(2x + 3)(3x - 1) ← expand product using FOIL
= 6x² + 7x - 3 ← in standard form
with a = 6, b = 7, c = - 3
Then
abc = 6 × 7 × (- 3) = - 126
Answer:2x(3-1) +3(3x-1)
6x^2 -2x+9x-3
6x^2+7x-3
a=6 b=7 c=3
product (x)=6x7x-3=-126
16. Which graph represents the solution to the following system of inequalities?

Answers
Answer:
D
Step-by-step explanation:
what is 1696 divided by 43
Answers
Answer:
39.44
Step-by-step explanation:
Answer:
39.4418605
Step-by-step explanation:
2. When graphed, which equation pair will be parallel lines?
Oy=42-4 and y = -x +9
Oy=1-1 and y = x + 1
Oy=x and y = 2x + 2
Oy=-x-8 and y = x + 5
Answers
One of the options is c=d because matching angles are made and the same corners are congruent when a line joins two parallel lines.
How do parallel lines work?In geometry, parallel lines are coplanar, straight lines that don't intersect anywhere. Parallel planes are those in the same three-dimensional space that never cross one another. Curves do not touch or intersect when they are parallel to one another and keep a predetermined minimum distance between them. Lines in a plane are considered to be parallel if their spacing is constant. Objects cannot cross parallel lines. Perpendicular lines are those that intersect at a precise angle of 90 degrees.
Due to vertical angles, A = d is also a possible answer. The outcome is that b+d=180 since b+c=180 and c=d, and b+d=180 as a result. Therefore, the answer is B, C, E, where a=d, c=d, and b+d=180.
To know more about parallel lines visit:
brainly.com/question/16701300
#SPJ1
help with my geometry please

Answers
Answer:
x = 11
z = 86
Step-by-step explanation:
8x + 6 and 10x-16 are vertical angles
Vertical angles are pairs of angles that are opposite each other and have the same vertex, or point of intersection. They are formed when two lines intersect at a point, and are always congruent, or of equal measure.
To solve this equation, we need to isolate the variable x on one side of the equation. To do this, we can start by subtracting 6 from both sides of the equation:
8x + 6 - 6 = 10x - 16 - 6
8x = 10x - 22
Now we can subtract 8x from both sides of the equation:
8x - 8x = 10x - 22 - 8x
0 = 2x - 22
To solve for x, we can add 22 to both sides of the equation:
0 + 22 = 2x - 22 + 22
22 = 2x
Finally, we can divide both sides of the equation by 2 to find the value of x: 22 / 2 = 2x / 2
x = 11
Therefore, the solution to the equation is x = 11.
Now that we have x, z is a supplementary angle to 8x + 6 (or you could do 10x - 16)
Supplementary angles are pairs of angles that add up to 180 degrees. They are formed when two lines intersect at a point, and the angles formed at the intersection are supplementary.
First plug in x, 8x + 6 = 8(11) + 6 = 88 + 6 = 94
180 - 94 = z
z = 86
Increase £200 by 12.5%
Answers
Answer:
£225
Step-by-step explanation:
12.5% × £200
>> £25
Since we want to increase £200 by 12.5%, then we will add the increment to the £200 to get;
£200 + £25 = £225
Answer: £25
Step-by-step explanation: Take £200 and multiply by 12.5% and you get £25. I hope this helped!
Which of the following equations is equivalent to 2x+7 = 27

Answers
Answer: C
Step-by-step explanation: The answer is C because x is equivalent to 10 in both problems. X is equal to 10 in the problem 2x + 7 =27 because 2 x 10 = 20 + 7 = 27. X is also equal to 10 in the problem 5x - 15 = 35 because 5 x 10 = 50 - 15 = 35. This means the answer is C.
18 is 1.2 percent of what number
Answers
Answer:
1500
Step-by-step explanation:
18 = 1.2%
100% ÷ 1.2% = 83 and 1/3
1.2% × 83 and 1/3 = 100%
18 × 83 and 1/3 = 1500
Put the following equation of a line into slope-intercept form. 12x-4y=12 Must be FULLY SIMPLIFIED.
Answers
The slope intercept form has the next form
\(y=mx+b\)We have
\(12x-4y=12\)we need to isolate the y
\(-4y=-12x+12\)\(y=\frac{-12}{-4}x+\frac{12}{4}\)\(y=3x-3\)ANSWER
y=3x-3
A company makes 140 bags.
30 of the bags have buttons but no zips.
27 of the bags have zips but no buttons.
20 of the bags have neither zips nor buttons.
A bag is selected at random.
What is the probability that the bag has buttons?
Answers
Answer: 41/75 or 54.67%
Step-by-step explanation:
Buttons --- yes, zips --- yes = 45 (compute as 150 - 37 - 45 - 23)
Buttons --- yes, zips --- no = 37
Buttons --- no, zips --- yes = 45
Buttons --- no, zips --- no = 23
Therefore, 82 out of 150 bags have buttons so probability = 82/150 = 41/75
the questionnaire is a carefully constructed measurement instrument. group of answer choices true false
Answers
Yes, its true that the questionnaire is very carefully constructed by an individual to provide the measurement instrument.
A questionnaire is a studies device which includes a sequence of questions for the motive of amassing facts from respondents. Questionnaires may be concept of as a form of written interview. A questionnaire is a listing of questions or gadgets used to collect facts from respondents approximately their attitudes, experiences, or opinions.
Questionnaires may be used to acquire quantitative and/or qualitative facts. Questionnaires are generally utilized in marketplace studies in addition to withinside the social and fitness sciences. Questionnaires are typically taken into consideration to be excessive in reliability. This is due to the fact it's miles feasible to invite a uniform set of questions. Any troubles withinside the layout of the survey may be ironed out after a pilot study. The greater closed questions used, the greater dependable the studies.
To learn more about questionnaire, refer: brainly.com/question/25685309
#SPJ4
Which interval notation represents the set of all number greater than or equal to 1
and less than 6?
Answers
Answer:
The interval notation for the set of all numbers greater than or equal to 1 and less than 6 is [1, 6).
Step-by-step explanation:
please help no links and don't cheat, please



Answers
Answer:
√441=21
√361=19
√401=20.02 or 20
One unit of A is composed of two units of B and three units of C. Each B is composed of one unit of F. C is made of one unit of D, one unit of E, and two units of F. Items A,B,C, and D have 20,50,60, and 25 units of on-hand inventory, respectively. Items A,B, and C use lot-for-lot (L4L) as their lot-sizing technique, while D,E, and F require multiples of 50,100 , and 100 , respectively, to be purchased. B has scheduled receipts of 30 units in period 1. No other scheduled receipts exist. Lead times are one period for items A, B, and D, and two periods for items C,E, and F. Gross requirements for A are 20 units in period 1,20 units in period 2, 60 units in period 6, and 50 units in period 8. Find the planned order releases for all items.
Answers
The planned order releases for each item are as follows: A: 20 units in period 1, B: 10 units in period 1, C: 40 units in period 3, D: No planned order release, E: 100 units in period 5, F: 100 units in period 5
To determine the planned order releases for all items, we need to calculate the net requirements for each period based on the given information. We will start with the highest-level item and work our way down the bill of materials.
Item A:
Period 1: Gross requirement of 20 units.
Since A uses lot-for-lot (L4L) as the lot-sizing technique, we release an order for 20 units of A.
Item B:
Item B is a component of A, and each A requires 2 units of B.
We need to calculate the net requirements for B based on the planned order release for A.
Period 1: Gross requirement of 20 units * 2 (requirement multiplier for B) = 40 units.
B has a scheduled receipt of 30 units in period 1.
Net requirement for B in period 1: 40 units - 30 units = 10 units.
Since B also uses L4L as the lot-sizing technique, we release an order for 10 units of B.
Item C:
Item C is a component of A, and each A requires 3 units of C.
We need to calculate the net requirements for C based on the planned order release for A.
Period 1: Gross requirement of 20 units * 3 (requirement multiplier for C) = 60 units.
C has a lead time of two periods, so we need to account for that.
Net requirement for C in period 3: 60 units - 20 units (scheduled receipt for A in period 1) = 40 units.
Since C uses L4L as the lot-sizing technique, we release an order for 40 units of C.
Item D:
Item D is a component of C, and each C requires 1 unit of D.
We need to calculate the net requirements for D based on the planned order release for C.
Period 3: Gross requirement of 40 units * 1 (requirement multiplier for D) = 40 units.
D has a lead time of one period, so we need to account for that.
Net requirement for D in period 4: 40 units - 60 units (scheduled receipt for C in period 3) = -20 units (no requirement).
Since the net requirement is negative, we do not release any planned order for D.
Item E:
Item E is a component of C, and each C requires 1 unit of E.
We need to calculate the net requirements for E based on the planned order release for C.
Period 3: Gross requirement of 40 units * 1 (requirement multiplier for E) = 40 units.
E has a lead time of two periods, so we need to account for that.
Net requirement for E in period 5: 40 units - 0 units (no scheduled receipt for E) = 40 units.
Since E requires a multiple of 100 to be purchased, we release an order for 100 units of E.
Item F:
Item F is a component of B and C, and each B requires 1 unit of F, while each C requires 2 units of F.
We need to calculate the net requirements for F based on the planned order releases for B and C.
Period 1: Gross requirement for B = 10 units * 1 (requirement multiplier for F) = 10 units.
Period 3: Gross requirement for C = 40 units * 2 (requirement multiplier for F) = 80 units.
F has a lead time of two periods, so we need to account for that.
Net requirement for F in period 5: 10 units + 80 units - 0 units (no scheduled receipt for F) = 90 units.
Since F requires a multiple of 100 to be purchased, we release an order for 100 units of F.
Learn more about calculate here:
https://brainly.com/question/30151794
#SPJ11
Write the number shown in standard notation.
5.29 x 10
Answers
Answer:
\(5.29 \times {10}^{1} \)
Use properties of logarithms to expand log(r^2 s^11)/t^14
Answers
Expanding log(r^2 s^11)/t^14 = 2 loga(r) + 11 loga(s) - 14 loga(t)
To expand log(r^2 s^11)/t^14 using properties of logarithms, follow these steps:
Step 1: Apply the quotient rule. The quotient rule states that log(a/b) = log(a) - log(b). So we have: log(r^2 s^11) - log(t^14)
Step 2: Apply the product rule. The product rule states that log(ab) = log(a) + log(b). So we have: (log(r^2) + log(s^11)) - log(t^14)
Step 3: Apply the power rule. The power rule states that log(a^n) = n*log(a). So we have: (2*log(r) + 11*log(s)) - 14*log(t)
Thus, the expanded form of log(r^2 s^11)/t^14 is: 2*log(r) + 11*log(s) - 14*log(t)
Learn more about logarithms here:
https://brainly.com/question/30085872#
#SPJ11
The table shows the relationship between the number of calories Darrell Burns while kayaking and the number of minutes he kayaks
How many calories will Darrell burn in 1 minute while kayaking? Please I need help :(
Answers
The number of calories that Darrell will burn in 1 minute while kayaking is given as follows:
4 calories.
How to obtain the number of calories?The number of calories that Darrell will burn in 1 minute while kayaking is obtained applying the proportions in the context of the problem.
For each input-output pair in the table, the constant of proportionality is of 4, hence the number of calories that Darrell will burn in 1 minute while kayaking is given as follows:
4 calories.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1

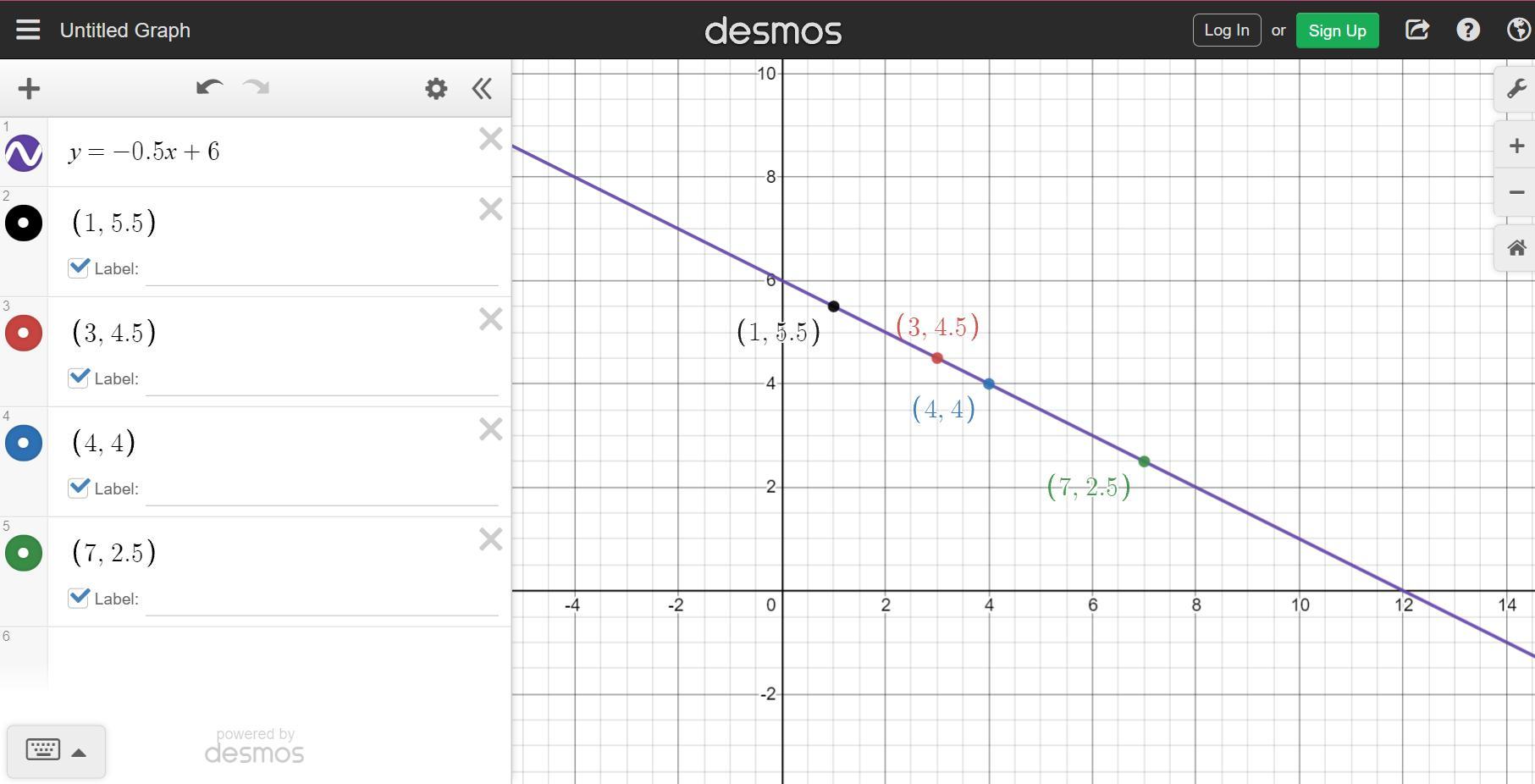
The line y=−0.5x+b passes through the points (1,5.5), (3,p), (4,4), and (7,n). Find b, n, and p.
Answers
Step-by-step explanation:
Finding b
If we use the given slope and use one of the points to write the equation in point-slope form (y - y₁) = m(x - x₁)), we can rearrange it to find the y-intercept:
Using (4,4)
⇒ y - 4 = - 0.5 (x - 4)
y - 4 = - 0.5 x + 2
y = -0.5 x + 6
∴ b = 6Calculating 'n' and 'p'
We can find n and p by plugging the points into the newly found equation y = -0.5 x + 6.
For n using (7, n)
since y = -0.5 x + 6
n = -0.5 (7) + 6
∴ n = 2.5For p using (3,p)
since y = -0.5 x + 6
p = - 0.5 (3) + 6
p = - 1.5 + 6
p = 4.5Checking the answer
We can check the answer we got in two ways:
We could also have found n a different way:Using the points (4, 4) and (7, n) as (x₁, y₁) & (x₂, y₂) respectively
The slope of the line (m) = (y₂ - y₁) ÷ (x₂ - x₁)
- 0.5 = (n - 4) ÷ (7 - 4)
- 0.5 = ⁿ⁻⁴/₃ [multiply both sides by 3]
- 1.5 = n - 4 [add four to both sides]
n = 2.5 [this matches the value of n we found]
We can plot the line and see if it goes through the points we found. As seen in the graph below, the line passes through all the points.
How many three-digit positive integers \(x\) satisfy \(3874x+481\equiv 1205 \pmod{23}\)
Answers
Answer:
40
Step-by-step explanation:
Any solution x will mod 23 will also have x+23n as a solution, for some integer n. Since 900/23 = 39 3/23, we know there are 39 or 40 three-digit integers of this form.
As it happens, 100 is the smallest 3-digit solution. So, there are 40 three-digit numbers that are of the form 100 +23n, hence 40 solutions to the equation.
_____
The equation reduces, mod 23, to ...
10x = 11
Its solutions are x = 23n +8.
What value of x makes this equation true?
15 –
1
2
x = 21
Answers
Answer:
x= -0.5
Step-by-step explanation:
You want to isolate x on one side of the equation
15-12x=21
-15 -15
-12x=6
/-12 /-12
x= -0.5
Find the area of the parallelogram with vertices k(2, 2, 1), l(2, 3, 3), m(6, 9, 3), and n(6, 8, 1).
Answers
Let \(\vec K,\vec L,\vec M,\vec N\) be vectors pointing the vertices K, L, M, and N, respectively.
The side KL is parallel to and has the same length as the vector
\(\vec L - \vec K = (2\,\vec\imath + 3\,\vec\jmath + 3\,\vec k) - (2\,\vec\imath+2\,\vec\jmath+\vec k) = \vec\jmath + 2\,\vec k\)
Similarly, the side KN is parallel and as long as
\(\vec N - \vec K = (6\,\vec\imath+8\,\vec\jmath+\vec k) - (2\,\vec\imath+2\,\vec\jmath+\vec k) = 4\,\vec\imath+6\,\vec\jmath\)
These vectors have magnitudes
\(\|\vec L - \vec K\| = \sqrt{0^2 + 1^2 + 2^2} = \sqrt5\)
\(\|\vec N - \vec K\| = \sqrt{4^2 + 6^2 + 0^2} = 2\sqrt{13}\)
and their dot product is
\((\vec L - \vec K) \cdot (\vec N - \vec K) = 0\cdot4 + 1\cdot6+1\cdot0 = 6\)
The parallelogram spanned by the vectors \(\vec L-\vec K\) and \(\vec N-\vec K\) has area equal to the magnitude of their cross product, for which we have the identity
\(\bigg\|(\vec L - \vec K) \times (\vec N - \vec K)\bigg\| = \|\vec L - \vec K\| \|\vec N - \vec K\| \sin(\theta) \\\\ \implies \text{area} = 2\sqrt{65} \, \sin(\theta)\)
where \(\theta\) is the angle between the sides KL and KN.
From the dot product identity, we have
\((\vec L - \vec K) \cdot (\vec N - \vec K) = \|\vec L - \vec K\| \|\vec N - \vec K\| \cos(\theta) \\\\ \implies 6 = 2\sqrt{65} \cos(\theta)\)
Then
\(\cos(\theta) = \dfrac3{\sqrt{65}} \implies \sin(\theta) = \sqrt{1-\cos^2(\theta)} = 2\sqrt{\dfrac{14}{65}} \\\\ \implies \text{area} = 2\sqrt{65} \cdot 2\sqrt{\dfrac{14}{65}} = \boxed{4\sqrt{14}}\)
what is 3/4 + 1/3 ? please hurryyyyyyy thanks

Answers
Answer:
13/12
Step-by-step explanation:
Hope this helps <3
Answer:
answer is 1 1/12 is the correct answer
If someone leave a 20% tip and the meal is 35$how much was the tip
Answers
Answer:
$7
Step-by-step explanation:
Since 100/20 = 5, 20% is 1/5, so 20% of 35 is 35/5, or 7
Answer:
If someone leave a 20% tip and the meal is 35$how much was the tip :
$35.00 ÷ 20% = $7.00
Which answer choice correctly represents the quotient of 28,134 divided by 71?
A.
B.
C.
D.
Answers
Answer:
please give brainliest
Step-by-step explanation:
396.253521127
a symbol such as 2/3 or 1/2 used to name part of a whole pat of a set or a location on the number line is called ______
Answers
The factory makes boxes that each hold one candle. The boxes measure 1 inch × 1 inch × 9 inches. The factory owner makes a stack of 40 candle boxes. What is the volume of the stack?
Answers
Answer:
360 inch^3
Step-by-step explanation:
Volume of 1 box = 1 x 1 x 9 = 9 inch^3
Volume of 40 boxes = 40 x 9 = 360 inch^3
This is a topic on mensuration. If you wish to explore more into this topic you can give me a follow on Instagram (learntionary). I'll be uploading notes on this topic soon. At the meantime, you can take a look at other topics or some tips as well!
Answer:
360 in
Step-by-step explanation:
L × W × H
1 × 1 ×9 = 9in
9 × 40 = 360 in
Lyle and Shaun open savings accounts at the same time. Lyle deposits $100 initially and adds $20 per week. Shaun deposits $500 initially and adds $10 per week. Lyle wants to know when he will have the same amount in his savings account as Shaun.
Part A: Write two equations to represent the amounts of money Lyle and Shaun have in their accounts
f(x=
g(x)=
Part B: to solve the system you will make f(x)=g(x)
Answers
A. The equations for the amounts of money Lyle and Shaun have in their accounts are represented as:
f(x) = 20x + 100
g(x) = 10x + 500
B. When f(x) = g(x), x = 40.
How to Write the Equation of a System?A linear equation can be written in slope-intercept form y = mx + b. Here, the value of m is the unit rate or slope, while the value of b is its y-intercept or initial value.
Part A:
Equation for Lyle:
y-intercept / initial value (b) = $100
Slope / unit rate (m) = $20
To write the equation, substitute m = 20 and b = 100 into f(x) = mx + b:
f(x) = 20x + 100.
Equation for Lyle:
y-intercept / initial value (b) = $500
Slope / unit rate (m) = $10
To write the equation, substitute m = 10 and b = 500 into g(x) = mx + b:
g(x) = 10x + 500.
Part B: To solve the system, we would do the following:
20x + 100 = 10x + 500
20x - 10x = -100 + 500
10x = 400
x = 400/10
x = 40
Learn more about system of linear equations on:
https://brainly.com/question/14323743
#SPJ1