Answers
30+[10+(3²-4)]
30+[10+(9-4)]
30+[10+5]
30+15
45
Related Questions
Find the particular antiderivative of the following derivative that satisfies the given condition. C''(x)=4x2-3x ; C(0)=2000
Answers
The particular antiderivative that satisfies the given condition is: C(x) = (4/9)x^4 - (9/8)x^3 + K1x + 2000
To find the particular antiderivative (or integral) of the given derivative \(C''(x) = 4x^2 - 3x\) that satisfies the condition C(0) = 2000, we need to integrate the given function twice.
First, we integrate C''(x) to find C'(x):
\(C'(x) = ∫ (4x^2 - 3x) dx\)
To find the antiderivative of \(4x^2\), we use the power rule for integration: the power of x increases by 1 and is divided by the new power. Similarly, the antiderivative of -3x is \(-(3/2)x^2\).
\(C'(x) = ∫ (4x^2 - 3x) dx = (4/3)x^3 - (3/2)x^2 + K1\)
Here, K1 is the constant of integration. Next, we integrate C'(x) to find C(x):
\(C(x) = ∫ (C'(x)) dx = ∫ ((4/3)x^3 - (3/2)x^2 + K1) dx\)
To find the antiderivative of \((4/3)x^3\), we again use the power rule for integration. Similarly, the antiderivative of \(-(3/2)x^2\) is \(-(3/2)(1/3)x^3\).
The constant of integration K1 will also be integrated with respect to x, resulting in another constant of integration, K2.
\(C(x) = (1/3)(4/3)x^4 - (1/2)(3/2)x^3 + K1x + K2\)
Simplifying further, we have:
\(C(x) = (4/9)x^4 - (9/8)x^3 + K1x + K2\)
Now, we can apply the initial condition C(0) = 2000 to find the particular solution for K2:
\(C(0) = (4/9)(0)^4 - (9/8)(0)^3 + K1(0) + K2 = 2000\)
Since all the terms involving x become zero when x = 0, we have:
K2 = 2000
Therefore, the particular antiderivative that satisfies the given condition is: \(C(x) = (4/9)x^4 - (9/8)x^3 + K1x + 2000\)
To know more about antiderivative refer here:
https://brainly.com/question/33243567
#SPJ11
simplify. enter the answer in the box. PLEASE I NEED IT ASAP
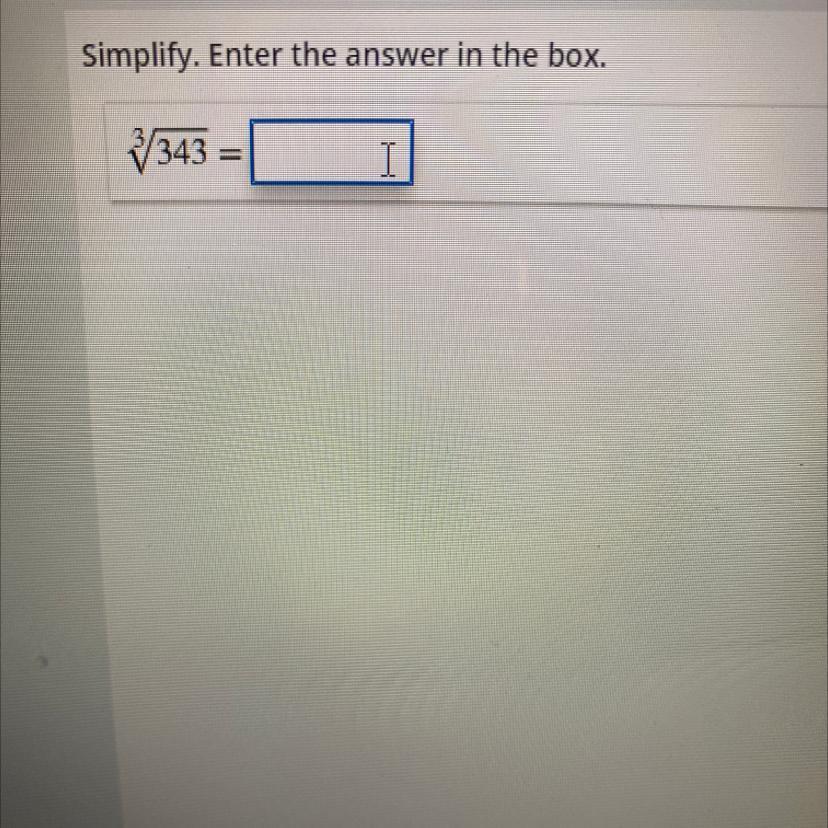
Answers
Answer:
21√7
Step-by-step explanation:
The scale on this drawing is 2 in: 5 ft. Based on this, how
many inches long and wide will the kitchen be?

Answers
The actual length and width of the kitchen in the scale drawing is: 22.5 ft x 17.5 ft
How to Interpret Scale Drawing?A scale drawing is defined as an enlargement of an object. An enlargement changes the size of an object by multiplying each of the lengths by a scale factor to make it larger or smaller. The scale of a drawing is usually stated as a ratio.
Now, the scale factor of the given drawing is seen as 2 in : 5 ft
From the drawing the dimensions of the kitchen are:
9" x 7"
Thus:
True length = (9 * 5)/2 = 22.5 ft
True width = (7 * 5)/2 = 17.5 ft
Read more about Scale Drawing at: https://brainly.com/question/15891755
#SPJ1
If sin x= (sqrt 2)/2 and tan x=-1 what is sec x
Answers
Answer:
\(-\frac{2}{\sqrt{2}}\) or \(-\sqrt{2}\)
Step-by-step explanation:
\(\sec{x}\) is the reciprocal of \(\cos{x}\), or in symbols: \(\sec{x}=\frac{1}{\cos{x}}\). \(\tan{x}\) is also the ratio of \(\sin{x}\) to \(\cos{x}\), and we can multiply both sides of the equation \(\tan{x}=\frac{\sin{x}}{\cos{x}}\) by \(\frac{1}{\sin{x}}\) to get the equation \(\frac{\tan{x}}{\sin{x}}=\frac{1}{\cos{x}}\). Of course, this is just the definition of \(\sec{x}\), so we can rewrite this fact as \(\sec{x}=\frac{\tan{x}}{\sin{x}}\).
In this problem, we're given that \(\sin{x}=\frac{\sqrt{2} }{2}\) and \(\tan{x}=-1\), so plugging those two values into our equation gives us
\(\sec{x}=\dfrac{-1}{\frac{\sqrt{2}}{2}} =-\frac{2}{\sqrt{2}}\)
We could leave our solution as \(-\frac{2}{\sqrt{2}}\), or we could rationalize the denominator to get a solution of
\(-\frac{2}{\sqrt{2}}\cdot\frac{\sqrt{2}}{\sqrt{2}}=-\frac{2\sqrt{2}}{2}=-\sqrt{2}\)
the ratio of length and breadth of a rectangle is 9:7 if its length is it is 18 metere find breadth of the rectangle
perimeter of the rectangle
area of the rectangle
Answers
Perimeter: 64
Area: 252
Explanations:
9•2=18 so, 7•2=14
Length is 18 and Width is now 14
Perimeter explanation:
18+18+14+14= 64
Area Explanation:
18•14= 252
hope this helps:)
1. If the measure of an angle is 38°, find the measure of its complement.
O 38°
O 52°
O 142
62°
Answers
Answer:
52
Complementary Angles:
Two angles that add up to 90.
What is the WACC for Snuggly Baby Corp. if the tax rate is 24.00% and the firm has 6,130,000.00 shares of common equity priced at $16.00 each with an expected return of 20.18% and an expected real return of 14.61%; 1,006,000.00 shares of preferred equity priced at $30.00 each with an expected return of 16.34% and an expected real return of 11.16%; and 81,400.00 bonds that are priced at $925.00 each, and have a current yield of 11.22%, a yield-to-maturity of 12.36%, and a coupon rate of 10.38%
Answers
The Weighted Average Cost of Capital (WACC) for Snuggly Baby Corp. is calculated based on the company's capital structure, including common equity, preferred equity, and bonds. The WACC is influenced by the expected returns and prices of these securities.
To calculate the WACC, we need to determine the proportion of each component in the capital structure and multiply it by its respective cost of capital.
For Snuggly Baby Corp., the capital structure consists of 6,130,000 shares of common equity, 1,006,000 shares of preferred equity, and 81,400 bonds.
The cost of common equity is calculated using the expected return and the price of the shares. Similarly, the cost of preferred equity is calculated using the expected return and the price of the preferred shares. The cost of debt, represented by the bonds, is calculated using the yield-to-maturity.
Once we have the costs of each component, we multiply them by their respective proportions in the capital structure and sum them up to determine the overall WACC. Please note that to provide an accurate calculation of the WACC, additional information is required, such as the market value of each component and the weights assigned to them in the capital structure. Without this information, it is not possible to provide an exact WACC calculation.
learn more about Weighted Average Cost of Capital (WACC) here:
https://brainly.com/question/30746642
#SPJ11
Sorry I didn't know what subject to put this in but Please help I really do need it u don't have to tho
Which fitness test measures the strength and flexibility of the lower back?
A.
Walk/Run test
B.
Curl ups
C.
Sit and Reach
D.
Trunk Lift
Answers
Answer:
Curl ups
Step-by-step explanation:
I'm not 100% sure, but it seems like the most logical answer to me.
Answer:
Trunk Lift
Step-by-step explanation:
we wish to construct a rectangular auditorium with a stage shaped as a semicircle of radius $r$, as shown in the diagram below (white is the stage and green is the seating area). for safety reasons, light strips must be placed on the perimeter of the seating area. if we have $45\pi 60$ meters of light strips, what should $r$ be so that the seating area is maximized?
Answers
To maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.
To maximize the seating area, we need to determine the dimensions of the rectangular auditorium that will give us the largest possible area while using the given length of light strips.
Let the length of the rectangular auditorium be L, and its width be W.
The seating area consists of the rectangular portion minus the semicircular stage. So, the seating area's length is L - 2r (subtracting the semicircle's diameter) and the seating area's width is W - 2r.
The perimeter of the seating area is the sum of the lengths of its four sides, excluding the semicircular stage. The perimeter is given as 45π + 60 meters.
Perimeter = 2(L - 2r) + 2(W - 2r) + πr = 45π + 60
Simplifying: 2L + 2W - 8r + πr = 45π + 60
Rearranging: 2L + 2W = 8r + 44π + 60
The area of the seating area is given by A = (L - 2r)(W - 2r).
We want to maximize A, so we need to express it in terms of a single variable. Since we have an equation with two variables (L and W), we can rewrite one of the variables in terms of the other.
Rearranging the perimeter equation: 2L + 2W = 8r + 44π + 60
Solving for L: L = (8r + 44π + 60 - 2W) / 2
Substituting L in terms of W into the area equation: A = [(8r + 44π + 60 - 2W) / 2 - 2r] (W - 2r)
Simplifying: A = (4r + 22π + 30 - W) (W - 2r)
Now we have the area equation in terms of a single variable, W. To maximize A, we can take the derivative of A with respect to W, set it equal to zero, and solve for W.
dA/dW = 2(4r + 22π + 30 - W) - (W - 2r) = 0
Solving for W: 8r + 44π + 60 - W = W - 2r
Simplifying: 10r + 44π + 60 = 2W
W = 5r + 22π + 30
Now that we have W in terms of r, we can substitute this expression back into the area equation to get the area in terms of r only.
A = (4r + 22π + 30 - (5r + 22π + 30)) ((5r + 22π + 30) - 2r)
Simplifying: A = (r - 22π) (3r + 22π + 30)
Expanding: A = 3r² + 8rπ + 30r - 66πr - 660π
Now, to find the maximum area, we can take the derivative of A with respect to r, set it equal to zero, and solve for r.
dA/dr = 6r + 8π + 30 - 66π = 0
Simplifying: 6r - 58π + 30 = 0
6r = 58π - 30
r = (58π - 30) / 6
r ≈ 29π/3 - 5
Therefore, to maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.
Learn more about maximum area click;
https://brainly.com/question/29635164
#SPJ12
The graphs below have the same shape. What is the equation of the bluegraph?

Answers
Horizontal translation for a parabola is changed by the value of a variable, h, that is subtracted from x before the squaring operation.
Our reference parabola has the following equation:
\(F(x)=x^2\)And the equation that includes a horizontal translation looks like this:
\(G(x)=(x-h)^2\)The sign of h is determined by whether the translated parabola is on the right (positive sign) or on the left (negative sign) side of the reference parabola, in this case, the vertex of the parabola is translated 3 units to the left, then h equals -3, replacing this value for h, we get:
\(\begin{gathered} G(x)=(x-(-3))^2 \\ G(x)=(x+3)^2 \end{gathered}\)Then, the correct answer is option D, G(x) = (x+3)^2
DUE FRIDAY WELL WRITTEN ANSWERS ONLY !!!!!!!HELP
The value of tan(3π/4) is -1. How does the value of tan(3π/4 – π) compare? Explain your reasoning.
Answers
Answer:
The value of tan(3π/4) is indeed -1.
Now, to evaluate tan(3π/4 - π), we can use the following trigonometric identity:
tan(a - b) = (tan(a) - tan(b)) / (1 + tan(a)*tan(b))
Applying this identity to the expression tan(3π/4 - π), we get:
tan(3π/4 - π) = (tan(3π/4) - tan(π)) / (1 + tan(3π/4)*tan(π))
Substituting the values of tan(3π/4) = -1 and tan(π) = 0, we get:
tan(3π/4 - π) = (-1 - 0) / (1 + (-1)*0) = -1
So the value of tan(3π/4 - π) is also -1, which is the same as the value of tan(3π/4).
In other words, the value of tan(3π/4 - π) is equal to the value of tan(3π/4) because π is equal to 180 degrees, which is a full rotation. Subtracting a full rotation from an angle simply results in an equivalent angle in the opposite direction. Thus, the values of tangent for both angles remain the same.
A maritime flag is shown what is the area of the shaded part of the flag
Answers
Please help fill out!!!

Answers
0, becous A (-1x2) can't be negative.
Help pls I give brainlest

Answers
Answer:
y = 1/2x - 3
Step-by-step explanation:
The line intercepts with the y-axis at -3 and its pattern is going right two and up one.
NEED IT NOW!!!! Use the work shown below to solve. 1. Identify the operation in the equation. 2. Use inverse operations to solve. Subtraction property of equality 3/2 = k + 11/2 3/2 − 11/2 = k + 11/2 − 11/2 Addition property of equality 3/2 + (− 11/2) = k + 11/2 + (− 11/2) The solution is k = -7 -4 13/2 15/2

Answers
Answer:
-4
Step-by-step explanation:
3/2 = k + 11/2
Add -11/2 to each side using the addition property of equality
3/2 + -11/2 = k + 11/2+ -11/2
-8/2 = k
Simplify
-4 =k
Solution of k = -4 after applying the property of equality of addition and subtraction operation.
What is properties of equality?"Properties of equality represents the relation between two equal quantities after applying any operation on left hand side same has to be applied on the right hand side to make the balance in the given equation."
According to the question,
(3 / 2) = k + (11 / 2)
Here given operation is addition,
Apply inverse operation of addition,
Case1: Subtract 11/2 from both the sides we have,
(3 /2) - (11 / 2) = k + (11/ 2) - (11 / 2)
⇒ ( -8 /2) = k
⇒ k = -4
Case 2. Add ( - 11 /2) to both the sides we have,
( 3/ 2) + ( -11 /2) = k +( 11 /2) + (-11/ 2)
⇒( -8 /2) = k
⇒k = -4
Hence, solution of k = -4 after applying the property of equality of addition and subtraction.
Learn more about properties of equality here
https://brainly.com/question/13130806
#SPJ2
Show that the functions f(t) = t and g(t) = e^2t are linearly independent linearly independent by finding its Wronskian.
Answers
f(t) = t and g(t) = \(e^{(2t)\) form a linearly independent set of functions.
To show that the functions f(t) = t and g(t) = \(e^{(2t)\) are linearly independent, we can calculate their Wronskian and verify that it is nonzero for all values of t.
The Wronskian of two functions f(t) and g(t) is defined as the determinant of the matrix:
| f(t) g(t) |
| f'(t) g'(t) |
Let's calculate the Wronskian of f(t) = t and g(t) = \(e^{(2t)\):
f(t) = t
f'(t) = 1
g(t) = \(e^{(2t)\)
g'(t) = 2\(e^{(2t)\)
Now we can form the Wronskian matrix:
| t \(e^{(2t)\)|
| 1 2\(e^{(2t)\) |
The determinant of this matrix is:
Det = (t * 2\(e^{(2t)\)) - (1 * \(e^{(2t)\))
= 2t\(e^{(2t)\) - \(e^{(2t)\)
= \(e^{(2t)\) (2t - 1)
We can see that the determinant of the Wronskian matrix is not zero for all values of t. Since the Wronskian is nonzero for all t, it implies that the functions f(t) = t and g(t) = \(e^{(2t)\) are linearly independent.
Therefore, f(t) = t and g(t) = \(e^{(2t)\) form a linearly independent set of functions.
Learn more about Linearly Independent at
brainly.com/question/30575734
#SPJ4
The length of a rectangle is 6 inches longer than its width. What are the possible widths if the area of the rectangle is at least 667 square inches?

Answers
Answer:
D, \(w\geq 23\)
Step-by-step explanation:
The length is 6 inches longer than it's width, therefore:
\(l=w+6\)
The area of the rectangle is AT LEAST 667 square inches, so:
\(lw\geq 667\)
Now, we have a system of two equations:
\(\left \{ {{l=w+6} \atop {lw\geq 667}} \right.\)
We want to isolate the width, so substitute the first equation into the second to eliminate the length.
\((w+6)w\geq 667\)
Simplify:
\(w^2+6w\geq 667\)
Since we now have a polynomial equation that needs to be factored, set the value of one side to 0.
\(w^2+6w-667\geq 0\)
Now factor the equation:
\((w-23)(w+29)\geq 0\)
Taking each factor individually, we find the following:
\(w\geq 23\\w\geq -29\)
Since a negative width doesn't make any sense, we can eliminate that solution, and we are left with the correct answer!
\(w\geq 23\)
15 more than twice a number is 37. Wht is the number
Answers
A number which is 15 more than twice a number is 37 is 11.
Let the number be N.
Let's set up the equation.
A number is 15 more than twice a number is 37,
2N + 15 = 37
Let's subtract 15 from both sides.
2N = 22
Divide both sides by 2, and you get
N = 11
So the number which is 15 more than twice a number is 37 is 11.
A number system is a means of representing numbers. It is a method of representing numbers in a set of numbers consistently by using digits or other symbols. Each number receives a unique representation as well as information about the arithmetic and algebraic structure of the numbers. Addition, subtraction, multiplication, and division are just a few of the mathematical operations we may carry out using it.
Learn more about Number problems:
https://brainly.com/question/4697588
#SPJ4
( pls answer if u know)
A. -2/3
B. -3/2
C. 2/3
D. 3/2

Answers
Answer:
your answer would be B I hope it's right
Answer:
B)
Step-by-step explanation:
I hope this helps:)
Determine whether each function represents X potential growth or decay select the correct option for each function.
Please help me
2x
ƒ(x) = ( 1 ) ²*
f(x) = (7)x-¹
3x
f(x) = (1.3) ³x
f(x) = (0.8)*
2x
f(x) = (-²)*
2
Growth Decay
0
0
0
0
O
O
Answers
Growth Decay
2x, ƒ(x) = (1)²*, 3x, f(x) = (1.3)³x - Growth
f(x) = (0.8)*2, f(x) = (-²)*2 - Decay
(The functions are categorized as either representing growth or decay.)
Growth Decay
2x - Growth (The function 2x represents exponential growth because as x increases, the value of 2x also increases.)
ƒ(x) = (1)²* - Growth (The function ƒ(x) = (1)² represents exponential growth because any number raised to the power of 2 will always be positive.)
f(x) = (7)x-¹ - Decay (The function f(x) = (7)x-¹ represents exponential decay because as x increases, the value of (7)x-¹ decreases.)
3x - Growth (The function 3x represents exponential growth because as x increases, the value of 3x also increases.)
f(x) = (1.3)³x - Growth (The function f(x) = (1.3)³x represents exponential growth because as x increases, the value of (1.3)³x also increases.)
f(x) = (0.8)*2 - Decay (The function f(x) = (0.8)*2 represents exponential decay because any number raised to the power of 2 will always be positive, and multiplying by 0.8 reduces the value.)
f(x) = (-²)*2 - Decay (The function f(x) = (-²)*2 represents exponential decay because any number raised to the power of 2 will always be positive, but the negative sign in front indicates a decay.)
For more such questions on Growth Decay, click on:
https://brainly.com/question/29634879
#SPJ11
I’m confused, I don’t know how to get the value

Answers
Answer:
i think 58, but if not, go to khan academy and watch a video!
Step-by-step explanation:
PLEASE HELP ME I'M BEHIND ON MY MATH AND I CAN'T FIND ANY ANSWERS!!! :(
Two banks offer to loan you money for a big purchase. How would you use your knowledge of simple interest to determine which loan to choose for this purchase?
(20 points)
(Written Response)
( E d g e n u i t y 2021)
(Mathematics 7 A)
(Journal Activity JAN 05.)
** Please answer to this if you did it already did this assignment already..! I'm behind on my math and I can't find any thing to help me out! **
Answers
Answer:
you would see which bank has a good business and have loyal customers and which one is giving you a better offer
Step-by-step explanation:
HOPE THIS HELPED!
Answer:
e.
Step-by-step explanation:
An article costing
Rs 6000 is sold at Rs 4,200.
Find the discount percentage?
Answers
Answer:
discount=30%
hope it helps.

I need help please help me

Answers
Answer:
did you use google.if not use it it is good to use.
Determine whether the given relation is an implicit solution to the given differential equation. Assume that the relationship does define y implicitly as a function of x and use implicit differentiation.
Answers
If it is not true, then the relation is not an implicit solution to the differential equation.
You have not provided the given relation and differential equation, so I will provide general steps to determine whether a given relation is an implicit solution to a differential equation using implicit differentiation and assuming that the relation defines y implicitly as a function of x.
1. Differentiate the relation with respect to x using implicit differentiation.
2. Substitute y' for dy/dx.
3. Simplify the resulting expression by collecting like terms.
4. Substitute the original relation into the simplified expression to obtain a statement that must be true if the relation is an implicit solution to the differential equation.
5. Check whether the resulting statement is true for all values of x and y that satisfy the original relation. If it is true, then the relation is an implicit solution to the differential equation.
To learn more about : equation
https://brainly.com/question/17145398
#SPJ8
A hot-air balloon 70 meters above the ground is falling at a constant rate of 6
meters per second while another hot-air balloon 10 meters above the ground is
rising at a constant rate of 15 meters per second. To the nearest tenth of a second,
after how many seconds will the 2 balloons be the same height above the
ground?
A. 8.9
B. 6.7
C. 2.9
D. 0.4
E. 0.2
Answers
Which phase of inferential statistics is sometimes considered to be the most crucial because errors in this phase are the most difficult to correct?
Answers
The phase of inferential statistics which is sometimes considered to be the most crucial because errors in this phase are the most difficult to correct is "data gathering".
What is inferential statistics?Inferential statistics are frequently employed to compare treatment group differences.
Some characteristics of inferential statistics are-
Inferential statistics compare treatments groups and make conclusions about the greater population of participants using measures from the experiment's sample of subjects.Inferential statistics aids in the development of explanations for a condition or phenomenon. It enables you to draw conclusions on extrapolations, which distinguishes it from descriptive statistics, which simply summarize the information that has been measured.There are numerous varieties of inferential statistics, each with its own set of research design & sample characteristics. To select the correct statistical test of their experiment, researchers should reference the numerous texts about experimental design and statistics.To know more about the inferential statistics, here
https://brainly.com/question/4774586
#SPJ4
In year N, the 300th day of the year is a Tuesday. In year N+1, the 200th day is also a Tuesday. On what day of the week did the 100thth day of year N-1 occur ?
Answers
Therefore, if the 300th day of year N is a Tuesday, the 100th day of year N-1 will be a Sunday.
To determine the day of the week on the 100th day of year N-1, we need to analyze the given information and make use of the fact that there are 7 days in a week.
Let's break down the given information:
In year N, the 300th day is a Tuesday.
In year N+1, the 200th day is also a Tuesday.
Since there are 7 days in a week, we can conclude that in both years N and N+1, the number of days between the two given Tuesdays is a multiple of 7.
Let's calculate the number of days between the two Tuesdays:
Number of days in year N: 365 (assuming it is not a leap year)
Number of days in year N+1: 365 (assuming it is not a leap year)
Days between the two Tuesdays: 365 - 300 + 200 = 265 days
Since 265 is not a multiple of 7, there is a difference of days that needs to be accounted for. This means that the day of the week for the 100th day of year N-1 will not be the same as the given Tuesdays.
To find the day of the week for the 100th day of year N-1, we need to subtract 100 days from the day of the week on the 300th day of year N. Since 100 is a multiple of 7 (100 = 14 * 7 + 2), the day of the week for the 100th day of year N-1 will be two days before the day of the week on the 300th day of year N.
To know more about year,
https://brainly.com/question/28206231
#SPJ11
Find the missing side of each triangle

Answers
The value of x using Pythagoras theorem is: x = √118 mi
How to use Pythagoras theorem?Pythagoras Theorem is defined as the way in which you can find the missing length of a right angled triangle.
The triangle has three sides, the hypotenuse (which is always the longest), Opposite (which doesn't touch the hypotenuse) and the adjacent (which is between the opposite and the hypotenuse).
Pythagoras is in the form of;
a² + b² = c²
Thus:
x = √(12² - (√26)²)
x = √(144 - 26)
x = √118 mi
Read more about Pythagoras theorem at: https://brainly.com/question/231802
#SPJ1
Use absolutely value to express the distance between 10 and -4
Answers
Answer:
16
Step-by-step explanation:
Answer:
The answer 14
Step-by-step explanation:
