I haven't did my math for a month since brainly tutoring wasn't working. I need you guys help

Answers
Answer:
$2.50
Step-by-step explanation:
Answer:
$25 per ft2
Step-by-step explanation:
you have to calculate the area (20x8=160sq ft) and then get 4000/160 which is $25.
Related Questions
Two graduate students are each doing a study and are pulling
their samples from the same population. The first investigator
takes a sample of 100 and the second takes a sample of 2,000.
Which student will tend to get the larger standard deviation in
his/her sample?
a The first investigaton
b The second investigator
c None of these
Answers
whats 27 x 300 / 34 x 6
Answers
Answer:
Use Calculator:
Approximately. 1429.411
Step-by-step explanation:
Hope it Helps!!!
look at the graph of a line and write its equation

Answers
Answer:
y = 5x + 0.25
Step-by-step explanation:
M is the slope which is 5 units y per 1 x unit. B is the y-intercept which seems to be 0.25.
fuihiudfgsguyfguygdfuys
send help

Answers
Answer:
a.2,3,4
Step-by-step explanation:
Two students have different ways to calculate as a decimal. On your answer sheet explain and show, in detail, how each student might compute this product. Find each answer. A. Elena says, "I find 25 times 44 and then move the decimal point. "
b. Diego says, "0. 25 is the same as , so I found of 0. 44. "
a. Elena's answer is:
Question Blank 1 of 2
type your answer. B. Diego's answer is:
Question Blank 2 of 2
type your answer
Answers
Both Elena and Diego get the same answer of 0.11, but they use different methods to calculate the product of 0.25 x 0.44 as a decimal.
A. Elena's method of calculating 0.25 x 0.44 as a decimal involves finding the product of 25 and 44, and then moving the decimal point. To do this, she would first multiply 25 x 44 to get 1100. Then, she would move the decimal point two places to the left, since there are two decimal places in the original numbers (0.25 and 0.44). This would give her the answer of 0.11. Therefore, Elena's answer is 0.11.
B. Diego's method of calculating 0.25 x 0.44 as a decimal involves finding 1/4 of 0.44. To do this, he would first divide 0.44 by 4 to get 0.11. This is the same as finding 25% of 0.44, since 1/4 is equivalent to 25%. Therefore, Diego's answer is 0.11.
Learn more about multiplication and decimals:https://brainly.com/question/28338004
#SPJ11
Solve 2(1-x)>2x
X<0.5
X<2
X>2
X>0.5

Answers
Answer:
x < 0.5
Step-by-step explanation:
2(1 - x) > 2x ( divide both sides by 2 )
1 - x > x ( add x to both sides )
1 > 2x ( divide both sides by 2 )
0.5 > x , that is
x < 0.5
when writing the equation of a line of fit, what strategies can you use to pick two points from which to write the equation?
Answers
The equation of the line of fit can be written as y = x + 4.
Two points from which to write the equation of a line of fit can be determined by using the mean, median, and mode of the data set. The mean, median, and mode help to identify the center of the data set, which helps to identify two points that can be used to write the equation of the line of fit. The mean is calculated by taking the sum of all the data points and dividing it by the total number of data points. The median is the middle value of the data set when the data points are ordered from lowest to highest. The mode is the value that appears the most in the data set. By finding the mean, median, and mode of the data set, two points that can be used to write the equation of the line of fit can be determined. The two points can be determined by taking the mean, median, or mode value of the data set, and adding or subtracting an arbitrary value from that point. For example, if the mean of the data set is 8 and an arbitrary value of 2 is added to it, the two points of the line of fit can be (8, 10) and (8, 6). Using these two points, the equation of the line of fit can be written as y = x + 4.
Learn more about equation here:
https://brainly.com/question/1566730
#SPJ4
The blue segment below is a diameter of oo. What is the length of the radius
of the circle?
A. 4.1 units
B. 16.4 units
C. 8.2 units
D. 12.3 units

Answers
Radius is half of the diameter:
8.2 / 2 = 4.1
The answer is A. 4.1 units.
The blue segment below is a diameter of 8.2 units. so, the length of the radius of the circle would be 4.1 units.
What is the diameter?Diameter is defined as the line from one point in the circle to the other point through the center of the circle.
Diameter = radius / 2
The radius is half of the diameter
The blue segment below is a diameter of oo. we need to find the length of the radius of the circle.
The diameter of the circle is given as 8.2 units.
We know that the radius is half of the diameter
= 8.2 / 2
= 4.1
Learn more about diameter;
https://brainly.com/question/5501950
SOMEONE PLEASE HELP ME WITH THIS!!!!!!

Answers
Because if you solved it
10z + 20 = 80
-20 -20
10z = 60
/10 /10
Given circle O , m∠EDF=31° . Find x .

Answers
The calculated value of x in the circle is 59
How to calculate the value of xFrom the question, we have the following parameters that can be used in our computation:
The circle
The measure of angle at the center of the circle is calculated as
Center = 2 * 31
So, we have
Center = 62
The sum of angles in a triangle is 180
So, we have
x + x + 62 = 180
This gives
2x = 118
Divide by 2
x = 59
Hence, the value of x is 59
Read more about circles at
https://brainly.com/question/32192505
#SPJ1
Help plzzzzzzzz do these two questions and I’ll mark Brainlynest


Answers
Answer:
Step-by-step explanation:
Q1: <B = 150 °
If two parallel lines are cut by a transversal, then alternate interior angles are congruent.
8x-10 = 3x+90
5x=100 (subtract 3 from both sides)
x=20 (divide both sides by 5)
x = 20
<B= 3x +90
<B = 3 (20) +90
<B = 60 + 90
<B = 150
Q2: 62
An angles complement (not compliment, haha) is what the number that added to the angle equals 90°.
An angle is 34 more than its complement.
Let x represent the angle.
Let y represent its complement.
x= 34 +(90-x)
y= (90-x)
x + y = 90
34 + (90-x) + (90-x) =90
x= 62
Check the answer.
x= 34 more than complement.
62-34=28
62+28=90
Checked!
Words of encouragement:
Good luck!
what does the coefficient used to assess the internal consistency of a measure represent?
Answers
The coefficient used to assess the internal consistency of a measure typically refers to Cronbach's alpha coefficient.
What does the coefficient used to assess the internal consistency of a measure represent?The coefficient used to assess the internal consistency of a measure typically refers to Cronbach's alpha coefficient. This coefficient is a statistic that quantifies the internal consistency reliability of a scale or measure, specifically for measures with multiple items or indicators that are intended to measure the same underlying construct.
The coefficient ranges between 0 and 1, with higher values indicating greater internal consistency. Cronbach's alpha measures the extent to which the items in a scale or measure correlate with each other. It assesses how well the items are measuring the same underlying construct or concept.
In other words, the coefficient represents the proportion of variance in the observed scores that can be attributed to the true score, rather than measurement error or other extraneous factors. It indicates the degree to which the items in the measure are interrelated or consistent with each other, with higher values suggesting stronger internal consistency.
Researchers often use Cronbach's alpha to assess the reliability of scales in various fields, such as psychology, education, and social sciences, to ensure that the measure is measuring the construct consistently and accurately.
Learn more about coefficient on:
https://brainly.com/question/1038771
#SPJ4
HELP WILL MARK BRAINLIST AND 5 STARS

Answers
Answer:
10 feet
Step-by-step explanation:
We are trying to find Madison's height plus Jade's height...
Turn the fractions into improper fractions
So,
5 1/6 = 31/6
4 5/6 = 29/6
31/6 + 29/6 = 10 feet
or
5+4= 10 and 1/6+5/6 = 1
so the answer is 10 feet
PLEASE RATE!! I hope this helps!!!
If you have any questions comment below
Find an equation for the plane containing the two (parallel) lines
v1 = (0, 1, −8) + t(6, 7, −5) and v2 = (8, −1, 0) + t(6, 7, −5).
Answers
The equation of the plane containing the two parallel lines v₁ = (0, 1, −8) t(6, 7, −5) and v₂ = (8, −1, 0) t(6, 7, −5) is 6x + 6y + 3z = 0.
What are parallel lines?
Parallel lines are coplanar infinite straight lines that do not intersect at any point in geometry. Parallel planes are planes that never meet in the same three-dimensional space. Parallel curves are those that do not touch or intersect and maintain a constant minimum distance.
To find an equation for the plane containing the two parallel lines v₁ = (0, 1, −8) t(6, 7, −5) and v₂ = (8, −1, 0) t(6, 7, −5),
We use the equation of a line: v = v₀ + tv₁
where v₀ and v₁ are points on the line and t is a real number.
Substitute the given points in for v₀ and v₁: v = (0, 1, −8) + t(6, 7, −5)
This equation of the plane is Ax + By + Cz = D, where A, B, C, and D are constants to be determined.
Equate the components:
0x + 1y - 8z = D....(1)
6x + 7y - 5z = D...(2)
Now, we subtract equation (1) from (2) and we get
6x - 0x + 7y - 1y - 5z + 8z = 0
6x + 6y + 3z = 0
Hence, the equation of the plane containing the two parallel lines v₁ = (0, 1, −8) t(6, 7, −5) and v₂ = (8, −1, 0) t(6, 7, −5) is 6x + 6y + 3z = 0.
To learn more about the parallel line from the given link
https://brainly.com/question/24607467
#SPJ4
Question 1: Draw one function which is discontinuous at x = -2, x = 1, and z = 3 where the discontinuities are caused by a jump, a vertical asymptote, and a hole in the graph. Question 2: Find the values of the constant c which makes the function continuous on the interval (-[infinity], [infinity]): f(x) = [cr¹ +7cx³+2, x < -1 |4c-x²-cr, x ≥ 1 Question 3: Show that the following equation has at least one real root on the following intervals: f(x) = 4x²-3x³ + 2x²+x-1 on [-0.6,-0.5]
Answers
1: Discontinuous functions can contain a hole, a vertical asymptote, and a jump.
2: The values of c that make the function continuous on the interval (-∞, ∞) are c = -2 or c = 1/3.
3: The function f(x) = 4x² - 3x³ + 2x² + x - 1 has at least one real root on the interval [-0.6, -0.5].
1: Discontinuous functions can exhibit different types of discontinuities, such as holes, vertical asymptotes, and jumps. Let's consider an example of a discontinuous function. In the given function, there are discontinuities at x = -2, x = 1, and z = 3. Each of these discontinuities corresponds to a different type. At x = -2, there is a jump in the function, which means the function changes abruptly at that point. The function is not differentiable at a jump. At x = 1, there is a vertical asymptote, where the function approaches infinity or negative infinity. This indicates that the function is not defined at that point. At z = 3, there is a hole in the graph. The function is undefined at the hole, but we can define it by creating a gap in the graph and connecting the points on either side of it.
2:
To find the values of the constant c that make the function continuous on the interval (-∞, ∞), we need to equate the two parts of the function at x = -1. By doing this, we can determine the value of c that ensures the function is continuous. The given function is f(x) = cr¹ + 7cx³ + 2, for x < -1, and f(x) = 4c - x² - cr, for x ≥ 1.
To make f(x) continuous at x = -1, we equate the two parts of the function:
cr¹ + 7cx³ + 2 = 4c - x² - cr
Simplifying this equation, we obtain:
cr² + 3cr - 5c + 2 = 0
This is a quadratic equation in terms of c, which can be solved to find the value(s) of c that make the function continuous. The solutions are c = -2 or c = 1/3.
3:
If the given equation has at least one real root on the interval [-0.6, -0.5], it means the function must change sign between -0.6 and -0.5. To demonstrate this, let's evaluate the function f(x) = 4x² - 3x³ + 2x² + x - 1 at the endpoints of the interval and check if the signs change.
First, we evaluate f(-0.6):
f(-0.6) = 4(-0.6)² - 3(-0.6)³ + 2(-0.6)² - 0.6 - 1 = -0.59
Next, we evaluate f(-0.5):
f(-0.5) = 4(-0.5)² - 3(-0.5)³ + 2(-0.5)² - 0.5 - 1 = -0.415
Since f(-0.6) and f(-0.5) have different signs, it implies that f(x) must have at least one real root on the interval [-0.6, -0.5]. Therefore, it can be concluded that the function f(x) = 4x² - 3x³ + 2x² + x - 1 has at least one real root on the interval [-0.6, -0.5].
Learn more about function
https://brainly.com/question/31062578
#SPJ11
If you reported to work at 18.00 on Wednesday and you get off at 02.50 on a Thursday, which day of pay period week are you working?
Answers
Answer:
Only Wednesday and Thursday
Step-by-step explanation:
A solid circular rod of diameter d undergoes a bending moment M-1000 lbf.in including a stress 32 Using a material strength of25 kpsi and a design factor of2.5 a) determine the minimum diameter of the rod. b) Using the following table, select a preferred fractional diameter and σ = do determine the resulting factor of safety
Answers
To determine the minimum diameter of the rod, we can use the formula for bending stress:
σ = (M * c) / (I * y)
Where:
σ is the bending stress
M is the bending moment
c is the distance from the neutral axis to the outermost fiber
I is the moment of inertia of the cross-section
y is the perpendicular distance from the neutral axis to the point where the stress is being calculated
Given:
M = -1000 lbf.in
σ = 32 kpsi = 32,000 psi
Strength = 25 kpsi
Design factor = 2.5
First, we need to convert the bending moment to pound-force feet (lbf.ft):
M = -1000 lbf.in = -83.33 lbf.ft (1 lbf.in = 0.0833 lbf.ft)
Next, we can rearrange the bending stress formula to solve for the moment of inertia (I):
I = (M * c) / (σ * y)
Since we are looking for the minimum diameter, we want to minimize the moment of inertia. This occurs when the rod is a solid cylinder with its maximum diameter.
The moment of inertia of a solid circular rod is given by the formula:
I = (π * d^4) / 64
Substituting the formulas and given values, we can solve for the minimum diameter (d):
(π * d^4) / 64 = (M * c) / (σ * y)
d^4 = (64 * M * c) / (π * σ * y)
d = ∛((64 * M * c) / (π * σ * y))^0.25
Once we have the minimum diameter (d), we can select a preferred fractional diameter from the table provided and calculate the resulting factor of safety using the formula:
Factor of Safety = (Strength * Design Factor) / σ
Please provide the values of c, y, and the preferred fractional diameter from the table so that I can help you with the calculations.
The minimum diameter of the rod is approximately 1.37 inches.A preferred fractional diameter that corresponds to a factor of safety greater than or equal to 0.78125, ensuring a safe design.
a) To determine the minimum diameter of the rod, we can use the formula for bending stress:
σ = M / (0.25 * π * (d^3))
Rearranging the formula, we have:
d^3 = M / (0.25 * π * σ)
Substituting the given values, we get:
d^3 = 1000 / (0.25 * π * 32)
Solving for d, we find:
d ≈ 1.37 inches
Therefore, the minimum diameter of the rod is approximately 1.37 inches.
b) To select a preferred fractional diameter and calculate the resulting factor of safety, we need to compare the calculated stress with the material strength and design factor.
Given the stress σ = 32 kpsi and a material strength of 25 kpsi, we can calculate the factor of safety:
Factor of Safety = (Material Strength) / (Design Stress)
Factor of Safety = 25 / 32
Factor of Safety ≈ 0.78125
Referring to the provided table, we can choose a preferred fractional diameter that corresponds to a factor of safety greater than or equal to 0.78125, ensuring a safe design.
To learn more about fractional diameter click here : brainly.com/question/19425002
#SPJ11
Solve: 4 1/5 − 3/7 plese sent the answer
Answers
Answer:
\(4 \frac{1}{5} - \frac{3}{7} \\ =4 + \frac{1}{5} - \frac{3}{7} \\ = \frac{21}{5} - \frac{3}{7} \\ \frac{147 - 15}{35} \\ = \frac{132}{35}
=3 27/35 \\ thank \: you\)
132/35
Step-by-step explanation:
\(4 \frac{1}{5} - \frac{3}{7} \\ \frac{21}{5} - \frac{3}{7} \\ lcm = 35 \\ \frac{21 \times 7}{5 \times 7} - \frac{3 \times 5}{7 \times 5} \\ \frac{147}{35} - \frac{15}{35} \\ = \frac{132}{35} \\ \)
Write an equation for a quadratic function that has x intercepts (-3, 0) and (5, 0)
Answers
Answer:
One possible equation is \(f(x) = (x + 3)\, (x - 5)\), which is equivalent to \(f(x) = x^{2} - 2\, x - 15\).
Step-by-step explanation:
The factor theorem states that if \(x = x_{0}\) (where \(x_{0}\) is a constant) is a root of a function, \((x - x_{0})\) would be a factor of that function.
The question states that \((-3,\, 0)\) and \((5,\, 0)\) are \(x\)-intercepts of this function. In other words, \(x = -3\) and \(x = 5\) would both set the value of this quadratic function to \(0\). Thus, \(x = -3\!\) and \(x = 5\!\) would be two roots of this function.
By the factor theorem, \((x - (-3))\) and \((x - 5)\) would be two factors of this function.
Because the function in this question is quadratic, \((x - (-3))\) and \((x - 5)\) would be the only two factors of this function. In other words, for some constant \(a\) (\(a \ne 0\)):
\(f(x) = a\, (x - (-3))\, (x - 5)\).
Simplify to obtain:
\(f(x) = a\, (x + 3)\, (x - 5)\).
Expand this expression to obtain:
\(f(x) = a\, (x^{2} - 2\, x - 15)\).
(Quadratic functions are polynomials of degree two. If this function has any factor other than \((x - (-3))\) and \((x - 5)\), expanding the expression would give a polynomial of degree at least three- not quadratic.)
Every non-zero value of \(a\) corresponds to a distinct quadratic function with \(x\)-intercepts \((-3,\, 0)\) and \((5,\, 0)\). For example, with \(a = 1\):
\(f(x) = (x + 3)\, (x - 5)\), or equivalently,
\(f(x) = x^{2} - 2\, x - 15\).
What is the place value of the 2 in 150.1235?
Hundredths
Tenths
Tens
Thousandths
Answers
Answer:
The 2 is placed in the Hundredths place
Step-by-step explanation:
Hope it helps! =D
Evaluate the following improper integral or determine whether it is convergent or divergent. Clearly state any rules used and/or reasons for your answer. dr z(Inz)
Answers
we can conclude that the integral ∫ z ln(z) dz is convergent for any positive limit of integration and can be evaluated using the expression \((1/2)z^2 ln(z) - z^2/4 + C\), where C is the constant of integration.
To evaluate the improper integral ∫ z ln(z) dz, we need to determine whether it is convergent or divergent.
First, let's check if there are any points where the integrand is undefined or approaches infinity within the given limits of integration.
The integrand z ln(z) is undefined for z ≤ 0 because the natural logarithm is not defined for non-positive numbers. Therefore, we need to make sure our integration limits do not include or cross over any values of z ≤ 0.
If the lower limit of integration is zero or approaches zero, the integral would be improper. However, if the lower limit of integration is a positive value, we can proceed with the evaluation.
Let's assume the lower limit of integration is a > 0.
Now, let's evaluate the integral using integration by parts. Integration by parts is a technique used to integrate the product of two functions, u and v, using the formula:
∫ u dv = uv - ∫ v du
In our case, we can choose u = ln(z) and dv = z dz. Taking the derivatives and integrating, we have:
du = (1/z) dz
v = \((1/2)z^2\)
Using the formula, we get:
∫ z ln(z) dz =\((1/2)z^2 ln(z)\) - ∫\((1/2)z^2 (1/z) dz\)
= \((1/2)z^2 ln(z) - (1/2)\) ∫ z dz
= \((1/2)z^2 ln(z) - (1/2) (z^2/2)\) + C
= \((1/2)z^2 ln(z) - z^2/4\) + C,
where C is the constant of integration.
Next, we need to determine whether this integral is convergent or divergent. For an improper integral to be convergent, the limit of the integral as the limit of integration approaches a certain value must exist and be finite.
In this case, as long as the limit of integration is a positive value, the integral is convergent. However, if the limit of integration approaches or crosses zero (z ≤ 0), the integral is divergent due to the undefined nature of the integrand in that region.
Therefore, we can conclude that the integral ∫ z ln(z) dz is convergent for any positive limit of integration and can be evaluated using the expression \((1/2)z^2 ln(z) - z^2/4 + C\), where C is the constant of integration.
Learn more about integral here:
brainly.com/question/18125359
#SPJ4
A ____ is a measuring stick that represents the actual wall frame, with markings made at the proper height for every horizontal member of the wall frame.
Answers
Answer:
Story Pole
Step-by-step explanation:
Source : Trust me bro
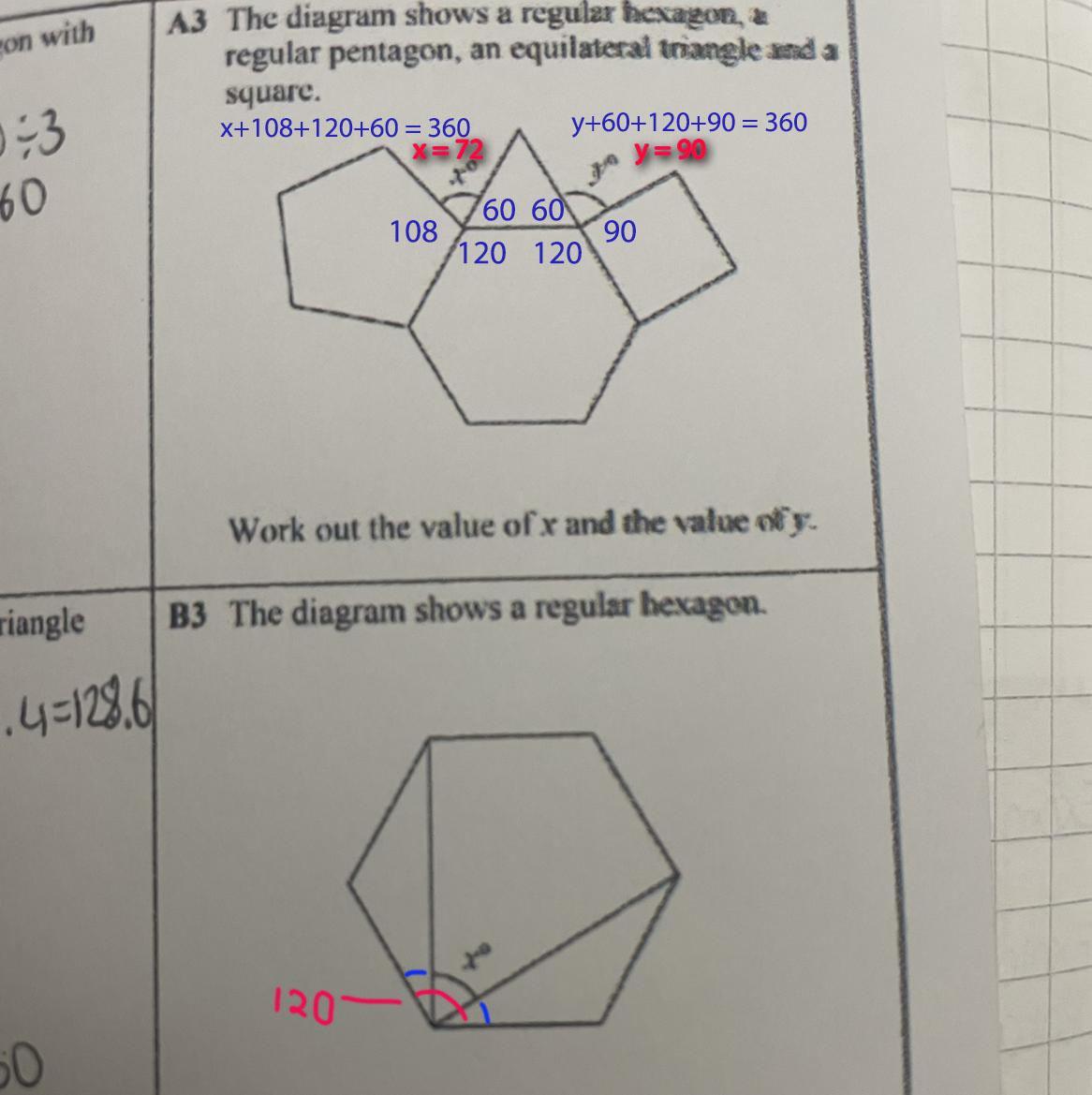
What is the answer to this question?

Answers
Answer:
A.3. x = 72° y = 90°
Step-by-step explanation:
The sum of the interior angles in a polygon is (n-2)*180, where n is the number of angles. In this diagram, we have:
Hexagon n=6): (6-2)*180° = 720°
Pentagon (n=5): (5-2)*180° = 540°
Triangle (n=3): (3-2)*180° = 180°
Square (n=4): (4-2)*180° = 360°
In this case, all are either regular or equilateral, so the angles will all be the same within each polygon.
Hexagon: (720°/6) = 120°
Pentagon: (540°/5) = 108°
Triangle = 60°
Square = 90°
Refer to the attached diagram for the calculations.
By adding up the angles we know that are adjacent to x and y, and setting the total to 360°, we can calculate the missing angles x and y.
x = 72°
y = 90°
I started the problem for B3, but am not certain how to find the angles for the isosceles triangles without more information.
Solve the following equations. Make sure you show each step of the work including any Distributive Property,
Inverse operations and combining/collecting of like terms that may be needed. Remember that what you do to
one side you must do to the other when using inverse equations. Label your response with the same letter of the
problem AND write the original problem first before you begin to solve.
A) 1d + 12 = 14-20
B) 4(20+5) = 10r
C) -2(5 + x) - 1 = 3(x + 3)
Answers
Answer:
A) -18 B) r=10 C) x=-4
Step-by-step explanation:
A) 1d + 12 = 14-20
Step 1: Simplify both sides of the equation.
1d+12=14−20
d+12=14+−20
d+12=(14+−20)(Combine Like Terms)
d+12=−6
d+12=−6
Step 2: Subtract 12 from both sides.
d+12−12=−6−12
d=−18
Answer:
d=−18
B) 4(20+5) = 10r
Step 1: Simplify both sides of the equation.
100=10r
Step 2: Flip the equation.
10r=100
Step 3: Divide both sides by 10.
r=10
C) -2(5 + x) - 1 = 3(x + 3)
Step 1: Simplify both sides of the equation.
−2(5+x)−1=3(x+3)
(−2)(5)+(−2)(x)+−1=(3)(x)+(3)(3)(Distribute)
−10+−2x+−1=3x+9
(−2x)+(−10+−1)=3x+9(Combine Like Terms)
−2x+−11=3x+9
−2x−11=3x+9
Step 2: Subtract 3x from both sides.
−2x−11−3x=3x+9−3x
−5x−11=9
Step 3: Add 11 to both sides.
−5x−11+11=9+11
−5x=20
Step 4: Divide both sides by -5.
x=−4
A rectangle has a perimeter of 64. the width is 3 less than the length. the equation for the scenario is 2x 2(x â€"" 3) = 64. what does the variable x represent in the equation? what will finding x â€"" 3 determine?
Answers
The variable x represent the length of rectangle.
What is Algebra?A branch of mathematics known as algebra deals with symbols and the mathematical operations performed on them.
Variables are the name given to these symbols because they lack set values.
In order to determine the values, these symbols are also subjected to various addition, subtraction, multiplication, and division arithmetic operations.
Given:
Perimeter= 64 units
let the length be x.
So, width = x - 3
So, Perimeter of rectangle = 2 ( l + w)
64 = 2( x+ x -3)
32 = 2x -3
29= 2x
x= 29/2
Hence, the variable x represent the length of rectangle.
Learn more about Algebra here:
https://brainly.com/question/24875240
#SPJ1
1. What does the mean of a dataset represent? What does knowing
the standard deviation of a
dataset add?
2. What is a p value? What is the meaning of a p value?
3. Define alpha. How is it related to p
Answers
1. The mean of a dataset represents the average value of the entire set of numbers. It is calculated by adding up all the values and dividing by the number of values in the dataset. Knowing the standard deviation of a dataset adds information about how much the values deviate from the mean.
A high standard deviation indicates that the values are more spread out from the mean, while a low standard deviation indicates that the values are closer to the mean. It can help to identify outliers and the overall variability of the dataset.
2. A p-value is a statistical measure that helps to determine whether the null hypothesis (there is no significant difference between two groups) is true or false.
It is the probability of obtaining a result as extreme or more extreme than the observed result, assuming that the null hypothesis is true. A p-value of less than 0.05 (typically) is considered statistically significant, which means that there is strong evidence to reject the null hypothesis and accept the alternative hypothesis (there is a significant difference between the two groups).
3. Alpha is the level of significance that is set to determine whether a p-value is statistically significant or not. It is typically set at 0.05, which means that if the p-value is less than 0.05, then the result is considered statistically significant and the null hypothesis is rejected.
If the p-value is greater than 0.05, then the result is not statistically significant and the null hypothesis cannot be rejected. In summary, alpha and p-value are related because alpha sets the threshold for determining whether a p-value is statistically significant or not.
Learn more about hypothesis from:
https://brainly.com/question/26185548
#SPJ11
a researcher would like to estimate p, the proportion of u.s. adults who support recognizing civil unions between gay or lesbian couples. if the researcher would like to be 95% sure that the obtained sample proportion would be within 1.5% of p (the proportion in the entire population of u.s. adults), what sample size should be used? group of answer choices 4,445 45 1,112 67 17,778
Answers
To estimate p, the proportion of U.S. adults who support recognizing civil unions between gay or lesbian couples, with 95% confidence and a margin of error of 1.5%.
We need to use the following formula to calculate the required sample size:n = (Z^2 * p * (1-p)) / E^2
Where:
- Z is the standard normal value corresponding to the desired level of confidence, which is 1.96 for 95% confidence.
- p is the estimated population proportion, which we don't know yet.
- E is the desired margin of error, which is 0.015 (1.5%).
Let's assume that p is 0.6, which means that we expect 60% of U.S. adults to support recognizing civil unions between gay or lesbian couples.
Plugging in the values:
n = (1.96^2 * 0.5 * (1-0.5)) / 0.015^2
n = (3.8416 * 0.5 * 0.5) / 0.000225
n = 1.9208 / 0.000225
n = 8531.55556
Rounding up to the nearest integer, we need a sample size of 4,445 to be 95% confident that the obtained sample proportion will be within 1.5% of the population proportion. Therefore, the correct answer is 4,445.
To learn more about sample : brainly.com/question/13287171
#SPJ11
What are the four conditions necessary for X to have a Binomial Distribution? Mark all that apply.
a. There are n set trials.
b. The trials must be independent.
c. Continue sampling until you get a success.
d. There can only be two outcomes, a success and a failure
e. You must have at least 10 successes and 10 failures
f. The population must be at least 10x larger than the sample. T
g. he probability of success, p, is constant from trial to trial
Answers
Options a, b, d, and g are the correct conditions for a Binomial Distribution.
The four conditions necessary for X to have a Binomial Distribution are:
a. There are n set trials: In a binomial distribution, the number of trials, denoted as "n," must be predetermined and fixed. Each trial is independent and represents a discrete event.
b. The trials must be independent: The outcomes of each trial must be independent of each other. This means that the outcome of one trial does not influence or affect the outcome of any other trial. The independence assumption ensures that the probability of success remains constant across all trials.
d. There can only be two outcomes, a success and a failure: In a binomial distribution, each trial can have only two possible outcomes. These outcomes are typically labeled as "success" and "failure," although they can represent any two mutually exclusive events. The probability of success is denoted as "p," and the probability of failure is denoted as "q," where q = 1 - p.
g. The probability of success, p, is constant from trial to trial: In a binomial distribution, the probability of success (p) remains constant throughout all trials. This means that the likelihood of the desired outcome occurring remains the same for each trial. The constant probability ensures consistency in the distribution.
The remaining options, c, e, and f, are not conditions necessary for a binomial distribution. Option c, "Continue sampling until you get a success," suggests a different type of distribution where the number of trials is not predetermined. Options e and f, "You must have at least 10 successes and 10 failures" and "The population must be at least 10x larger than the sample," are not specific conditions for a binomial distribution. The number of successes or failures and the size of the population relative to the sample size are not inherent requirements for a binomial distribution.
Learn more about Binomial Distribution at: brainly.com/question/29137961
#SPJ11
Alex knits hats and scarves to sell at an art fair. He can make at most 20 hats and 30 scarves, but no more than 40 items altogether, in time for the art fair. Write and graph a system of inequalities that show possible numbers of hats and scarves Alex can bring to the art fair if he wants to bring at least 25 items.
Answers
Let h be the number of hats and s be the number of scarves that Alex can bring to the art fair. Then, the system of inequalities can be written as:
h ≤ 20 (since he can make at most 20 hats)
s ≤ 30 (since he can make at most 30 scarves)
h + s ≤ 40 (since he can make no more than 40 items altogether)
h + s ≥ 25 (since he wants to bring at least 25 items)
To graph this system of inequalities, we can plot the lines h = 20, s = 30, h + s = 40, and h + s = 25, and shade the region that satisfies all the inequalities. The resulting graph will be a quadrilateral with vertices at (20, 0), (0, 30), (10, 15), and (20, 5). The shaded region will be the area inside this quadrilateral that is above the line h + s = 25.
1.75 x 1.25 estimate
Answers
Answer:
2.1875
(in nearest hundredths it would be 2.19(rounded)