how do you
graph y= -3x-10
Answers
Answer:
slope: -3
Y- intercept: (0,10)
Related Questions
What is the value of n 8n-5=139
Answers
The value of 'n' is 18 in the given equation 8n - 5 = 139.
We have to find the value of 'n'.
To do so, we need to solve the given equation.
Let us see how we can solve the given equation.8n - 5 = 139
We need to find the value of 'n'.
Let us bring the constant term to the right side by adding 5 to both the sides of the equation.8n - 5 + 5 = 139 + 5On simplifying, we get8n = 144Now, we need to isolate 'n' to find its value.
To do so, we divide both the sides by 8.8n/8 = 144/8On simplifying, we get n = 18
Therefore, the value of 'n' is 18.
Hence, the value of 'n' is 18 in the given equation 8n - 5 = 139.
For more questions on equation
https://brainly.com/question/17145398
#SPJ8
Which equations represent circles that have a diameter of 12 units and a center that lies on the y-axis? Select two options.
x2 + (y – 3)2 = 36
x2 + (y – 5)2 = 6
(x – 4)² + y² = 36
(x + 6)² + y² = 144
x2 + (y + 8)2 = 36
Answers
Since the center of the circle lies on the y-axis, the x-coordinate of the center must be 0.
Also, since the diameter of the circle is 12 units, the radius is half of that, or 6 units.
Using the standard form of the equation of a circle, which is (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center of the circle and r is the radius, we can substitute the values we know and simplify the equations to see which ones match.
The equations that represent circles with a diameter of 12 units and a center that lies on the y-axis are:
x^2 + (y - 6)^2 = 36 (center is at (0, 6))
x^2 + (y + 6)^2 = 36 (center is at (0, -6))
Therefore, the two options that represent the circles with the given properties are:
x^2 + (y - 6)^2 = 36 and x^2 + (y + 6)^2 = 36.
5. 3y(4 + 5x) *
(1 Point)
12y + 15x
12y + 15xy
4 + 15x
Answers
Answer:
The answer should be 12y+15xy
Step-by-step explanation:
Answer:
12y+15yx
Step-by-step explanation:
If the border number is 9 and the expression is 6(b-2)+7 how many tiles are there
Answers
Answer:
8(9).894 is the correct answer
The elephant's water tank holds 2,560 gallons of water. After two weeks, the zookeeper measures and finds that the tank only has 1,934 gallons of water left. If the elephant drinks the same amount of water each day, how many days will a full tank of water last?
Answers
Answer:
aprox 57 days
Step-by-step explanation:
2560-1934=626
626/14=44.7142857143
2560/44.7142857143=57.25 days
A veteran records whether her clients own a cat, a dog need neither or both. The results are shown in two ways.
Answers
50 Points! Multiple choice algebra question. Photo attached. Thank you!

Answers
Log22/Log9 express log_9 22 in terms of common logarithms
What is common logarithms?Common logarithms, is commonly describd as base-10 logarithms.
It is a type of logarithm that involve taking the logarithm of a number with respect to the base of 10.
This means that common logarithms show how many powers of ten must be increased to get a particular number. The usual logarithm of 100, for example, is 2, because 10 raised to the power of 2 equals 100.
The answer provided is based on the full question below;
-------------express log_9 22 in terms of common logarithms
a. Log 22/9
b. Log 198
c. Log22/Log9
d. Log9/Log22
Find more exercises on common logarithms;
https://brainly.com/question/30085872
#SPJ1
(b) The population consists of all 15-year-olds living in the attendance district of a local high school. You plan to obtain a simple random sample of 200 such residents by using the student roster of the high school as the sampling frame. (Select all that apply.)
Home-schooled students cannot be sampled.
Dropouts cannot be sampled.
Students who are on a school trip cannot be sampled.
Students who are out sick cannot be sampled.
Students who are skipping class cannot be sampled.
Answers
The people that would not be sampled would be:
Dropouts cannot be sampled.Home-schooled students cannot be sampled.How to determine the groups that cannot be sampled.We have to do this by considering the people that would be seen present in the roster of the school. The people that are dropouts as well as homeschooled cannot appear in a school register because they are not students.
The students that are sick and on the trip are all students of the high school so they can be included in the sampling that is being done by the school.
Read more on random sampling here:
https://brainly.com/question/17831271
#SPJ1
HELPPP ME PLSSSS
I NEED THIS FOR MY CLASS

Answers
Answer:
hii im looking at the same thing you just have to do the multiplication on that
Step-by-step explanation:
John put $3, 000 in his savings at 2% annually for 3 years. What did he earn in simple interest?
Answers
Answer:
End Balance: $5,160.00
Total Interest: $2,160.00
Step-by-step explanation:
Total Interest =$3000 × 2% × 3 × 12
=$2,160.00
End Balance =$3000 + $2,160.00
=$5,160.00
Answer:
can we get the options
Step-by-step explanation:
please graph! 90 points! And BRAINLIEST
Graph f(x)=3sin(12x)−5. Use 3.14 for π. Use the sine tool to graph the function. The first point must be on the midline and the second point must be a maximum or minimum value on the graph closest to the first point.
Answers
The points on the graph are:
Midline point = (0,-5)Maximum point = (0.65,-2)(a) The graph of the functionThe equation of the function is given as:
\(f(x) = 3\sin(12x) - 5\)
See attachment for the graph of the function f(x)
(b) The midline pointThis is the point that is on the line that passes through the midsegment of the function.
From the graph, a point on the midline is (0,-5)
(c) The maximum pointThis is the point that is on the vertex of the function.
From the graph, a point on the midline is (5pi/24,-2)
This can be rewritten as: (0.65,-2)
Read more about trigonometry graphs at:
https://brainly.com/question/8120556

Answer:
(0,0) (1.75,3)
Step-by-step explanation:
Took the test
A Company manufactures and sells a specialty watch. The financial research department, using statistical methods, determined that a price of $88 each, the demand would be 2 thousand watches, and at $38 each, 12 thousand watches. Assuming a linear relationship in the form p(q) = mq + b. (a) What would be the price at a demand of 8 thousand watches? (b) 15 thousand watches?
Answers
Given:
a.) A price of $88 each, the demand would be 2 thousand watches.
b.) At $38 each, 12 thousand watches.
Since it was mentioned that the relationship is linear, we will be generating the equation in slope-intercept form (y = mx + b).
Let,
x = q = number of watches
y = p(q) = price of the watches
x1, y1 = 2,000, 88
x2, y2 = 12,000, 38
We get,
Step 1: The slope (m).
\(\text{ m = }\frac{y_2-y_1}{x_2-x_1}\text{ = }\frac{\text{ 38 - 88}}{\text{ 12,000 - 2,000}}=\text{ }\frac{\text{ -50}}{\text{ 10000}}\text{ = -}\frac{\text{ 1}}{\text{ 200}}\)Step 2: Determine the y-intercept (b). Substitute x,y = 2,000, 88 and m = -1/200 in y = mx + b.
\(\begin{gathered} \text{ y = mx + b} \\ \text{ 88 = (-}\frac{\text{ 1}}{\text{ 200}})(\text{2000) + b} \\ \text{ 88 = -}\frac{\text{ 2000}}{\text{ 200}}\text{ + b} \\ \text{ 88 = -10 + b} \\ \text{ b = 88 + 10} \\ \text{ b = 98} \end{gathered}\)Step 3: Complete the equation. Substitute m = -1/200 and b = 78 in y = mx + b.
\(\begin{gathered} \text{ y = mx + b} \\ \text{ y = (-}\frac{\text{ 1}}{\text{ 200}})\text{x + (98)} \\ \text{ y = -}\frac{\text{ 1}}{\text{ 200}}\text{x + 98} \\ \text{ p(q) = -}\frac{\text{ 1}}{\text{ 200}}\text{q + 98} \end{gathered}\)Question a: What would be the price at the demand of 8 thousand watches?
\(\begin{gathered} \\ \text{p(q) = -}\frac{\text{ 1}}{\text{ 200}}\text{q + 98} \end{gathered}\)\(\text{ p(8000) = -}\frac{\text{ 1}}{\text{ 200}}(8,000)\text{ + 98}\)\(=\text{ - }\frac{\text{ 8,000}}{\text{ 200}}\text{ + 98}\)\(=\text{ -40 + 98}\)\(\text{ p(8,000) = 58 = \$58}\)Therefore, at the demand of 8 thousand watches, the price will be $58.
Question b:
\(\text{p(q) = -}\frac{\text{ 1}}{\text{ 200}}\text{q + 98}\)\(\text{ p(15000) = -}\frac{\text{ 1}}{\text{ 200}}(15,000)\text{ + 98}\)\(\text{ = -}\frac{15,000}{\text{ 200}}\text{ + 98}\)\(=\text{ }-75\text{ + 98}\)\(\text{ p(15,000) = }23\text{ = \$23}\)Therefore, at the demand of 15 thousand watches, the price will be $23.
Choose the equation that represents a line that passes through points (-6, 4) and (2.0).
Answers
make x the subject of

Answers
Answer:
x=2.5y+30Step-by-step explanation:
lets add 12 for a start
y+12=2/5x
x everything by 5
5y+60=2x
divide by 2
2.5y+30=x
hope it helps
5y+12=2x
x=5/2y+6
A survey of 85 families showed that 36 owned at least one DVD player. Find the 99% confidence interval estimate of the true proportion of families who own at least one DVD player. Place your limits, rounded to 3 decimal places, in the blanks. Place the lower limit in the first blank and the upper limit in the second blank
Answers
Answer:
\(0.424 - 2.58\sqrt{\frac{0.424(1-0.424)}{85}}=0.286\)
\(0.424 + 2.58\sqrt{\frac{0.424(1-0.424)}{85}}=0.562\)
The 99% confidence interval would be given by (0.286;0.562)
Step-by-step explanation:
Information given:
\( X= 36\) represent the families owned at least one DVD player
\( n= 85\) represent the total number of families
\(\hat p=\frac{36}{85}= 0.424\) represent the estimated proportion of families owned at least one DVD player
In order to find the critical value we need to take in count that we are finding the interval for a proportion, so on this case we need to use the z distribution. Since our interval is at 99% of confidence, our significance level would be given by \(\alpha=1-0.99=0.01\) and \(\alpha/2 =0.05\). And the critical value would be given by:
\(z_{\alpha/2}=-2.58, z_{1-\alpha/2}=2.58\)
The confidence interval for the mean is given by the following formula:
\(\hat p \pm z_{\alpha/2}\sqrt{\frac{\hat p (1-\hat p)}{n}}\)
If we replace the values obtained we got:
\(0.424 - 2.58\sqrt{\frac{0.424(1-0.424)}{85}}=0.286\)
\(0.424 + 2.58\sqrt{\frac{0.424(1-0.424)}{85}}=0.562\)
The 99% confidence interval would be given by (0.286;0.562)
sA bag contains 7 red marbles, 5 white marbles, and 7 blue marbles. You draw 3 marbles out at random, without replacement. Find the following probabilities and round to 4 decimal places. a. The probability that all the marbles are red is b. The probability that none of the marbles are red is
Answers
A) The probability that all the marbles are red is; P(3 red marbles) = 0.0361
B) The probability that none of the marbles are red is; 0.2270
How to find the probability of selection?The contents of the bag are;
Number of red marbles = 7
Number of White Marbles = 5
Number of Blue Marbles = 7
Total number of marbles = 7 + 5 + 7
= 159
A) When we draw 3 marbles without replacement, the probability that all the marbles are red is;
P(3 red marbles) = (7/19) * (6/18) * (5/17)
P(3 red marbles) = 0.0361
B) When we draw 3 marbles without replacement, the probability that none of the marbles are red is;
P(3 non-red marbles) = (12/19) * (11/18) * (10/17)
P(3 non-red marbles) = 0.2270
Read more about Probability of selection at; https://brainly.com/question/251701
#SPJ1
Solve for a side in right angles picture added

Answers
Because 90+70=160
Answer:
AC=5
in a basic right triangle the small side is always one shorter than the big side which is always one smaller than the hypotenuse.
Find the value of the test statistic for testing whether there is a linear relationship between the and the estimated number. Round your answer to two decimal places, if necessary.
Answers
the value of the test statistic for testing whether there is a linear relationship between the and the estimated number is 0.3506905
Using a correlation Coefficient calculator, the correlation Coefficient, r for the data = 0.127
The test statistic :
T = r² / √(1 - r²) / (n - 2)
Sample size, n = 10
Hence,
T = 0.127² / √(1 - 0.127²) / (10 - 2)
T = (0.016129 / 0.3506905)
T = 0.3506905
The value of the test statistic to 2 decimal places is 0.35
learn more about of statistic here
https://brainly.com/question/23382433
#SPJ4
Express the answers in simplest form. A list contains the names of six anthropology students, two sociology students, and four psychology professor's new study, find the probability that the chosen student (a) A psychology student (c) A psychology student or an anthropology (d) Not a sociology student.
Answers
a) P(psychology student) = 1/3. b) P(psychology or anthropology student) = 5/6. c) P(not sociology student)= 5/6
How to find the probability that the chosen student(a) The probability of choosing a psychology student is the number of psychology students divided by the total number of students:
P(psychology student) = 4/(6+2+4) = 4/12 = 1/3
(b) The probability of choosing a psychology student or an anthropology student is the sum of the number of psychology and anthropology students divided by the total number of students:
P(psychology or anthropology student) = (4+6)/(6+2+4) = 10/12 = 5/6
(c) The probability of not choosing a sociology student is the number of non-sociology students divided by the total number of students:
P(not sociology student) = (6+4)/(6+2+4) = 10/12 = 5/6
Learn more about probability at https://brainly.com/question/24756209
#SPJ1
Given an example of two terms that are like terms and two terms that are not like terms.
Answers
Answer:
example of two like terms:
5xy and 71xyexample of two unlike terms:
4b and 78a
Answer:
2x and 5x are like terms, 2x and 3y are not. 3 and 5 are like terms, 6y and 2 are not. etc.
Step-by-step explanation:
For something to be a like term, they either have to have the same variable (ex: x and y and any other variable that may be used) or both be whole numbers (1, 2, 3, etc.) No mixing the two.
Please only answer if you know the answer!!!

Answers
Answer:
y=3x
Step-by-step explanation:
y=mx+b, m is slope, b is y-intercept
The origin is (0,0)
Find the standard form of the equation of the circle with endpoints of a diameter at the points (7,8) and (-3,6). Type the standard form of the equation of this circle
Answers
Answer:
The equation of the circle is \((x - 2)^2 + (y - 7)^2 = 21\)
Step-by-step explanation:
Equation of a circle:
The equation of a circle, with center \((x_0,y_0)\) and radius r is given by:
\((x - x_0)^2 + (y - y_0)^2 = r^2\)
Distance between two points:
Suppose that we have two points, \((x_1,y_1)\) and \((x_2,y_2)\). The distance between them is given by:
\(D = \sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
Diameter at the points (7,8) and (-3,6).
The diameter is the distance between these two points, so:
\(D = \sqrt{(-3-7)^2+(6-8)^2} = \sqrt{104}\)
Radius is half the diameter, so:
\(r = \frac{\sqrt{104}}{2} = \frac{\sqrt{104}}{\sqrt{4}} = \sqrt{\frac{104}{4}} = \sqrt{21}\)
So
\(r^2 = (\sqrt{21})^2 = 21\)
Center:
Midpoint of the diameter, which is the mean of the coordinates. So
\(x_0 = \frac{7 - 3}{2} = \frac{4}{2} = 2\)
\(y_0 = \frac{8 + 6}{2} = \frac{14}{2} = 7\)
Then
\((x - 2)^2 + (y - 7)^2 = 21\)
Determine the domain of each relation, and determine whether each relation describes y as a function of b, x = b
Answers
The Y value can therefore be either positive or negative for every given X number, proving that this isn't a function.
Explain about the domain of the function?X is equal to why's absolute value, and we're looking for the domain. Remember that the domain is simply the set of all conceivable X values. As a result, when we look at the this equation, an absolute value sign just on y axis makes all negative numbers positive and all positive numbers, just that one number, positive.For the stated question:
Relation : x = b
Its absolute value of a negative is equal to the absolute amount of a positive alone.
Since zero's absolute value was only zero, all of the X values has to be positive or zero. Because if an exit value had a negative value, the absolute value sign could be in contravention of its rule.Therefore, the domain includes only positive numbers plus zero, and we can use any value for X to check whether or not it is a function. Let's assume that X is 20. 20 equals are available. the reason in its entirety Since we just observed that absolute value of the a negative number up above, y in this case may either equal only positive 20 and y can equal negative 20. This case's negative 20 is only 20, after all.Thus, the Y value can therefore be either positive or negative for every given X number, proving that this isn't a function.
To know more about the domain, here
https://brainly.com/question/2264373
#SPJ4
In the diagram below of triangle BCD, E is the midpoint of BD and F is the midpoint of CD. If EF = 43-4x, and BC = 32-2x, what is the measure of EF?

Answers
The measure of the length EF of the triangle is; 21
How to solve similar triangles?We are given the the triangle BCD and we are told that;
E is the midpoint of BD.
F is the midpoint of CD
Now, since we have those midpoints, it means that by the concept of similar triangles, that;
DF/DC = EF/BC
Since F is the midpoint of DC, then it means that;
2DF = DC
We are given;
EF = 43 - 4x, and BC = 32 - 2x
Thus, we now have;
DF/(2DF) = (43 - 4x)/(32 - 2x)
1/2 = (43 - 4x)/(32 - 2x)
Cross multiply to get;
32 - 2x = 43 - 4x
4x - 2x = 43 - 32
2x = 11
x = 11/2
x = 5.5
Thus;
EF = 43 - 4(5.5)
EF = 43 - 22
EF = 21
Thus, we conclude that the side length EF is 21.
Read more about similar triangles at; https://brainly.com/question/14285697
#SPJ1
HELP HELP HELPPPP OMG

Answers
Answer:
hope it helps you.............!

Please help me this is due in 10 minutes.

Answers
Answer:
1.07
Step-by-step explanation:
3.21/3=1.07
5.35/5=1.07
8.56/8=1.07
Find the area of the shape shown below.
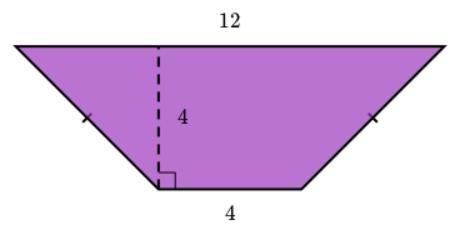
Answers
Answer:
The answer is 32
Step-by-step explanation:
The formula for finding the area of a trapezoid is:
((base 1 + base 2)/ 2 )* h
Now all you have to do is substitute the numbers in.
Note: bases will always be the ones like 12 and 4 in this case. We have just named then 1 and 2.
Answer:
32 square units
Step-by-step explanation:
\(\displaystyle A=\frac{1}{2}(b_1+b_2)h=\frac{1}{2}(12+4)(4)=\frac{1}{2}(16)(4)=\frac{1}{2}(64)=32\)
Note that \(b_1\) and \(b_2\) are the lengths of each base of the trapezoid, so it doesn't matter which is which.
employees at an arcade are paid according to the number of hours worked as shown in the graph

Answers
Answer:
B, C, G
Step-by-step explanation:
If we look at the graph, it shows that if an employee works for 5 hours, then they will earn $36.25.
We can take 36.25 and divide that by 5 to get the hourly wage.
36.25 ÷ 5 = 7.25
We now know that employees get $7.25 every hour.
Using this we can look back to the graph.
'A' says that if an employee does not work, they will earn $7.25.
We know that this is wrong because if you do not work, then you do not earn money.
Let's look at 'B'.
If employees work for one hour, they will earn $7.25
We know this is correct because we now know the hourly wage.
Let's look at 'C'
If employees work for 4 hours, then they will get a revenue of $29.
We can figure this out by using this equation.
number of hours × hourly wage = total payment
4 × 7.25 = 29
Then this means that this is correct.
Let's look at 'D'
It says that if employees work for 10 hours, they will earn $73
Let's use that same equation again.
10 × 7.25 = 72.5
Employees earn $72.5 for working 10 hours, not $73.
So, this is obviously incorrect.
Let's look at 'E'
It says that if employees work for 3.5 hours, they will get a revenue of $21.75.
3.5 × 7.25 = 25.375
Therefore, this is incorrect.
Now let's take a look at 'F'.
It says that if employees work for 7.25 hours, then they earn $1.
This is incorrect.
And lastly, 'G'.
We know that if you do not work, then you do not earn money.
Therefore, A, B and G are the correct answers.
Find the complete factored form of the
polynomial :
-8m²n-7m² nª
Enter the correct answer.
Answers
The polynomial -8m²n - 7m²n can be factored using the common factor -m²n. The complete factored form of the polynomial is (-m²n) (8 + 7a).
To find the complete factored form of the polynomial -8m²n - 7m²n, we can factor out common terms from both the terms. The common factor in the terms -8m²n and -7m²n is -m²n. We can write the polynomial as:
-8m²n - 7m²n = (-m²n) (8 + 7a)
Therefore, the complete factored form of the polynomial -8m²n - 7m²n is (-m²n) (8 + 7a). This expression represents the original polynomial in a multiplied form. We can expand this expression using distributive law to verify that it is equivalent to the original polynomial.
For more such questions on polynomial, click on:
https://brainly.com/question/1600696
#SPJ8
© Dan invests £1500 into his bank account.
He receives 3% per year simple interest.
How much will Dan have after 2 years?
Give your answer to the nearest penny where appropriate.
Answers
Answer:
\(i = p \times r \times t \div 100\)
1500×3×2÷100=90
Answer:
£1590
Step-by-step explanation:
Simple interest = PTR/100
= £1500x2x3/100
= 15x2x3 = 90
£90 is simple interest
So amount = Principcal + Interest
Amount = 1500 + 90
= £1590