Help PLEASE!
Two distinct number cubes are rolled together. Each number cube has sides numbered 1 through 6.
What is the probability that the outcome of the roll is an even sum or a sum that is a multiple of 3?
Enter your answer, in simplest fraction form, in the box.
Answers
Answer:
The probability that the outcome of the roll is an even sum or a sum that is a multiple of 3
P( E∪F) \(= \frac{24}{36}\)
Step-by-step explanation:
Given Each number cube has sides numbered 1 through 6
Two distinct number cubes are rolled together
Total number of exhaustive cases n(S) = 36
Let 'E' be the event of getting sum is even
n(E) = {(1,3),(1,5), (2,2),(2,4),(2,6),(3,1),(3,3),(3,5),(4,2),(4,4), (4,6),(5,1),(5,3),(5,5),(6,2),(6,4),(6,6)} = 17
\(P(E) = \frac{n(E)}{n(S)} = \frac{17}{36}\)
Let 'F' be the event of getting sum that is a multiple of '3'
n(F) = {(1,2),(1,5),(2,1),(2,4),(3,3),(3,6),(4,2),(4,5),(5,1),(5,4),(6,3),(6,6) = 12
\(P(F) = \frac{n(E)}{n(S)} = \frac{12}{36}\)
n((E∩F) = {(2,4),(3,3),((4,2),(5,1),(6,6)} =5
\(P(En F) = \frac{n(En F)}{n(S)} = \frac{5}{36}\)
The probability that the outcome of the roll is an even sum or a sum that is a multiple of 3
P( E∪F) = P(E) + P(F) - P( E∩F)
\(\frac{17}{36} +\frac{12}{36} - \frac{5}{36} = \frac{24}{36}\)
Related Questions
Which item on the diagram below of a circle, with center
0, represents a radius of the circle?
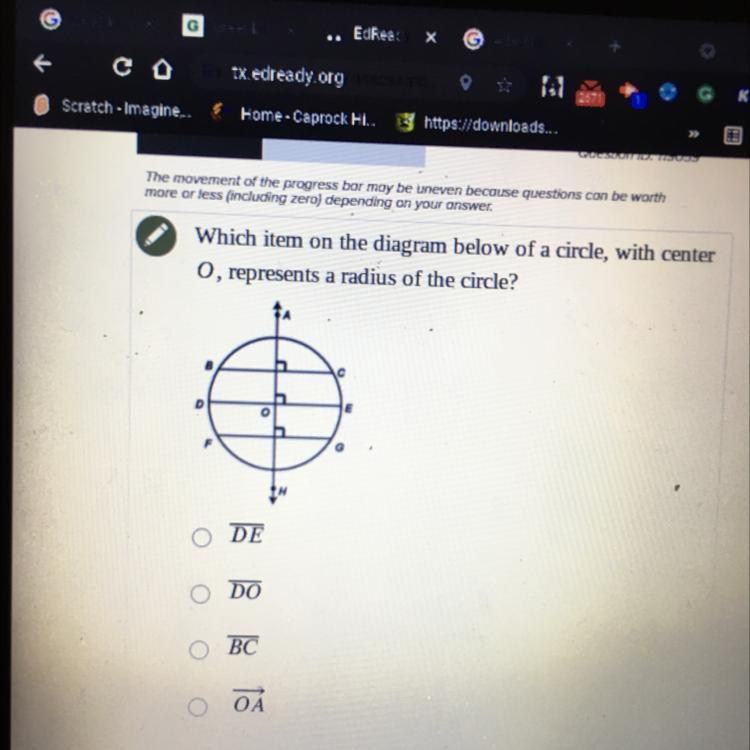
Answers
Answer:
The answer is DE
Step-by-step explanation:
I learned about it in school.
The radius of a circle with center O is DO. Therefore, option B is the correct answer.
Given that, a circle, with center O, represents a radius of the circle.
What is the circle?A circle is a two-dimensional figure formed by a set of points that are at a constant or at a fixed distance (radius) from a fixed point (center) on the plane. The fixed point is called the origin or center of the circle and the fixed distance of the points from the origin is called the radius.
Radius of a circle is the distance from the center of the circle to any point on it's circumference.
Here, DO and OC is the radius of a circle with center O.
The radius of a circle with center O is DO. Therefore, option B is the correct answer.
To learn more about the circle visit:
https://brainly.com/question/11833983.
#SPJ2
Kristy bought a new tv the total cost of the tv was £360 including vat at 20% work out the cost of the tv before the vat was added
Answers
Answer:
£288
Step-by-step explanation:
Kathy bought a TV for the cost of 360 pounds, including VAT (taxes).
Since the taxes are 20% of the TV's value, we can calculate 20% of 360.
20% of 360 = 72
360 - 72 = 288
Therefore, the cost of the TV before taxes was £288.
what is f(x)/g(x) when f(x) = 6x3 – 19x2 + 16x – 4 and g(x) = x - 2? 6x^2 - 7x + 2 3x² - 9x + 8 6x2 – 7x + 2 - 8/(x - 2) 3x2 - 9x + 8 - 8/(x - 2)
Answers
Problem
what is f(x)/g(x) when f(x) = 6x3 – 19x2 + 16x – 4 and g(x) = x - 2?
Solution
We can use the long division and we have:
\(\frac{6x^3-19x^2+16x-4}{x-2}=6x^2+\frac{-7x^2+16x-4}{x-2}\)We can simplify the last expression on this way:
\(6x^2+\frac{-7x^2+16x-2}{x-2}=6x^2-7x+\frac{2x-4}{x-2}=6x^2-7x+2\)And the best solution for this case would be:
6x^2 - 7x + 2
if you randomly select a mechanical component, what is the probability that it weighs more than 11.5lbf
Answers
The probability that randomly selected mechanical component weighs: more than 11.5 lbf is 0.0099, less than 8.7 lbf is 0.3208, less than 5.0 lbf is 0.0228, between 6.2 lbf and 7.0 lbf is 0.1314, between 10.3 lbf and 14.0 lbf is 0.0436, between 6.8 lbf and 8.9 lbf is 0.3464.
We can use the standard normal distribution and z-scores to answer these questions:
P(X > 11.5) = P(Z > (11.5 - 8) / 1.5) = P(Z > 2.33) = 0.0099
Therefore, the probability that a randomly selected mechanical component weighs more than 11.5 lbf is 0.0099, or about 1%.
P(X < 8.7) = P(Z < (8.7 - 8) / 1.5) = P(Z < 0.47) = 0.3208
Therefore, the probability that a randomly selected mechanical component weighs less than 8.7 lbf is 0.3208, or about 32%.
P(X < 5.0) = P(Z < (5 - 8) / 1.5) = P(Z < -2) = 0.0228
Therefore, the probability that a randomly selected mechanical component weighs less than 5.0 lbf is 0.0228, or about 2%.
P(6.2 < X < 7.0) = P((6.2 - 8) / 1.5 < Z < (7 - 8) / 1.5) = P(-1.2 < Z < -0.67) = 0.1314
Therefore, the probability that a randomly selected mechanical component weighs between 6.2 lbf and 7.0 lbf is 0.1314, or about 13%.
P(10.3 < X < 14.0) = P((10.3 - 8) / 1.5 < Z < (14 - 8) / 1.5) = P(1.53 < Z < 2.67) = 0.0436
Therefore, the probability that a randomly selected mechanical component weighs between 10.3 lbf and 14.0 lbf is 0.0436, or about 4%.
P(6.8 < X < 8.9) = P((6.8 - 8) / 1.5 < Z < (8.9 - 8) / 1.5) = P(-0.47 < Z < 0.60) = 0.3464
Therefore, the probability that a randomly selected mechanical component weighs between 6.8 lbf and 8.9 lbf is 0.3464, or about 35%.
To know more about probability :
https://brainly.com/question/15016913
#SPJ4
____The given question is incomplete, the complete question is given below:
Suppose that the weight of a mechanical component is normally distributed with mean p = 8.0 Ibf and standard deviation = 1.5 lbf. Answer the following questions: 1. If you randomly select a mechanical component, what is the probability that it weighs more than 11.5 lbf? 2. If you randomly select a mechanical component, what is the probability that it weighs less than 8.7 lbf? 3. If you randomly select a mechanical component, what is the probability that it weighs less than 5.0 lbf? 5. If you randomly select a mechanical component, what is the probability that it weighs between 6.2 lbf and 7.0 lbf? 6. If you randomly select a mechanical component, what is the probability that it weighs between 10.3 lbf and 14.0 lbf? 7. If you randomly select a mechanical component, what is the probability that it weighs between 6.8 lbf and 8.9 lbf?
If f(x)={
−2x+6
9x−3
for x≤0
for x>0
, find the following values. 1. f(−4)=
Answers
f(-4) is equal to 14.
To find the value of f(-4), we need to evaluate the function f(x) at x = -4.
Since x ≤ 0, we use the first part of the function, which is f(x) = -2x + 6, when x is less than or equal to 0.
Substituting x = -4 into the first part of the function, we have:
f(-4) = -2(-4) + 6
Simplifying the expression, we get:
f(-4) = 8 + 6
f(-4) = 14.
Learn more about equal here :-
https://brainly.com/question/33293967
#SPJ11
Use the given graph to determine the limit, if it exists. (4 points)
A coordinate graph is shown with an upward sloped line crossing the y axis at the origin that ends at the open point 3, 1, a closed point at 3, 7, and a horizontal line starting at the open point 3, 3.
Find limit as x approaches three from the right of f of x. .
Answers
Explanation:
Refer to the graph below. It should be similar to what your teacher gave you, based off the description.
Since we're approaching 3 from the right side, this means we'll be working with the horizontal line portion. We could start at something like x = 3.2 and move closer to 3 by getting to x = 3.1 then x = 3.01 then x = 3.001 and so on. We never actually get to 3 itself.
As x gets closer to 3 from this direction, the y values are approaching 3 since every point on this horizontal line has the same y coordinate. Technically the y value is already at 3, but it's the same idea.
In terms of notation, we can write \(\displaystyle \lim_{x\to3^{+}}f(x) = 3\)
The portion \(x \to 3^{+}\) means we're approaching 3 from the positive side, aka the right hand side on the number line.

7. At Winco, Faith can buy 3 candy bars for $0.99.
At Food for Less, she can buy 4 candy bars for
$1.25. Which store has the better buy? Explain why.
Answers
Answer:
Step-by-step explanation:
If Winco buys 3 candies for $0.99 and each candy costs $0.33
If he buys 4 candies at $1.25 then each candy costs $0.31
Therefore the latter is a better choice.
The square root of 83 can be plotted on the nurnber line below. 8.5 8.6 8.7 8.7 8.8 8.9 9.0 9.1 9.2 9.3 9.4 9.5 Use the drop-down menus to explain where ✓83 should be plotted on the number line. Click the arrows to choose an answer for each menu. Choose... The square root of 83 to the nearest tenth is . The square root of 83 to the nearest hundredth is choose..! On the number line above, ✓83 should be plotted Choose.. of Choose... slightly to the

Answers
Answer: the square root 83 to the nearest tenths is: 9.1. The square root of 83 to the nearest hundredth is: 9.11. On the number line above, 83^square root should be plotted slightly to the: right of: 9.1.
Step-by-step explanation:
Hope this help!
The required fill-in-the-blanks are "9.1, 9.11, right, and 9.1" which represents below in the solution part.
What is Number Line?In math, a number line can be defined as a straight line with numbers arranged at equal segments or intervals throughout. A number line is typically shown horizontally and can be extended indefinitely in any direction.
The square root of 83 can be plotted on the number line below.
As per the given question, the required solution would be as:
The square root of 83 to the nearest tenths is "9.1". The square root of 83 to the nearest hundredth is "9.11". On the number line above, √83 should be plotted slightly to the "right" of "9.1".
Learn more about the number line here:
brainly.com/question/17617832
#SPJ5

if bill has an apple, an orange, a pear, a grapefruit, a banana, and a kiwi at home and he wants to bring three pieces of fruit to school, how many combinations of fruit can he bring?
Answers
There are 20 different combinations of fruit that Bill can bring to school.
The number of combinations of fruit that Bill can bring to school can be determined using the concept of combinations.
In this case, Bill has 6 different types of fruit at home: apple, orange, pear, grapefruit, banana, and kiwi. He wants to bring 3 pieces of fruit to school.
To find the number of combinations, we can use the formula for combinations, which is given by:
C(n, r) = n! / (r!(n-r)!)
Where n is the total number of items and r is the number of items chosen.
In this case, n = 6 (total number of fruits) and r = 3 (number of fruits Bill wants to bring to school).
Plugging in the values, we get:
C(6, 3) = 6! / (3!(6-3)!)
Simplifying this equation, we get:
C(6, 3) = (6 x 5 x 4) / (3 x 2 x 1)
C(6, 3) = 20
Therefore, there are 20 different combinations of fruit that Bill can bring to school.
Here are some examples of possible combinations:
1. Apple, orange, pear
2. Apple, orange, grapefruit
3. Apple, orange, banana
4. Apple, orange, kiwi
5. Apple, pear, grapefruit
6. Apple, pear, banana
7. Apple, pear, kiwi
8. Apple, grapefruit, banana
9. Apple, grapefruit, kiwi
10. Apple, banana, kiwi
And so on, for a total of 20 combinations.
To know more about combinations refer here:
https://brainly.com/question/31586670
#SPJ11
4,264 rounded to the nearest hundred
Answers
Answer:
4,300
Step-by-step explanation:
Another learning aid provides a step-by-step walk-through showing the solution process for a problem similar to the one you are working on. You would click Continue to advance through each step in the solution process to show intermediate calculations at key points. While you are attempting to solve the given exercise, this learning aid can be referenced multiple times and does not load a new version of the exercise when it is accessed. Choose the described learning aid below.
Answers
Choose the described learning aid below.
a. Animation
b. view an example
c. video
d. help me solve this
e. Textbook
The answer to this question is option b. View an example helps to give a step by step walk through showing the solution to a similar problem.
The learning aid that is referenced in this question is view an example because it is a learning aid that helps the learner to solve a problem just by looking at the solution of another problem.
The solution that is being viewed has to be quite similar with the problem. Such that the learner would be able to follow through with finding a solution to his problem by following the steps in the example.
here is a similar question https://brainly.com/question/24454973?referrer=searchResults
I don’t understand this equation please help me

Answers
Answer:
Evaluated, you get 4x^4
Step-by-step explanation:
Differentiated, you get 16x^3
It all depends on what you're looking for, really
let be a subset of such that no pair of distinct elements in has a sum divisible by . what is the maximum number of elements in ?
Answers
The maximum number of elements in the subset is 5. No two distinct elements in the subset have a sum divisible by 5, as the sum of any two elements with distinct remainders is either a multiple of 5 or has a remainder of 1, 2, 3, or 4 when divided by 5.
To maximize the number of elements in the subset, we need to choose elements that have the most possible distinct remainders when divided by 5. Since no pair of distinct elements in the subset has a sum divisible by 5, the remainders of any two distinct elements in the subset must add up to a non-multiple of 5.
The remainders when dividing the first 5 positive integers by 5 are 1, 2, 3, 4, and 0, respectively. Therefore, the maximum number of elements we can choose from the set such that no pair has a sum divisible by 5 is 5.
We can achieve this maximum by selecting one element from each of the following subsets of the original set:
Elements with a remainder of 1 when divided by 5
Elements with a remainder of 2 when divided by 5
Elements with a remainder of 3 when divided by 5
Elements with a remainder of 4 when divided by 5
The element that is a multiple of 5.
Learn more about subsets here: brainly.com/question/31739353
#SPJ11
if sample variances are significantly different, we cannot conduct t-test on those data
T/F
Answers
False. The statement is not entirely accurate. While significantly different sample variances can impact the assumptions of a t-test, it does not necessarily mean that a t-test cannot be conducted.
In traditional t-tests, there is an assumption of equal variances between the compared groups or populations. This assumption is known as the assumption of homogeneity of variances. If the sample variances are significantly different, it violates this assumption.
However, there are alternative versions of the t-test that can be used when the variances are not equal, such as the Welch's t-test or the modified t-test. These tests do not assume equal variances and can be applied when the variances are significantly different.
Therefore, it is possible to conduct a t-test even when the sample variances are significantly different, using appropriate modifications to the test. However, it is essential to consider the validity and appropriateness of the chosen test based on the specific circumstances and assumptions of the data.
Learn more about t-test here:
https://brainly.com/question/30818311
#SPJ11
Question 1
A sweater was originally $38.00, but the department
store is running a 15% off sale. What is the sale price of
the sweater?
Answers
Answer:
$32
Step-by-step explanation:
Find the distance between the two numbers on a number line . -2.2,8.4
Answers
Answer:
10.6
Step-by-step explanation:
To find the difference between 2 numbers, subtract the bigger one from the smaller one. In this case, 8.4-(-2.2) = 8.4 + 2.2 = 10.6
Suppose A Monument in Texas casts a shadow of 285 feet. At the same time, a nearby tourist, who is 5 feet tall casts a 2.5 foot shadow. How tall is the Monument?
Answers
The height of the monument is 570 feet. Since the shadow of the monument and the shadow of the tourist are cast at the same time, their angles of elevation are the same.
To solve this problem, we need to use the concept of similar triangles. We can set up a proportion: (height of Monument) / (length of Monument's shadow) = (height of tourist) / (length of tourist's shadow)
Let x be the height of the Monument. Then we have:
x / 285 = 5 / 2.5
Cross-multiplying, we get:
2.5x = 5 * 285
Simplifying, we get:
x = 570
Therefore, the Monument is 570 feet tall.
To find the height of the monument, we can use the concept of similar triangles. Since the shadow of the monument and the shadow of the tourist are cast at the same time, their angles of elevation are the same.
Set up a proportion using the height and shadow length of the tourist and the monument:
(height of monument) / (shadow of monument) = (height of tourist) / (shadow of tourist)
Let x represent the height of the monument. Then:
x / 285 = 5 / 2.5
Now, solve for x:
x = (5 / 2.5) * 285
x = 2 * 285
x = 570 feet
The height of the monument is 570 feet.
Visit here to learn more about angles : https://brainly.com/question/28451077
#SPJ11
PLS HELP I NEED HELP
What is the area of this shape?

Answers
the amplitude of a sector of πcm² of area and 1.5 cm of radius
Answers
The area of the sector is A = π cm² and amplitude is a = 0.75 cm
Given data ,
The area of a sector is given by the formula:
A = (1/2) x r² x θ
where r is the radius of the sector and θ is the central angle in radians.
On simplifying , we get
A = π cm²
r = 1.5 cm
Substituting these values into the formula, we get:
π = (1/2) x (1.5)² x θ
π = (3/4) x θ
Solving for θ, we get:
θ = (4/3) x π
The amplitude of the sector is half of the radius, so:
amplitude = (1/2) x 1.5 cm = 0.75 cm
Hence , the amplitude of the sector is 0.75 cm
To learn more about area of sector click :
https://brainly.com/question/28180776
#SPJ1
Find the linear function with the following properties.f( -4)= 7f( -8)= - 2answer : f (x) = ?
Answers
First, we find the slope
\(m=\frac{y_2-y_1}{x_2-x_1}\)Where,
\(\begin{gathered} x_1=-4 \\ x_2=-8 \\ y_1=7 \\ y_2=-2 \end{gathered}\)\(m=\frac{-2-7}{-8-(-4)}=\frac{-9}{-8+4}=-\frac{9}{-4}=\frac{9}{4}\)Then, we use the point-slope formula to find the equation
\(\begin{gathered} y-y_1=m(x-x_1) \\ y-7=\frac{9}{4}(x-(-4)) \\ y-7=\frac{9}{4}x+9 \\ y=\frac{9}{4}x+9+7 \\ y=\frac{9}{4}x+16 \end{gathered}\)Hence, the function is
\(f(x)=\frac{9}{4}x+16\)Answer:
its one-half
Step-by-step explanation:
tested

At LaGuardia Airport for a certain nightly flight, the probability that it will rain is 0.15 and the probability that the flight will be delayed is 0.11. The probability that it will not rain and the flight will leave on time is 0.75. What is the probability that the flight would be delayed when it is raining? Round your answer to the nearest thousandth.
Answers
If At LaGuardia Airport for a certain nightly flight. The probability that the flight would be delayed when it is raining is: 0.140.
What is the probability?First step is to find the P(rain and on time)
P(rain and on time) = 1 - P(not rain and on time)
P(rain and on time) = 1 - 0.75
P(rain and on time)= 0.25
Now we can calculate P(delay and rain):
P(delay and rain) = P(delay | rain) * P(rain)
= P(rain and on time) - P(not rain and on time)
= 0.25 - 0.11
= 0.14
Therefore the probability that the flight would be delayed is 0.140 .
Learn more about probability here:https://brainly.com/question/13604758
#SPJ1
Suppose that f'(x)=2x for all x
A.)Find f(-5) if f(0)=0
B.)Find f(-5) if f(1)=0
C.) Find f(-5) if f(-4)=17
Answers
The final value is:
f(-5) = (-5)^2 = 25.
f(-5) = (-5)^2 - 1 = 24.
f(-5) = (-5)^2 + 1 = 26.
We can solve this problem by using the fact that the derivative of a function gives us the slope of the tangent line at any point. We can then use this information to find the equation of the function that satisfies the given derivative and the additional information about the function's values at certain points.
A) To find f(-5) if f(0)=0, we need to integrate the derivative f'(x)=2x with respect to x, since integration is the inverse operation of differentiation.
∫f'(x)dx = ∫2xdx = x^2 + C
where C is the constant of integration.
Since f(0)=0, we can find C by substituting x=0:
f(0) = 0^2 + C = 0
C = 0
Thus, the equation of the function is:
f(x) = x^2
So, f(-5) = (-5)^2 = 25.
B) To find f(-5) if f(1)=0, we can use the same method:
∫f'(x)dx = ∫2xdx = x^2 + C
Since f(1)=0, we can find C by substituting x=1:
f(1) = 1^2 + C = 0
C = -1
Thus, the equation of the function is:
f(x) = x^2 - 1
So, f(-5) = (-5)^2 - 1 = 24.
C) To find f(-5) if f(-4)=17, we can again use the same method:
∫f'(x)dx = ∫2xdx = x^2 + C
Since f(-4)=17, we can find C by substituting x=-4:
f(-4) = (-4)^2 + C = 17
C = 1
Thus, the equation of the function is:
f(x) = x^2 + 1
So, f(-5) = (-5)^2 + 1 = 26.
To learn more about derivative visit:https://brainly.com/question/30365299
#SPJ11
3+1/2(5-x)<-5
the solution is______
Answers
Answer:
\(x>21\)
Step-by-step explanation:
\(3+1/2(5-x)<-5\\\\3 + \frac{1}{2} (5-x)< -5\\\\\mathrm{Subtract\:}3\mathrm{\:from\:both\:sides}\\3+\frac{1}{2}\left(5-x\right)-3<-5-3\\\\Simplify\\\frac{1}{2}\left(5-x\right)<-8\\\\\mathrm{Multiply\:both\:sides\:by\:}2\\2\cdot \frac{1}{2}\left(5-x\right)<2\left(-8\right)\\\\Simplify\\5-x<-16\\\\\mathrm{Subtract\:}5\mathrm{\:from\:both\:sides}\\5-x-5<-16-5\\\\Simplify\\-x<-21\\\\Multiply\:both\:sides\:by\:-1\:\left(reverse\:the\:inequality\right)\\\)
\(\left(-x\right)\left(-1\right)>\left(-21\right)\left(-1\right)\\\\Simplify\\x>21\)
Answer:
x > 21
I hope this helps!
45°
9
X
Find the value of x. Write your answer in simplest form.

Answers
9514 1404 393
Answer:
x = (9/2)√2 = 4.5√2
Step-by-step explanation:
We know the hypotenuse of an isosceles right triangle is √2 times the length of the side:
9 = x·√2
9√2 = 2x . . . . multiply by √2
(9/2)√2 = x . . . divide by 2
NEED HELP ASAP - Algebra 2
Choose three graphs and identify the x - and y - intercepts. State whether the graph has symmetry.

Answers
For graph A: The x intercept is -1.5 or 2 and the y intercept is 12
For graph B : The x intercept is -3,-2 and 1 and the y intercept is -6
For graph C : The x intercept is -3 and 4 and y intercept is 90.
Graph A and B are symmetric graphs.
What are symmetric graphs?A graph is symmetric with respect to a line if reflecting the graph over that line leaves the graph unchanged. This line is called an axis of symmetry of the graph.
A symmetric graph is a graph that is both edge- and vertex-transitive.
In other words, Graph A and B are symmetric graphs because the group of its auto morphisms has degree greater than 1.
learn more about symmetric graph from
https://brainly.com/question/30085550
#SPJ1
Plsss I need help ASAP

Answers
Answer:
The first is True.
The second is False.
The third is True.
The fourth is True.
The Fifth is False.
Answer:
true, true, false
Step-by-step explanation:
Lucy has $7 less than Kristine and $5 more than Nina together,the three have $35 how much does Lucy have?
Answers
Lucy has $7 less than Kristine and $5 more than Nina together, the three have $35. Lucy has $11.
Let's denote the amount of money that Kristine has as K, the amount of money that Lucy has as L, and the amount of money that Nina has as N.
According to the given information, we can form two equations:
Lucy has $7 less than Kristine: L = K - 7
Lucy has $5 more than Nina: L = N + 5
We also know that the three of them have a total of $35: K + L + N = 35
We can solve this system of equations to find the values of K, L, and N.
Substituting equation 1 into equation 3, we get:
K + (K - 7) + N = 35
2K - 7 + N = 35
Substituting equation 2 into the above equation, we get:
2K - 7 + (L - 5) = 35
2K + L - 12 = 35
Since Lucy has $7 less than Kristine (equation 1), we can substitute K - 7 for L in the above equation:
2K + (K - 7) - 12 = 35
3K - 19 = 35
Adding 19 to both sides:
3K = 54
Dividing both sides by 3:
K = 18
Now we can substitute the value of K into equation 1 to find L:
L = K - 7
L = 18 - 7
L = 11
Finally, we can find the value of N by substituting the values of K and L into equation 3:
K + L + N = 35
18 + 11 + N = 35
N = 35 - 18 - 11
N = 6
Therefore, Lucy has $11.
Learn more about Kristine from
https://brainly.com/question/27244350
#SPJ11
specify the bayesian form of the simple linear regression model. what does the joint likelihood depend on for this model?
Answers
1. The Bayesian form of the simple linear regression model is: y = β0 + β1 * x + ε.
where y is the dependent variable, x is the independent variable, β0 is the intercept, β1 is the slope, and ε is the error term.
2. The joint likelihood depends on the likelihood function, the prior distributions, and the data.
The Bayesian form of the simple linear regression model and explain what the joint likelihood depends on for this model.
The Bayesian form of the simple linear regression model involves updating our beliefs about the parameters of the model (slope and intercept) based on the observed data.
The model can be represented as:
y = β0 + β1 * x + ε
Where y is the dependent variable, β0 is the intercept, β1 is the slope, x is the independent variable, and ε is the error term.
In the Bayesian approach, we assign prior distributions to the model parameters, β0 and β1, and update these priors using the observed data to obtain posterior distributions.
The joint likelihood for the Bayesian simple linear regression model depends on three components:
The likelihood function:
This represents the probability of observing the data given the model parameters.
For a simple linear regression model, the likelihood function is typically based on the assumption that the errors (ε) follow a normal distribution with mean 0 and variance \(\sigma^2.\)
The prior distributions:
These represent our beliefs about the model parameters (β0 and β1) before observing the data.
Common choices for priors include normal or uniform distributions.
The data:
The observed values of the dependent variable (y) and the independent variable (x) are used to update the prior distributions and obtain the posterior distributions for the model parameters.
The Bayesian form of the simple linear regression model involves updating our beliefs about the model parameters (intercept and slope) using prior distributions and observed data.
For similar question on linear.
https://brainly.com/question/30621842
#SPJ11
1. Jada earns $7 per hour mowing her neighbors' lawns.
Write an
equation.
Answers
Answer:
y=7x
Step-by-step explanation:
for a period of time, an island's population growth exponential. if the population doubles every 17 years and the current population is 1,725, what will the population be 10 years from now?
Answers
Using the exponential growth formulas, we get the population at 10 years would be 2593.
What is exponential growth?
A process called exponential growth sees a rise in quantity over time. It happens when the derivative of a quantity's instantaneous rate of change with respect to time is equal to the original quantity.
Given Initial population is Pt= 1725.
The doubling time of the population is D=17
Now we need to find the population at time t=10
Using the formula,
\(P_{t}=P_{0} (2)^{\frac{t}{D} } \\P_{t}=1725 (2)^{\frac{10}{17} } \\P_{t}=1725 (2)^{0.588}\\P_{t}=1725 \space\ . \space\ 1.503\\P_{t}=2593\)
Hence the population of the island in 10 years would be 2593.
To learn more about exponential growth, visit the link below:
https://brainly.com/question/12490064
#SPJ4