Answers
Answer:
73/39
Step-by-step explanation:
The slope of the given line is the coefficient of x in the given equation, which we recognize as being in slope-intercept form.
The slope of the perpendicular line will be the opposite reciprocal of this:
-1/(-39/73) = 73/39 . . . . slope of the perpendicular line
Related Questions
Simplify:
2
(
3
x
)
+
(
x
+
10
)
2(3x)+(x+10)
Answers
Answer:
7x+10
Step-by-step explanation:
2x(3x)+1x(x+10)
6x+1x+10
7x+10
Is {(0,6),(0,-6),(1,5),(1,-5),(7,10)} a function?
Please help
Answers
Answer: Yes, it is a function
Step-by-step explanation:
Trigonometry: Measure tal Excel In Opt. Mathematics - Book 9 ) If the number of degrees of a certain angle added to the number of gra same angle is 152, find the angle in degrees.
Answers
The angle in degrees is 873.1843.
Let the measure of the angle be θ in degrees. Therefore, the measure of the same angle in gradians is (θ × π/180).
According to the given information, the number of degrees of a certain angle added to the number of gradians of the same angle is 152.(θ) + (θ × π/180) = 152.
Simplifying the above equation, we get:(θ) + (θ/180 × π) = 152.
Multiplying both sides of the equation by 180/π, we get:
θ + θ = (152 × 180)/π2θ = (152 × 180)/πθ = (152 × 180)/(3.14)θ = 873.1843
Thus, the angle in degrees is 873.1843.
For more such questions on angle, click on:
https://brainly.com/question/25770607
#SPJ8
In a recent survey of 36 people, 18 said that their favorite color of car was blue.
What percent of the people surveyed liked blue cars? Explain your answer with every step you took to get to it.
Answers
Answer: The percentage of people surveyed who liked blue cars is 50%.
Step-by-step explanation:
Total number of people partaking in survey= 36
number of people who like blue cars= 18
therefore, fraction of people who liked blue cars= \(\frac{18}{36}\)
hence, percentage of people who liked blue cars= (18/36)*100 %
= 50%
To learn more about percentages visit the link below:
https://brainly.com/question/30399396
Answer:
Percentage of people who like blue-coloured cars is 50%
Number of people who were surveyed=36
Number of people who like blue-colored cars=18
Therefore, Percentage of people who like blue cars= (Number of people who like blue cars/ Number of people who were surveyed)*100
=(18/36)*100
=50%
Read more about percentage
https://brainly.in/question/21062337#:~:text=Answer%3A,has%20no%20unit%20of%20measurement.
fraction bears the same ratio to 1/27 as 3/7 does to 5/9.then the fraction is?
Answers
The fraction that bears the same ratio to 1/27 as 3/7 does to 5/9 is 27/35.
To find the fraction that bears the same ratio to 1/27 as 3/7 does to 5/9, we can set up a proportion.
Let's represent the unknown fraction as x. The ratio can be configured as follows:
x / (1/27) = (3/7) / (5/9)
To solve this proportion, we can cross-multiply:
(x * 5/9) = (3/7) * (1/27)
Simplifying the right side:
(x * 5/9) = 3/189
To eliminate the fraction on the left side, we can multiply both sides by the reciprocal of 5/9, which is 9/5:
(x * 5/9) * (9/5) = (3/189) * (9/5)
Simplifying further:
x = 27/35
Therefore, the fraction that bears the same ratio to 1/27 as 3/7 does to 5/9 is 27/35.
for such more question on fraction
https://brainly.com/question/1622425
#SPJ8
help me pls this is hard
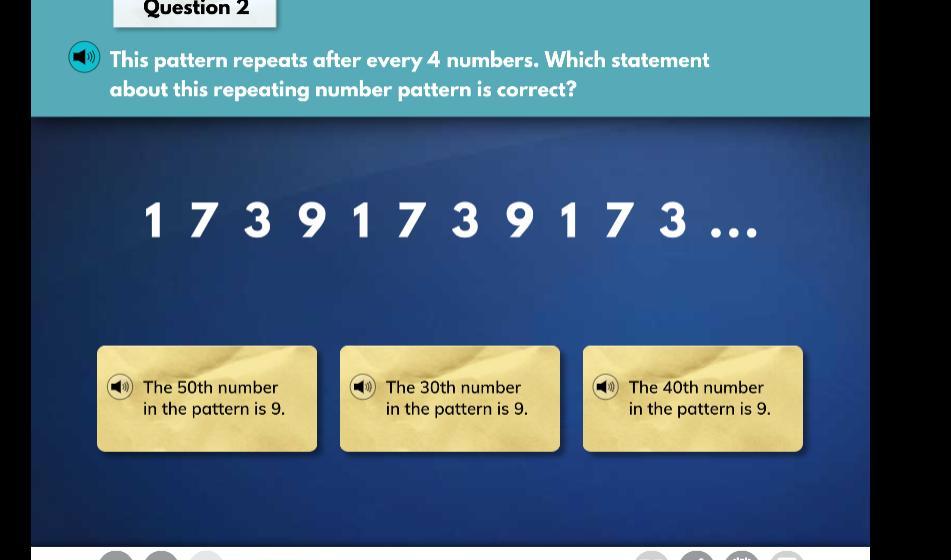
Answers
Answer:
the 40th number in the pattern is 9
Pls help;)))))))))))))))(

Answers
Answer:
Option 2)
Step-by-step explanation:
In a function, any number can be an x-coordinate only once.
Option 1) The 2 appears twice as x.
Option 2) All x-coordinates are different.
Option 3) The 2 appears twice as an x-coordinate.
Option 4) The 3 appears twice as an x-coordinate.
Answer: Option 2)
fraction that is equivalent to 3/9 and has a denominator of 3."
Answers
, The fraction equivalent to 3/9 with denominator 3 is the proper fraction p=1/3
What is a fraction in math?A fraction is a part of a whole. In arithmetic, the number is expressed as a quotient, in which the numerator is divided by the denominator. In a simple fraction, both are integers. A complex fraction has a fraction in the numerator or denominator. In a proper fraction, the numerator is less than the denominator.
Given here: The fraction 3/9
Now we can simplify the fraction further by dividing both the numerator and denominator by 3
let p=3/9
then p=3/3 / 9/3
p= 1/3
Hence, The fraction equivalent to 3/9 with denominator 3 is the proper fraction p=1/3
Learn more about fractions here:
https://brainly.com/question/29264210
#SPJ1
Please help me with this
Answers
An example of a function that models a linear relationship between two quantities, x and y is y = mx + b
How to explain the functionWe need to use the equation of a straight line, which is commonly expressed in slope-intercept form as:
y = mx + b
In this function, x represents the independent variable, m is the slope of the line, and b is the y-intercept. To use this function, we simply plug in the values of x, m, and b that correspond to the specific relationship we are modeling.
Learn more about functions on
https://brainly.com/question/11624077
#SPJ1
2 more than n is the quotient of n and 6
Answers
Answer:
6n+2
Step-by-step explanation:
Find x for the side given

Answers
Answer:
3) 5
Step-by-step explanation:
Sine = \(\frac{opposite}{hyphotenuse}\)
Sine 30 = \(\frac{x}{10}\)
sine of 30 is 0.5
0.5 = \(\frac{x}{10}\)
multiply 10 on both sides
0.5 x 10 = \(\frac{x}{10}\) x 10
5 = x
What is the meaning of "A group G whose order is a prime number has only two subgroups, G itself and 1 = {1}"?

Answers
If G is not a cyclic group, then it does not have a subgroup of order p. Conversely, if G is cyclic, then it has a unique subgroup of order p.
The statement "A group G whose order is a prime number has only two subgroups, G itself and 1 = {1}" means that if G is a group with a prime number of elements, then the only subgroups of G are the trivial subgroup {1} and the group G itself.
In other words, there are no other proper subgroups of G besides these two.
The statement "A group G whose order is (p-1) where p is a prime number has a subgroup of order p if and only if G is cyclic" means that if G is a group with (p-1) elements, where p is a prime number, then G has a subgroup of order p if and only if G is a cyclic group.
In other words, if G is not a cyclic group, then it does not have a subgroup of order p. Conversely, if G is cyclic, then it has a unique subgroup of order p.
for such more question on prime number
https://brainly.com/question/18187355
#SPJ11
1.A car is traveling down a highway at a constant speed, described by the equation ca st waveleng topres his tay istance, in miles, that the car travels at this speed in
d = 65t, where d represents the distance, in miles, that the car travels at this speed in
1 hours.
a. What does the 65 tell us in this situation?
b. How many miles does the car travel in 1.5 hours?
c. How long does it take the car to travel 26 miles at this speed?
Answers
a) The 65 in the equation, d = 65t, where d is the distance in miles and t is the time, is the speed of the car.
b) In 1.5 hours, the car will cover a distance of 97.5 miles.
c) At the speed of 65 miles per hour, the car will take 24 minutes to travel 26 miles.
What is the distance?The distance represents the length covered based on speed and time.
We compute distance as the product of speed and time.
On the other, speed or time is the quotient of distance and time or speed.
Distance in miles in 1 hour, d = 65t
t = time
a) The formula for distance = speed x time
Since t is the time, 65 is the driving speed of the car.
b) Distance in 1.5 hours = 65(1.5) = 97.5 miles
c) At 65 miles per hour, the car will take 24 minutes to travel 26 miles (26/65 x 60).
Thus, there is a ratio relationship between distance, time, and speed.
Learn more about distance, speed, and time at https://brainly.com/question/2854969
#SPJ1
Which of the following expressions are equivalent to 256^x? Select all that apply.
a. 8^2x
b. 4^4x
c. (64^x)^2
d. (16^x)^2
e. 2^8x
Answers
Answer:
d
Step-by-step explanation:
i did it on eduhguenity
The solutions which satisfy the equation A = 256ˣ are
Option B. 4⁴ˣ
Option D. 16²ˣ
Option E. 2⁸ˣ
What is an Equation?
Equations are mathematical statements with two algebraic expressions flanking the equals (=) sign on either side.
It demonstrates the equality of the relationship between the expressions printed on the left and right sides.
Coefficients, variables, operators, constants, terms, expressions, and the equal to sign are some of the components of an equation. The "=" sign and terms on both sides must always be present when writing an equation.
Given data ,
Let the equation be represented as A
Now , the value of A is given by A = 256ˣ
a)
The value of equation B = 8^2x
B = 8²ˣ
On simplifying the equation , we get
B = 8²⁽ˣ⁾
B = 64ˣ
And the value of B ≠ A
b)
The value of equation B = 4^4x
B = 4⁴ˣ
On simplifying the equation , we get
B = 4⁴⁽ˣ⁾
B = 256ˣ
And the value of B = A
c)
The value of equation B = (64^x)^2
B = (64ˣ)²
On simplifying the equation , we get
B = 64²⁽ˣ⁾
B = 4096ˣ
And the value of B ≠ A
d)
The value of equation B = (16^x)^2
B = (16ˣ)²
On simplifying the equation , we get
B = 16²⁽ˣ⁾
B = 256ˣ
And the value of B = A
e)
The value of equation B = 2^8x
B = (2⁸)ˣ
On simplifying the equation , we get
B = 256⁽ˣ⁾
And the value of B = A
Hence , the equations which satisfy are
Option B. 4⁴ˣ
Option D. 16²ˣ
Option E. 2⁸ˣ
To learn more about equations click :
https://brainly.com/question/19297665
#SPJ2
PLEASE ANSWER BOTH QUESTIONS!

Answers
The correct statement regarding the transformation is given as follows:
Dilation bu a scale factor of 0.5 about the origin. Then reflect over the x-axis.
The true statements about the dilation are given as follows:
Dilating a line segment can change the length.Dilating a polygon can change the area.What is a dilation?A dilation can be defined as a transformation that multiplies the distance between every point in an object and a fixed point, called the center of dilation, by a constant factor called the scale factor.
For this problem, the coordinates of the dilated line segment are half the coordinates of the original line segment, hence the scale factor is given as follows:
k = 0.5.
The y-coordinate then has the signal exchange, hence the figure is also reflected over the x-axis.
More can be learned about dilation at brainly.com/question/3457976
#SPJ1
I’ll give brainiest need a answer asap
Use the given information to find
m∠A.
m∠D=121°
m∠A= (2x)°
m∠B=(x+40)°
M
Answers
Answer:
m∠A = 38/3
Step-by-step explanation:
Sum of 3 angles of a triangle = 180°
m∠D + m∠A + m∠B = 180°
Substituting values given for each angle
121 + 2x + (x + 40) = 180
121 + 3x + 40 = 180
3x + 161 = 180
3x = 180-161 = 19
x = 19/3
m∠A = 2x = 38/3
write the first 10 multiplies of 6 and 8 pairs of numbers and find this LCM
Answers
↪\( \huge\rm{answer: } \: \boxed{ \purple{24 }}\)
➖➖➖➖➖➖➖➖➖➖➖➖➖➖➖
M(6)= {0, 6, 12, 18, 24, 30, 36, 42, 48, 54, 60 ...}
M(8) = {0, 8, 16, 24, 32, 40, 48, 56, 64, 72, 80...}
➖➖➖➖➖➖➖➖➖➖➖➖➖➖➖
I hope you understood!✏
➖➖➖➖➖➖➖➖➖➖➖➖➖➖➖
\( \huge\boxed{ \boxed{ \rm{Hope \: this \: helps }}}\)
Answer:
6=0,6,12,18,24,30,36,42,48,54,60
8=0,8,16,24,32,40,48,56,64,72,80
HCF=24,48
LCM=0,6,12,18,,30,36,42,48,54,60,8,16,24,32,40,,56,64,72,80
1.2 Mr John is to deposit R 5,000 in his bank account. Deposits are calculated as follows: Cash deposit : At ATM: R4,80 +1,20% of the above value. At the Branch R8,00 + 1,50% of the above value. John claims that the difference of depositing R5 000 at ATM and at the Branch is R18, 20 Verify the stament (5) [23
Answers
The calculated difference is -R18.20, which means the deposit at the branch is higher than the deposit at the ATM by R18.20
What is simple interest?The simple interest formula is J = C ∙ i ∙ t, where J is interest, C is principal, i is interest rate, and t is time. To calculate the simple interest, just substitute the values in the formula and perform the calculation.
Calculate the deposit:
\(D=R4.80 + 1.20% of R5,000\\=R4.80 + (1.20/100) * R5,000\\= R4.80 + R60\\= R64.80\)
Now, the deposit amount for the branch:
\(DB=R8.00 + 1.50% of R5,000\\= R8.00 + (1.50/100) * R5,000\\= R8.00 + R75\\= R83.00\)
The difference will be:
\(Difference = R64.80 - R83.00\\Difference = -R18.20\)
The calculated difference is -R18.20, which means the deposit at the branch is higher than the deposit at the ATM by R18.20. Therefore, John's statement is incorrect.
See more about finances at brainly.com/question/10024737
#SPJ1
1. The population of a town was 112 in 2014. The population doubles every year.
(a) Use the exponential growth model to write an equation that estimates the population t years after 2014.
(b) Estimate the population of the town in 2023.
Show your work.
Answer:

Answers
Answer: 2048
Step-by-step explanation: 1. Write the exponential growth model.
The population of the town doubles every year, so the growth factor is 2. The initial population is 112, so the equation is: P(t) = 112(2)^t where P(t) is the population in year t and t is the number of years since 2014.
2. Estimate the population of the town in 2023. To estimate the population of the town in 2023, we can set t=9, since 2023 is 9 years after 2014. Plugging this into the equation, we get: P(9) = 112(2)^9 = 2048
Therefore, the estimated population of the town in 2023 is 2048.
A square painting has an area of 9 square metres. How long is each side?
Answers
Answer:
2
Step-by-step explanation:
Solve for x in the diagram below?

Answers
The value of x in the triangle with angles 80, 40 and (13x - 5) degrees is 5.
Sum of angle in a triangleThe sum of angle in a triangle is equals to 180 degrees. Recall a triangle have three sides. Therefore,
80 + 40 + 13x - 5 = 180
120 - 5 + 13x = 180
115 + 13x = 180
subtract 115 from both sides of the equation
115 - 115 + 13x = 180 - 115
13x = 65
divide both sides by 13
13x / 13 = 65 / 13
x = 5
learn more on triangle here: https://brainly.com/question/15268683
Find the slope of the
given graph.

Answers
Answer:
X=-4
Step-by-step explanation:
No y value so just count ur x value
Patel is solving 8x2 + 16x + 3 = 0. Which steps could he use to solve the quadratic equation? Select three options. 8(x2 + 2x + 1) = –3 + 8 x = –1 Plus or minus StartRoot StartFraction 5 Over 8 EndFraction EndRoot x = –1 Plus or minus StartRoot StartFraction 4 Over 8 EndFraction EndRoot 8(x2 + 2x + 1) = 3 + 1 8(x2 + 2x) = –3
Answers
The steps peter could use in solving the quadratic equation is by applying the quadratic formula; x = –1 Plus or minus StartRoot StartFraction 5 Over 8 EndFraction EndRoot
How to solve quadratic equation?8x² + 16x + 3 = 0
using Quadratic formula
The following steps are involved
\(x = \frac{ -b \pm \sqrt{b^2 - 4ac}}{ 2a }\)
\(x = \frac{ -16 \pm \sqrt{16^2 - 4(8)(3)}}{ 2(8) }\)
\(x = \frac{ -16 \pm \sqrt{256 - 96}}{ 16 }\)
\(x = \frac{ -16 \pm \sqrt{160}}{ 16 }\)
\(x = \frac{ -16 \pm 4\sqrt{10}\, }{ 16 }\)
\(x = \frac{ -16 }{ 16 } \pm \frac{4\sqrt{10}\, }{ 16 }\)
\(x = -1 \pm \frac{ \sqrt{10}\, }{ 4 }\)
\(x = -1 \pm \frac{ \sqrt{5}\, }{ 2 }\)
\(x = -0.209431\)
or
\(x = -1.79057\)
Therefore, the steps peter could follow will give the result
\(x = -1 \pm \frac{ \sqrt{5}\, }{ 2 }\)
Read more on quadratic equation:
https://brainly.com/question/1214333
#SPJ1
What is the approximate 95% prediction interval for the dependent variable when the independent variable value is 20, assuming the fitted regression line is: Y = 1.50 + 6.0(X). Assume the sample size is 20 and the standard error of the regression (SEE) is 1.2. You should use the "rule of thumb" used in class here.
Answers
Answer:
Step-by-step explanation:
95% prediction interval for the dependent variable when the independent variable value is 20,
assuming the fitted regression line is: Y = 1.50 + 6.0(X).
Assume the sample size is 20
Y = 1.50 + 6.0(X)
sample X = 20
here predicted value = 1.5+6*20
= 121.5
therefore 95% prediction interval for the dependent variable
the standard error of the regression (SEE) is 1.2.
\(=121.5 \pm 2*1.2\\\\=121.1+2*1.2\\\\=121.1+2.4\\\\=123.9\ \texttt {and} \\\\=121.1-2*1.2\\\\=121.1-2.4\\\\=119.1 \\\\(119.10 \ \text {to}\ 123.90)\)
-3
n is an integer.
Write down the possible values of n.
I
Answers
Answer:
since n is an integer you substitute n with all the integers. But since that is too much you should use infinity.
n=[-∞,+∞] where -∞ is a negative infinity which stands for negative numbers and +∞ is a positive infinity where it stands for positive numbers.
an integer contains negative and positive numbers so above is the answer.
Step-by-step explanation:
Find the terminal point on the unit circle determined by 5pi over 6 radians.
Use exact values, not decimal approximations.
Answers
The terminal point on the unit circle determined by 5π/6 radians is (-√3/2, 1/2).
To find the terminal point on the unit circle determined by an angle of 5π/6 radians, we need to consider the coordinates of the point (x, y) on the unit circle that corresponds to this angle.
The unit circle has a radius of 1 unit, and the terminal point can be found by using the trigonometric functions cosine and sine.
For the angle 5π/6, the cosine function gives us the x-coordinate, and the sine function gives us the y-coordinate.
Using the exact values of cosine and sine for 5π/6, we have:
cos(5π/6) = -√3/2
sin(5π/6) = 1/2
Therefore, the terminal point on the unit circle determined by 5π/6 radians is (-√3/2, 1/2).
For more such answers on trigonometric functions
https://brainly.com/question/25618616
#SPJ8
The pressure, P (in lbs/ft2 ), in a pipe varies over time. Five times an hour, the pressure oscillates from a low of 90 to a high of 230 and then back to a low 90. The pressure at t = 0 is 90.
Question: Find a possible formula for P=f(t)
Answers
P=160-70*cos(2π*(1/12)*t)
• Pressure in mathematics or physics is defined as force per unit area. The pressure exerted by a solid object which is resting on top of a solid surface is the weight divided by the area of the object . SI unit of force is Newton (N).
First, we find the amplitude by taking the difference between the peak values and dividing by two:
Amplitude=(230+90)/2=70
We can find the center value by either adding 70 to the minimum or subtracting 70 from the maximum:
90+70=160
230-70=160
This tells us that the Center Value=160
The frequency that we are operating at is given as:
f = 5/hour
so in minutes
f=5*(1/60)/minute=5/60=1/12 per minute
So, combining everything we get
P=160-70*cos(2π*(1/12)*t)
Learn more about Pressure here
https://brainly.com/question/26040104
#SPJ9
use the math properties to write an equivalent expression to the following x + 5+2x + 2
Answers
Answer:
3x + 7
Step-by-step explanation:
combining like terms there is 2x + x = 3x and 5 + 2 = 7
Look at this triangle work out length BC

Answers
Answer:
The length of BC is √105 cm or 10.2 cm.
Step-by-step explanation:
You have to apply Pythagoras Theorem, c² = a² + b² where c represents hypotenuse, a and b are the sides :
\( {c}^{2} = { a}^{2} + {b}^{2} \)
\(let \:a =BC \:, \: b = 8 \: , \: c = 13\)
\( {13}^{2} = {BC}^{2} + {8}^{2} \)
\(169 = {BC}^{2} + 64\)
\( {BC}^{2} = 169 - 64\)
\( {BC}^{2} = 105\)
\(BC = \sqrt{105} \)
\(BC = 10.2 \: cm \: (3s.f)\)

Answer:
\( \boxed{\sf Length \ of \ BC = \sqrt{105} \ cm} \)
Given:
AB = 13 cm
AC = 8 cm
To Find:
Length of BC
Step-by-step explanation:
Pythagoras theorem states that “In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.
\( \therefore \\ \sf {AC}^{2} + {BC}^{2} = {AB}^{2} \\ \sf \implies {8}^{2} + {BC}^{2} = {13}^{2} \\ \\ \sf {8}^{2} = 64 : \\ \sf \implies 64 + {BC}^{2} = {13}^{2} \\ \\ \sf {13}^{2} = 169 : \\ \sf \implies 64 + {BC}^{2} = 169 \\ \\ \sf Substract \: 64 \: from \: both \: sides : \\ \sf \implies (64 - 64) + {BC}^{2} = 169 - 64 \\ \\ \sf 64 - 64 = 0 : \\ \sf \implies {BC}^{2} = 169 - 64 \\ \\ \sf 169 - 64 = 105 : \\ \sf \implies {BC}^{2} = 105 \\ \\ \sf \implies BC = \sqrt{ 105 } \ cm \)
So,
Length of BC = \( \sqrt{105} \) cm
Determine the average value of the following functions on the interval [1,2]. Give your answers first in exact form, and then in decimal form with at least three decimal digits.
y = x, Average Value = (exact)
Average Value %u2248 (decimal)
y = x2, Average Value = (exact)
Average Value %u2248 (decimal)
y = x3, Average Value = (exact)
Average Value %u2248 (decimal)
y = x4, Average Value = (exact)
Average Value %u2248 (decimal)
Answers
The exact average value of \(y = x^4\) on [1,2] is 31/5, and the decimal approximation is 6.200.
To find the average value of a function f(x) on an interval [a,b], we use the formula:
Average Value = (1/(b-a)) * ∫(a to b) f(x) dx
Using this formula, we can find the average values of the given functions on the interval [1,2]:
y = x
Average Value =\((1/(2-1)) * ∫(1 to 2) x dx= ∫(1 to 2) x dx= [x^2/2] from 1 to 2= (2^2/2) - (1^2/2)\)
= 3/2
Therefore, the exact average value of y = x on [1,2] is 3/2, and the decimal approximation is 1.500.
\(y = x^2\)
Average Value =\((1/(2-1)) * ∫(1 to 2) x^2 dx\)
\(= ∫(1 to 2) x^2 dx= [x^3/3] from 1 to 2= (2^3/3) - (1^3/3)\)
= 7/3
Therefore, the exact average value of\(y = x^2\) on [1,2] is 7/3, and the decimal approximation is 2.333.
y = x^3
Average Value = \((1/(2-1)) * ∫(1 to 2) x^3 dx\)
\(= ∫(1 to 2) x^3 dx= [x^4/4] from 1 to 2= (2^4/4) - (1^4/4)\)
= 15/4
Therefore, the exact average value of\(y = x^3\) on [1,2] is 15/4, and the decimal approximation is 3.750.
\(y = x^4\)
Average Value =\((1/(2-1)) * ∫(1 to 2) x^4 dx\)
\(= ∫(1 to 2) x^4 dx= [x^5/5] from 1 to 2= (2^5/5) - (1^5/5)\)
= 31/5
For more such Questions on Average value
https://brainly.com/question/30460573
#SPJ4
