Answers
a) Function f(x) = 2^x is an exponential growth function because a = 2 is greater than 0 and b = 2 is greater than 1. The graph of the function starts at (0,1) and increases rapidly as x increases.
b) Function f(x) = (1/2)^x is an exponential decay function because a = 1/2 is greater than 0 and b = 1/2 is between 0 and 1. The graph of the function starts at (0,1) and decreases rapidly as x increases.
What are the key features of both graphs?Key features of both graphs:
x-intercept: There is no x-intercept for either function.y-intercept: The y-intercept for both functions is (0,1).Domain: The domain of both functions is all real numbers.Range: The range of f(x) = 2^x is all positive real numbers, while the range of f(x) = (1/2)^x is all positive numbers between 0 and 1.Asymptote: The x-axis is a horizontal asymptote for both functions.Growth/decay factor: The growth/decay factor for f(x) = 2^x is 2, while the growth/decay factor for f(x) = (1/2)^x is 1/2.Learn more about exponential growth function at:
https://brainly.com/question/11487261
#SPJ1

Related Questions
Fill in the blank with an appropriate word, phrase, or symbol(s). The number of regions created when constructing a Venn diagram with three overlapping sets is The number of regions created when constructing a Venn diagram with three overlapping sets is 8 3 6
Answers
The number of regions created when constructing a Venn diagram with three overlapping sets is 8.
In a Venn diagram, each set is represented by a circle, and the overlapping regions represent the elements that belong to multiple sets.
When three sets overlap, there are different combinations of elements that can be present in each region.
For three sets, the number of regions can be calculated using the formula:
Number of Regions = 2^(Number of Sets)
In this case, since we have three sets, the formula becomes:
Number of Regions = 2^3 = 8
So, when constructing a Venn diagram with three overlapping sets, there will be a total of 8 regions formed.
Each region represents a unique combination of elements belonging to different sets.
These regions help visualize the relationships and intersections between the sets, providing a graphical representation of set theory concepts and aiding in analyzing data that falls into multiple categories.
Therefore, the correct answer is 8.
To know more about Venn diagram refer here :
https://brainly.com/question/318076803
#SPJ11
what values represent the sum of 1/2 + 3/12
Answers
How many milliliters of water do you need to fill up a 1.5 quart bottle
Answers
Answer:
1419 millimeters
Step-by-step explanation:
Trust me!:)
given any collection of n points in the plane with distinct x-coordinates, one can prove that there a unique polynomial of degree ≤n −1 that passes through these points. (we will prove this in class, soon). write down a linear system of equations that can be used to confirm this. describe the matrix of coefficients.
Answers
To prove that there is a unique polynomial of degree ≤n − 1 passing through a collection of n points in the plane with distinct x-coordinates, we can create a linear system of equations. The matrix of coefficients in the linear system is a Vandermonde matrix, which is a square matrix with powers of the x-coordinates as its entries.
To confirm the existence of a unique polynomial, we create a linear system of equations. Suppose we have n points (x₁, y₁), (x₂, y₂), ..., (xₙ, yₙ). We seek a polynomial of the form p(x) = a₀ + a₁x + a₂x² + ... + aₙ₋₁xⁿ⁻¹ that passes through these points.
For each point, we can substitute the x-coordinate into the polynomial and set it equal to the corresponding y-coordinate. This gives us n equations of the form a₀ + a₁x + a₂x² + ... + aₙ₋₁xⁿ⁻¹ = y.
We can rewrite these equations in matrix form as AX = Y, where A is the Vandermonde matrix of coefficients, X is the column vector of coefficients a₀, a₁, ..., aₙ₋₁, and Y is the column vector of y-coordinates.
The Vandermonde matrix A is a square matrix of size n × n. The entry in the i-th row and j-th column is xᵢⁿ⁻ʲ, where xᵢ is the x-coordinate of the i-th point and j ranges from 0 to n-1.
The uniqueness of the solution to this linear system can be proven by showing that the Vandermonde matrix is invertible, which means it has a unique inverse. This guarantees that there is a unique polynomial of degree ≤n − 1 passing through the given collection of points with distinct x-coordinates.
Learn more about polynomials here:
https://brainly.com/question/11536910
#SPJ11
How long should a person cool down after physical activity? responses 1 to 2 minutes 1 to 2 minutes 5 to 10 minutes 5 to 10 minutes 10 to 15 minutes 10 to 15 minutes 15 to 20 minutes
Answers
Answer:
15 to 20 minutes
Step-by-step explanation:
Answer:
10-15
Step-by-step explanation:
i took the test
imagine you proposed a linear relationship between x and y. x is distributed with a mean of 3 and a standard deviation of 7 and y is distributed with mean of 6 and a standard deviation of 2. answer the two questions below based on the information above. (4 points; note: you do not need the raw scores to answer the questions. you have all of the information you need) a) if the correlation between the x and y is 1 what would the value of error variance for your linear model be? why? b) if the correlation between x and y is 0 what would the value of error variance be? why?
Answers
This is because when there is no correlation, the variation in y cannot be explained by the variation in x, leaving a lot of room for error. the error variance for the linear model
a) If the correlation between x and y is 1, it means that the two variables have a perfect positive linear relationship. In this case, the error variance for the linear model would be 0. This is because when there is a perfect correlation, all the variation in y can be explained by the variation in x, leaving no room for error. Therefore, the error variance would be 0.
b) If the bet correlation ween x and y is 0, it means that the two variables have no linear relationship. In this case, the error variance for the linear model would be the sum of the variances of x and y. This is because when there is no correlation, the variation in y cannot be explained by the variation in x, leaving a lot of room for error. Therefore, the error variance would be the sum of the variances of x and y, which is 49+4=53.
a) If the correlation between x and y is 1, the value of the error variance for your linear model would be 0. This is because a correlation of 1 indicates a perfect positive linear relationship between x and y, meaning there is no error or deviation from the predicted values of y based on the linear model.
b) If the correlation between x and y is 0, the value of the error variance would be equal to the variance of y. This is because a correlation of 0 indicates that there is no linear relationship between x and y, meaning that the linear model provides no predictive value for y. In this case, the error variance is equal to the variance of y, which can be calculated by squaring the standard deviation of y (2^2 = 4).
learn more about correlation here : https://brainly.com/question/22085260
#SPJ11
math workkk
pls help me
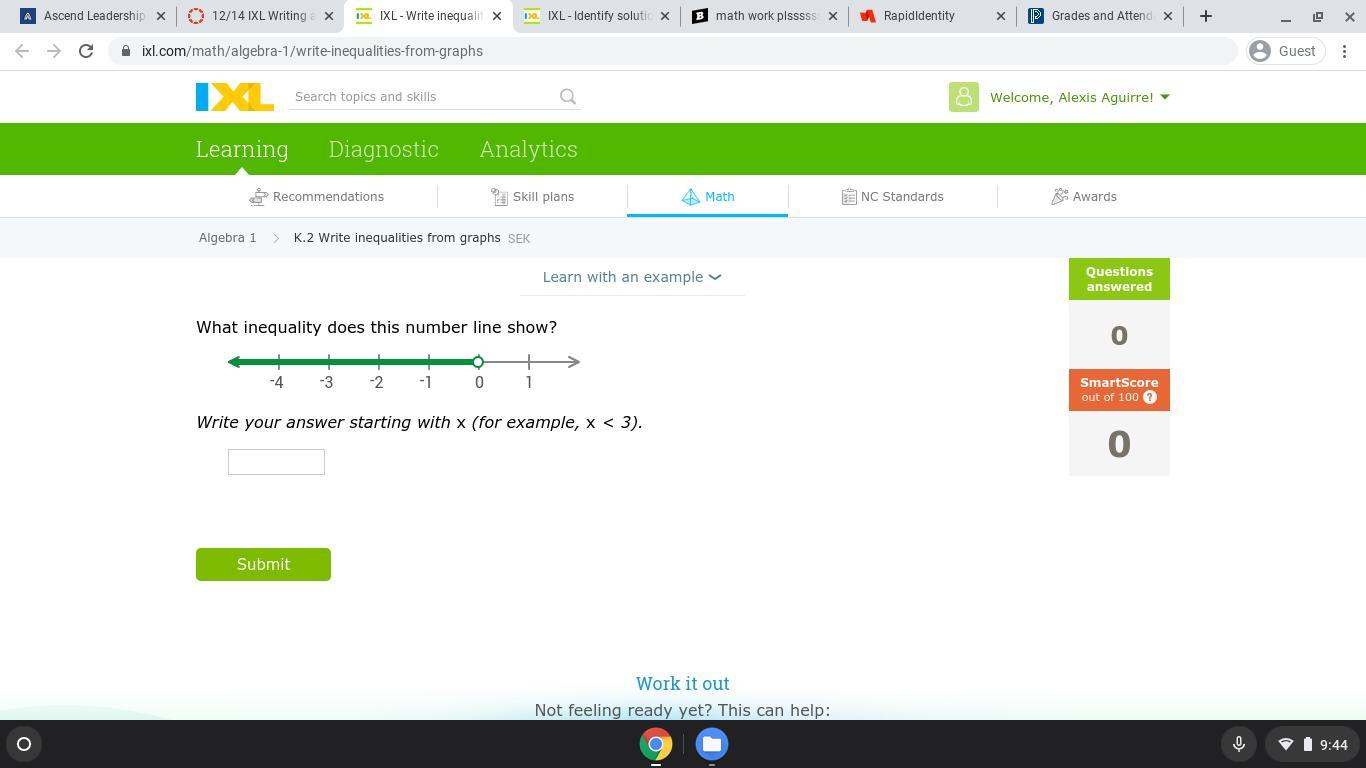
Answers
Answer:
x<0
Step-by-step explanation:
open dot means regular symbol and it is pointing to the left <-
Answer:
x< 1
Step-by-step explanation:
So in the number line we can see that x=0 So therefore we can put any number that is greater that 0 for example:
x<1
x<2
x<1000
x>-1
x>-2
find the solution to the following system by substitution
4x+y=40
Answers
There are many ways of doing it, but here is mine.
Step-by-step explanation:
x = 6, y = 16
So now you multiply six by four and you will get twenty four plus sixteen, if you add them then you will get forty.
When a ball is thrown or kicked, the path it travels is shaped like a parabola. Suppose a football is kicked from ground level, reaches a maximum height of 25 feet, and hits the ground 100 feet from where it was kicked. Assuming that the ball was kicked at the origin, write an equation of the parabola that models the flight of the ball.
Answers
The equation of the parabola that models the flight of the ball is y = ax^2 + 25, where a can be any non-zero real number.
The equation of the parabola that models the flight of the ball can be expressed in the standard form: y = ax^2 + bx + c.
Since the ball is kicked from the origin, the equation simplifies to y = ax^2 + c.
To find the values of a and c, we can use the given information. The ball reaches a maximum height of 25 feet, which means the vertex of the parabola is at the point (0, 25). This gives us c = 25.
Now we need to determine the value of a. Since the maximum height occurs at the vertex, the x-coordinate of the vertex is 0. Additionally, we know that the ball hits the ground 100 feet from where it was kicked. The x-coordinate at that point is 100. Therefore, we can use the vertex form of the parabola equation, which is x = -b/2a, to find a.
Substituting the known values, we have 0 = -b/2a, which implies b = 0. Therefore, a can be any non-zero value.
Know more about parabola here:
https://brainly.com/question/11911877
#SPJ11
Find the distance between the two points in simplest radical form.

Answers
The distance bewteen the points (-3,5) and (3,1) in a simple radical form is 2√13.
What is the distance between the given points?The distance formula used in finding the distance between two points is expressed as;
d = √( ( x₂ - x₁ )² + ( y₂ - y₁ )² )
From the graph;
Point A: (-3,5)
x₁ = -3
y₁ = 5
Point B: (3,1)
x₂ = 3
y₂ = 1
Plug the given values into the distance formula and simplify.
\(d = \sqrt{( x_2 - x_1)^2 + (y_2 -y_1 )^2} \\\\d = \sqrt{( 3-(-3))^2 + (1 -5)^2} \\\\d = \sqrt{( 3+ 3)^2 + (1 -5)^2} \\\\d = \sqrt{( 6)^2 + (-4)^2} \\\\d = \sqrt{36 + 16} \\\\d = \sqrt{52}\\\\d = 2\sqrt{13}\)
Therefore, the distance between the points is 2√13.
Learn more about the distance formula here: brainly.com/question/24509115
#SPJ1
Assume Mr. Crawley always eats 1 biscuit (B) with every 2 cups of tea (T) that he drinks. He only enjoys an additional cup of tea if he gets of an additional biscuit to go with it, and he only enjoys an additional biscuit if he also gets an additional 2 cups of tea. Suppose he has $56 per week to spend on tea and biscuits, and a cup of tea costs $3 while a biscuit costs $2. Mr. Crawley's utility function can be expressed as , and his optimal bundle is (a) U(B,T)-min(2B, T); 7 biscuits and 14 cups of tea (b) U(B,T)-min(2B, 2T); 7 biscuits and 14 cups of tea (c) U(B,T)-mind(B, 27)16 biscuits and 8 cups of tea (d) U(B,T)-min(23, T); 16 biscuits and 8 cups of tea (e) None of the above
Answers
To determine Mr. Crawley's optimal bundle, we need to find the combination of biscuits and cups of tea that maximizes his utility within the given budget constraint.
Let's analyze the options provided:
(a) U(B,T) - min(2B, T); 7 biscuits and 14 cups of tea
(b) U(B,T) - min(2B, 2T); 7 biscuits and 14 cups of tea
(c) U(B,T) - min(dB, 27); 16 biscuits and 8 cups of tea
(d) U(B,T) - min(2B, T); 16 biscuits and 8 cups of tea
(e) None of the above
From the given options, it seems that options (a), (b), and (d) all suggest the same bundle: 7 biscuits and 14 cups of tea. This is consistent with the statement that Mr. Crawley always eats 1 biscuit with every 2 cups of tea.
Now, let's analyze the utility function provided:
U(B, T) - min(2B, T)
The utility function subtracts the minimum value between 2B and T from the main utility function U(B, T). This implies that Mr. Crawley would prefer a higher value for T compared to 2B. In other words, he values cups of tea more than biscuits.
Among the given options, only option (d) satisfies this preference: U(B, T) - min(2B, T). Therefore, the correct answer is (d) U(B, T) - min(2B, T); 16 biscuits and 8 cups of tea.
The optimal bundle for Mr. Crawley, given his utility function and budget constraint, is 16 biscuits and 8 cups of tea.
learn more about functions here:
https://brainly.com/question/30721594
#SPJ11
LaTanya will walk (3 mi/hr) her bike from her house to the
bike shop, which is 1.5 mi from her house, to get the bike
fixed. She expects to wait 30 min for the repair. Then she
will ride (10 mi/hr) her bike home. How long will it take
her to get home?
A)2.12 hr
B)2hr
C)1.15
D)4hr
Answers
Answer:
1.15 hr
Step-by-step explanation:
Given that:
To get bike fixed :
Speed = 3 miles per hour
Distance = 1.5 miles
Time taken = distance / speed
Time taken = 1.5 / 3 = 0.5 hour
Waiting time for repair = 30 minutes = 0.5 hours
Return home :
Speed = 10 miles per hour
Distance = 1.5 miles
Time taken = distance / speed
Time taken = 1.5 / 10 = 0.15 hour
Total time : (0.5 + 0.5 + 0.15) = 1.15 hr
Answer the question considering an event to be unusual it its probability is les than or equal to 0.05.
Assume that a study of 300 randomly selected school bus routes showed that 274 arrived on time. is it unusual for a school bus to arrive late?
NEED WORK SHOWN PLEASE!
Answers
Based on the given information, we can determine whether it is unusual for a school bus to arrive late. To do this, we need to calculate the probability of a school bus arriving late and compare it to the threshold of 0.05.
To determine if it is unusual for a school bus to arrive late, we need to calculate the probability of a school bus arriving late and compare it to the threshold of 0.05. In this case, out of the 300 randomly selected school bus routes, 274 arrived on time. This means that the number of school buses arriving late would be 300 - 274 = 26. To calculate the probability of a school bus arriving late, we divide the number of buses arriving late by the total number of buses:
P(arriving late) = 26/300 = 0.0867
The probability of a school bus arriving late is 0.0867, which is greater than the threshold of 0.05. Therefore, it is not considered unusual for a school bus to arrive late based on this sample.
To further explain, we can interpret the probability of 0.0867 as the likelihood of randomly selecting a school bus route from the population and it is late. Since this probability is greater than 0.05, we conclude that the event of a school bus arriving late is not considered unusual based on the given sample.
It's important to note that this conclusion is based on the assumption that the sample is representative of the population of school bus routes. If there are specific factors or circumstances that may affect the timeliness of the buses in the population, further analysis and consideration of those factors would be necessary to make a more accurate determination.
Learn more about probability here:- brainly.com/question/31828911
#SPJ11
Miguel made $264 for 12 hours of work.At the same rate, how much would he make for 7 hours of work?

Answers
First, divide the money that Miguel made (264) by the number of hours worked (12)
264/12 = $22 per hour
For 7 hours:
22 x 7 = $154
Formalize the following in terms of atomic propositions r, b, and w, first making clear how they correspond to the
English text. (a) Berries are ripe along the path, but rabbits have not been seen in the area.
(b) Rabbits have not been seen in the area, and walking on the path is safe, but berries are ripe along the path.
(c) If berries are ripe along the path, then walking is safe if and only if rabbits have not been seen in the area.
(d) It is not safe to walk along the path, but rabbits have not been seen in the area and the berries along the path are ripe.
e) For walking on the path to be safe, it is necessary but not sufficient that berries not be ripe along the path and for rabbits not to
pave been seen in the area.
Walking is not safe on the path whenever rabbits have been seen in the area and berries are ripe along the path.
Answers
Walking is not safe on the path whenever rabbits have been seen in the area, and berries are ripe along the path. This is formalized by using the →(if-then) and ∧(logical and) operators.
Given information and corresponding atomic propositions:
We need to formalize the given statements in terms of atomic propositions r, b, and w, which are defined as follows:
r: Rabbits have been seen in the area.
b: Berries are ripe along the path.
w: Walking on the path is safe.
Now, let us formalize each of the given statements in terms of these atomic propositions:
a) Berries are ripe along the path, but rabbits have not been seen in the area.
b: Rabbits have not been seen in the area, and walking on the path is safe, but berries are ripe along the path.
c: If berries are ripe along the path, then walking is safe if and only if rabbits have not been seen in the area.
d: It is not safe to walk along the path, but rabbits have not been seen in the area, and the berries along the path are ripe.
e) For walking on the path to be safe, it is necessary but not sufficient that berries not be ripe along the path and for rabbits not to have been seen in the area.
Walking is not safe on the path whenever rabbits have been seen in the area, and berries are ripe along the path.
The formalizations in terms of atomic propositions are:
a) b ∧ ¬r.b) ¬r ∧ w ∧
b.c) (b → w) ∧ (¬r → w).
d) ¬w ∧ ¬r ∧
b.e) (¬r ∧ ¬b) → w.b ∧
Berries are ripe along the path, but rabbits have not been seen in the area.
This is formalized by using the ∧(logical and) operator.
(¬r ∧ ¬b) → w: It means For walking on the path to be safe, it is necessary but not sufficient that berries not be ripe along the path and for rabbits not to have been seen in the area.
For more related questions on area:
https://brainly.com/question/1631786
#SPJ8
What quadrant is the terminal side of the angle graphed in standard position? Then, find a coterminal angle.
7π/4 = 7pi/4
QI
QII
QIII
QIV
Answers
The terminal side of the angle 7π/4 is in Quadrant IV. A coterminal angle for 7π/4 is 15 π/4.
To determine the quadrant of the terminal side of an angle graphed in standard position, we look at the sign of the coordinates (x, y) of a point on the terminal side. In this case, the angle is 7π/4.
When evaluating 7π/4, we can convert it to degrees by multiplying by the conversion factor (180°/π). The result is 315°.
In the coordinate system, starting from the positive x-axis and moving counterclockwise, 315° falls in Quadrant IV. Therefore, the terminal side of the angle 7π/4 is in Quadrant IV.
To find a coterminal angle, we can add or subtract multiples of 2π (or 360°) to the given angle.
For example, adding 2π to 7π/4 gives:
7π/4 + 2π = 7π/4 + 8π/4 = 15 π/4
Thus, a coterminal angle for 7π/4 is 15 π/4.
To know more about angle:
https://brainly.com/question/30147425
#SPJ11
The spray from a sprinkler reaches 21 feet from the sprinkler and creates a circle as it spins. What is the circumference of the circle sprayed by the sprinkler? Use
22
7
for π.
21 ft
66 ft
120 ft
132 ft
Answers
Answer:
D: 132 ft
Step-by-step explanation:
C= π2r
So, take pi and multiply that by two..
π2= 6.28
Now, take your radius and multiply it by 6.28.
21 x 6.28= 131.88
Round it two the nearest whole number, which is 132!
Hope this helps!
Answer:
132
Step-by-step explanation:
Conrad has 6 more marbles than rory. If r represents the number of marbles that rory has, which expression represents the number of marbles that conrad has?.
Answers
r+6 is the expression represents the number of marbles that conrad has
Conrad has 6 more marbles than rory.
If r represents the number of marbles that rory has,
r = marbles that Rory has
r+6 = marbles that Conrad has
learn more about of expression here
https://brainly.com/question/11791594
#SPJ4
Describe the transformation(s) of the parent function f(x)
k(x) = -2 f(-x)
Answers
We reflect it about the x axis and stretch it by -2 in the y direction
The vertex form of a quadratic equation is: y = a(x - h)² + k where
a is the vertical stretch
-a is a reflection over the x-axis
(h, k) is the vertex
--> h is the horizontal shift (positive is RIGHT, negative is LEFT)
--> k is the vertical shift (positive is UP, negative is DOWN)
reflect it about the x axis and stretch it by 2 in the y direction
k(x) = -2 f(-x)
y = −f(x) Reflects it about x-axis
y = Cf(x) C > 1 stretches it in the y-direction
Therefore, we reflect it about the x axis and stretch it by -2 in the y direction
Learn more parent function f(x) about here:
https://brainly.com/question/17476031
#SPJ4
Tony is solving the equation 4x = 12x + 20 for x. Tony uses the multiplicative property of equality to rewrite the equation as x = 3x + 20. Which statement correctly explains whether
A Tony used the property correctly? Tony used the property correctly because he multiplied one term on each side of the equals sign by 1/4
B Tony did not use the property correctly because he should have multiplied both sides of the equals sign by 1/12 not 1/4
C Tony did not use the property correctly because he did not multiply every term on both sides of the equals sign by 1/4
D Tony used the property correctly because he multiplied every term containing x by 1/4
Answers
Answer:
C)
Step-by-step explanation:
The correct answer is C: Tony did not use the property correctly because he did not multiply every term on both sides of the equals sign by 1/4.
To solve the equation 4x = 12x + 20, Tony used the multiplicative property of equality but made an error in the application. The correct approach would be to multiply every term on both sides of the equals sign by the reciprocal of the coefficient of x, which is 1/4 in this case.
However, Tony only multiplied one term on each side by 1/4, resulting in equation x = 3x + 20. This action is incorrect because it does not apply the property to every term containing x. To solve the equation correctly, Tony should have multiplied both sides by 1/4, resulting in x/4 = (3x + 20)/4.
help me with these 3 and i’ll love you forever and give you brainliest:)

Answers
Answer:
18. 5
19. 1
20. B) (0,-11)
My neighbor just got two new cats and now she has more than 5 cats. How many cats did she have before?
Answers
The number of cats is an illustration of inequalities and your neighbor has 4 cats before she bought the 2 new cats
What are inequalities?Inequalities are expressions, numbers and mathematical statements that have unequal values when compared and/or evaluated
How to determine the number of cats?We start by representing the initial number of cats with x.
From the question, the new cats she bought are
New = 2
So, we have
x + New greater than 5
Rewrite properly as
x + New > 5
Substitute known values in the above inequality
x + 2 > 5
Subtract 2 from both sides
x + 2 - 2 > 5 - 2
Evaluate the right-hand side
x + 2 - 2 > 3
Evaluate the left-hand side
x > 3
The smallest integer number greater than 3 is 4
Hence, your neighbor has 4 cats before she bought the 2 new cats
Read more about inequality at:'
https://brainly.com/question/24372553
#SPJ1
KM=
What is the measure of KM?
Help please

Answers
And it’s 2
please help i don’t know what to do

Answers
What is the probability of selecting a heart replacing then selecting a star?
Answers
The probability of selecting a heart and then a star with replacement is approximately 0.1875 or 18.75%.
Assuming that a standard deck of 52 playing cards is used, with 13 cards of each suit (including hearts) and 4 suits in total, the probability of selecting a heart on the first draw and then selecting a star (presumably meaning a card from a different suit) on the second draw with replacement is
P (heart than star)
= P (heart) × P (star)
= 13/52 × 39/52
= 507/2704
= 0.1875
where P (heart) is 13/52 is the probability of selecting a heart on the first draw (since there are 13 hearts in the deck), and P (star) is the probability of selecting a card that is not a heart on the second draw (since there are 39 non-heart cards left in the deck after the heart is replaced).
Therefore, the probability of selecting a heart and then a star with replacement is approximately 0.1875 or 18.75%.
To know more about probability here
https://brainly.com/question/30034780
#SPJ4
-- The given question is incomplete, the complete question
"What is the probability of selecting a heart and then a star with replacement?" --
The triangles are congruent by SSS and HL

Answers
Answer:
D
Step-by-step explanation:ik
PLEASE HELP ME:
John took a 5-mile walk to his friend's house.
.
He left at 11 a.m. and arrived at his friend's house at 12:45 p.m.
What was his average speed of walking in miles per hour?
Answers
Answer:
it would take 21 minutes per mile
Step-by-step explanation:
Which of the following statements is true of the function ? Question 2 options: A) g(x) can be graphed by translating the basic rational function ƒ(x) 1∕x right by 3 units and downward by 5 units. B) g(x) can be graphed by translating the basic rational function ƒ(x) 1∕x left by 3 units and downward by 5 units. C) g(x) can be graphed by translating the basic rational function ƒ(x) 1∕x right by 3 units and downward by 5 units. D) g(x) can be graphed by translating the basic rational function ƒ(x) 1∕x left by 5 units and downward by 3 units.

Answers
Transformations are operators that can act on functions, modifying them in different ways. In this particular problem, we see the translations.
The correct option is B:
g(x) can be graphed by translating the basic rational function ƒ(x)= 1∕x left by 3 units and downward by 5 units.
Let's describe the transformations:
Horizontal translation:
For a general function f(x), a horizontal translation of N units is written as:
g(x) = f(x + N)
If N is positive, the shift is to the left.
If N is negative, the shift is to the right
Vertical translation:
For a general function f(x), a vertical translation of N units is written as:
g(x) = f(x) + N
If N is positive, the shift is upwards.
If N is negative, the shift is downwards.
Now that we know this, let's see the problem.
We have:
\(g(x) = \frac{1}{x + 3} - 5\)
So, the original function is:
\(f(x) = \frac{1}{x}\)
Now from f(x) we can apply translations to create g(x).
If first, we apply a translation of 3 units to the left, we get:
\(g(x) = f(x + 3) = \frac{1}{x + 3}\)
If now we apply a translation of 5 units downwards, we get:
\(g(x) = f(x + 3) - 5 = \frac{1}{x + 3} - 5\)
So we can conclude that the correct option is B:
g(x) can be graphed by translating the basic rational function ƒ(x) 1∕x left by 3 units and downward by 5 units.
If you want to learn more about translations, you can read:
https://brainly.com/question/12463306
Please Help ASAP!!!!!!!!

Answers
Answer:
A
Step-by-step explanation:
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
y = 6x - 4 ← is in slope- intercept form
with y- intercept c = - 4 → A
Line AB goes through points A(-4,-3) and B(-2,0). What is the slope of the line?
Answers
Answer:
3/2
Step-by-step explanation:
\((x_{1},y_{1}) = (-4,-3) \\\\\\(x_{2},y_{2}) = (-2 , 0)\\\\Slope =\dfrac{y_{2}-y_{1}}{x_{2}-x_{1}}\\\\\\=\dfrac{0-[-3]}{-2-[-4]}\\\\\\=\dfrac{0+3}{-2+4}=\dfrac{3}{2}\)
Answer:
\( \sf \frac{3}{2} \)
Step-by-step explanation:
\( \large \sf = \frac{ y_{2} - y _{1} }{ x_{2} - x_{1} } \)
\( \sf = \frac{ 0 - ( - 3)}{( - 2) - ( - 4)} \)
\( \sf = \frac{0 + 3}{( - 2) + 4} \)
\( \sf = \frac{0 + 3}{4 - 2} \)
\( \sf = \frac{3}{2} \)
