Given the following information Period Year Sales (yd) 156 2019 102 178 191 2019-period 1 2019-period 2 2019-period 3 2020-period 1 2020-period 2 2020-period 3 2021-period 1 2021-period 2 2021-period 3 2020 229 227 141 2021 230 165 Find the seasonal index (SI) for period 2 (Round your answer to 2 decimal places)
Answers
Therefore, the seasonal index (SI) for period 2 is 1.08.
The formula to compute the seasonal index (SI) for the period is as follows;
SI = Period Average / Overall Average
Given the following information
To calculate the Seasonal Index for period 2, find the average for 2019, 2020, and 2021.
Period Year Sales (yd)
2019-period 1 102
2019-period 2 178
2019-period 3 191
2020-period 1 229
2020-period 2 227
2020-period 3 141
2021-period 1 230
2021-period 2 165
2021-period 3
Overall Average = (102 + 178 + 191 + 229 + 227 + 141 + 230 + 165) / 9
Overall Average = 180.67
Average for the three years (2019, 2020, and 2021) = (102 + 178 + 191 + 229 + 227 + 141 + 230 + 165) / 8
Average for the three years = 180.25
Period 2 average sales = (191 + 227 + 165) / 3
Period 2 average sales = 194.33
Seasonal index (SI) = Period Average / Overall Average
Seasonal index (SI) = 194.33 / 180.67
Seasonal index (SI) = 1.0769
Seasonal index (SI) ≈ 1.08 (rounded to 2 decimal places).
Therefore, the seasonal index (SI) for period 2 is 1.08.
to know more about Seasonal index visit:
https://brainly.com/question/30889595
#SPJ11
Related Questions
What is the answer to 3a + 18 = 12
Answers
Hope this helps
Does the quadratic function
f(x) = 5x² + 5x + 21 have one,
two, or no real zeros? Utilize the
quadratic formula to determine
the answer.
Answers
No real solution
no x - intercept
Answer:
No real zeros.
Step-by-step explanation:
\(\boxed{\begin{minipage}{7.4 cm}\underline{Discriminant of the Quadratic Formula}\\\\$b^2-4ac$ \quad when $ax^2+bx+c=0$\\\\When $b^2-4ac > 0 \implies$ two real zeros.\\When $b^2-4ac=0 \implies$ one real zero.\\When $b^2-4ac < 0 \implies$ no real zeros.\\\end{minipage}}\)
The value of the discriminant shows how many zeros the function has.
Given quadratic function:
\(f(x)=5x^2+5x+21\)
Therefore:
a = 5b = 5c = 21Substitute the values into the discriminant and solve:
\(\begin{aligned}\implies b^2-4ac&=(5)^2-4(5)(21)\\& = 25 - 20(21)\\&=25-420\\&=-395\end{aligned}\)
Therefore, as -395 < 0, there are no real zeros.
circuit optimation problem georgina orwell wants to build a rectangular pig pen along the side of a pre-existing barn, so she will only need fencing on three sides. she has 200 feet of fencing and will use all of it to maximize the pig pen. find the maximum area (in square feet) she can enclose.
Answers
The maximum area that Georgina Orwell can enclose is 10000 square feet.
The maximum area that Georgina Orwell can enclose with 200 feet of fencing is:
Let x be the length of the side of the pig pen along the barn and y be the width of the pig pen.
We know that:
x + 2y = 200
We can use this equation to find the value of one variable in terms of the other. For example, we can solve for y:
y = (200 - x) / 2
We can use this equation to find the area of the pig pen:
A = xy = x((200 - x) / 2)
= (200x - x^2) / 2
To find the maximum area, we need to find the value of x that maximizes A. To do this, we can take the derivative of A with respect to x and set it equal to zero:
A'(x) = (200 - 2x) / 2 = 0
200 - 2x = 0
x = 100
So the maximum area is:
A = (200x - x^2) / 2 = (200 * 100 - 100^2) / 2 = 10000 sq ft.
Therefore, the maximum area that Georgina Orwell can enclose is 10000 square feet.
Solve more problems on maximum area at : https://brainly.com/question/11906003
#SPJ4
Which expression is equivalent to -3 ( x - y )?
A. -3x + y
B. -3x - y
C. -3x - 3y
D. -3x + 3y
I already know it’s not A or B
Answers
Answer:
D. -3x+3y
Step-by-step explanation:
Start by distributing the -3 to each term in the parentheses:
-3(x)+(-3)(-y)
Here are some multiplication sign rules that will make this problem easier:
- - make a +
- + make a -
+ - make a -
+ + make a +
-3x+3y (note that since both 3 and y are negative, they make a positive)
We use _____ statistics to determine whether results from a sample can be generalized to a population.
Answers
To establish whether findings from a sample may be extrapolated to the entire population, we use inferential statistics.
What makes a sample different from the population, please?A population is the total group about which you want to reach conclusions. A sample is the specific group from which you will collect data. Always, the sample size is less than the whole population. The term "population" in research rarely refers to people. Using an office as an example, all of the employees would represent the Population, and all of the managers would represent the Sample. To create a comparison, picture your population as an ocean, and your sample as an aquarium.
Inferential statistics is a branch of statistics that uses data to draw inferences and generalizations about a certain situation or fact.
To learn more about sample and population, click here:
https://brainly.com/question/9295991
#SPJ4
Seth is comparing scenarios to determine which starting point he should get dropped off at to get him to school faster. Seth's house is 17 miles from school. In which scenario does seth start closer to school? in which scenario does seth travel at a greater speed, and what was the rate?.
Answers
In linear equation, He travels at a greater speed from his friend's house, at a rate of 0.6667 miles per minute, that is, 2 miles in 3 minutes.
What in mathematics is a linear equation?
A linear equation is a first-order (linear) term plus a constant in the algebraic form y=mx+b, where m is the slope and b is the y-intercept.
Sometimes, the aforementioned is referred to as a "linear equation of two variables," where x and y are the variables.
Using linear function concepts, it is found that:
Seth starts closer to the school in the bus.
He travels at a greater speed from his friend's house, at a rate of 0.6667 miles per minute, that is, 2 miles in 3 minutes.
From the graph of the bus, when he takes the bus, he is 13 miles from the school, as he starts at position 4 and ends at 17.
At his friend house, he is 13 miles from the school only after 3 minutes, which means that the bus will be closer.
The scenario with the greater speed is the one with the higher slope, that is, higher rate of change, which is the change in distance divided by the change in time.
In the bus, change of 13 miles in 24 minutes, thus
mᵇ = 13/24 = 0.5417
From the friend's house, change of 2 miles in 3 minutes, thus
mf = 2/3 = 0.6667
Thus, he travels at a greater speed from his friend's house, at a rate of 0.6667 miles per minute, that is, 2 miles in 3 minutes.
Learn more about linear equation
brainly.com/question/11897796
#SPJ4
What are Sternberg's 3 components to his triarchic theory of intelligence?
Answers
The three components to Triarchic Theory of Intelligence are practical intelligence, creative intelligence and analytical intelligence .
The Triarchic theory of Intelligence by Robert J. Sternberg states that there are three different types of intelligence: practical, creative , and analytical.
The three components are :
(i) Practical Intelligence -
having this type intelligence means we can find solutions that work in everyday life by applying knowledge based on experiences. Practical intelligence appears to be separate from traditional understanding of IQ .
(ii) Creative Intelligence -
This type of intelligence helps in inventing or imagining a solution to a problem or situation.
(iii) Analytical Intelligence -
This type of intelligence is defined as the ability to analyze, evaluate, judge, compare, and contrast .
Learn more about Triarchic Theory of Intelligence here
https://brainly.com/question/14884534
#SPJ4
ASHLEY IS GOING ON A TRIP TO LONDON. SHE SAVED $100.00 IN SPENDING MONEY. WHEN SHE ARRIVES IN ENGLAND, SHE GOES TO A BANK TO CHANGE HER MONEY INTO POUNDS. SHE IS TOLD THE EXCHANGE RATE IS 1 BRITISH POUND = 1.43 AMERICAN DOLLARS. THE BANK CHARGES A FEE OF 4 POUNDS TO CHANGE THE MONEY FROM DOLLARS TO POUNDS. HOW MUCH MONEY, IN BRITISH POUNDS, WILL ASHLEY HAVE IF SHE CHANGES ALL OF HER DOLLARS TO POUNDS
Answers
Answer:
65.93
Step-by-step explanation:
Okay, first lets convert the money.
100 ÷ 1.43 = 69.9300699301 (69.93)
69.93 - 4 = 65.93
Ashley has 65.93 pounds.
Hope this helps! Brainliest would be appreciated. :)
The number of British pounds when Ashley changes all of her dollars to pounds will be 65.93 pounds.
What is conversion?Conversion means to convert the same thing into different units.
Ashley is going on a trip to London.
She saved $100.00 in spending money.
When she arrives in England, she goes to a bank to change her money into pounds.
She is told the exchange rate is 1 British pound = 1.43 American dollars.
The bank charges a fee of 4 pounds to change the money from dollars to pounds.
Convert dollars into pounds. Then the total number of pounds will be
⇒ 100 / 1.43
⇒ 69.93 pounds
The number of British pounds when Ashley changes all of her dollars to pounds will be
⇒ 69.93 pounds - bank charges a fee
⇒ 69.93 - 4
⇒ 65.93 pounds
The number of British pounds when Ashley changes all of her dollars to pounds will be 65.93 pounds.
More about the conversion link is given below.
https://brainly.com/question/9414705
#SPJ2
Use the drop-down menus to complete the statements.
The ordered pair given in the first row in the table can
be written using function notation as
f(x) = -2 when x is

Answers
Answer:
f(-2)=1.
f(x)=-2 when x is 4
Answer:
f(-2)=1.
f(x)=-2 when x is 4
Step-by-step explanation:
find the value of the expression. (5+2)×(7-3)^2
Answers
In order to find the value of the expression, first let's calculate the operations inside the parenthesis:
\(\begin{gathered} (5+2)\cdot(7-3)^2_{} \\ =(7)\cdot(4)^2 \end{gathered}\)Then, calculating the exponential expression and after that the product, we have:
\(\begin{gathered} 7\cdot4^2 \\ =7\cdot16 \\ =112 \end{gathered}\)So the final value is 112.
find the equation of the line that passes through (-2,-3) and whose slope is 2/5
Answers
Answer:
y= mx+c
-3=2/5×-2+c
-3+4/5=c
c=-11/5
y=2/5x-11/5
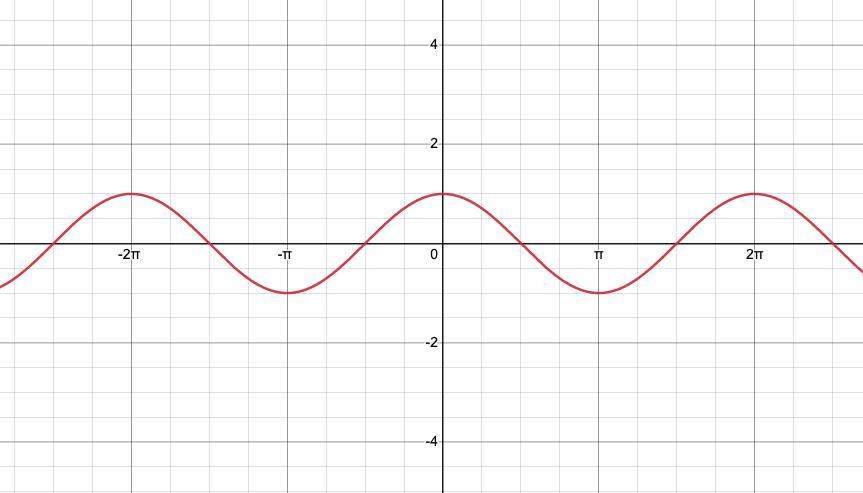
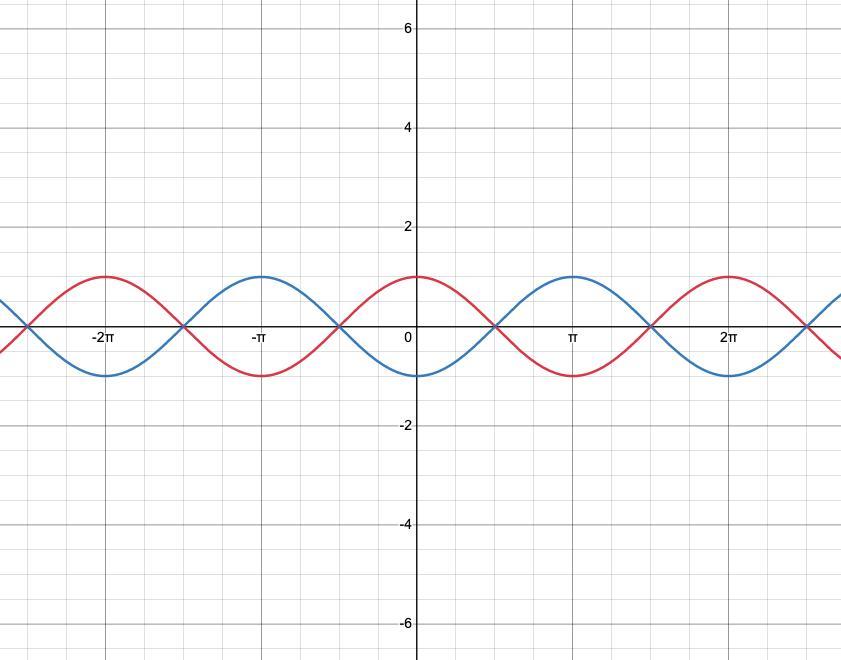
Draw the graph of -3 times f(x) where f(x)=cosx And give it's period
Answers
It is required to draw the graph of -3f(x), where f(x)=cos x.
It is also required to give its period.
To do this, start with the graph of the parent function f(x)=cos x, then perform transformations on the graph of the parent function to draw the required graph.
The graph of the parent function f(x)=cos x is shown below:
Reflect the graph over the x-axis to draw the graph of -f(x) (in blue):
Stretch the graph of -f(x) vertically by a factor of 3 to draw the graph of -3f(x) (in green):
The required graph of -3f(x) is:
Recall that the period of a sinusoid is the length of a complete cycle.
From the graph, the period is 2π.


A sail is shaped like an isosceles
triangle. The short side is 9 ft
long and is half the length of
each longer side. The sail has
holes for rope spaced 1 ft apart
around its perimeter. How many
holes are there? PLEASE ANSWER ASAP!!!
Answers
Answer: 45 holes
Step-by-step explanation:
If the short side is half the length of each longer side, then the longer sides are 18 ft long (9 ft * 2).
Let us find the perimeter of the triangle:
9 ft + 18 ft + 18 ft = 45 ft
Now, there is a hole every 1 ft.
45 ft / 1 ft = 45 holes

a
b
c
whuch is the answer look at the picture
d

Answers
Answer:
Step-by-step explanation:

15.6 ÷0.24 In long division form
Answers
Ethan works in a bakery and makes cakes. Yesterday he made one cake and used 1/4 cup of milk in order to make the Batter. If ethan needs make 3 times as many cakes today how much milk we he need
Answers
Ethan would need to use 3/4 cups of milk to make three cakes today.
If Ethan needs to make three times as many cakes today, he would need to use 3 times as much milk as he did yesterday. Since he used 1/4 cup of milk to make one cake yesterday, he would need to use 3/4 cups of milk to make three cakes today.
To break it down, if Ethan made one cake yesterday using 1/4 cup of milk, he would use 1/4 cup of milk for each cake he makes. If he needs to make three cakes today, he would need to use 1/4 cup of milk for each cake, which adds up to 3/4 cups of milk in total.
Therefore, Ethan would need 3/4 cups of milk to make three cakes today, which is three times the amount of milk he used yesterday for one cake.
More on milk: https://brainly.com/question/29003251
#SPJ11
A fossil contains 18% of the carbon-14 that the organism contained when it was alive. Graphically estimate its age. Use 5700 years for the half-life of the carbon-14.
Answers
Graphically estimating the age of the fossil with 18% of the original carbon-14 content involves determining the number of half-lives that have passed. Therefore, the fossil is estimated to be between 11400 and 17100 years old.
Since the half-life of carbon-14 is 5700 years, we can divide the remaining carbon-14 content (18%) by the initial amount (100%) to obtain 0.18. Taking the logarithm base 2 of 0.18 gives us approximately -2.5146.
In the graph, we can plot the ratio of remaining carbon-14 to the initial amount on the y-axis, and the number of half-lives on the x-axis. The value of -2.5146 lies between -2 and -3 on the x-axis, indicating that the fossil is between 2 and 3 half-lives old.
Since each half-life is 5700 years, multiplying the number of half-lives by the half-life period gives us the age estimate.
to learn more about number click here:
brainly.com/question/30752681
#SPJ11
What is the function graphed below?
O y= x + 0.5
O y= 2 x + 0.5
O y=x
O y=(0.5)

Answers
Answer:
A. y= x + 0.5Step-by-step explanation:
We see that:
y- intercept is b = 0.5, point (0, 0.5) Rate of change is m = 1 as each next value of y is greater by 1.The function is:
y = mx + by = x + 0.5Correct choice is A
Please help and show work only do the left side

Answers
Writing linear equations given two points can be a useful skill when graphing linear equations.
Write a linear equation that passes through the given two points?To write a linear equation given two points, you need to first find the slope of the line. You can do this by finding the change in the y-value and dividing it by the change in the x-value. From there, you can use the slope to solve for the y-intercept and write the equation.For example, if the two points are (3, 4) and (0,5), you would find the slope by calculating the change in the y-value (5-4 = 1) and dividing it by the change in the x-value (0-3 = -3).The slope would then be 1/-3, which can be simplified to -1/3. To solve for the y-intercept, you can plug in one of the points and solve for b. In this case, you would plug in (3, 4) and solve for b, giving you b = 5. Now that you have the slope and y-intercept, you can write the equation as y = -1/3x + 5.y = -1/2xy = 5/3x + 5/3y = 3/5x + 1y = -1/2x - 2y = 6/5x + 5y = -4/4x - 8y = -3/5x - 7/5y = -2x - 4y = 5/6x + 7y = -1/2x + 4To learn more about linear equation refer to:
https://brainly.com/question/2030026
#SPJ1
If you could answer both, it’ll be very appreciated (15 points if answered)

Answers
Answer: 1) 2x + 1 = 3
2) 8 + x = 7
Step-by-step explanation:
1) x would be equal to 1
2) x would be equal to -1
please give me brainliest or at least a ty!
the angle \theta 1θ 1 theta, start subscript, 1, end subscript is located in quadrant \text{iii}iiistart text, i, i, i, end text, and \sin(\theta 1)
Answers
The value of the angle θ₁, located in Quadrant IV with sin(θ₁) = -13/85, is such that cosθ₁ = 84/85.
In the given scenario, the angle θ₁ is located in Quadrant IV and sin(θ₁) is given as -13/85. We can use the trigonometric identity to determine the value of cosθ₁.
Using the Pythagorean identity sin²θ + cos²θ = 1, we can substitute sin(θ₁) = -13/85:
(-13/85)² + cos²θ₁ = 1
Simplifying:
169/7225 + cos²θ₁ = 1
cos²θ₁ = 1 - 169/7225
cos²θ₁ = (7225 - 169)/7225
cos²θ₁ = 7056/7225
Taking the square root of both sides, we get:
cosθ₁ = √(7056/7225)
Since the angle θ₁ is located in Quadrant IV where cosθ₁ is positive, the value of cosθ₁ is:
cosθ₁ = √(7056/7225)
Simplifying the square root:
cosθ₁ = 84/85
Therefore, the value of the angle cosθ₁ is 84/85.
Learn more about trigonometric identity here: https://brainly.com/question/31484998
#SPJ11
The complete question is:
The angle θ₁ is located in Quadrant IV and sin(θ₁) = -13/85. What is the value of the angle cosθ₁?
In your reservoir, you have a production well which flows for 48 hours at 200 STB/day, and then shut-in for 24 hours. The following additional data are given : Pi = 3100 psi Ct = 15x10^-6 psi^-1 Bo = 1.3 bbl/STB ϕ = 15% μ=1.2 cp K = 45 md and h = 60 ft
a-) Calculate the pressure in this production well at 12 hours of shut in
b-) Explain how can you use superposition in time to analyze a pressure build-up test.
Answers
a) To calculate the pressure at 12 hours of shut-in:
substitute the given values into the pressure buildup equation and solve for P(t=12).
b) Superposition in time is used in pressure buildup analysis by adding or summing the responses of multiple transient tests to analyze and interpret reservoir behavior and properties.
We have,
a) To calculate the pressure in the production well at 12 hours of a shut-in, we can use the equation for pressure transient analysis during shut-in periods, known as the pressure buildup equation:
P(t) = Pi + (Q / (4πKh)) * log((0.14ϕμCt(t + Δt)) / (Bo(ΔP + Δt)))
Where:
P(t) = Pressure at time t
Pi = Initial reservoir pressure
Q = Flow rate
K = Permeability
h = Reservoir thickness
ϕ = Porosity
μ = Viscosity
Ct = Total compressibility
t = Shut-in time (12 hours)
Δt = Time since the start of the flow period
Bo = Oil formation volume factor
ΔP = Pressure drop during the flow period
Given:
Pi = 3100 psi
Q = 200 STB/day
K = 45 md
h = 60 ft
ϕ = 15%
μ = 1.2 cp
Ct = 15x10^-6 psi^-1
Bo = 1.3 bbl/STB
t = 12 hours
Δt = 48 hours
ΔP = Pi - P(t=Δt) = Pi - (Q / (4πKh)) * log((0.14ϕμCt(Δt + Δt)) / (Bo(ΔP + Δt)))
Substituting the given values into the equation:
ΔP = 3100 - (200 / (4π * 45 * 60)) * log((0.14 * 0.15 * 1.2 * 15x\(10^{-6}\) * (48 + 48)) / (1.3 * (3100 - (200 / (4π * 45 * 60)) * log((0.14 * 0.15 * 1.2 * 15 x \(10^{-6}\) * (48 + 48)) / (1.3 * (0 + 48))))))
After evaluating the equation, we can find the pressure in the production well at 12 hours of shut-in.
b) Superposition in time is a principle used in pressure transient analysis to analyze and interpret pressure build-up tests.
It involves adding or superimposing the responses of multiple transient tests to simulate the pressure behavior of a reservoir.
The principle of superposition states that the response of a reservoir to a series of pressure changes is the sum of the individual responses to each change.
Superposition allows us to combine the information obtained from multiple tests and obtain a more comprehensive understanding of the reservoir's behavior and properties.
It is a powerful technique used in reservoir engineering to optimize production strategies and make informed decisions regarding reservoir management.
Thus,
a) To calculate the pressure at 12 hours of shut-in:
substitute the given values into the pressure buildup equation and solve for P(t=12).
b) Superposition in time is used in pressure buildup analysis by adding or summing the responses of multiple transient tests to analyze and interpret reservoir behavior and properties.
Learn more about equations here:
https://brainly.com/question/17194269
#SPJ12
Paula bought a ski jacket on sale for $8 less than half its original price. She paid $84 for the jacket. What was the original price?
Answers
The original price of the ski jacket is $184.
What is price?
The sum of money paid or the amount of compensation offered by one party to another in exchange for goods or services is known as a price. The cost of production can take on different names depending on the circumstance. If the item is a "good" in a commercial transaction, the cost of the item will probably be referred to as its "price".
Given:
Paula bought a ski jacket on sale for $8 less than half its original price.
She paid $84 for the jacket.
We have to find the original price of the ski jacket.
Let
The original price = x
The amount she bought it = x/2 - 8
She paid $84 for the jacket
Therefore,
84 = x/2 - 8
Add 8 on both sides,
84 + 8 = x/2 - 8 + 8
92 = x/2
x = 184
Hence, the original price of the ski jacket is $184.
To know more about price, click on the link
https://brainly.com/question/11938988
#SPJ1
how many degrees does a unit angle measure a 10° B 90° c 180 degrees d 100 degrees
Answers
Sjhhzjz
pls pls help whoever gets it right gets marked brainliest

Answers
Answer:
13 units
Step-by-step explanation:
\(d=√((x_2-x_1)²+(y_2-y_1)²) \)
\( \sqrt{( - 2 - ( -14)^{2} } + ( - 4 - 1) {}^{2} \)
\( \sqrt{12 {}^{2} } + ( - 5) {}^{2} \)
\( \sqrt{169} \)
= 13 Units1. the motherboard has connections for ram, expansion cards, and external i/o devices.
Answers
Answer:
The motherboard acts as a central hub, providing the necessary connections and pathways for communication between different hardware components in a computer system.
Step-by-step explanation:
The motherboard serves as the main circuit board in a computer system and provides connections for various components. Some of the key connections found on a motherboard include:
1. RAM Slots: These are the slots where the computer's memory modules (RAM) are installed. The motherboard determines the type and maximum capacity of RAM that can be supported.
2. Expansion Slots: These slots allow for the installation of expansion cards, such as graphics cards, sound cards, network cards, and other add-on components. The type and number of expansion slots vary depending on the motherboard model.
3. I/O Ports: The motherboard features various input/output (I/O) ports that allow for connectivity with external devices. This includes USB ports, audio jacks, Ethernet ports, HDMI or DisplayPort connectors, and more. These ports facilitate communication between the computer and peripherals.
4. Power Connectors: The motherboard has power connectors to receive power from the computer's power supply unit (PSU). These connectors ensure that the motherboard and its components receive the necessary power to operate.
5. CPU Socket: The motherboard contains a specific socket where the computer's central processing unit (CPU) is installed. The socket type must match the CPU being used for compatibility.
Overall, the motherboard acts as a central hub, providing the necessary connections and pathways for communication between different hardware components in a computer system.
Learn more about RAM:https://brainly.com/question/28483224
#SPJ11
In a company, 85% of the workers are women. If 390 people work for the company who aren't women, how many workers are there in all?
Answers
Answer:
2210
Step-by-step explanation:
390 =15%
390*3 = 130=5%
130*17=2210
Consider the function shown. How can you restrict the domain so that f(x) has an inverse? What is the equation of the inverse function?
Answers
The correct option is D f⁻¹(x) = 2 + √(x + 2).
What is a function?A function is defined as the expression that set up the relationship between the dependent variable and independent variable.
The inverse function of a function f in mathematics is a function that reverses the operation of f.
The given function is f(x) = 1 / (2 + √(x + 2)). The inverse function of the given function is,
f⁻¹(x) = 2 + √( x + 2).
Therefore, the correct option is D f⁻¹(x) = 2 + √( x + 2 ).
To know more about a function follow
brainly.com/question/3831584
#SPJ1
logarithm question please!

Answers
The solution for x is x = (log₅(2) - 3) / 5.
To solve the equation log₂((5x + 3)²) - log₍₅ₓ₊₃₎ 2 = 1, we can simplify it using logarithmic properties.
First, we can combine the two logarithms using the quotient rule of logarithms:
log₂(((5x + 3)²) / log₅(2) = 1.
Next, we can convert the equation to exponential form:
2¹ = ((5x + 3)²) / log₅(2).
2 = ((5x + 3)²) / log₅(2).
2 . log₅(2) = (5x + 3)².
log₅(2²) = (5x + 3)².
Using the property logₐ(b²) = 2 * logₐ(b), we have:
2 . log₅(2) = 2 * (5x + 3).
log₅(2) = 5x + 3.
Finally, we isolate x by subtracting 3 and dividing by 5:
log₅(2) - 3 = 5x.
x = (log₅(2) - 3) / 5.
So, the solution for x is x = (log₅(2) - 3) / 5.
Learn more about Logarithm here:
https://brainly.com/question/30226560
#SPJ1
Which system of linear equations can be solved using the information below?-314.1--2014-1-22 208-192|Ax| ==

Answers
Given
\(\begin{gathered} |A_x|=det\begin{bmatrix}{20} & {-3} \\ {-192} & {8}\end{bmatrix} \\ |A_y|=det\begin{bmatrix}{2} & {20} \\ {12} & {-192}\end{bmatrix} \end{gathered}\)To find:
The system of equation.
Explanation:
It is given that,
\(\begin{gathered} |A_x|=det\begin{bmatrix}{20} & {-3} \\ {-192} & {8}\end{bmatrix} \\ |A_y|=det\begin{bmatrix}{2} & {20} \\ {12} & {-192}\end{bmatrix} \end{gathered}\)That implies,
Since,
\(\begin{gathered} |A_x|=det\begin{bmatrix}{20} & {-3} \\ {-192} & {8}\end{bmatrix} \\ |A_y|=det\begin{bmatrix}{2} & {20} \\ {12} & {-192}\end{bmatrix} \end{gathered}\)Then,
\(AX=B\Rightarrow\begin{bmatrix}{2} & {-3} \\ {12} & {8}\end{bmatrix}\begin{bmatrix}{x} & {} \\ {y} & {}\end{bmatrix}=\begin{bmatrix}{20} & {} \\ {-192} & \end{bmatrix}\)Therefore,
The system of equation is,
\(\begin{gathered} 2x-3y=20 \\ 12x+8y=-192 \end{gathered}\)Hence, the answer is option A).